Math Antics - Circles, Circumference And Area

mathantics
25 Jul 201407:55
EducationalLearning
32 Likes 10 Comments

TLDRIn this Math Antics video, viewers learn to calculate the circumference and area of a circle using the fundamental ratio Pi. The script introduces two key formulas: Circumference (C = Pi Γ— d) and Area (A = Pi Γ— rΒ²), emphasizing the importance of memorizing them. It clarifies the difference between squaring the radius for area and doubling it for circumference, using a practical example with an 8-meter radius circle. The video also demonstrates real-world applications, such as calculating Earth's circumference at the equator and a pizza's area, encouraging practice to solidify understanding.

Takeaways
  • πŸ“š Pi is a special ratio used in calculating the circumference and area of a circle.
  • πŸ” Memorizing the formulas for circumference and area is important for solving circle-related problems.
  • πŸ”’ The formula for circumference is C = Pi Γ— d, where 'C' stands for circumference and 'd' for diameter.
  • πŸ“ The formula for area is A = Pi Γ— rΒ², using 'A' for area and 'r' for radius, with 'rΒ²' indicating the radius squared.
  • πŸ€” Squaring the radius means multiplying the radius by itself, which is different from doubling the radius.
  • 🚫 A common mistake is confusing rΒ² with 2r; they are not the same and lead to different results.
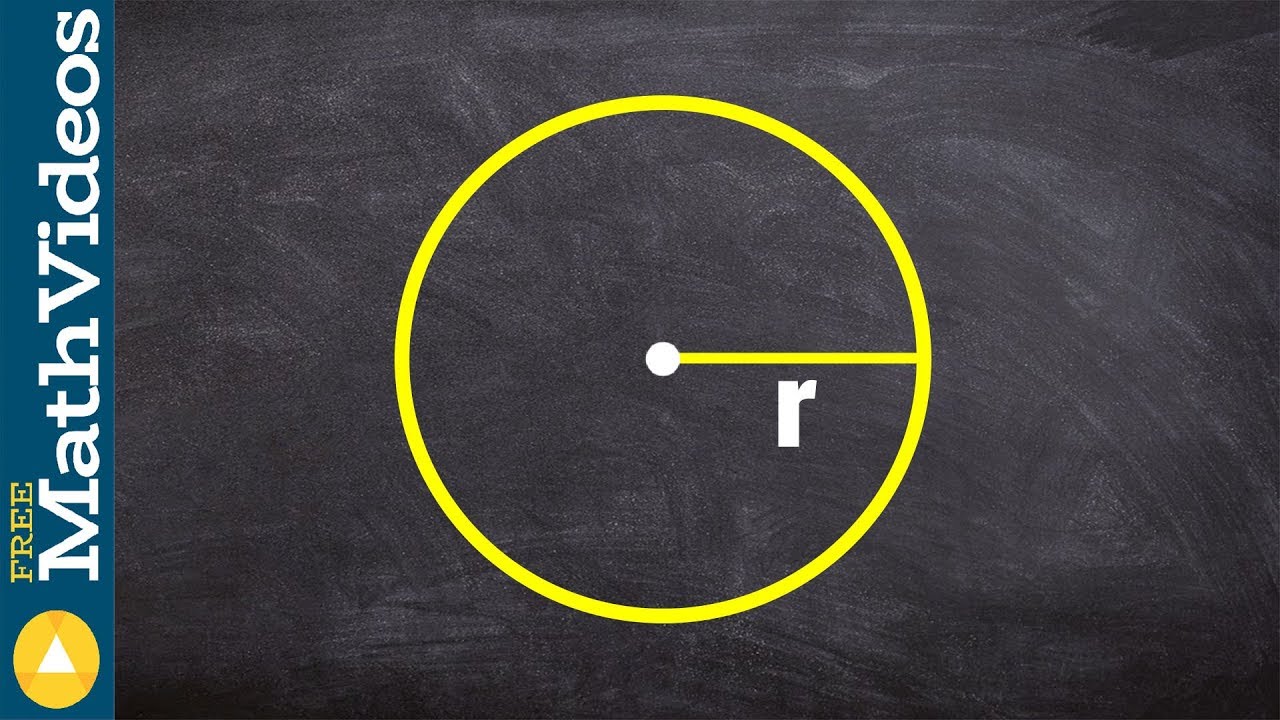
  • πŸ”„ The relationship between radius and diameter is crucial: diameter is 2 times the radius.
  • 🌐 The formula for circumference can also be written as C = Pi Γ— 2 Γ— r, showing the connection between radius and diameter.
  • πŸ“ To find the circumference, you double the radius and multiply by Pi; for area, you square the radius and multiply by Pi.
  • 🌍 Real-world examples include calculating the circumference of the Earth at the equator and the area of a pizza.
  • πŸ“ Practice using the formulas is essential for mastering the concepts of circle circumference and area calculation.
Q & A

  • What is the special ratio called Pi used for in the context of circles?

    -Pi is a mathematical constant used to calculate the circumference and area of a circle.

  • What is the formula for calculating the circumference of a circle?

    -The formula for the circumference of a circle is C = Pi Γ— d, where 'C' stands for circumference and 'd' for diameter.

  • What does the abbreviation 'd' represent in the circumference formula?

    -The abbreviation 'd' represents the diameter of the circle.

  • What is the formula for calculating the area of a circle?

    -The formula for the area of a circle is A = Pi Γ— rΒ², where 'A' stands for area and 'r' for radius.

  • What does 'r squared' mean in the area formula?

    -'r squared' means the radius of the circle multiplied by itself (r Γ— r).

  • Why is it a common mistake to confuse 2 times r with r squared?

    -It's a common mistake because both involve the radius, but they represent different mathematical operations: doubling for circumference and squaring for area.

  • What is the relationship between the radius and the diameter of a circle?

    -The diameter of a circle is twice the length of its radius.

  • How can the formula for circumference be rewritten using the radius?

    -The formula for circumference can be rewritten as C = Pi Γ— 2 Γ— r, using the fact that the diameter is twice the radius.

  • What is the difference between the units of circumference and area?

    -The units of circumference are 1-dimensional (like meters), while the units of area are 2-dimensional (like square meters).

  • Can you provide an example of how to find the circumference of a circle with a radius of 8 meters?

    -First, double the radius to find the diameter (16 meters), then multiply by Pi (3.14) to get the circumference, which is 50.24 meters.

  • How do you calculate the area of a circle with a radius of 8 meters?

    -Square the radius (64 square meters) and then multiply by Pi (3.14) to get the area, which is 200.96 square meters.

  • What is the diameter of the Earth, and how can you find its circumference?

    -The diameter of the Earth is about 12,750 km. To find the circumference, multiply the diameter by Pi (3.14159), resulting in approximately 40,055 km.

  • If a pizza has a diameter of 24 inches, how do you calculate its total area?

    -First, find the radius by dividing the diameter by 2 (12 inches), then square the radius (144 square inches), and multiply by Pi (3.14) to get the area, which is 452.16 square inches.

Outlines
00:00
πŸ“š Introduction to Circle Calculations

This paragraph introduces the video's focus on using the mathematical constant Pi to calculate the circumference and area of a circle. The presenter emphasizes the importance of memorizing the formulas for circumference (C = Pi Γ— d) and area (A = Pi Γ— rΒ²), explaining the meaning of 'squaring' the radius and the difference between squaring and doubling it. The relationship between the radius and diameter is also highlighted, with an example provided to demonstrate the calculation process using a circle with a radius of 8 meters.

05:04
🌐 Real-World Applications of Circle Formulas

The second paragraph delves into real-world applications of the formulas introduced in the first paragraph. It starts with an example of calculating the circumference of the Earth at the equator, using its diameter and a more precise value of Pi. The presenter then moves on to a pizza example, illustrating how to find the area of a circle when given the diameter, by first determining the radius and then squaring it. The importance of practicing these formulas is stressed, and the video concludes with a reminder of the formulas for circumference and area, along with a prompt to visit the Math Antics website for more information.

Mindmap
Keywords
πŸ’‘Pi (Ο€)
Pi (Ο€) is a mathematical constant approximately equal to 3.14159, representing the ratio of a circle's circumference to its diameter. In the video, Pi is fundamental in calculating both the circumference and area of a circle. For example, the circumference formula uses Pi multiplied by the diameter, while the area formula uses Pi multiplied by the radius squared.
πŸ’‘Circumference
The circumference is the distance around the edge of a circle. It's a 1-dimensional measure, like the perimeter of a shape. The video explains that to find the circumference of a circle, you multiply Pi by the circle's diameter. For instance, the circumference of a circle with a diameter of 16 meters is calculated as 16 Γ— 3.14, giving a circumference of 50.24 meters.
πŸ’‘Diameter
The diameter of a circle is the distance across the circle through its center, or twice the length of the radius. It is used in the formula for finding the circumference. In the script, the diameter is crucial for converting to circumference, and an example given is the Earth's diameter, which is 12,750 km, used to calculate its circumference.
πŸ’‘Radius
The radius is the distance from the center of a circle to any point on its perimeter. It is half the diameter. The video emphasizes its importance in calculating the area of a circle, where you square the radius and then multiply by Pi. For example, a radius of 8 meters results in an area calculation using 8 squared, which is 64, multiplied by Pi.
πŸ’‘Area
The area of a circle is the space contained within its perimeter, measured in square units. The formula for area is Pi times the radius squared. The video illustrates this by showing how squaring the radius of 8 meters gives 64, and then multiplying by Pi results in an area of 200.96 square meters.
πŸ’‘Square (squared)
To square a number means to multiply it by itself. In the context of the video, squaring the radius is essential for calculating the area of a circle. For instance, squaring the radius of 8 meters (8 Γ— 8) gives 64. The video highlights that squaring is different from doubling, which is a common point of confusion.
πŸ’‘1-dimensional vs. 2-dimensional
1-dimensional refers to measurements in one direction, such as length or circumference. 2-dimensional involves measurements with both length and width, like area. The video explains that circumference is a 1-dimensional measure since it is just a length around a circle, while area is 2-dimensional because it covers the surface inside a circle.
πŸ’‘Real-world examples
The video uses practical examples to explain the concepts, like calculating the circumference of the Earth using its diameter, or the area of a pizza based on its diameter. These examples help illustrate how the formulas for circumference and area are applied in everyday situations.
πŸ’‘Formulas
Formulas are mathematical expressions that show how to calculate specific quantities. In the video, two key formulas are introduced: C = Ο€ Γ— d for the circumference of a circle and A = Ο€ Γ— rΒ² for the area. Understanding these formulas is essential for solving problems related to circles.
πŸ’‘Memorization and Practice
The video stresses the importance of memorizing the formulas for circumference and area, and practicing using them to solve problems. This approach helps reinforce the concepts and ensures a deeper understanding. The script encourages viewers to engage with exercises to solidify their grasp of the material.
Highlights

Introduction to the video on using Pi to calculate the circumference and area of a circle.

Memorization of the formulas for circumference and area is emphasized for their importance.

The formula for circumference is presented as C = Pi Γ— d.

The formula for area is presented as A = Pi Γ— rΒ², with an explanation of squaring the radius.

Clarification that squaring the radius is not the same as doubling it, a common mistake among students.

The relationship between the radius and diameter is highlighted, with diameter being 2 times the radius.

Rewriting the circumference formula to emphasize the relationship between radius and diameter.

Demonstration of calculating the circumference of a circle with a radius of 8 meters.

Demonstration of calculating the area of the same circle, emphasizing the difference between squaring and doubling the radius.

Explanation of the difference in units between 1-dimensional (circumference) and 2-dimensional (area) quantities.

Real-world example of calculating the circumference of the Earth at the equator using its diameter.

Real-world example of calculating the area of a pizza using its diameter.

Instruction on converting diameter to radius for area calculation in the pizza example.

Final calculation of the pizza's area in square inches.

Reiteration of the key formulas for circumference and area, urging practice for better understanding.

Closing remarks with a prompt to visit www.mathantics.com for more information.

Transcripts

Browse More Related Video

Math Antics - Circles, What Is PI?

Quick Visual Proof: Area of a Circle

Rates-of-change (part 2)

How to find the radius when the area and circumference are changing at the same rate

What are radians? Simply explained

Circles: Radius, Diameter, Chords, Circumference, and Sectors

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Circle FormulasCircumferenceArea CalculationMathematicsEducational VideoPi RatioRadius SquaredDiameterGeometryMath AnticsReal-World Examples