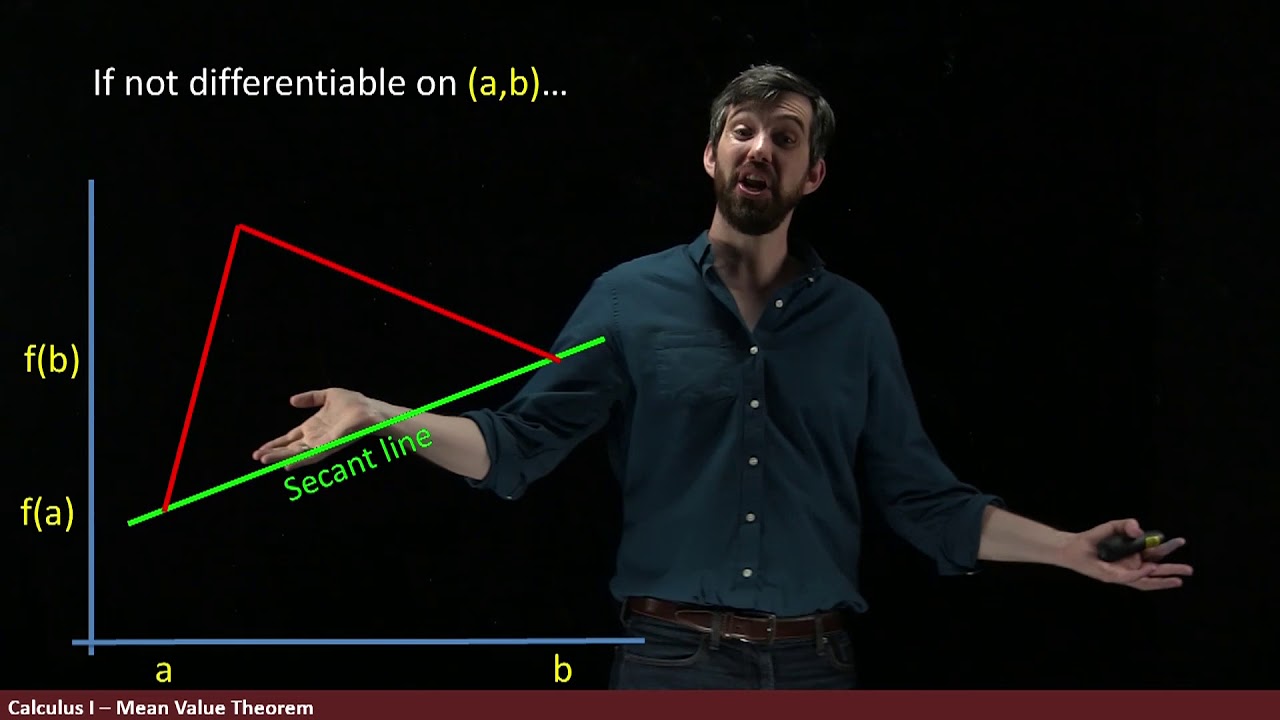
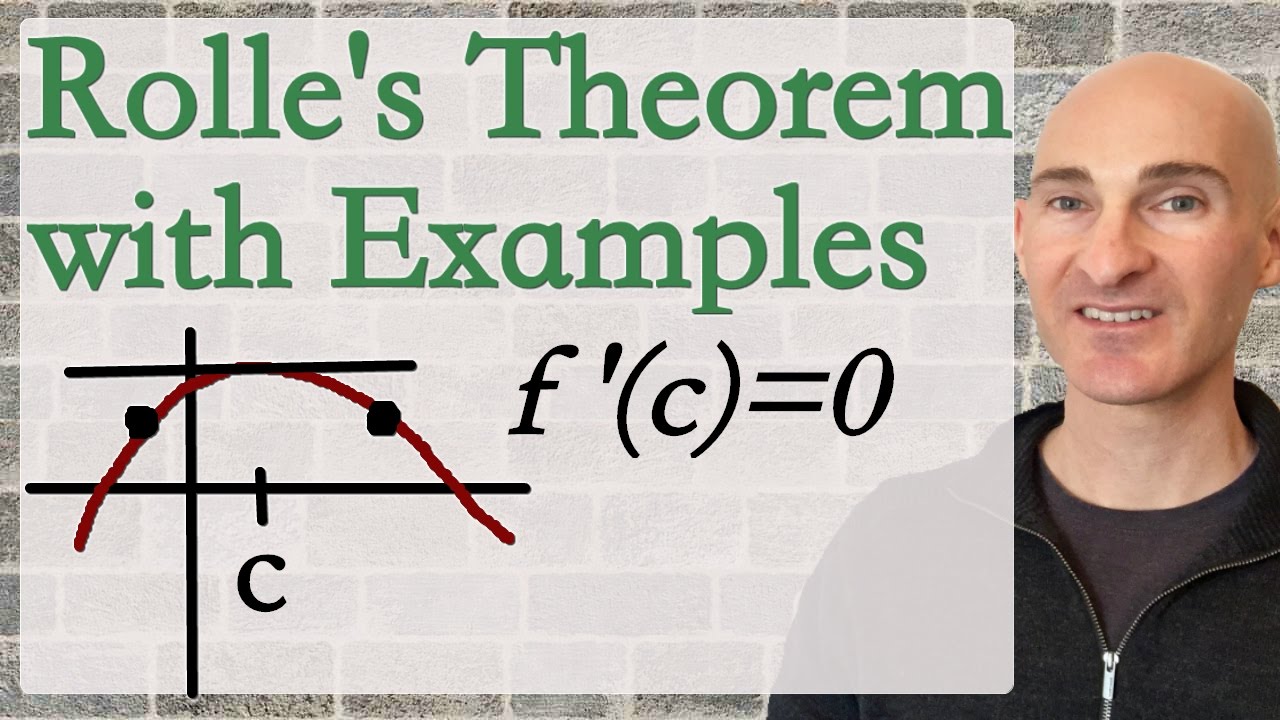
Rolle’s Theorem Proof
TLDRThe video script explores Rolle's Theorem, a foundational principle in calculus that is instrumental in proving the Mean Value Theorem. It posits that for a continuous function on a closed interval [a, b] with equal endpoints, there exists at least one point where the derivative is zero, indicating a horizontal tangent. The script delves into the proof using the Extreme Value Theorem and Fermat's Theorem, illustrating how a maximum or minimum within the interval necessitates a zero derivative at that point. This mathematical exposition is aimed at viewers interested in a deeper understanding of calculus.
Takeaways
- 📚 The video discusses Rolle's Theorem, a foundational concept in calculus used to prove the Mean Value Theorem.
- 🔍 Rolle's Theorem applies to continuous functions on a closed interval [a, b] that are differentiable within the interval and have equal function values at the endpoints (f(a) = f(b)).
- 📌 It states that there must be at least one point 'c' in the open interval (a, b) where the derivative of the function, f'(c), equals zero, indicating a horizontal tangent line.
- 🔑 The theorem is proven by using the Extreme Value Theorem, which guarantees the existence of a maximum or minimum for continuous functions on a compact interval.
- 📈 If the maximum and minimum values of the function are equal, the function is constant, and its derivative is zero everywhere, satisfying Rolle's condition.
- 🔄 The proof assumes that the maximum and minimum are not equal, implying at least one extreme value must be strictly inside the interval (a, b), not at the endpoints.
- 📊 By Fermat's Theorem, if a function has a maximum at a point 'c' in the open interval (a, b), then the derivative at that point, f'(c), is zero.
- 🤔 The proof involves taking limits from the right and left to show that f'(c) must be less than or equal to zero and greater than or equal to zero, thus concluding f'(c) = 0.
- 📐 The video emphasizes the importance of the function's continuity on a closed and finite interval for the application of these theorems.
- 📝 The script clarifies that the condition f(a) = f(b) is crucial for Rolle's Theorem, as it ensures an extremum within the interval, which is not guaranteed otherwise.
- 🎓 The video aims to provide a clear understanding of Rolle's Theorem and its proof, encouraging viewers interested in mathematics to subscribe for more content.
Q & A
What is Rolle's Theorem?
-Rolle's Theorem is a special case of the Mean Value Theorem used to prove it. It states that if a function is continuous on a closed interval [a, b], differentiable on the open interval (a, b), and f(a) = f(b), then there exists at least one c in (a, b) such that the derivative of the function at c, f'(c), is equal to zero.
What is the significance of Rolle's Theorem in finding critical points?
-Rolle's Theorem provides a neat way of finding critical points of a function. It guarantees the existence of at least one point where the derivative of the function is zero, indicating a horizontal tangent line, which corresponds to a local maximum or minimum.
Why is it necessary for the function to be continuous on a closed interval in Rolle's Theorem?
-The continuity of the function on a closed interval [a, b] is crucial because it allows the use of the Extreme Value Theorem, which states that a continuous function on a closed and bounded interval attains both a maximum and a minimum.
What is the Extreme Value Theorem and how is it related to Rolle's Theorem?
-The Extreme Value Theorem states that if a function is continuous on a closed interval, it must attain both a maximum and a minimum on that interval. Rolle's Theorem relies on this theorem to ensure that there is at least one maximum or minimum inside the interval, where the derivative can be zero.
What is the condition for a function to have a maximum or minimum at a point inside an interval?
-For a function to have a maximum or minimum at a point inside an interval, it must be differentiable at that point. Additionally, the function's derivative at that point must be zero, indicating a horizontal tangent line.
Why is it assumed that the maximum and minimum values are not equal in Rolle's Theorem?
-If the maximum and minimum values are equal, it implies that the function is constant between the interval endpoints. Since the derivative of a constant function is zero everywhere, Rolle's Theorem would be trivially true, and the assumption helps to focus on the non-trivial cases where the function changes within the interval.
What is the relationship between the endpoints of the interval and the existence of a maximum or minimum inside the interval?
-If the maximum and minimum values of the function are attained at the endpoints, then there is no maximum or minimum inside the interval. However, if the function's values at the endpoints are equal, it guarantees the existence of at least one maximum or minimum inside the interval.
What is the role of the Mean Value Theorem in proving Rolle's Theorem?
-The Mean Value Theorem is not directly used to prove Rolle's Theorem. Instead, Rolle's Theorem is a special case of the Mean Value Theorem and is often used as a stepping stone to understand and prove the Mean Value Theorem itself.
What is the difference between Rolle's Theorem and Fermat's Theorem?
-While both theorems deal with the derivative being zero at a point of maximum or minimum, Fermat's Theorem specifically applies to functions that have a maximum or minimum at a critical point, without the requirement that the function's values at the endpoints of an interval be equal.
How does the proof of Rolle's Theorem demonstrate that the derivative at a maximum point is zero?
-The proof shows that the limit defining the derivative at a maximum point, as h approaches zero from both the right and the left, results in a ratio that is less than or equal to zero and greater than or equal to zero, respectively. Combining these inequalities, it concludes that the derivative at the maximum point must be zero.
Why is it important to approach the limit from both the right and the left in the proof of Rolle's Theorem?
-Approaching the limit from both directions ensures that the derivative's value is consistent regardless of the direction from which the limit is taken. This consistency is necessary to conclude that the derivative at the point of maximum (or minimum) is indeed zero.
Outlines
📚 Introduction to Rolle's Theorem and Its Significance
The video script begins with an introduction to Rolle's Theorem, which is a precursor to the Mean Value Theorem. The theorem states that if a function is continuous on a closed interval [a, b], differentiable on the open interval (a, b), and its values at the endpoints are equal (f(a) = f(b)), then there exists at least one point c in (a, b) where the derivative of the function is zero (f'(c) = 0). This is a critical concept for identifying critical points and is proven by reducing it to Fermat's Theorem, which deals with the existence of a maximum or minimum within an interval.
🔍 Rolle's Theorem Proof Using Fermat's Theorem
The script proceeds to explain the proof of Rolle's Theorem by assuming that the function has a maximum or minimum within the interval. It uses the Extreme Value Theorem to establish that a continuous function on a closed and finite interval must have a maximum and a minimum. If these maximum and minimum values are equal, the function is constant, and its derivative is zero everywhere. If not, one of the extreme values must be strictly inside the interval. The script then introduces Fermat's Theorem, which states that if a function has a maximum or minimum at a point within an interval, the derivative at that point is zero. The proof involves taking the limit of the function's values as h approaches zero from both the right and the left, leading to the conclusion that the derivative at the point of maximum or minimum is zero.
📉 Demonstrating the Derivative at Maximum or Minimum Equals Zero
In the final paragraph, the script delves into the specifics of proving that the derivative at the point of maximum or minimum equals zero. It uses the definition of the derivative as the limit of (f(c+h) - f(c))/h as h approaches zero. By comparing the values of the function at c and c+h, and considering the nature of a maximum, it is shown that the ratio is less than or equal to zero when h approaches from the right. Similarly, for h approaching from the left, the ratio is shown to be greater than or equal to zero. Combining these two inequalities, it is concluded that the derivative at the point of maximum or minimum must be zero, thus completing the proof of Rolle's Theorem.
Mindmap
Keywords
💡Rolle's Theorem
💡Mean Value Theorem
💡Critical Points
💡Differentiable
💡Continuous
💡Extreme Value Theorem
💡Compact Interval
💡Maximum and Minimum
💡Fermat's Theorem
💡Tangent Line
💡Derivative
Highlights
Introduction to Rolle's Theorem as a special case of the Mean Value Theorem.
Rolle's Theorem states that if a function has equal beginning and ending values, there must be a point where its derivative is zero.
This theorem is useful for finding critical points where the tangent line is horizontal.
The proof of Rolle's Theorem involves reducing it to Fermat's Theorem.
If a function has the same starting and ending points and is not constant, it must have a maximum or minimum within the interval.
The Extreme Value Theorem is used to establish the existence of a maximum and minimum for continuous functions on a closed interval.
If the maximum and minimum are not equal, at least one extreme must be strictly inside the interval.
If the maximum is at the endpoints, the minimum must be inside the interval, and vice versa.
Fermat's Theorem is introduced, stating that if a function has a maximum or minimum on an open interval, its derivative at that point is zero.
A proof of Fermat's Theorem is provided, using the definition of the derivative and the concept of a strict maximum.
The proof involves comparing the function values at the point of maximum and nearby points as the variable approaches zero from both sides.
The derivative at the point of maximum (or minimum) must be less than or equal to zero when approaching from the right.
Similarly, the derivative must be greater than or equal to zero when approaching from the left, leading to the conclusion that the derivative is zero.
The necessity of having the function's maximum or minimum strictly inside the interval is explained to ensure the applicability of Fermat's Theorem.
Rolle's Theorem is confirmed to be true for continuous functions on an interval where the endpoints are equal.
The importance of the condition that the function's values at the endpoints are equal is highlighted for the theorem's application.
The video concludes with an invitation to subscribe for more math content, emphasizing the educational value of the presented material.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: