Calc BC 2022 FRQs 1-6 Compilation

turksvids

12 May 202246:21

EducationalLearning

32 Likes 10 Comments

TLDRThis video script covers a detailed walkthrough of calculus problems from the 2022 AP exams, including rate of change, average value, and inflection points. The video tackles a variety of questions, such as finding the area of a region bounded by a curve, the volume of a solid generated by revolving a region around an axis, and the convergence of a power series. Each problem is methodically solved using calculus concepts like integrals, derivatives, and series tests, providing a comprehensive review for students preparing for calculus exams.

Takeaways

📝 The video covers problems from the 2022 Calc AB and BC exams, focusing on various calculus concepts such as rates of change, integrals, and series.
🚗 The first problem involves calculating the total number of vehicles arriving at a toll plaza and the average rate of arrival, using the given rate of change function.
📊 The video demonstrates the use of integrals to find the area under a curve, which is applied to determine the volume of a cone and the area of a region bounded by certain functions.
🔄 The concept of the average value of a function is introduced and applied to find the average rate at which vehicles arrive at a toll plaza over a given time interval.
📈 The video explains how to use the derivative to determine if a function is increasing or decreasing, and how to find the rate at which a vehicle arrives at a specific time.
🏢 The transcript includes a detailed walkthrough of solving calculus problems, emphasizing the importance of understanding the underlying concepts and the steps involved in the calculations.
📝 The video also covers the use of the ratio test to determine the interval of convergence for a power series and how to find the error term in a series approximation.
🔢 The importance of precision and careful calculation is highlighted, especially when dealing with decimals and avoiding unnecessary simplification that could lead to errors.
🎓 The video provides insights into the problem-solving process, including the use of the Intermediate Value Theorem and the Candidate's Test for finding points of inflection and absolute extrema.
📊 The concept of improper integrals and their application in finding volumes of solids generated by revolving regions about an axis is discussed.
📝 The video concludes with a problem involving the differentiation of a power series to find the series representation of the derivative function and the calculation of specific function values.

Q & A

What is the rate of change problem discussed in the video?
-The rate of change problem discussed in the video involves determining the total number of vehicles arriving at a toll plaza between specific times, as well as calculating the average rate at which vehicles arrive during a given interval.
How is the average value of the rate of vehicles arriving at the toll plaza calculated?
-The average value is calculated by taking the integral of the rate function over the given time interval (from t=1 to t=5) and dividing it by the length of the interval (5-1). The result is the average number of vehicles per hour over that time period.
What does the derivative of the rate function represent?
-The derivative of the rate function, a'(t), represents the rate at which the rate of vehicles arriving is changing at any given time t. If a'(t) is positive, the rate is increasing, and if it's negative, the rate is decreasing.
How is the maximum number of vehicles in line at the toll plaza determined?
-The maximum number of vehicles in line is determined by using the Candidate's Test on the continuous function n(t), which represents the number of vehicles in line. The absolute maximum value is found either at the endpoints or at a critical point within the interval from t=a to t=4.
What is the significance of the integral in the context of the toll plaza problem?
-The integral is used to calculate the total number of vehicles arriving at the toll plaza over a specified time interval and to find the area under the rate function, which represents the total change in the number of vehicles over time.
How does the video approach the problem of finding the slope of the tangent line to the path of a moving particle?
-The video finds the slope of the tangent line by calculating the derivative of the y-coordinate with respect to the x-coordinate (dy/dx) at a specific time t=4, using the given rate of change functions dx/dt and dy/dt.
What is the speed of the particle at time t equals 4?
-The speed of the particle at t=4 is found by taking the square root of the sum of the squares of the rate of change of the x and y coordinates (dx/dt and dy/dt) at that time.
How does the video solve for the y-coordinate of the particle's position at time t equals 6?
-The y-coordinate at t=6 is found by taking the y-coordinate at t=4 and adding the displacement of y, which is the integral of the rate of change of y (dy/dt) from t=4 to t=6.
What is the total distance the particle travels along the curve from time t equals 4 to t equals 6?
-The total distance traveled is the integral of the speed (the magnitude of the velocity vector) from t=4 to t=6, which is the square root of the sum of the squares of dx/dt and dy/dt over that interval.
How does the video address the problem of finding the function values for a given derivative graph?
-The video uses the Fundamental Theorem of Calculus to find the function values by integrating the derivative function from known points to the points of interest, and also by subtracting or adding areas under the derivative graph as necessary.
What method is used in the video to find the intervals where a function g(x) is decreasing?
-The video uses the derivative of the function g(x) and determines where g'(x) is less than zero to find the intervals of decrease. It also visually analyzes the graph of the derivative to confirm these intervals.

Outlines

00:00

📚 Calculus Exam Problem: Rate of Change and Average Value

This paragraph discusses a calculus problem from the 2022 exam, focusing on the rate of change of vehicles at a toll plaza and calculating the total number of vehicles that arrive during a specific time interval. It also covers finding the average rate at which vehicles arrive and determining whether the rate is increasing or decreasing at a particular time. The problem involves integral calculus and the use of the fundamental theorem of calculus.

05:01

📈 Vector/Parametric Equations and Motion Analysis

The paragraph presents a problem involving a particle moving along a curve in the xy-plane, with given derivatives for position and velocity. The task is to find the slope of the tangent line at a specific time, calculate the speed and acceleration vector of the particle, determine the y-coordinate of the particle's position at a later time, and finally, calculate the total distance traveled by the particle between two time points.

10:02

📊 Interpreting Derivative Graphs and Finding Function Values

This section deals with a problem where a graph of the derivative of a function is provided, and the goal is to find the values of the function at specific points, using the graph and the fundamental theorem of calculus. The problem also asks for the x-coordinates of points of inflection and the analysis of a related function's decreasing intervals.

15:03

🏗️ Table Problem: Conical Sculpture and Volume Rates

The paragraph describes a table problem where a conical sculpture's radius changes over time, and the rate of change of the radius is given. The task involves approximating the second derivative at a specific time, determining if a certain rate of change exists within a time interval, using a right Riemann sum to approximate an integral, and calculating the rate of change of the cone's volume with respect to time.

20:04

🌀 Area, Volume, and Improper Integrals

This paragraph covers a multi-part problem involving areas and volumes associated with functions y equals 1/x and y equals 1/x^2. The tasks include finding the area of a region bounded by these functions, calculating the volume of a solid formed by revolving a region around the x-axis, and dealing with an improper integral to find the volume of an unbounded region.

25:06

📊 Series Convergence and Power Series Derivatives

The paragraph focuses on a series question from the 2022 Calc BC exam, involving the convergence of a power series and the use of the ratio test. It covers finding the interval of convergence, showing the error bound for a specific value, and deriving the general term for the series representing the function's derivative. The problem concludes with finding the value of the derivative at a particular point.

Mindmap

Keywords

💡Rate of Change

The rate of change refers to the speed at which a variable changes with respect to another variable. In the context of the video, it is used to describe the rate at which vehicles arrive at a toll plaza, given by the function a(t). It is a fundamental concept in calculus, used to analyze the behavior of functions, particularly in optimization problems and motion studies.

💡Integral

An integral is a mathematical concept that represents the accumulation of a quantity, such as the area under a curve or the total number of vehicles passing through a toll booth over a given time interval. In calculus, it is used to find the anti-derivative of a function and to calculate the accumulated change, which in the video's context, is used to determine the total number of vehicles that arrive at the toll plaza between t=1 and t=5.

💡Average Value

The average value of a function over an interval is the mean value of the function's rate over that interval. It is calculated by integrating the function over the interval and dividing by the length of the interval. In the video, the average value of the rate at which vehicles arrive at the toll plaza from t=1 to t=5 is determined by integrating the rate function a(t) over this interval and dividing by 4.

💡Derivative

A derivative is a mathematical concept that represents the rate of change or the slope of a function at any given point. In the context of the video, the derivative of the arrival rate function a(t) is used to determine whether the rate of vehicle arrival is increasing or decreasing at t=1, which corresponds to 6 am in the real-world scenario.

💡Parametric Equations

Parametric equations are a set of equations that describe the coordinates of a point moving in a plane or space as a function of a parameter. In the video, the position of a particle moving along a curve in the xy-plane is given by parametric equations x(t) and y(t), where t is the time parameter.

💡Vector

A vector is a mathematical object that has both magnitude and direction. In physics and engineering, vectors are used to represent quantities like velocity, force, and acceleration. In the video, the acceleration vector of the particle is found by taking the second derivative of the position functions x(t) and y(t) with respect to time t.

💡Fundamental Theorem of Calculus

The Fundamental Theorem of Calculus connects the concepts of differentiation and integration. It states that if a function is continuous on a closed interval and has an antiderivative on that interval, then the definite integral of the function can be computed by evaluating the antiderivative at the endpoints of the interval. In the video, this theorem is used to find the area of a region under a curve and the volume of a solid generated by revolving a region around an axis.

💡Series Convergence

Series convergence refers to the process of determining whether the sum of an infinite series approaches a finite value. The ratio test, as mentioned in the video, is a common method for determining the interval of convergence for a power series. A series converges if the limit of the ratio of consecutive terms approaches zero or a value less than one.

💡Power Series

A power series is an infinite series where the general term is a variable raised to a power multiplied by a coefficient. Power series are used to represent functions in a way that allows for differentiation and integration term by term. In the video, the function f(x) is defined by a power series, and the series is analyzed for convergence and to find the function's derivative.

💡Alternating Series

An alternating series is a type of infinite series where the terms alternate in sign. The Alternating Series Test is a method to determine the convergence of such series. If the terms of an alternating series decrease in magnitude and approach zero, the series converges. In the video, the series at x = -1 is shown to converge by the Alternating Series Test.

Highlights

The problem involves calculating the total number of vehicles arriving at a toll plaza using an integral expression.

The average value of the rate at which vehicles arrive is found using the integral over the interval from t=1 to t=5, divided by the interval length.

To determine if the rate of vehicle arrival is increasing or decreasing, the derivative of the rate function is evaluated at t=1.

The maximum number of vehicles in line at the toll plaza is found using the candidate's test and the integral from a to 4 of the rate function minus 400.

The slope of the line tangent to the path of a particle is calculated using the derivatives of the position functions and evaluating at t=4.

The speed of the particle at time t=4 is found by taking the square root of the sum of the derivatives of the position functions squared.

The acceleration vector of the particle at t=4 is determined by finding the second derivatives of the position functions.

The y-coordinate of the particle's position at t=6 is found by integrating the derivative of the y-position function from t=4 to t=6.

The total distance the particle travels from t=4 to t=6 is calculated using the integral of the speed function over that time interval.

The function f is reconstructed from its derivative using the fundamental theorem of calculus and the given value of f at t=4.

Points of inflection for the function f are identified by where the derivative changes from increasing to decreasing or vice versa.

The function g is defined as the difference between f and x, and its intervals of decrease are found by analyzing the sign of its derivative.

The absolute minimum value of g on the interval from 0 to 7 is determined using the candidate's test and the critical points found from the derivative.

The average rate of change of the radius of a cone is used to approximate the second derivative at t=8.5.

The intermediate value theorem is applied to conclude that there exists a time t between 0 and 3 where the rate of change of the radius equals -6.

A right Riemann sum is used to approximate the integral of the rate of change of the radius from t=0 to t=12.

The rate of change of the volume of a cone is calculated using the given rate of change of its height and the radius at a specific time.

The volume of a solid generated by revolving a region about the x-axis is found using integration by parts and an improper integral.

The interval of convergence for a given power series is determined using the ratio test and by testing the endpoints.

The error in approximating a function using the first term of its power series is shown to be less than 0.1 using the alternating series error term.

The first four non-zero terms and the general term of the power series representing the derivative of the function are identified using the power rule.

The value of the function's derivative at a specific point is found using the sum of a geometric series and the general term of the derivative's power series.

Transcripts

Browse More Related Video

2022 AP Calculus BC Exam FRQ #5

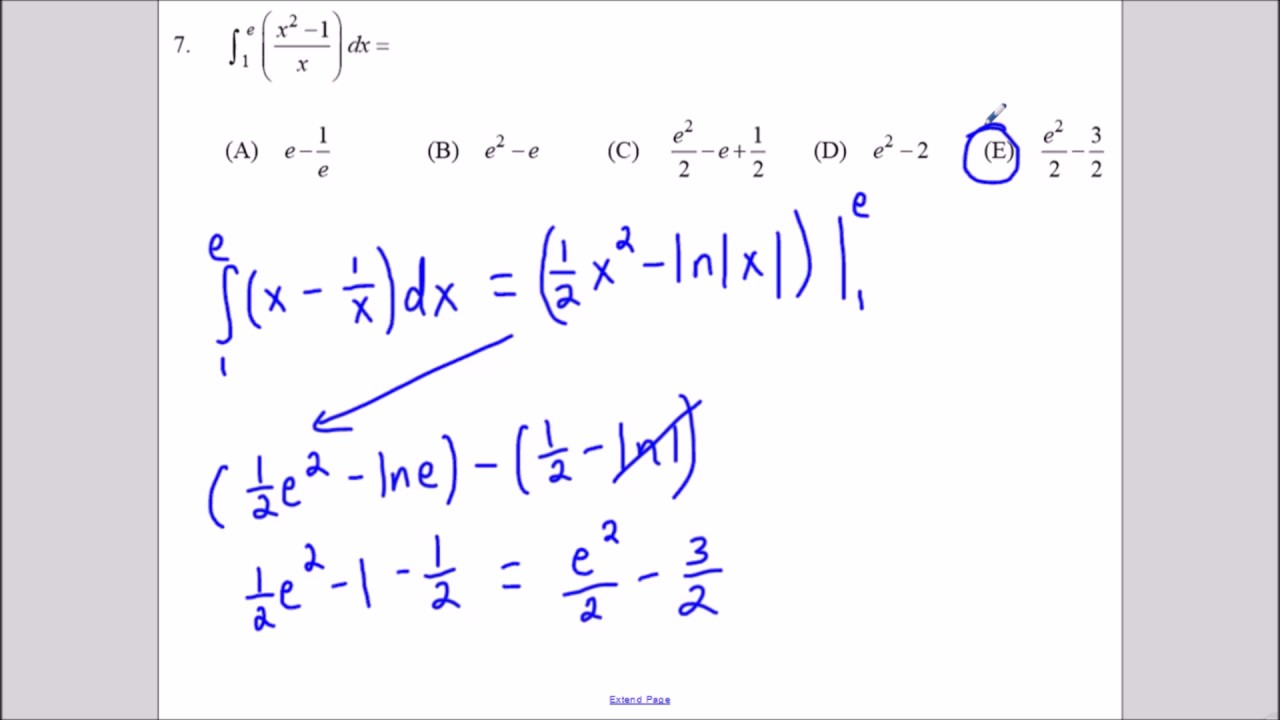
AP Calculus AB 1998 Multiple Choice No Calculator

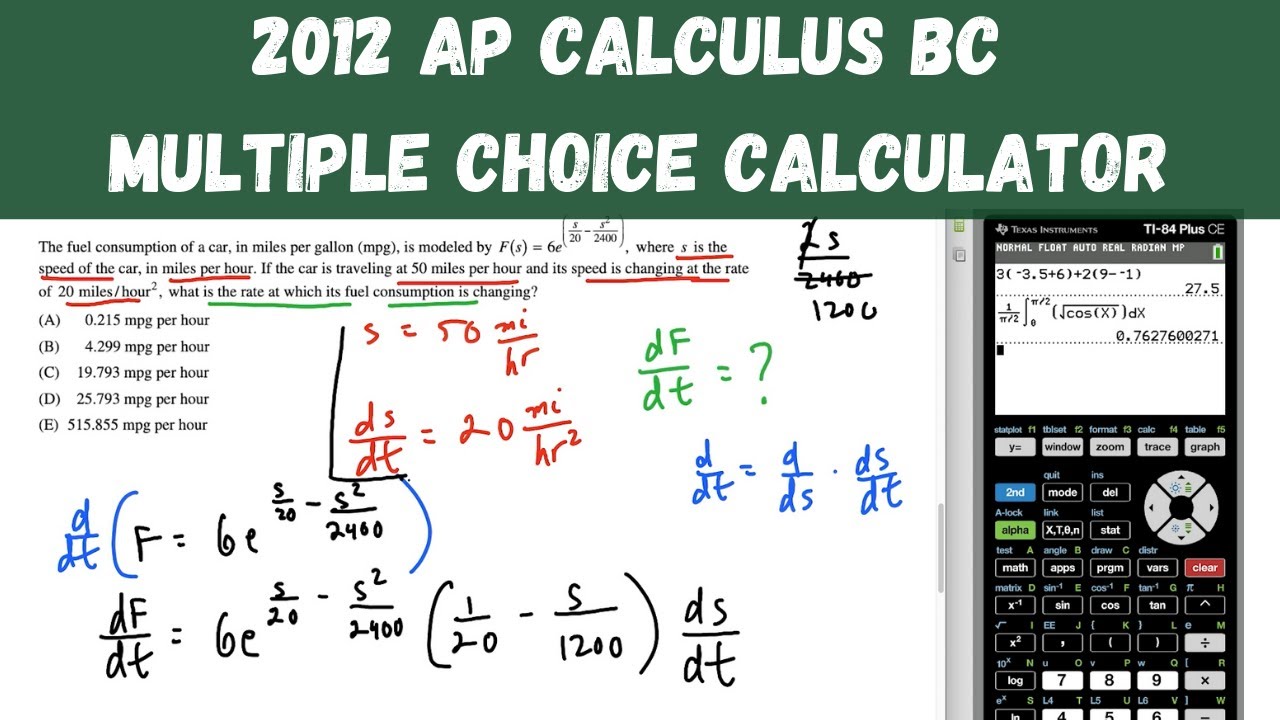
AP Calculus BC Practice Exam 2012 - Calculator Multiple Choice questions 76-92

2006 AP Calculus AB Free Response #1

7 | FRQ (No Calculator) | Practice Sessions | AP Calculus AB

AP Calculus AB 2012 Multiple Choice (calculator) - Questions 76 - 92

Related Tags

Calculus Exam Rate of Change Series Convergence Improper Integrals Mathematics Education Exam Strategies Problem Solving AP Calculus Video Tutorial Study Guide