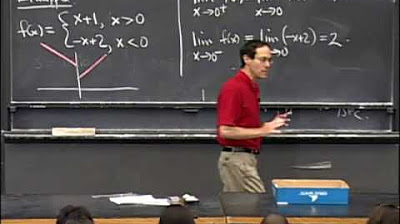Understand Calculus in 35 Minutes
TLDRThis video script introduces the fundamentals of calculus, focusing on three core areas: limits, derivatives, and integration. Limits are used to evaluate functions as variables approach certain values, derivatives calculate the instantaneous rate of change or the slope of a function's tangent line, and integration, the reverse process of differentiation, is used to find accumulated quantities over time. The script provides examples and explanations to illustrate these concepts, emphasizing their practical applications in solving real-world problems.
Takeaways
- 📌 Limits are used to evaluate functions when x approaches a certain value, even when the function is undefined at that point.
- 📈 Derivatives represent the slope of the tangent line to a function at a specific x value and are used to calculate rates of change.
- 🔄 Integration is the process of finding the anti-derivative and is useful for calculating the area under a curve, representing accumulation over time.
- 🔢 The three main areas of calculus are limits, derivatives, and integration, each with its own rules and applications.
- 🌟 The power rule is a fundamental rule for finding derivatives: the derivative of x^n is n*x^(n-1).
- 📊 To find the slope of the tangent line, one can either use the derivative directly or approximate it using the slope of the secant line.
- 🛠️ Factoring expressions can simplify the process of finding limits, especially when dealing with indeterminate forms like 0/0.
- 📈 The rate of water accumulation in a tank can be modeled by an integration problem, where the integral of the flow rate function gives the total volume accumulated.
- 📐 The area under the curve from t=20 to t=100 minutes represents the change in water volume in the tank during this period, not the total volume.
- 🔍 The concept of instantaneous rate of change is key to understanding derivatives and their physical interpretation.
- 📚 Practicing with specific problems on limits, derivatives, and integration is essential for solidifying understanding of these calculus concepts.
Q & A
What are the three fundamental areas of calculus discussed in the video?
-The three fundamental areas of calculus discussed are limits, derivatives, and integration.
What is the primary use of limits in calculus?
-Limits are used to evaluate what happens to a function as the input approaches a certain value, even when the function may be undefined or cannot be directly evaluated at that point.
How are derivatives related to the slope of a function?
-Derivatives represent the slope of the tangent line to a function at a specific point, which is useful for calculating rates of change.
What is the relationship between derivatives and anti-derivatives?
-Derivatives and anti-derivatives are inverse processes; the derivative of a function gives the slope of the tangent line, while the anti-derivative (integration) finds the original function from its derivative.
How can you calculate the slope of the tangent line using the power rule?
-The slope of the tangent line can be calculated using the power rule by taking the derivative of the function, which for a function f(x) = x^n is n*x^(n-1).
What is the process of finding the area under a curve in calculus?
-The process of finding the area under a curve is called integration. It involves evaluating a definite integral to calculate the accumulated quantity over a specified interval.
How does the video demonstrate the concept of instantaneous rate of change?
-The video demonstrates the concept of instantaneous rate of change by showing how derivatives can be used to find the slope of the tangent line at a specific point, which represents the rate of change at that exact moment.
What is the difference between the slope of the tangent line and the slope of the secant line?
-The slope of the tangent line represents the instantaneous rate of change at a specific point, while the slope of the secant line represents the average rate of change between two points.
How does the video illustrate the accumulation of water in a tank?
-The video illustrates the accumulation of water in a tank by using integration to calculate the net change in water volume over a period of time, represented by the area under the curve of the function representing the rate of water flow.
What is the significance of the definite integral in the context of the video?
-In the context of the video, the definite integral is significant because it is used to calculate the accumulated amount of water in the tank over a specific time interval, which is the area under the curve of the function representing the rate of water flow.
How does the video connect the concepts of limits, derivatives, and integration?
-The video connects these concepts by showing how limits can help evaluate a function near a certain value, derivatives can find the rate of change or slope of a function, and integration can find the accumulated quantity or area under the curve of a function.
Outlines
📚 Introduction to Calculus: Fundamental Concepts
This paragraph introduces the viewer to the basics of calculus, highlighting three key areas: limits, derivatives, and integration. Limits are explained as a tool to evaluate functions when direct evaluation is not possible, showing what happens as a variable approaches a certain value. Derivatives are introduced as functions that provide the slope of the original function at a specific point, useful for calculating rates of change. Integration is presented as the process of finding the area under a curve, which is useful for understanding accumulation over time. The video aims to teach these concepts in a short span, providing foundational knowledge for further study.
📈 Understanding Limits and Their Applications
The second paragraph delves deeper into the concept of limits, explaining how they help in evaluating functions when direct substitution leads to an indeterminate form, such as 0/0. It uses the example of f(x) = (x^2 - 4) / (x - 2) and shows how to evaluate the limit as x approaches 2 by factoring and canceling terms. The paragraph emphasizes that even if f(2) does not exist, the limit as x approaches 2 exists and equals four, illustrating how limits can reveal the behavior of functions near certain points.
🔄 Power Rule and Derivatives
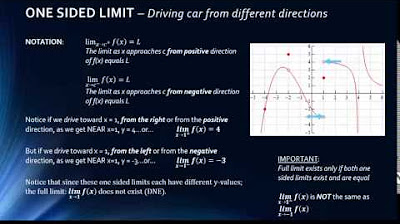
This paragraph focuses on the power rule for finding derivatives, explaining that the derivative of a variable raised to a constant power is the constant times the variable to the power minus one. Examples are provided to demonstrate how to find the derivative of functions like x^2, x^3, and x^4. The paragraph then explains the geometric interpretation of derivatives as the slope of the tangent line to a curve at a given point. It introduces the concepts of tangent and secant lines, using examples to show how the slope of the secant line approximates the slope of the tangent line as the points used approach the point of interest.
📊 Secant Lines and Tangent Line Slopes
The third paragraph continues the discussion on the relationship between secant lines and tangent lines, illustrating how the slope of the secant line can be used to estimate the slope of the tangent line. It provides a step-by-step calculation of the slope of the secant line using the formula (y2 - y1) / (x2 - x1) and shows how this slope approximates the tangent line slope as the points get closer to the point of interest. The paragraph also demonstrates how to use a limit process to find the slope of the tangent line, connecting the concept of limits with derivatives.
🌊 Integration and Anti-Differentiation
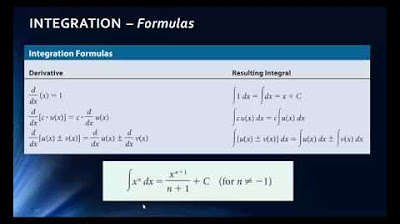
The fourth paragraph introduces integration, also known as anti-differentiation, as the process of finding the anti-derivative. It explains how to find the integral of a function using the power rule, adding 1 to the exponent and dividing by the result, while also accounting for a constant of integration. The paragraph contrasts derivatives, which give the slope of the tangent line and are useful for instantaneous rates of change, with integration, which helps in determining the accumulated amount over a period of time. It emphasizes that integration is the opposite process of differentiation.
📈 Calculating Water Levels and Rates of Change
This paragraph presents a practical problem involving the calculation of water levels in a tank over time. It demonstrates how to use the original function to find the amount of water in the tank at specific times and how to calculate the rate of change of water in the tank at a particular time by finding the derivative. The paragraph shows the process of evaluating the function a(t) = 0.01t^2 + 0.5t + 100 at different times (t = 0, 9, 10, 11, 20) and how to find the derivative a'(t) to determine the rate of change of water in the tank when t equals 10 minutes.
🚰 Water Flow Rate and Accumulation
The fifth paragraph addresses another practical problem, focusing on the rate of water flowing into a tank represented by the function r(t) = 0.5t + 20. It explains how to use integration to calculate the total amount of water accumulated in the tank over a period of time, specifically from t = 20 to t = 100 minutes. The paragraph provides a step-by-step guide on finding the anti-derivative of r(t), evaluating it between the given limits, and calculating the net change in water volume. It also visually represents the problem by graphing the function and calculating the area under the curve to represent the change in water volume.
🎓 Summary of Calculus Concepts
The final paragraph summarizes the main concepts taught in the video: limits, derivatives, and integration. It reiterates that limits allow the evaluation of functions when x approaches a certain value, derivatives calculate the instantaneous rate of change, and integration determines the accumulation over time. The paragraph emphasizes the importance of understanding these fundamental concepts for anyone studying calculus and encourages viewers to practice these concepts further through additional problems and video resources provided in the video description.
Mindmap
Keywords
💡Calculus
💡Limits
💡Derivatives
💡Integration
💡Slope
💡Tangent Line
💡Power Rule
💡Secant Line
💡Anti-Derivative
💡Definite Integral
💡Instantaneous Rate of Change
Highlights
The video attempts to teach the fundamentals of calculus in a short time.
There are three main areas of calculus: limits, derivatives, and integration.
Limits help evaluate a function when it's undefined or cannot be directly calculated.
Derivatives are functions that give the slope of the original function at a specific value, useful for calculating rates of change.
Integration is the process of finding the area under the curve, useful for calculating accumulation over time.
Derivatives and integration are opposite processes; the derivative of a function is the integral of its derivative.
The power rule is a basic rule for finding derivatives, where the derivative of x to the n power is n times x to the (n-1) power.
The slope of a tangent line is equal to the derivative of a function at a specific value.
The slope of a secant line approximates the slope of the tangent line as the points get closer to the point of interest.
The limit expression can be used to evaluate the slope of the tangent line using the formula (f(x) - f(a)) / (x - a) as x approaches a.
The process of anti-differentiation involves adding 1 to the exponent, dividing by the result, and including a constant of integration.
Integration is the process of multiplying y values by x values, while differentiation involves dividing y values by x values.
The function a(t) = 0.01t^2 + 0.5t + 100 represents the amount of water in gallons inside a tank at time t in minutes.
The derivative a'(t) = 0.02t + 0.5 represents how fast the amount of water is changing in the tank at any time t.
The slope of the secant line can be used to approximate the slope of the tangent line, representing the average rate of change between two points.
The function r(t) = 0.5t + 20 represents the rate of water flowing into the tank in gallons per minute.
Integration is used to calculate the accumulation of water in the tank from t=20 to t=100 minutes, resulting in a 4,000-gallon increase.
The area under the curve from t=20 to t=100 represents the change in the volume of water in the tank over those 80 minutes.
The video concludes by emphasizing the importance of understanding limits, derivatives, and integration as the three fundamental concepts in calculus.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: