AP Calculus AB/BC Unit 8 Practice Test
TLDRIn this video, Vin walks through an AP Calculus practice test, focusing on Unit 8. He explains how to approach multiple choice questions involving rates of change, average values, and the fundamental theorem of calculus. Vin demonstrates the process of solving for various scenarios, such as a hot dog eating contest, temperature changes, particle motion, and finding the area and volume of specific regions and solids. His step-by-step breakdown of each problem provides viewers with a clear understanding of the concepts and methods used in calculus to solve real-world problems.
Takeaways
- 📝 The video is a walkthrough of an AP Calculus practice test, focusing on solving various problems related to rates, volumes, and areas in calculus.
- 🔢 The host, Vin, begins by discussing a problem involving the rate of hot dog consumption in a contest, emphasizing the use of average rate of change and average function value formulas.
- ⏱️ Vin highlights the importance of using a calculator for multiple-choice questions and provides a step-by-step guide on how to use it effectively for calculus problems.
- 🌡️ A temperature modeling problem in San Diego is solved using the average function value formula, with attention to converting the initial time to the correct unit (hours after midnight).
- 🚀 The concept of position, velocity, and acceleration is discussed in the context of a particle moving along the x-axis, with the application of the fundamental theorem of calculus.
- 📈 Vin demonstrates how to find the area of a region bounded by two graphs, using integral calculus and the concept of horizontal cross-sections.
- 🏢 A coffee factory scenario is used to illustrate the application of calculus in real-world situations, specifically in determining the rate of coffee bean arrival and the minimum amount of coffee beans at the factory.
- 🌀 Vin explains how to calculate the volume of a solid generated by revolving a region around the x-axis, using washer method and the concept of pi times (radius squared times differential dx).
- 🏗️ The video also covers how to find the volume of a solid with cross-sections perpendicular to the x-axis, using the formula for the volume of a single cross-section and integration.
- 📊 The host provides a detailed explanation of how to calculate the total distance traveled by a particle, differentiating between displacement and distance in the process.
- 📚 The importance of understanding the geometric interpretation of definite integrals is emphasized, as it is key to solving problems related to areas and volumes in calculus.
Q & A
What is the main topic of the video?
-The main topic of the video is going through an AP Calculus practice test, specifically Unit 8, and solving various problems related to rates, temperatures, particle motion, and geometric solids.
How does the presenter approach the problem of finding the average rate at which Hank eats hot dogs?
-The presenter approaches the problem by identifying the correct formula to use, which is the average function value formula, and then applying it to the given function R(T) to find the average rate from time t=5 to t=8.
What is the significance of the fundamental theorem of calculus in the context of this video?
-The fundamental theorem of calculus is significant in this video as it is used to find the position of a particle at a given time, given its velocity function, and to evaluate definite integrals in various problems.
How does the presenter determine the average temperature in San Diego on a particular April day?
-The presenter determines the average temperature by using the average function value formula with the given temperature function f(T) and evaluating the integral from T=2 (2 a.m.) to T=8 (8 a.m.).
What is the main concept behind finding the area between two curves?
-The main concept behind finding the area between two curves is to use the top function minus the bottom function and then integrate over the given interval to find the enclosed area.
How does the presenter calculate the volume of a solid generated by revolving a region about the x-axis?
-The presenter calculates the volume of a solid generated by revolving a region about the x-axis by identifying the type of cross-section (washer or disc), determining the big and little radii, and then using the washer formula (if applicable) or the disc formula to integrate the volume over the given interval.
What is the key to solving the problem of finding the area of a region bounded by the graphs of x = y^2 and y = x?
-The key to solving this problem is to recognize that it involves finding the horizontal distance between the two curves and then integrating this distance over the interval defined by the intersection points of the curves.
How does the presenter use the concept of symmetry to simplify the calculation of the volume of a solid?
-The presenter uses the concept of symmetry by recognizing that the function y = x^2 is even and has symmetry over the y-axis. This allows the presenter to integrate from 0 to 2 and then multiply the result by 2 to account for the full interval from -2 to 2, effectively simplifying the calculation.
What is the method used by the presenter to find the total distance traveled by a particle?
-The presenter uses the method of integrating the absolute value of the velocity function over the given time interval to find the total distance traveled by the particle.
How does the presenter calculate the volume of a solid with cross-sections that are equilateral triangles?
-The presenter calculates the volume of the solid by finding the area of an equilateral triangle using the formula (1/2) * base * height and then integrating this area over the interval defined by the x-axis limits of the region.
Outlines
📚 Introduction to AP Calculus Practice Test
The video begins with Vin introducing the AP Calculus practice test, emphasizing the use of a calculator for multiple-choice questions. He discusses the importance of understanding the correct formulas for average rate of change and average function value, using the example of a hot dog eating contest to illustrate the concept.
🌡️ Temperature Variation in San Diego
Vin tackles a question about the average temperature in San Diego, explaining the use of the average function value formula. He emphasizes the importance of using the correct function and the fundamental theorem of calculus to find the position of a particle, given its velocity function.
🚀 Particle Motion and Position Calculation
The paragraph focuses on calculating the position of a particle at a specific time, given its velocity function. Vin explains the process of using the fundamental theorem of calculus and the anti-derivative to find the position function, ultimately determining the particle's position at time T equals 4.
📈 Area Calculation Between Two Curves
Vin discusses how to find the area between two curves, using the functions X = y^2 and Y = x as examples. He explains the concept of horizontal cross-sections and the use of the fundamental theorem of calculus to calculate the area of the region in the first quadrant bounded by these functions.
🏞️ Volume Calculation of a Solid
The video segment covers the calculation of the volume of a solid generated by revolving a region around the x-axis. Vin explains the difference between using a disc (solid) and a washer (with a hole) for the cross-section and demonstrates how to find the volume by integrating the area of the cross-section.
🔄 Volume of Solid Generated by Revolving a Region
Vin continues the discussion on calculating the volume of a solid by revolving a region around an axis. He provides a detailed explanation of how to find the volume when the cross-sections are rectangles with heights equal to twice the length of the base of the rectangle.
📊 Interpreting Graphs and Calculating Volumes
The paragraph involves interpreting the graphs of functions and calculating the volume of a solid with cross-sections perpendicular to the x-axis. Vin explains how to use the fundamental theorem of calculus and the concept of symmetry to find the volume of the solid.
🏃 Total Distance Traveled by a Particle
Vin addresses the concept of total distance traveled by a particle, differentiating it from displacement. He sets up an integral to calculate the total distance from time T equals 0 to T equals 4, using the absolute value of the velocity function.
🏢 Coffee Factory Production Analysis
The video segment discusses a scenario involving a coffee factory's production, focusing on the rate of arrival of raw coffee beans and the rate of roasting. Vin explains how to use integration to find the total amount of coffee beans arriving during the workday and how to calculate the rate of arrival at a specific time.
📈 Rate of Change and Coffee Bean Inventory
Vin calculates the rate of change of the coffee bean arrival function at a specific time and interprets its meaning in the context of the coffee factory's operations. He then determines whether the amount of raw coffee beans at the factory is increasing or decreasing at that time.
📊 Minimum Raw Coffee Beans at the Factory
The video concludes with Vin calculating the minimum amount of raw coffee beans at the factory during the workday. He sets up a function representing the amount of coffee beans and uses derivatives to find the minimum value, justifying his answer with a step-by-step explanation.
📈 Area Calculation for a Region
Vin introduces a new problem involving the calculation of the area of a region bounded by two functions. He explains the process of finding the points of intersection and setting up an integral to calculate the area, emphasizing the use of vertical cross-sections.
🏗️ Volume of a Solid with Square Cross-Sections
The video segment focuses on finding the volume of a solid with cross-sections parallel to the y-axis. Vin explains how to visualize the solid and calculate the volume by integrating the area of a single cross-section, using the top and bottom functions to determine the dimensions of the cross-sections.
📊 Equal Area Partition by a Horizontal Line
Vin discusses a problem involving a horizontal line that divides a region into two areas of equal size. He explains how to set up an equation involving integrals to find the value of the line's y-coordinate, without solving the equation, and how this relates to the area of the region.
Mindmap
Keywords
💡AP Calculus
💡Hot Dog Eating Rate
💡Average Function Value
💡Integral
💡Fundamental Theorem of Calculus
💡Velocity Function
💡Position Function
💡Area of a Region
💡Volume of a Solid
💡Cross-Sections
Highlights
The video begins with an introduction to the AP Calculus practice test and a link to the test in the description.
The first problem involves calculating the average rate at which a hot dog eater consumes hot dogs over a specific time interval.
The average rate of change formula and the average function value formula are discussed for solving the first problem.
For the second problem, the average temperature in San Diego is calculated using the average function value formula.
The third problem requires the use of the fundamental theorem of calculus to find the position of a particle given its velocity function.
The area of a region bounded by two graphs is calculated in the fourth problem using definite integration.
The fifth problem involves finding the area between two curves, which is solved using the top-minus-bottom method and absolute value for simplification.
In the sixth problem, the volume of a solid generated by revolving a region around the x-axis is calculated using washers and the fundamental theorem of calculus.
The seventh problem requires setting up an integral to find the volume of a solid generated by revolving a region around a vertical axis.
The eighth problem involves finding the volume of a solid with cross-sections that are equilateral triangles, using trigonometric formulas and integration.
The ninth problem asks for the volume of a solid with rectangular cross-sections, using Cavalier's principle and integration.
The tenth problem deals with calculating the total distance traveled by a particle, distinguishing between distance and displacement.
The eleventh problem involves finding the volume of a solid with cross-sections that are semicircles, using inverse functions and integration.
The twelfth problem calculates the average value of a function over an interval, using the concept of definite integrals as area under the curve.
The thirteenth problem uses anti-derivatives and the fundamental theorem of calculus to relate the value of a function at different points in time.
The fourteenth problem models the increase in social media followers with a function and calculates future follower counts using integration.
The fifteenth problem finds the ratio of the area of a rectangle to the shaded area under a cubic function, using integration to determine the shaded area.
The sixteenth problem calculates the length of a graph using the arc length formula and integration.
The seventeenth and final multiple-choice problem involves finding the volume of a solid generated by revolving an unbounded region around the x-axis, using improper integrals.
Transcripts
Browse More Related Video
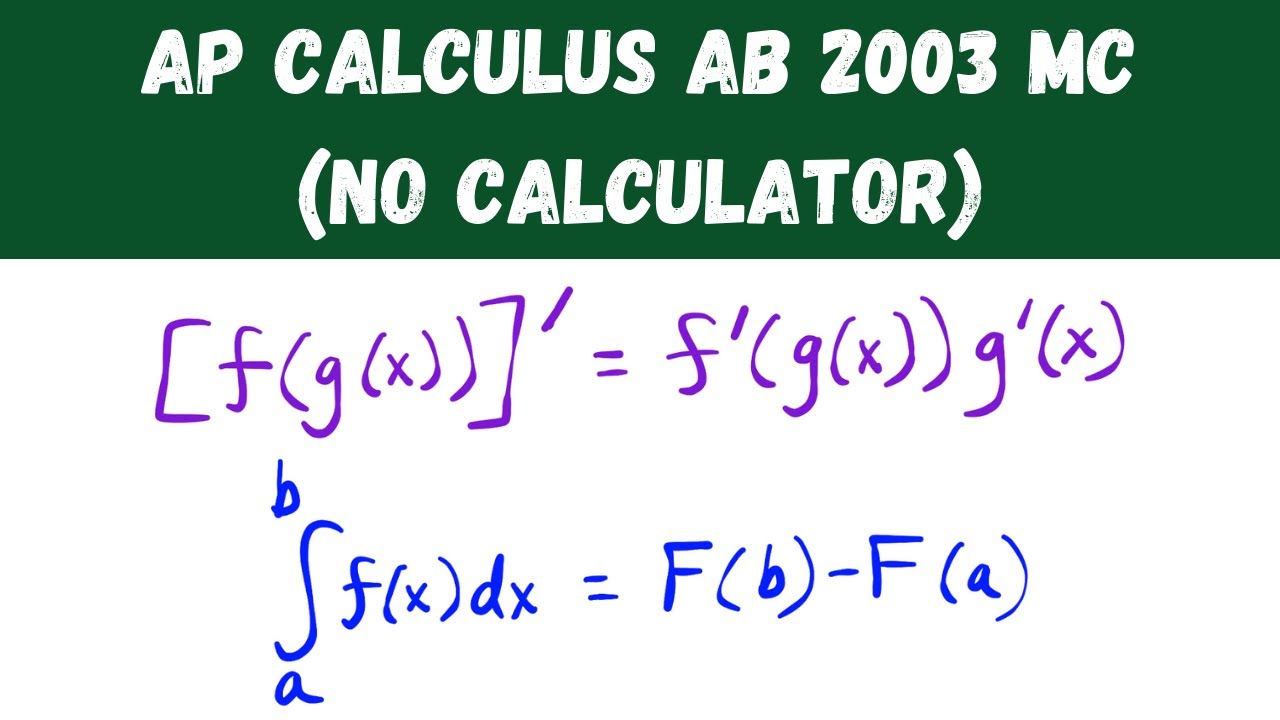
AP Calculus AB 2003 Multiple Choice (no calculator) - Questions 1-28

AP Calculus AB/BC Unit 7 Practice Test

AP Calculus BC 2003 Multiple Choice (calculator) - Questions 76 - 92
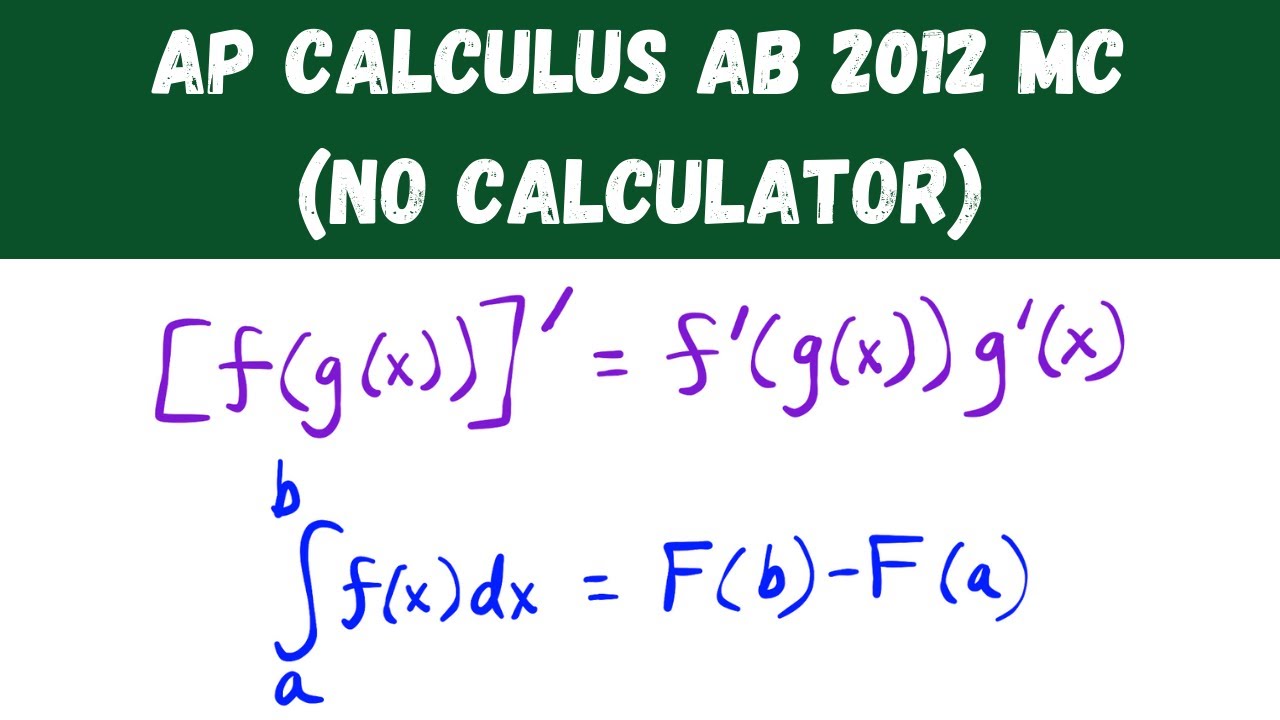
AP Calculus AB 2012 Multiple Choice (no calculator) - Questions 1-28
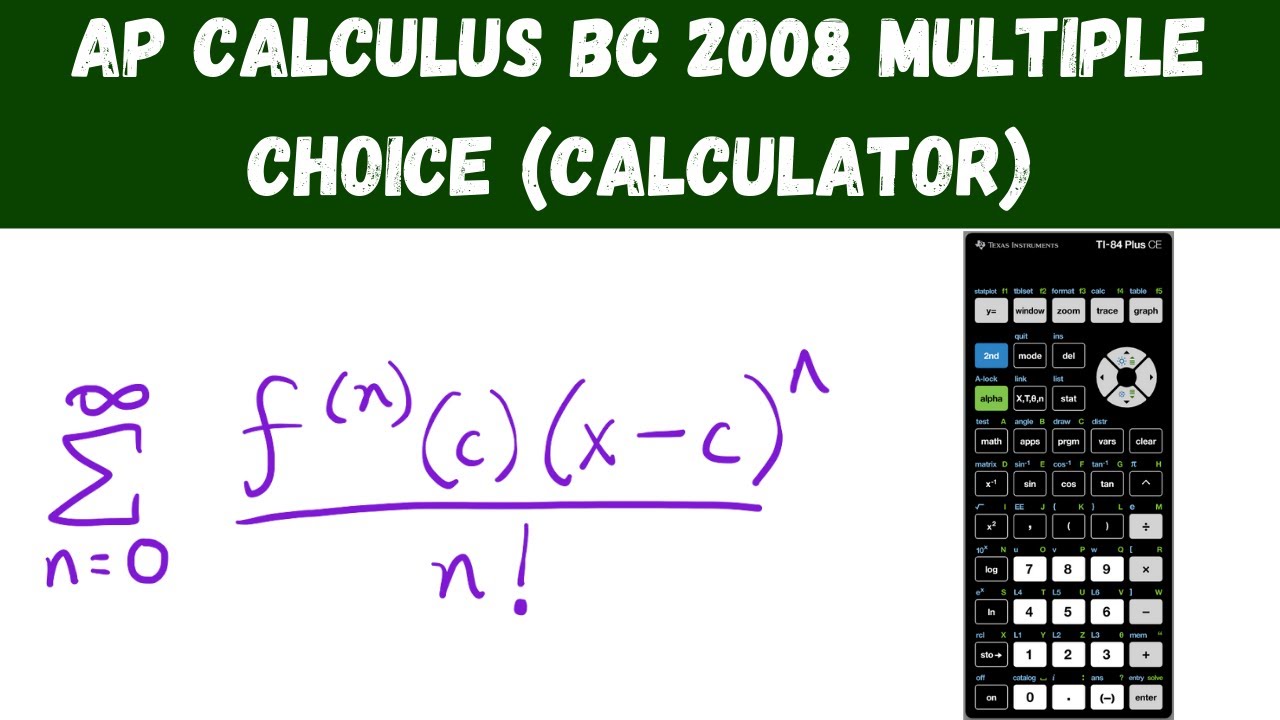
AP Calculus BC 2008 Multiple Choice (calculator) - Questions 76 - 92
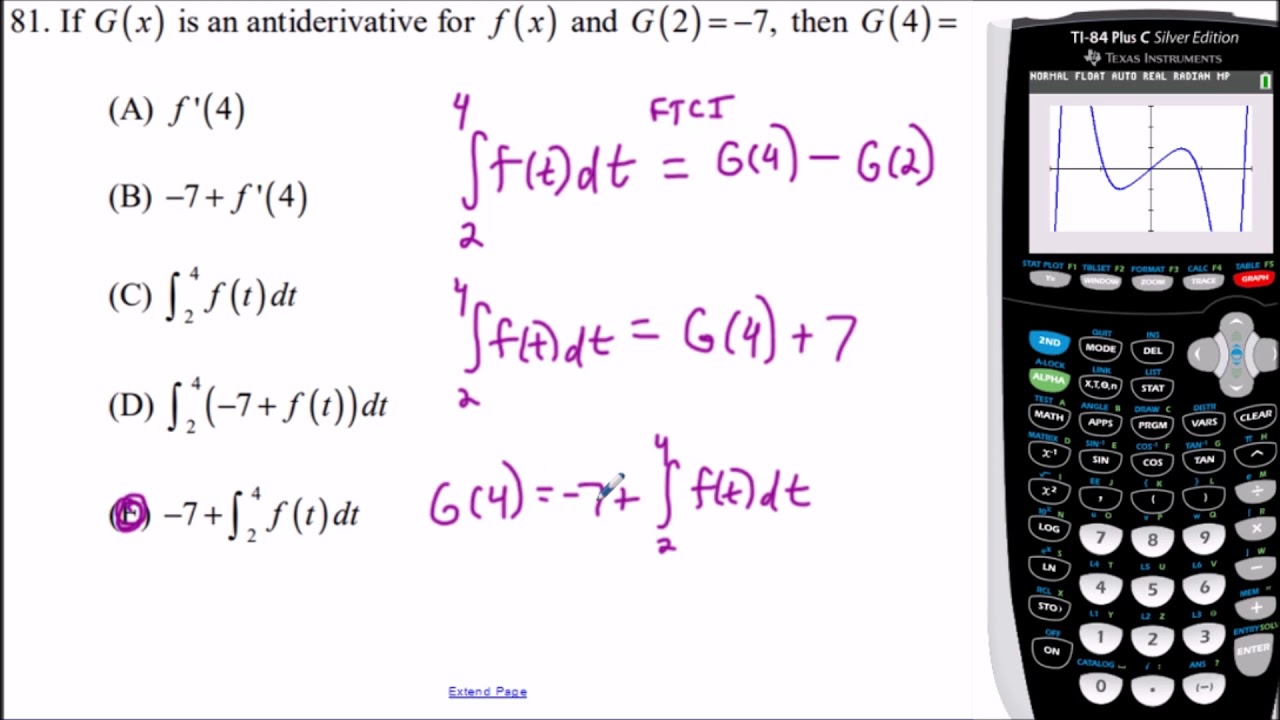
AP Calculus AB 2008 Multiple Choice (Calculator) - Questions 76-92
5.0 / 5 (0 votes)
Thanks for rating: