AP Calculus AB and BC Unit 5 Review [Analytical Applications of Differentiation]
TLDRThis comprehensive video script covers advanced calculus concepts, focusing on optimization through derivatives. It introduces the mean value theorem, extreme value theorem, and methods for identifying critical points and inflection points. The script explains how to use the first and second derivative tests to classify local extrema and the candidates test for global extrema. It also transitions from theoretical concepts to real-world applications, such as maximizing the volume of a box, and concludes with solving for critical points in implicitly defined functions. The content is aimed at preparing students for AP Calculus exams and enhancing their problem-solving skills.
Takeaways
- 📚 Unit 5 focuses on using derivatives for optimization, transitioning from derivatives to integrals in AP Calculus, and the importance of understanding optimization for success on the AP test.
- 📈 The Mean Value Theorem states that within a given interval, there exists a point where the instantaneous rate of change equals the average rate of change, applicable in real-world scenarios like road trips and temperature changes.
- 🔍 To apply the Mean Value Theorem, the function must be continuous over the closed interval (including endpoints) and differentiable on the open interval (excluding endpoints).
- 🏆 The Extreme Value Theorem guarantees at least one minimum and one maximum point on an open interval, which is intuitive as a continuous function will naturally achieve both minimum and maximum values.
- 🔢 For optimization, critical points are found by setting the derivative of a function equal to zero, which are potential locations for local maxima or minima.
- 📊 The first derivative test uses the sign of the derivative to determine if a critical point corresponds to a local maximum or minimum based on the function's increasing or decreasing behavior.
- 🔄 The second derivative test classifies critical points by plugging them into the second derivative; a negative result indicates a local maximum, and a positive result indicates a local minimum.
- 📐 Inflection points are identified by setting the second derivative equal to zero, marking where the graph changes concavity from concave up to down or vice versa.
- 📝 The candidate's test is used to find absolute or global extrema over a specific interval by evaluating the function at critical points and endpoints, with the highest value being the global maximum and the lowest being the global minimum.
- 🎨 The relationship between the graphs of the original function, its first derivative, and its second derivative can be used to sketch one graph given information about the others, aiding in visualizing the function's behavior.
- 🛠️ Real-world optimization problems require translating the mathematical process into a practical context, such as maximizing profit or minimizing cost, and answering the specific question asked in the context of the problem.
Q & A
What is the main focus of Unit 5 in the context of AP Calculus?
-Unit 5 focuses on using derivatives for optimization. It involves understanding and applying the mean value theorem, identifying critical points, determining where a function is increasing or decreasing, finding inflection points, and using the first and second derivative tests to classify local extrema.
What is the significance of the Mean Value Theorem in optimization problems?
-The Mean Value Theorem states that within a given interval, there exists at least one point where the instantaneous rate of change of a function is equal to the average rate of change over that interval. This theorem is crucial in optimization as it helps in identifying potential maximum and minimum values of a function on a closed interval.
How does the Extreme Value Theorem relate to optimization?
-The Extreme Value Theorem guarantees that a continuous function on a closed interval will have both a minimum and a maximum value within that interval. This is important in optimization because it ensures that we can find the global extrema of a function over a specific interval, which is often the goal in optimization problems.
What is the First Derivative Test used for in optimization?
-The First Derivative Test is used to determine whether a critical point of a function represents a local maximum, local minimum, or neither. It does this by examining the sign of the derivative on either side of the critical point: if the derivative changes from positive to negative, the function has a local maximum; if it changes from negative to positive, the function has a local minimum.
How does the Second Derivative Test help in identifying local extrema?
-The Second Derivative Test provides another method to classify critical points as local maxima, local minima, or neither. If the second derivative at a critical point is positive, the function has a local minimum at that point; if it's negative, the function has a local maximum. A zero value from the second derivative test makes the test inconclusive, and the First Derivative Test may be used instead.
What is the Candidates Test, and when is it used?
-The Candidates Test is used to find the absolute or global maximum and minimum values of a function on a closed interval. It involves evaluating the function at all critical points within the interval and at the endpoints, then comparing these values to determine the highest and lowest points.
How can the behavior of a function (increasing/decreasing) be determined from its derivative graph?
-The behavior of the original function in terms of increasing or decreasing can be determined from the derivative graph by looking at the sign of the derivative. If the derivative is positive (above the x-axis), the original function is increasing. If the derivative is negative (below the x-axis), the original function is decreasing.
What is an inflection point, and how can it be found?
-An inflection point is a point on the graph of a function where the concavity of the function changes, that is, where the function transitions from concave up to concave down or vice versa. Inflection points can be found by taking the second derivative of the function, setting it equal to zero, and solving for the variable. The solutions represent the x-values of the inflection points.
How does the graph of the second derivative relate to the concavity of the original function?
-The graph of the second derivative directly reflects the concavity of the original function. If the second derivative is positive at a point, the original function is concave up at that point. If the second derivative is negative, the original function is concave down. Zero crossing of the second derivative indicates an inflection point where the concavity changes.
What is the process for solving real-world optimization problems?
-The process for solving real-world optimization problems involves several steps: (1) identifying the function that models the real-world scenario, (2) transforming the equation into one variable by eliminating other variables, (3) differentiating the function to find the critical points, (4) using the first derivative test (and possibly the second derivative test) to classify these critical points as local maxima or minima, and (5) interpreting the results in the context of the real-world problem to answer the specific question asked.
Outlines
📚 Introduction to Unit 5 and Optimization
The paragraph introduces Unit 5, focusing on the use of derivatives for optimization. It mentions the availability of worksheets, practice problems, and tests for AP Calculus preparation. The unit is described as a crossroads, transitioning from derivatives to integrals in the AP Calculus course. Emphasis is placed on the importance of understanding optimization, particularly for the free-response questions on the AP test.
📈 Mean Value Theorem and its Applications
This paragraph discusses the Mean Value Theorem, explaining its significance in understanding the instantaneous and average rates of change within a function's interval. The theorem is applied to various real-world scenarios, such as road trips and daily temperature changes, to illustrate how the average rate of change can be equal to the instantaneous rate at some point within the interval. The paragraph also covers the conditions required for the theorem to apply, including the function's continuity and differentiability over the interval.
🔍 Extreme Value Theorem and Critical Points
The Extreme Value Theorem is introduced, stating that a continuous function on an open interval must have at least one minimum and one maximum point. The paragraph uses graphical examples to demonstrate how the theorem applies to different functions, including those that extend to infinity. The concept of local and global extrema is also explained, differentiating between maximum and minimum values within a specific interval versus the entire domain of a function.
📊 Increasing, Decreasing Behavior and Critical Points
This section delves into how to determine where a function is increasing or decreasing by using its derivative. It explains the process of finding critical points by setting the derivative equal to zero and solving for the variable. The behavior of the original function based on the sign of the derivative is discussed, with examples illustrating how to use the derivative to understand the function's increasing and decreasing intervals.
📈 Using Derivative Graphs to Understand Function Behavior
The paragraph explains how to use the graph of the derivative to understand the behavior of the original function. It describes how the sign of the derivative (positive or negative) relates to the original function's increasing or decreasing behavior. The concept of inflection points and concavity (concave up or down) is introduced, explaining how they can be identified from the second derivative and how they relate to the graph of the original function.
📊 First Derivative Test for Local Extrema
This section introduces the First Derivative Test, a method for determining whether a critical point represents a local maximum or minimum. The test involves analyzing the increasing and decreasing behavior of the function around the critical point. The paragraph provides a step-by-step explanation of the test, using examples to illustrate how to apply it to different functions and interpret the results.
📈 Candidates Test for Absolute Extrema
The Candidates Test is explained as a method for finding absolute or global extrema of a function over a specific interval. The test involves considering critical points within the interval and the endpoints, evaluating the function at these points, and comparing the values to determine the maximum and minimum. The paragraph walks through a detailed example, showing how to apply the test and interpret the results to find the global maximum and minimum values.
📊 Finding Inflection Points and Concavity
This paragraph focuses on identifying inflection points and determining the concavity of a function using the second derivative. It explains that inflection points occur where the second derivative is zero and discusses how the sign of the second derivative indicates whether the function is concave up or down. The process of finding inflection points by setting the second derivative to zero and solving for x is described, along with an example to illustrate the concept.
📈 Second Derivative Test for Local Extrema
The Second Derivative Test is introduced as an alternative to the First Derivative Test for identifying local maxima and minima. The paragraph explains how to apply the test by plugging critical points from the first derivative into the second derivative. It highlights that a negative result indicates a local maximum, while a positive result indicates a local minimum. The test's limitations are also discussed, noting that it is inconclusive if a critical point results in a zero value.
📊 Sketching Derivatives from Function Graphs
This section explains how to sketch the graphs of the derivative and second derivative functions based on the graph of the original function. It uses a visual chart to show the relationship between the features of the original function and its derivatives. The paragraph provides examples of how to translate information from the original function to its derivatives, emphasizing the importance of understanding the connection between the graphs of the three functions.
📈 Applying Optimization to Real-World Problems
The paragraph discusses the application of optimization to real-world problems, emphasizing the practical importance of finding maximum or minimum values in various contexts. It explains that the same steps used for optimization in mathematical functions can be applied to real-world scenarios, such as maximizing profit or area. The paragraph concludes by highlighting the importance of interpreting the results in the context of the problem and answering the specific question asked.
📊 Solving Real-World Optimization Problems
This section provides a step-by-step guide on solving real-world optimization problems, using a specific example of maximizing the volume of an open-top box made from a 10x10 inch paper. The process involves translating the problem into a mathematical equation, differentiating to find critical points, and using the first derivative test to identify the maximum or minimum value. The paragraph emphasizes the importance of restating the answer in the context of the original problem and cautions about ensuring the answer matches the question asked.
📊 Implicitly Defined Functions and Critical Points
The paragraph discusses the process of finding critical points for implicitly defined functions, which differs slightly from explicitly defined functions. It explains the steps of taking the derivative, applying the chain rule, and setting the derivative equal to zero to find critical points. The paragraph provides a detailed example of solving for critical points in an implicit function, highlighting the importance of substituting the derived equations back into the original function to find the critical points.
📚 Summary of Unit 5 and Transition to Integrals
The final paragraph summarizes the key concepts and skills covered in Unit 5, including the Mean Value Theorem, finding and classifying extrema using the first and second derivative tests, sketching the graphs of functions and their derivatives, and solving real-world optimization problems. It emphasizes the importance of understanding the relationship between the original function, its derivative, and second derivative. The paragraph concludes by encouraging students to move on to Unit 6, where the focus shifts to integrals.
Mindmap
Keywords
💡Derivatives
💡Mean Value Theorem
💡Optimization
💡Critical Points
💡First Derivative Test
💡Second Derivative Test
💡Increasing/Decreasing Function
💡Inflection Points
💡Concavity
💡Implicit Functions
Highlights
Worksheets and practice problems provided for AP Calculus preparation.
Unit 5 focuses on using derivatives for optimization, a common topic on the AP test.
Mean Value Theorem states that within a given interval, there exists a point where the instantaneous rate of change equals the average rate of change.
Function must be continuous and differentiable over a closed interval to apply the Mean Value Theorem.
The Extreme Value Theorem guarantees at least one minimum and one maximum point on a continuous open interval.
Critical points are found by setting the derivative of a function equal to zero and solving for the variable.
The behavior of a function (increasing/decreasing) can be determined using its derivative.
The first derivative test is used to identify local maxima and minima based on the sign change of the derivative.
The second derivative test helps to classify critical points as local maxima, minima, or neither by examining the concavity.
Inflection points are identified by setting the second derivative equal to zero and can change the concavity of a function.
The graph of a function and its derivatives can be sketched based on the behavior and critical points of the original function.
Applied optimization problems involve translating mathematical concepts to real-world scenarios, such as maximizing profit or area.
Implicitly defined functions require the use of chain rule and algebraic manipulation to find critical points and analyze behavior.
The first and second derivative tests can be applied to implicitly defined functions by treating y as a function of x and solving for y'.
Unit 5 concludes with the transition from derivatives to integrals, marking the end of the first half of the AP Calculus course.
Transcripts
Browse More Related Video

Calculus Review Maximum and Minimum Values of Functions
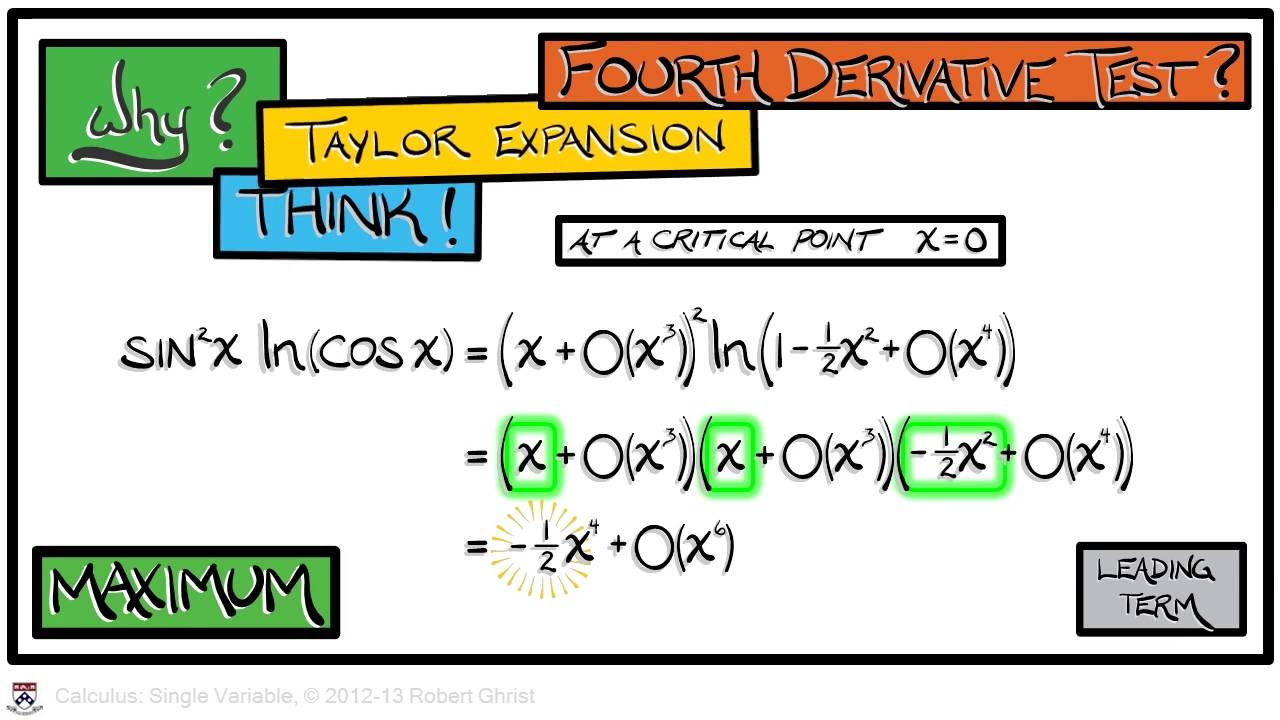
Calculus Chapter 2 Lecture 14 Optimization
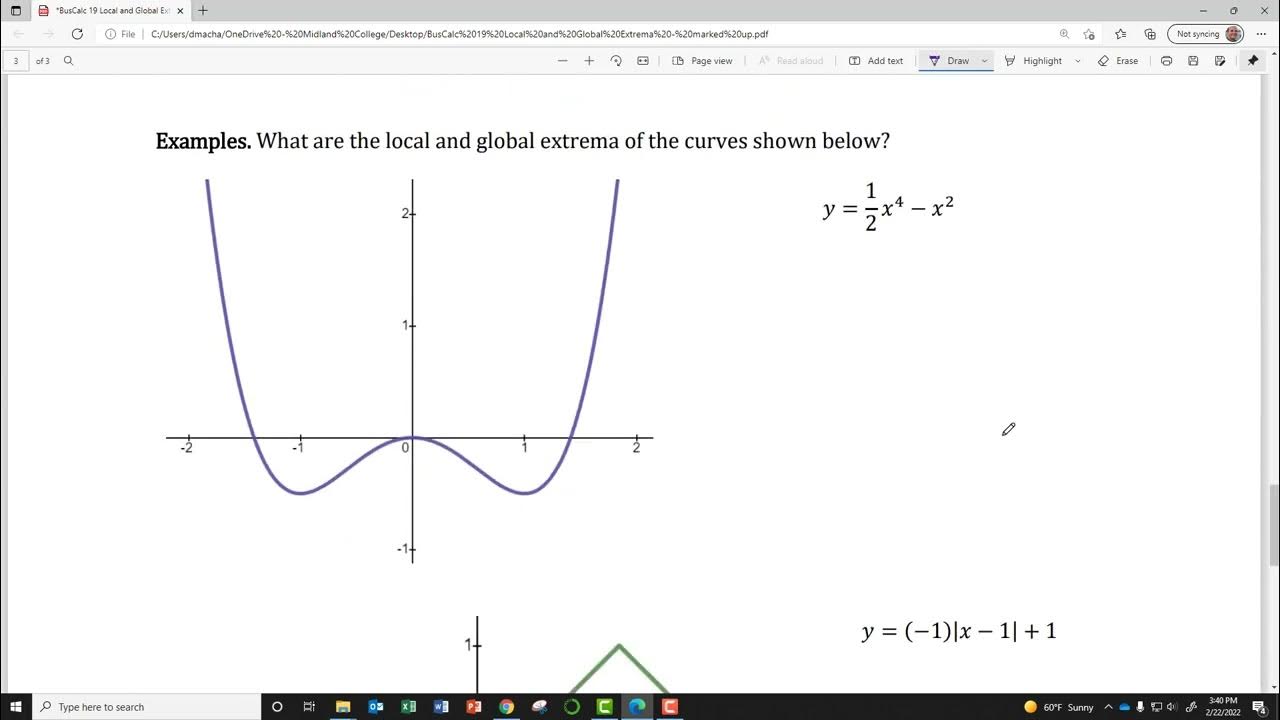
BusCalc 19 Local and Global Extrema

Calculus AB Homework 4.5: Second Derivative Test
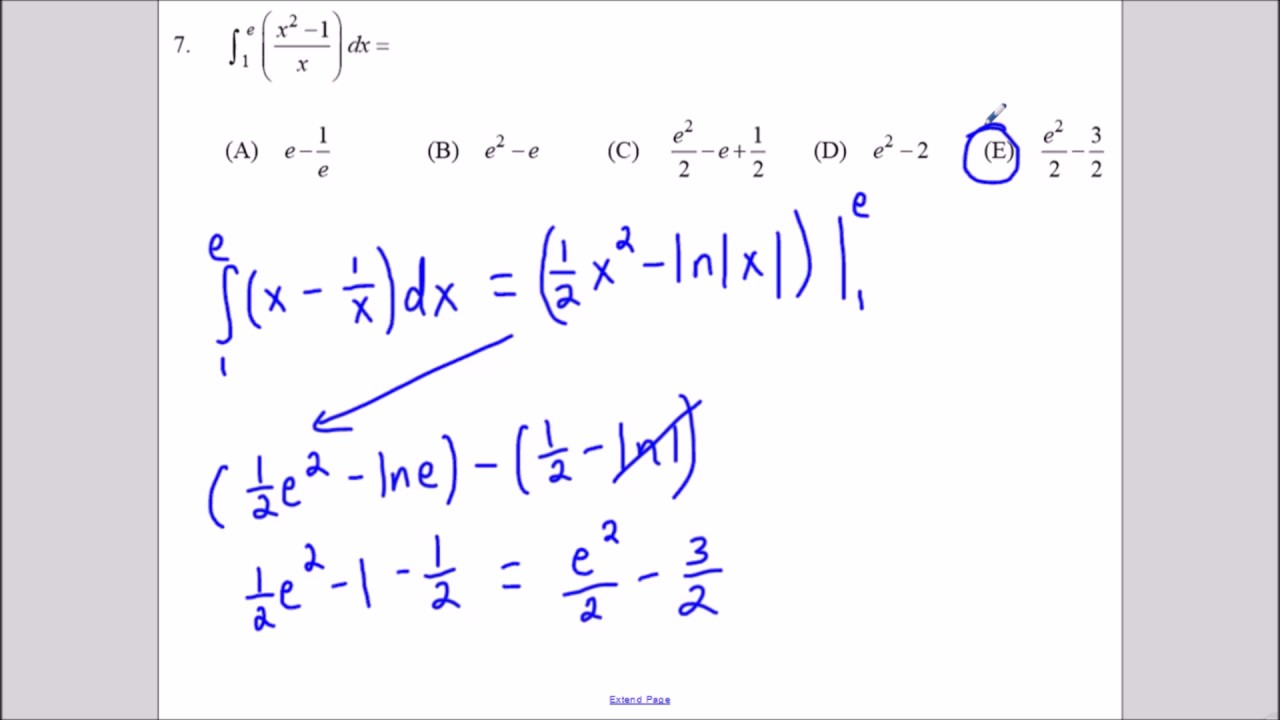
AP Calculus AB 1998 Multiple Choice No Calculator

Calculus 3: Maximum and Minimum Values (Video #17) | Math with Professor V
5.0 / 5 (0 votes)
Thanks for rating: