Rolle's Theorem with Examples

Mario's Math Tutoring
27 Jul 201606:35
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video, Mario from Mario's Math Tutoring explains Rolle's Theorem, a fundamental concept in calculus that states if a function is continuous on a closed interval and differentiable on the open interval with equal endpoints, there exists at least one point where the derivative is zero. Mario illustrates the theorem with a clear example and emphasizes the importance of ensuring the function meets the criteria of continuity, differentiability, and equal endpoint values. He also highlights the potential for multiple points of tangency and sets the stage for a follow-up video on the Mean Value Theorem.

Takeaways
  • 📚 Rolle's Theorem is a fundamental concept in calculus that helps identify points on a continuous and differentiable function where the derivative equals zero.
  • 📈 The theorem applies to functions that are continuous on a closed interval and differentiable on the open interval between two endpoints.
  • 🔍 For Rolle's Theorem to be applicable, the function's values at the endpoints must be equal, indicating a potential 'bend' or 'turn' in the graph.
  • 📉 The theorem guarantees the existence of at least one point 'c' within the interval where the derivative (slope of the tangent line) is zero, indicating a horizontal tangent.
  • 📝 Mario's math video provides a clear definition and explanation of Rolle's Theorem, making it accessible for students to understand and apply.
  • 📊 The script uses a graphical approach to illustrate the concept, showing how a function's graph can have a horizontal tangent line between two points with the same y-value.
  • 🔢 An example is given with the function f(x) = x^2 - 2x + 1, demonstrating how to apply Rolle's Theorem by finding where the derivative equals zero within the interval.
  • 📌 The process involves taking the derivative of the function, setting it to zero, and solving for 'x' to find the point where the tangent is horizontal.
  • 🚫 The video script also highlights cases where Rolle's Theorem does not apply, such as when a function is not continuous over the interval, using the function x^2 - 4/x as an example.
  • 📚 The importance of checking the conditions for continuity, differentiability, and equal y-values at endpoints is emphasized before applying Rolle's Theorem.
  • 🔄 The video promises a follow-up on the Mean Value Theorem, which is an extension of Rolle's Theorem, indicating a series of educational content on related topics.
Q & A

  • What is Rolle's Theorem?

    -Rolle's Theorem states that if a function is continuous on the closed interval [a, b], differentiable on the open interval (a, b), and f(a) = f(b), then there exists at least one c in the interval (a, b) such that the derivative f'(c) equals zero.

  • What does it mean for a function to be continuous?

    -A function is continuous if there are no breaks in its graph, meaning you can draw the graph without picking up your pen or pencil.

  • What is the significance of a function being differentiable?

    -A function is differentiable if at every point on its curve, you can find the slope of the tangent line, indicating that the function is smooth without corners or cusps.

  • What does it imply if f(a) equals f(b) for a function?

    -If f(a) equals f(b), it implies that the function has the same y-value or output at both endpoints a and b, which means the graph of the function reaches the same height at these points.

  • Why does Rolle's Theorem require the condition that f(a) = f(b)?

    -The condition f(a) = f(b) is required because it ensures that the function must 'bend' or change direction somewhere between a and b to return to the same y-value, allowing for the possibility of a horizontal tangent line.

  • Can Rolle's Theorem be applied to any function?

    -No, Rolle's Theorem can only be applied to functions that are continuous on a closed interval and differentiable on the corresponding open interval, with the same y-values at the endpoints.

  • What does the derivative being equal to zero signify in the context of Rolle's Theorem?

    -In the context of Rolle's Theorem, a derivative equal to zero signifies a horizontal tangent line to the function's graph at a certain point c in the interval (a, b).

  • What is an example of a function that satisfies the conditions of Rolle's Theorem?

    -An example is the function f(x) = x^2 - 2x + 1 with the interval [-1, 3]. It is continuous, differentiable, and f(-1) = f(3) = 4, so Rolle's Theorem can be applied.

  • Why can't Rolle's Theorem be applied to the function f(x) = x^2 - 4 divided by x with the interval [-2, 2]?

    -Rolle's Theorem cannot be applied because the function has a vertical asymptote at x = 0, making it discontinuous on the interval [-2, 2], and thus not satisfying the conditions for the theorem.

  • What is the relationship between Rolle's Theorem and the Mean Value Theorem?

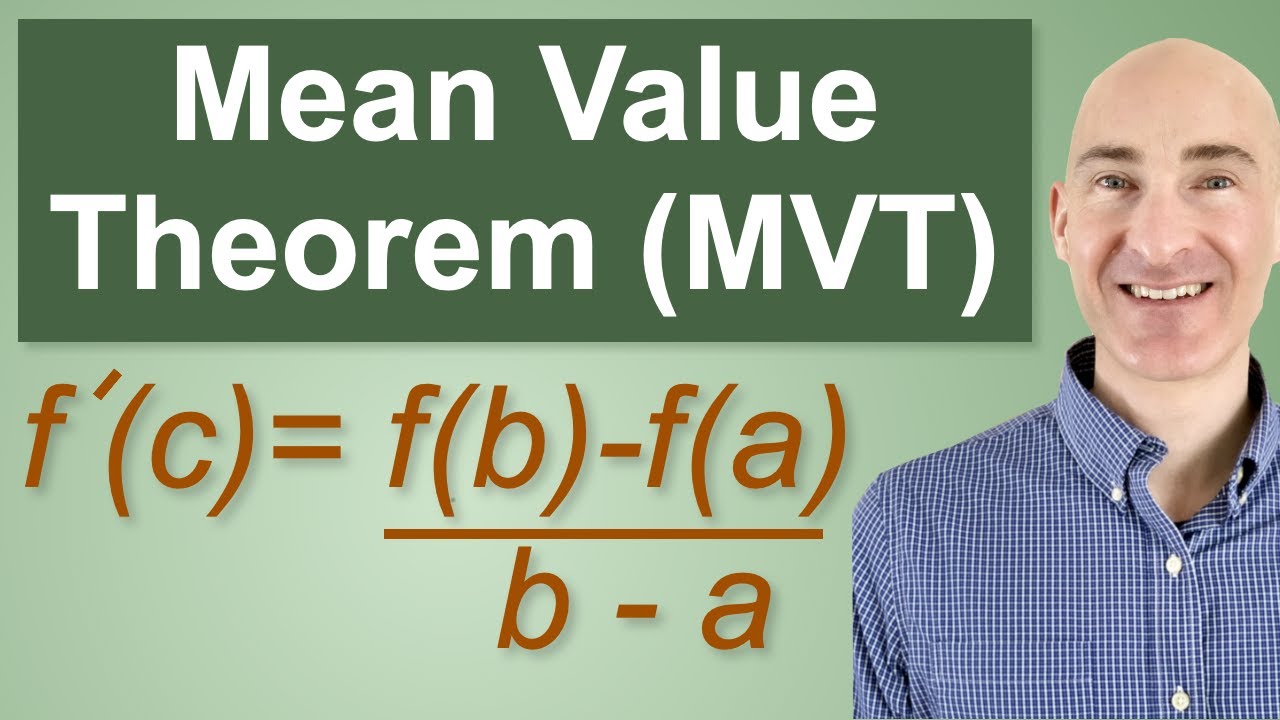
    -The Mean Value Theorem is an extension of Rolle's Theorem. While Rolle's Theorem requires the function to have the same y-values at the endpoints, the Mean Value Theorem does not have this requirement and states that there must be at least one point in the interval where the derivative equals the average rate of change of the function over that interval.

Outlines
00:00
📚 Introduction to Rolle's Theorem

In this video, Mario from Mario's Math Tutoring introduces Rolle's Theorem, a fundamental concept in calculus. He explains the theorem's definition, which states that if a function is continuous on a closed interval and differentiable on the open interval, with the same function values at the endpoints, there must be at least one point 'C' within the interval where the derivative is zero, indicating a horizontal tangent line. Mario uses a graphical representation to illustrate the continuous and differentiable nature of a function and how the theorem applies when the function values at the endpoints are equal. He then begins an example with a polynomial function to demonstrate how to apply Rolle's Theorem.

05:01
📘 Applying Rolle's Theorem with Examples

Mario continues the tutorial by applying Rolle's Theorem to a specific example involving the function f(x) = x^2 - 2x + 1 over the interval [-1, 3]. He verifies the conditions for Rolle's Theorem: the function is continuous and differentiable, and the function values at the endpoints are equal. After finding the derivative, he sets it equal to zero to find the point where the tangent line is horizontal, concluding that this occurs at x = 1. Mario also discusses the importance of checking the conditions before applying the theorem. He then presents a second example with a function that has a vertical asymptote at x = 0, explaining why Rolle's Theorem does not apply in this case due to the discontinuity. The video concludes with a mention of a future video on the Mean Value Theorem, an extension of Rolle's Theorem, and an invitation for viewers to subscribe and explore more content.

Mindmap
Keywords
💡Rolle's Theorem
Rolle's Theorem is a fundamental concept in calculus that states if a function is continuous on a closed interval and differentiable on the open interval, and if the function values at the endpoints are equal, then there exists at least one point 'c' within the interval where the derivative of the function is zero. In the video, Rolle's Theorem is the central theme, with the script explaining its definition and providing examples to illustrate its application.
💡Continuous
Continuous, in the context of calculus, refers to a function that has no breaks or gaps in its graph. It ensures that the function can be drawn without lifting the pen from the paper. In the video, the concept of continuity is used to establish one of the conditions for Rolle's Theorem, where the function must be continuous on the closed interval [a, b].
💡Differentiable
Differentiable means that a function has a derivative at every point in its domain, indicating that the function has a well-defined slope at every point. In the script, differentiability is a key condition for Rolle's Theorem, as it requires the function to have a derivative on the open interval (a, b), excluding the endpoints.
💡Function
A function in mathematics is a relation between a set of inputs and a set of permissible outputs, with the property that each input is related to exactly one output. The video discusses functions in the context of Rolle's Theorem, where the function's properties, such as continuity and differentiability, are crucial for the theorem's application.
💡Closed Interval
A closed interval in mathematics includes all the numbers between its endpoints, including the endpoints themselves. In the video, the closed interval [a, b] is the domain over which the function must be continuous for Rolle's Theorem to apply.
💡Open Interval
An open interval includes all the numbers between its endpoints but does not include the endpoints themselves. The script explains that the function must be differentiable on the open interval (a, b), which is a condition for Rolle's Theorem to hold.
💡Derivative
The derivative of a function at a given point is the rate at which the function's value changes with respect to changes in its input. In the video, finding the point where the derivative is zero is the goal of applying Rolle's Theorem, as it represents the point where the tangent line to the function's graph is horizontal.
💡Tangent Line
A tangent line is a straight line that touches a curve at a single point without crossing it. In the context of the video, the tangent line's slope being zero is a key outcome of Rolle's Theorem, indicating a horizontal line at the point where the derivative of the function is zero.
💡Slope
Slope, in the context of a line, is a measure of its steepness, indicating the ratio of the vertical change to the horizontal change between any two points on the line. The video discusses the slope of the tangent line, which becomes zero at the point satisfying Rolle's Theorem.
💡Vertical Asymptote
A vertical asymptote is a vertical line that the graph of a function approaches but never crosses, typically occurring where the function's denominator is zero. In the script, the concept is used to explain why Rolle's Theorem does not apply to a function with a vertical asymptote within the interval, as the function is not continuous.
💡Polynomial
A polynomial is an algebraic expression consisting of variables and coefficients, involving only the operations of addition, subtraction, multiplication, and non-negative integer exponents of variables. The video uses a polynomial function as an example to demonstrate the application of Rolle's Theorem, as polynomials are inherently continuous and differentiable everywhere.
Highlights

Introduction to Rolle's Theorem and its significance in boosting math scores and understanding.

Definition of Rolle's Theorem with conditions for a function to be continuous, differentiable, and having equal endpoints' values.

Explanation of the continuity condition with a visual graph example.

Clarification of differentiability meaning the function can have a derivative without corners or cusps.

Illustration of the theorem's condition where the function values at endpoints are the same, leading to a horizontal tangent line somewhere in between.

Rolle's Theorem application in finding points where the tangent line is horizontal on a graph.

Example 1: Applying Rolle's Theorem to a polynomial function with the interval (-1, 3) and finding the point where the derivative equals zero.

Step-by-step process of finding the derivative and setting it to zero to find the critical point in the first example.

Graphical representation of the parabola and identification of the horizontal tangent line at the midpoint.

Explanation that the horizontal tangent point is not always at the midpoint, emphasizing the importance of conditions for Rolle's Theorem.

Example 2: Analyzing a function with a denominator that leads to a vertical asymptote and explaining why Rolle's Theorem does not apply.

Emphasizing the importance of checking for continuity and differentiability before applying Rolle's Theorem.

Teaser for the next video on the Mean Value Theorem as an extension of Rolle's Theorem.

Encouragement to subscribe for more math tutorials and a promise of continued support in future videos.

Mario's Math's commitment to making learning math less stressful and more understandable.

Transcripts

Browse More Related Video

Calculus 1 Lecture 3.2: A BRIEF Discussion of Rolle's Theorem and Mean-Value Theorem.

Mean Value Theorem with Example

The MEAN Value Theorem is Actually Very Nice

Mean Value Theorem and Rolle's Theorem

AP Calculus Review Three Theorems You Must Know (EVT, IVT, MVT)

Rolle’s Theorem Proof

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Rolle's TheoremCalculus TutorialMath TutoringDerivative ApplicationGraph AnalysisContinuous FunctionsDifferentiable GraphsTangent SlopeMidpoint TheoremMario's MathEducational Content