AP Calculus AB and BC Unit 1 Review [Limits and Continuity]
TLDRThis comprehensive video script covers the foundational concepts of calculus, focusing on limits, derivatives, and integrals. It explains how to evaluate limits from functions, tables, and graphs, and introduces various methods for solving limits, including substitution, factoring, conjugate method, and squeeze theorem. The script also delves into the concepts of continuity and discontinuity, detailing how to address removable discontinuities and understand different types of asymptotes. Finally, it touches on the intermediate value theorem, reinforcing the importance of these concepts for AP Calculus preparation.
Takeaways
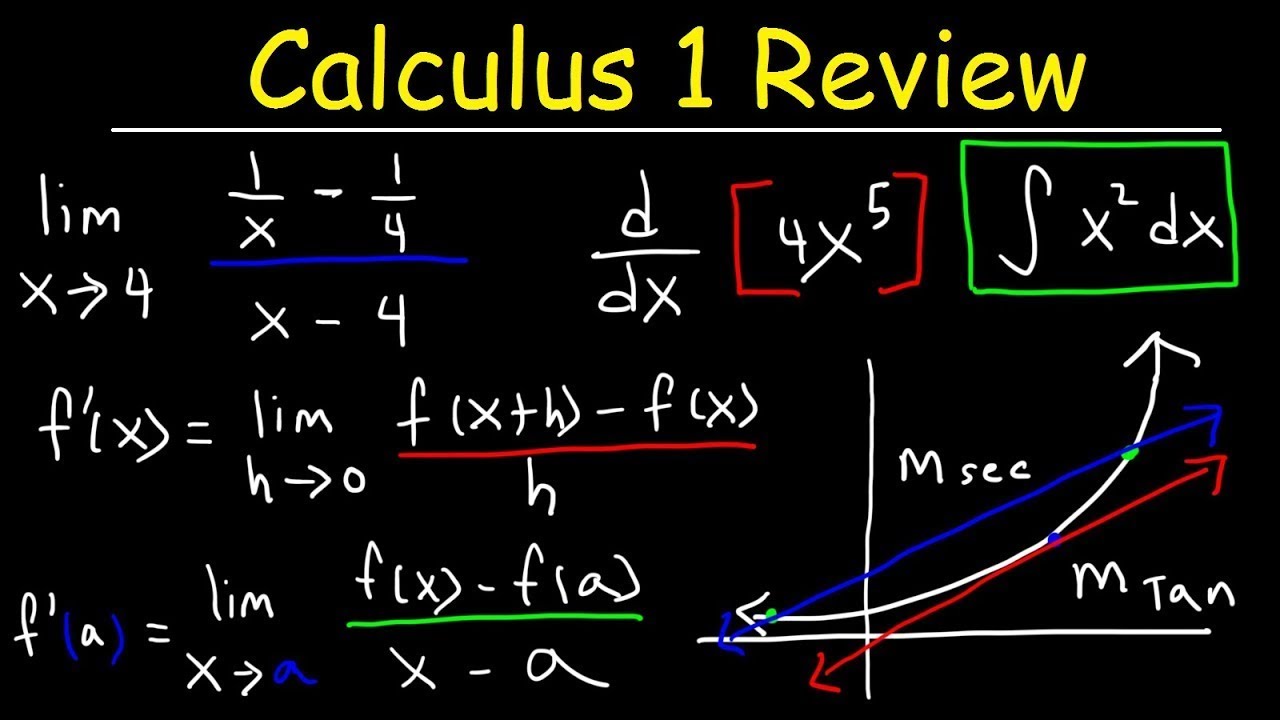
- 📈 The main concepts in calculus are derivatives, integrals, and limits, with limits being foundational for the course.
- 🔄 Average rate of change is the difference in Y divided by the difference in X, while instantaneous rate of change involves limits to narrow the interval to a specific point.
- 📊 Understanding limits involves recognizing one-sided limits (left and right) and general limits, which exist if the one-sided limits are equal.
- 📐 The process of finding instantaneous rate of change involves applying limits to the difference quotient formula, allowing for the interval to approach zero.
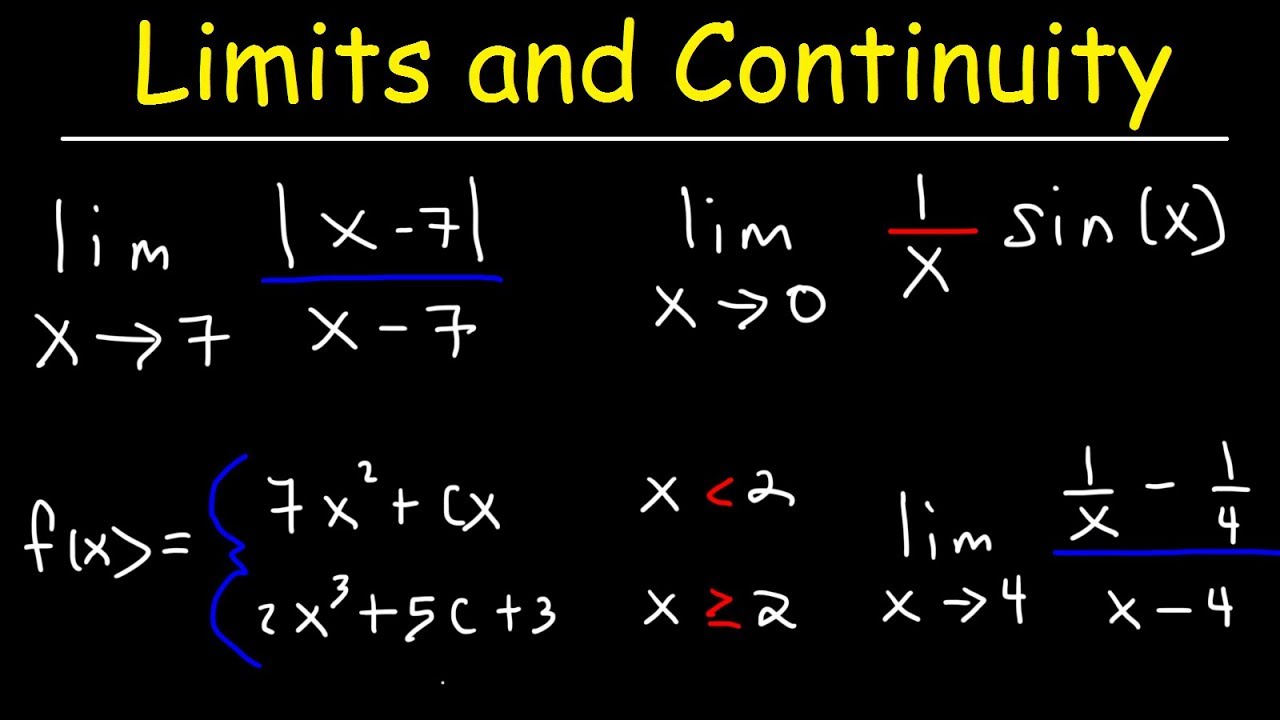
- 📝 Estimating limits can be done from graphs, tables, or by using algebraic manipulation such as factoring and the conjugate method.
- 🔢 Limit rules or properties include the ability to pull out constants, take limits term by term in sums and differences, and handle products and quotients.
- 🌀 Trigonometric limits often involve matching the given function to known trigonometric functions with established limit values.
- 📈 Squeeze theorem is used to find limits by bounding the function between two known functions with established limits.
- 🔄 Continuity in a function is determined by the existence of the function's value at a point, the existence of the limit, and the equality of the function's value and the limit.
- 🔧 Discontinuities such as jump, infinite, and point discontinuities can be identified and, in some cases, removable discontinuities can be addressed by redefining the function.
Q & A
What are the main concepts covered in Unit 1 of the calculus course?
-Unit 1 covers the foundational concept of limits, which are essential for understanding derivatives and integrals covered in subsequent units. It also introduces different ways to represent and estimate limits, methods for solving limits, and the intermediate value theorem.
How does the average rate of change differ from the instantaneous rate of change?
-The average rate of change is calculated using the formula (Delta Y / Delta X) and represents the change in Y values divided by the change in X values over an interval. In contrast, the instantaneous rate of change is found by taking the limit as the interval (Delta X) approaches zero, which gives the slope of the function at a specific point.
What is the difference quotient formula and how is it used in finding limits?
-The difference quotient formula is used to find the average rate of change of a function over an interval. It is represented as (f(x) - f(a)) / (x - a) or (f(x + h) - f(x)) / h. By applying limits to this formula, we can find the instantaneous rate of change at a particular point, which is the derivative of the function at that point.
How can you estimate the average rate of change from a table of values?
-To estimate the average rate of change from a table, you select two points on either side of the value you're interested in, calculate the change in Y (Delta Y) and the change in X (Delta X), and then use the formula (Delta Y / Delta X) to find the average rate of change.
What is the intermediate value theorem and what does it imply?
-The intermediate value theorem states that if a function is continuous on an interval and takes on values of opposite signs at the endpoints of the interval, then it must also take on every value between those two extremes within the interval. This implies that a continuous function will cross the x-axis somewhere within the interval if it has different signs at the endpoints.
How can you determine if a limit exists at a particular point on a graph?
-To determine if a limit exists at a point on a graph, you need to check if the left-hand limit (approaching the point from the left) and the right-hand limit (approaching the point from the right) both exist and are equal. If these conditions are met, the general limit exists and is equal to the common value of the left and right limits.
What are the different types of discontinuities mentioned in the script?
-The script mentions jump discontinuities, infinite discontinuities (also known as vertical asymptotes), and point or removable discontinuities. Jump discontinuities occur when there is a gap in the function, infinite discontinuities occur at vertical asymptotes, and point or removable discontinuities occur when the function has a single point that is not part of the curve.
How do you define a function as continuous at a point?
-A function is considered continuous at a point if the function's value at that point exists, the limit as X approaches that point exists, and the limit equals the function's value at that point. In other words, there is no break or hole in the graph at that point, and the function's value does not 'jump' or change abruptly.
What are horizontal asymptotes and how do they relate to limits?
-Horizontal asymptotes are lines that a graph of a function approaches as the variable X approaches positive or negative infinity. They represent the end behavior of a function and are used to describe the limit of a function as X goes to infinity or negative infinity. The existence and position of a horizontal asymptote depend on the relationship between the highest degree terms in the numerator and denominator of a rational function.
How can you use the intermediate value theorem to find roots of a function?
-The intermediate value theorem can be used to find roots of a function by showing that the function changes sign over an interval. If the function is continuous on the interval and takes on a negative value at one endpoint and a positive value at the other, the theorem guarantees that there is at least one root within that interval where the function crosses the x-axis.
Outlines
📚 Introduction to Calculus: Derivatives, Integrals, and Limits
This paragraph introduces the foundational concepts of calculus, emphasizing the importance of limits in understanding derivatives and integrals. It outlines the main topics covered in the course, including the derivative (units 2-5), the integral (units 6-8), and the limit, which is crucial for both. The paragraph also mentions the availability of practice materials and highlights the transition from average rate of change to instantaneous rate of change as a key idea in limits.
🔢 Understanding Limits and Average Rate of Change
This section delves deeper into the concept of limits, explaining how they allow for the calculation of instantaneous rates of change. It contrasts this with the average rate of change, which is calculated using the formula ΔY/ΔX. The paragraph uses a graphical example to illustrate how the average rate of change can be found between two points on a graph and how limits refine this to a single point, providing the instantaneous rate of change. It also introduces the difference quotient formula and its role in calculating limits.
📈 Estimating Limits from Graphs and Tables
This paragraph discusses the methods for estimating limits from graphical and tabular data. It explains how to interpret one-sided and general limits from a graph and how to identify points of discontinuity. The section also covers how to estimate limits from a table of values by using the difference quotient formula with points on either side of the given value. The importance of understanding limit notation and the behavior of functions as they approach certain values is emphasized.
📊 Analyzing Discontinuities and Continuity
This section focuses on identifying and understanding different types of discontinuities, such as jump, point, and infinite discontinuities, using graphical examples. It also explains how to determine the continuity of a function by examining the existence of function values, limits, and their equality at a given point. The concept of a cusp, which is a sharp point in a graph but not a discontinuity, is introduced. The definition of continuity is provided, stating that a function is continuous at a point if the function value exists, the limit exists, and both are equal.
🔧 Solving Limits Using Algebraic Techniques
This paragraph outlines various algebraic techniques for solving limits, including factoring, conjugate method, trigonometric limits, and squeeze theorem. It explains how to manipulate the function algebraically to evaluate limits, especially in cases where direct substitution results in an indeterminate form. The paragraph provides examples of each method, demonstrating how they can be applied to different types of limit problems and emphasizing the order in which these techniques should be attempted.
📐 Applying Squeeze Theorem and Understanding Discontinuities at Vertical Asymptotes
This section introduces the squeeze theorem as a method for evaluating limits when direct substitution is not possible. It provides a step-by-step example of how to use the theorem with a trigonometric function. The paragraph also discusses the concept of vertical asymptotes, explaining how to identify them and how they relate to one-sided limits of positive or negative infinity. The difference between removable and non-removable discontinuities is highlighted, with an emphasis on the importance of understanding these concepts for the AP Calculus exam.
🔄 Converting Between Limit Notation, Tables, and Graphs
This paragraph explains the interplay between limit notation, tables of values, and graphical representations. It demonstrates how to translate between these different forms to understand and solve problems involving limits. The importance of being comfortable with these translations is emphasized, as it is crucial for success in the AP Calculus course and exam.
🚫 Identifying and Handling Different Types of Discontinuities
This section provides a comprehensive overview of different types of discontinuities, including jump, point, infinite, and removable discontinuities. It uses a graphical example to illustrate each type and explains how to identify them within a function's graph. The paragraph also discusses the concept of continuity in relation to discontinuities, clarifying that a function is continuous if there are no breaks or holes in its graph.
📝 Removing Removable Discontinuities in Functions
This paragraph focuses on the process of removing removable discontinuities in functions, particularly in rational functions and piecewise functions. It explains how to redefine a function as a piecewise function to eliminate the discontinuity at a specific point, ensuring continuity across the entire function. The paragraph provides a step-by-step method for solving for the value that will achieve continuity, using a given piecewise function as an example.
🌐 Understanding Vertical and Horizontal Asymptotes
This section differentiates between vertical and horizontal asymptotes, explaining their relationship with infinity and the end behavior of functions. It describes how to determine the presence and location of horizontal asymptotes by comparing the degrees of the leading terms in the numerator and denominator of rational functions. The concept of one-sided limits at vertical asymptotes and the end behavior at horizontal asymptotes are also discussed, with examples to illustrate the ideas.
📈 Applying the Intermediate Value Theorem
This paragraph explains the Intermediate Value Theorem, which states that if a function is continuous on an interval and takes on opposite signs at the endpoints of the interval, then it must have a root within that interval. The theorem is used to demonstrate the existence of a zero-crossing point for a given function over a specified interval. The explanation includes an algebraic example to show how the theorem can be applied to determine the presence of a root.
Mindmap
Keywords
💡Derivative
💡Integral
💡Limit
💡Average Rate of Change
💡Instantaneous Rate of Change
💡Continuity
💡Discontinuity
💡Intermediate Value Theorem
💡Horizontal Asymptote
💡Vertical Asymptote
Highlights
The main concepts of calculus are the derivative and the integral, with limits being foundational to the course.
The transition from average rate of change to instantaneous rate of change is central to understanding limits.
The difference quotient formula is used to calculate average rate of change and can be manipulated to find instantaneous rate of change through limits.
The intermediate value theorem is a key concept that asserts if a function is continuous on an interval, it must take on all values between the function values at the endpoints of the interval.
The concept of continuity and discontinuity is crucial in calculus, with different types of discontinuities such as jump, point, and infinite discontinuities.
The definition of continuity requires that a function is defined at a point, the limit exists at that point, and the limit value equals the function's value at that point.
Polynomial, rational, power, exponential, logarithmic, and trigonometric functions are all continuous within their domains.
A function is continuous over an interval if it is continuous at every point within that interval.
Removable discontinuities can be addressed by redefining the function as piecewise, ensuring continuity at the problematic point.
Vertical asymptotes occur where the denominator of a rational function is zero, leading to one-sided limits of infinity or negative infinity.
Horizontal asymptotes describe the end behavior of a function, with the function approaching a specific y-value or infinity as x approaches positive or negative infinity.
The relationship between the degrees of the leading terms in the numerator and denominator of a rational function determines the presence and position of horizontal asymptotes.
The intermediate value theorem is a powerful tool for proving the existence of roots within a given interval for continuous functions.
The process of evaluating limits involves translating between different representations such as tables, graphs, and function notation.
Various methods for solving limits include substitution, factoring, conjugate method, squeeze theorem, and trigonometric limits.
Understanding and applying the rules of limits is essential for solving calculus problems and comprehending the behavior of functions.
The concept of limits is not only fundamental to calculus but also critical for describing the behavior of functions near specific points and intervals.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: