The Most Unusual Ways Pi Shows Up In Mathematics | Can You Explain These?

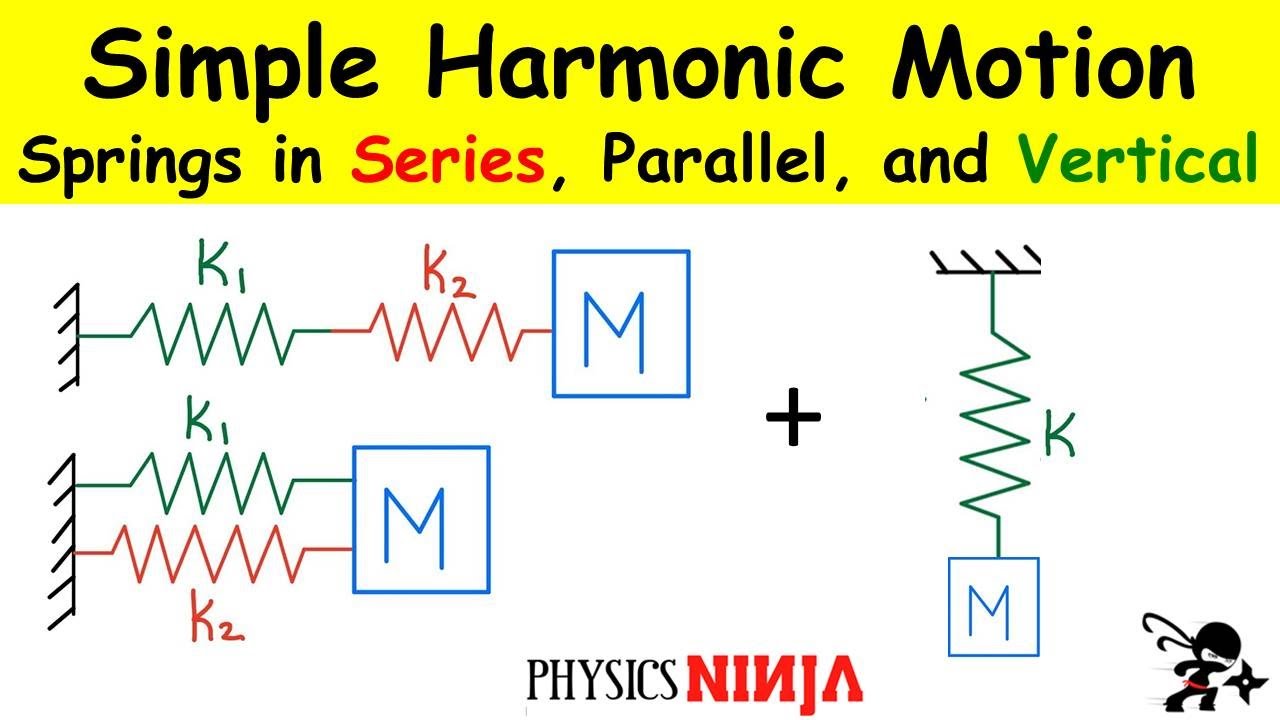
This paragraph delves into the fascinating ways the mathematical constant Pi appears in various unexpected scenarios. It starts by discussing the presence of Pi in the equation for the period of an object in circular orbit and the time it takes for a mass on a spring to complete one cycle. It also mentions a surprising demonstration by the YouTuber 'Three Blue One Brown', where the number of collisions in a two-block system equates to the digits of Pi. The script then introduces the concept of 'Buffon's Needle', a famous probability experiment where the ratio of dropped needles to those intersecting a line approximates Pi. The explanation touches on the geometric reasoning behind this phenomenon, involving the probability of the needle's angle and its vertical distance from the center. The paragraph concludes with a mention of other series and products where Pi appears, some of which are intuitively clear due to the presence of a circle, while others are more mysterious.

The second paragraph explores the appearance of Pi in mathematical formulas that may not seem to involve circles at first glance. It discusses Sterling's approximation, which approximates the factorial of an integer, and how Pi and e appear in this formula. The script also highlights a modification to Sterling's formula that significantly improves its accuracy. Moving on, the paragraph touches on series where Pi unexpectedly appears, such as an alternating sum of odd reciprocals and the Wallace product, which are known to equal Pi-related values. The focus then shifts to Ramanujan's formula for calculating Pi, which is highly efficient and can provide a high degree of precision with relatively few terms. The Gaussian integral is also mentioned, where the area under the curve is the square root of Pi, and the paragraph concludes with a discussion of random walks, explaining how the expected distance from the origin after a series of coin flips relates to Pi.

This paragraph examines the role of Pi in probability and physical constants. It starts with a discussion on the probability of two randomly chosen numbers being co-prime, which is related to Pi squared. The script then connects this probability to the Riemann zeta-function and the infinite sum that equates to Pi squared over 6. The paragraph also covers the physical significance of Pi, mentioning its presence in physical constants such as the magnetic permeability of free space and the buckling equation, which describes the force an ideal column can support before becoming unstable. The video concludes by highlighting the educational value of exploring these mathematical mysteries and puzzles, and it promotes CuriosityStream, a streaming service that offers documentaries and nonfiction titles on a wide range of subjects. The sponsor is thanked for their support, and an offer for a free month of membership using a promo code is provided to the audience.