Simplifying Trigonometric Expressions

The Organic Chemistry Tutor
16 Feb 202212:15
EducationalLearning
32 Likes 10 Comments

TLDRThis video tutorial teaches viewers how to simplify trigonometric expressions using key formulas and identities. It covers reciprocal identities, trigonometric Pythagorean identities, and demonstrates step-by-step simplification of various expressions, emphasizing the importance of recognizing and applying these mathematical relationships.

Takeaways
  • 📝 The video provides a tutorial on simplifying trigonometric expressions using specific formulas and identities.
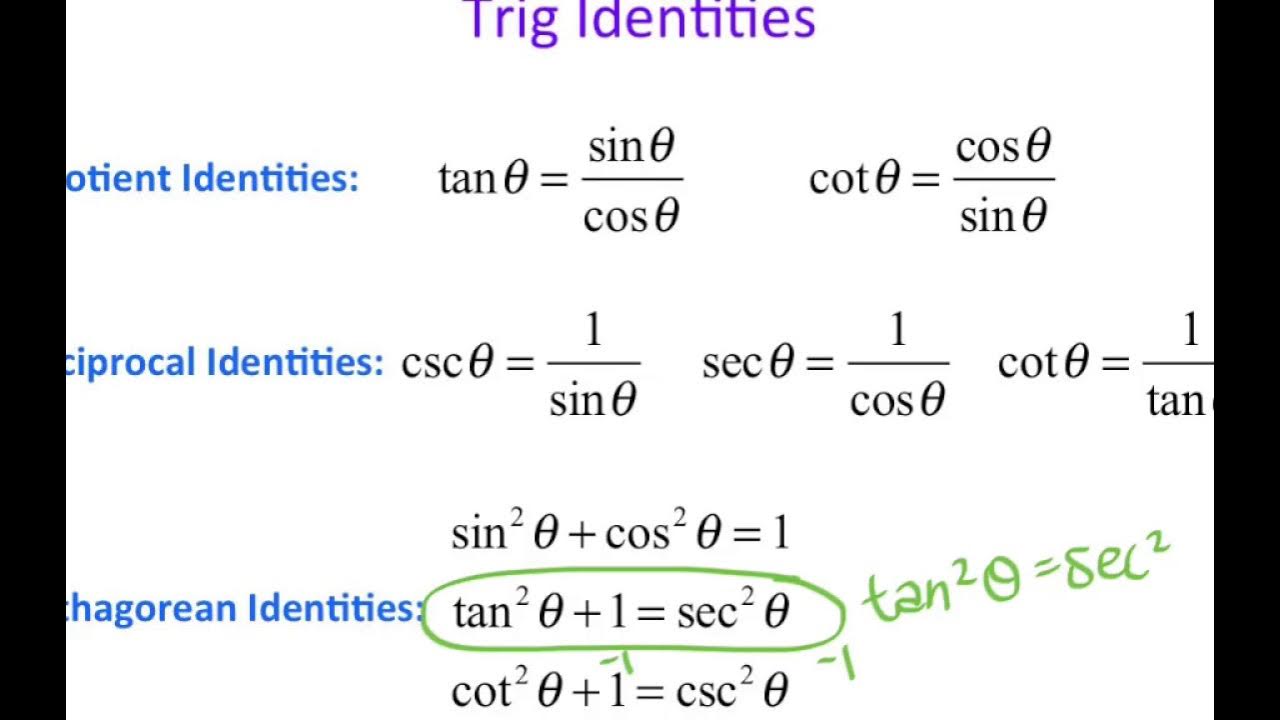
  • 📚 Essential formulas to remember include the reciprocal identities for secant and cosecant, and the definitions of tangent and cotangent.
  • 🔍 The script emphasizes the importance of understanding and applying the trigonometric Pythagorean identities, such as sine squared plus cosine squared equals one.
  • 📉 The first example demonstrates simplifying an expression by canceling out terms, resulting in sine theta.
  • 📶 The second example uses the Pythagorean identity to simplify an expression involving tangent squared, sine squared, and cosine squared to secant squared theta.
  • 🔢 The third example simplifies an expression by canceling terms and converting sine squared times cosecant to tangent theta.
  • 🔄 The fourth example shows that distributing and converting cotangent and tangent to their respective sine and cosine forms simplifies the expression to one.
  • 🔄 The fifth example uses the Pythagorean identity to replace one minus sine squared with cosine squared, simplifying the expression to one.
  • 📌 In the sixth example, the expression is simplified by converting tangent to sine over cosine and combining fractions to get secant theta.
  • 🔑 The seventh example involves using reciprocal identities and distributing sine theta to simplify a complex fraction to one.
  • 📈 The video concludes by reinforcing the use of reciprocal and Pythagorean identities in simplifying various trigonometric expressions.
Q & A

  • What are the reciprocal identities in trigonometry?

    -The reciprocal identities in trigonometry are secant (sec) being equal to 1 over cosine (cos), and cosecant (csc) being equal to 1 over sine (sin).

  • What is the relationship between tangent and sine and cosine?

    -Tangent (tan) is equal to sine (sin) divided by cosine (cos).

  • How is cotangent related to sine and cosine?

    -Cotangent (cot) is equal to cosine (cos) divided by sine (sin).

  • What is the first trigonometric Pythagorean identity mentioned in the script?

    -The first trigonometric Pythagorean identity mentioned is sin²θ + cos²θ = 1.

  • What is the relationship between tangent squared and secant squared?

    -The relationship is that 1 + tan²θ = sec²θ.

  • How does the script simplify the expression secant over sine?

    -The script simplifies secant over sine by recognizing that secant is 1 over cosine and then canceling out the cosine in the numerator and denominator.

  • What is the simplified form of the expression tan²θ + sin²θ + cos²θ?

    -The expression simplifies to sec²θ by using the Pythagorean identity sin²θ + cos²θ = 1.

  • How does the script simplify the expression sin² * csc * sec?

    -The script simplifies it by recognizing that csc² is equivalent to 1/sin and sec is 1/cos, then canceling out the sin in the numerator and denominator, resulting in tanθ.

  • What is the simplified form of the expression cot * tan + cot²?

    -The expression simplifies to csc²θ by converting cot to cos/sin and tan to sin/cos, and then using the identity 1 + cot² = csc².

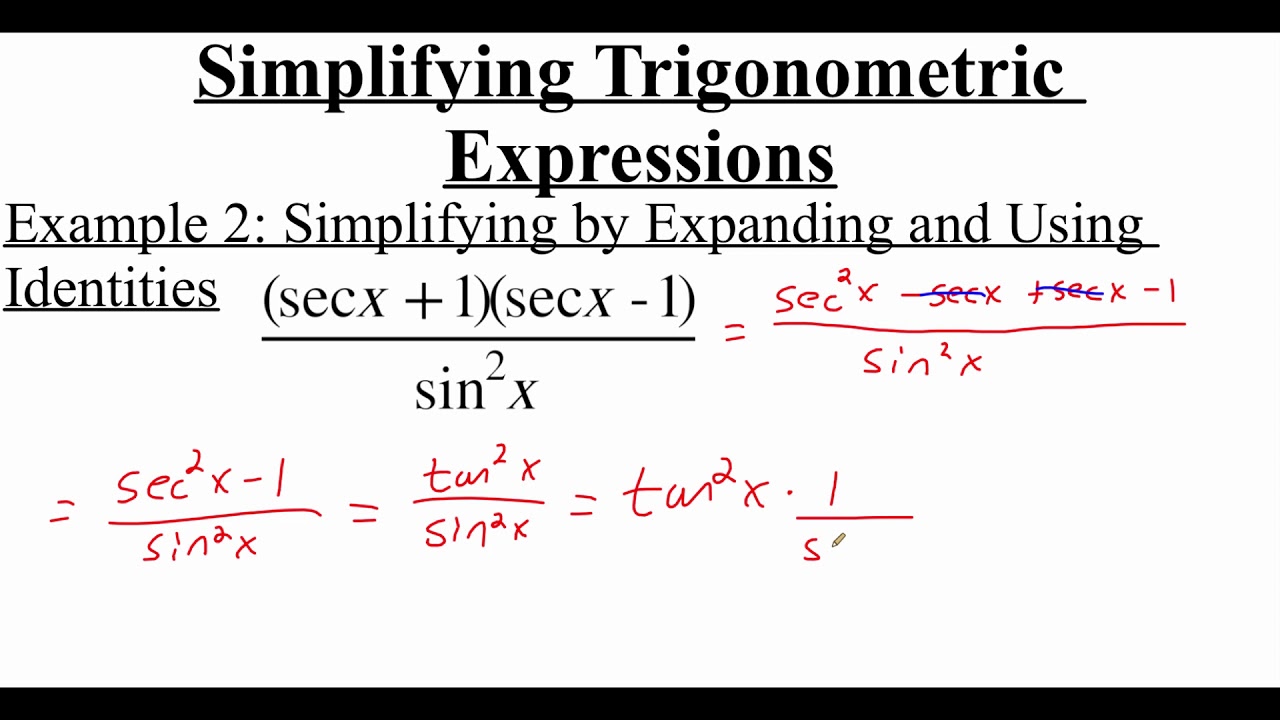
  • How does the script simplify the expression sec² * (1 - sin²)?

    -The script simplifies it by replacing 1 - sin² with cos², then recognizing that sec² * cos² simplifies to 1.

  • How does the script simplify the expression cosθ + sinθ * tanθ?

    -The script simplifies it by converting tan to sin/cos, then combining the terms into a single fraction and using the Pythagorean identity sin² + cos² = 1, resulting in secθ.

  • What is the final simplified form of the expression sec * sin * (cot + tan)?

    -The final simplified form is 1, as the script shows that the complex fraction simplifies to a single term of 1 over 1.

Outlines
00:00
📚 Simplifying Trigonometric Expressions

This paragraph introduces the topic of simplifying trigonometric expressions. Key formulas are highlighted, including reciprocal identities (secant = 1/cosine theta, cosecant = 1/sine theta) and the relationships between tangent, cotangent, sine, and cosine. The trigonometric Pythagorean identities are also introduced, emphasizing their importance in simplifying expressions. The paragraph demonstrates how to simplify an expression involving secant by canceling out the cosine theta term, resulting in sine theta.

05:06
🔍 Applying Trigonometric Identities

This section delves deeper into the application of trigonometric identities to simplify expressions. It starts by simplifying an expression involving tangent squared, sine squared, and cosine squared, using the Pythagorean identity. The expression is simplified to secant squared theta by recognizing and applying the identity one plus tangent squared equals secant squared. The paragraph also covers simplifying an expression involving sine squared times cosecant theta times secant theta, using reciprocal identities to cancel terms and ultimately simplifying to tangent theta.

10:10
🧩 Further Simplification Techniques

The final paragraph continues the theme of simplifying trigonometric expressions, focusing on more complex scenarios. It starts by simplifying an expression involving cotangent times tangent plus cotangent squared theta, using the identity one plus cotangent squared equals cosecant squared. The paragraph also explores simplifying secant squared times one minus sine squared, using the Pythagorean identity to replace terms and simplify to one. The section concludes with an example of simplifying cosine theta plus sine theta times tangent theta, demonstrating the process of converting tangent to sine over cosine and combining fractions to simplify the expression to secant theta.

Mindmap
Keywords
💡Trigonometric Expressions
Trigonometric expressions are mathematical formulas involving trigonometric functions, which relate the angles of a triangle to the lengths of its sides. In the video, the main theme revolves around simplifying these expressions using various identities. For example, the script discusses simplifying expressions like 'secant squared times one minus sine squared' to 'one' by using trigonometric identities.
💡Reciprocal Identities
Reciprocal identities in trigonometry are relationships where one function is the reciprocal of another. The script mentions that secant is the reciprocal of cosine, and cosecant is the reciprocal of sine, which are used to simplify expressions by converting them into more manageable forms.
💡Tangent
Tangent is a trigonometric function defined as the ratio of the opposite side to the adjacent side in a right-angled triangle. In the script, tangent is used in expressions and simplified using the identity 'tangent is equal to sine theta divided by cosine theta'.
💡Cotangent
Cotangent, the reciprocal of tangent, is another trigonometric function that represents the ratio of the adjacent side to the opposite side in a right-angled triangle. The script uses the cotangent identity to simplify expressions, such as converting 'cotangent times tan' to 'one'.
💡Pythagorean Identities
Pythagorean identities in trigonometry are fundamental relationships between the squares of sine and cosine functions. The script emphasizes the identity 'sine squared plus cosine squared equals one' as a key tool for simplifying trigonometric expressions, as seen when reducing 'tangent squared theta plus sine squared theta plus cosine squared theta' to 'secant squared theta'.
💡Simplification
Simplification in the context of the video refers to the process of reducing complex trigonometric expressions to simpler forms using identities. The script demonstrates several examples of simplification, such as transforming 'secant squared times one minus sine squared' into 'one' by applying trigonometric identities.
💡Cosine Theta
Cosine theta represents the cosine of an angle theta in trigonometry. The script uses cosine theta in various expressions and demonstrates its simplification, such as canceling 'cosine theta' in the expression 'secant with one over cosine theta'.
💡Sine Theta
Sine theta is the sine of an angle theta, a fundamental trigonometric function. The script shows how sine theta can be left as is in some expressions, or used in identities to simplify others, such as in 'sine squared times cosecant theta times secant theta'.
💡Secant
Secant is the reciprocal of the cosine function, used in the script to simplify expressions by converting them into a form involving sine and cosine directly, as demonstrated when replacing 'secant' with 'one over cosine theta'.
💡Cosecant
Cosecant, the reciprocal of the sine function, is used in the script to simplify expressions involving the ratio of the hypotenuse to the opposite side in a right-angled triangle. The script shows its use in simplifying 'sine squared times cosecant theta times secant theta' to 'tangent theta'.
💡Common Denominators
Finding common denominators is a technique used in the script to combine fractions within a trigonometric expression, as seen when converting 'cosine theta plus sine theta times tangent theta' into a single fraction with a common denominator of 'cosine'.
Highlights

Introduction to simplifying trigonometric expressions with key formulas to remember.

Reciprocal identities: secant as 1/cosine theta and cosecant as 1/sine theta.

Tangent and cotangent definitions in terms of sine and cosine.

Importance of writing down and using these formulas throughout the video.

Trigonometric Pythagorean identities: sine squared plus cosine squared equals one.

Identity involving tangent squared and secant squared.

Identity involving cotangent squared and cosecant squared.

Simplification of an expression using secant and cosine cancellation.

Simplification of tangent squared plus sine squared plus cosine squared using Pythagorean identities.

Conversion of secant squared to 1/cosine squared and simplification of an expression.

Simplification of sine squared times cosecant theta times secant theta to tangent theta.

Simplification of cotangent times tan plus cotangent to cosecant squared theta.

Simplification of secant squared times one minus sine squared to one.

Simplification of cosine theta plus sine theta times tangent theta to secant theta.

Use of reciprocal identities to simplify a complex trigonometric expression.

Distributing sine theta and simplifying fractions within fractions.

Final simplification of a complex expression to one using Pythagorean identities.

Conclusion summarizing the use of reciprocal and Pythagorean identities in simplifying trigonometric expressions.

Transcripts

Browse More Related Video

Precalc 5.1 Using Fundamental Trig Identities

How do you simplify trigonometric expressions

Proving Trigonometric Identities | Fundamental Trigonometric Identities | Formulas | Sample Problems

Proving Trigonometric Identities | Fundamental Trigonometric Identities | Formulas | Part 2

Tips to verifying trigonometric identities

5.1.5 Simplifying Trigonometric Expressions

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
TrigonometrySimplificationReciprocal IdentitiesPythagorean IdentitiesTrig ExpressionsMath TutorialEducational VideoSine CosineTangent CotangentSecant Cosecant