5.1.2 Pythagorean Identities
TLDRIn this video, Mr. Banker explores Pythagorean identities, focusing on three basic forms and their rearranged versions. He demonstrates how to use these identities to solve problems, such as finding sine and cosine of theta when given tangent and cosine values. The video concludes with a step-by-step solution using reciprocal and quotient identities.
Takeaways
- 📚 The video discusses Pythagorean identities, focusing on three basic forms and their rearranged versions.
- 🔍 The first identity presented is \( \sin^2(\theta) + \cos^2(\theta) = 1 \), which can be rearranged to find expressions for \( \sin^2(\theta) \) and \( \cos^2(\theta) \).
- 🔄 The rearranged forms of the first identity are \( \sin^2(\theta) = 1 - \cos^2(\theta) \) and \( \cos^2(\theta) = 1 - \sin^2(\theta) \).
- 📐 The second identity is \( \sec^2(\theta) = 1 + \tan^2(\theta) \), which can be rearranged to find \( \tan^2(\theta) \) and \( \sec^2(\theta) \).
- 📈 The rearranged forms of the second identity are \( \tan^2(\theta) = \sec^2(\theta) - 1 \) and \( 1 = \sec^2(\theta) - \tan^2(\theta) \).
- 📉 The third identity is \( \csc^2(\theta) = 1 + \cot^2(\theta) \), with rearranged forms \( \cot^2(\theta) = \csc^2(\theta) - 1 \) and \( 1 = \csc^2(\theta) - \cot^2(\theta) \).
- 🧩 The video uses these identities to solve problems, starting with finding \( \sin(\theta) \) and \( \cos(\theta) \) given \( \tan(\theta) = 5 \) and \( \cos(\theta) > 0 \).
- 🔢 The secant squared is calculated using the identity \( \sec^2(\theta) = 1 + \tan^2(\theta) \), resulting in \( \sec^2(\theta) = 26 \).
- 📌 The secant value is determined to be the positive square root of 26, as \( \cos(\theta) \) must be positive.
- 📉 The cosine of theta is found using the reciprocal identity \( \cos(\theta) = \frac{1}{\sec(\theta)} \), leading to \( \cos(\theta) = \frac{\sqrt{26}}{26} \).
- 📈 The sine of theta is derived using the identity \( \tan(\theta) = \frac{\sin(\theta)}{\cos(\theta)} \), resulting in \( \sin(\theta) = \tan(\theta) \times \cos(\theta) \) or \( \frac{5\sqrt{26}}{26} \).
Q & A
What are the three basic Pythagorean identities mentioned in the video?
-The three basic Pythagorean identities are: 1) sine squared of theta plus cosine squared of theta equals one, 2) secant squared of theta equals one plus tangent squared of theta, and 3) cosecant squared of theta equals one plus cotangent squared of theta.
How can the first Pythagorean identity be rearranged to find sine squared of theta?
-The first Pythagorean identity can be rearranged by subtracting cosine squared of theta from both sides, resulting in sine squared of theta equals one minus cosine squared of theta.
What rearrangement of the first Pythagorean identity gives cosine squared of theta equals one minus sine squared of theta?
-By subtracting sine squared of theta from both sides of the first Pythagorean identity, you get cosine squared of theta equals one minus sine squared of theta.
How is the secant squared of theta related to the tangent squared of theta in the second Pythagorean identity?
-In the second Pythagorean identity, secant squared of theta is equal to one plus tangent squared of theta.
What rearrangement of the second Pythagorean identity can be used to find tangent squared of theta?
-By subtracting one from both sides of the second Pythagorean identity, you can find tangent squared of theta equals secant squared of theta minus one.
What is the rearrangement of the third Pythagorean identity that relates cotangent squared of theta to cosecant squared of theta?
-By subtracting one from both sides of the third Pythagorean identity, you get cotangent squared of theta equals cosecant squared of theta minus one.
Given that the tangent of theta is five and the cosine of theta is greater than zero, how can you find the secant of theta?
-You can use the identity secant squared of theta equals one plus tangent squared of theta. Plugging in the tangent value of five and squaring it, you get secant squared of theta equals 26. Taking the square root gives secant of theta as plus or minus the square root of 26, but since cosine is positive, you only consider the positive square root.
How is the cosine of theta related to the secant of theta?
-The cosine of theta is equal to one over the secant of theta, which means you can find the cosine by taking the reciprocal of the secant.
What is the relationship between the sine of theta and the tangent of theta?
-The tangent of theta is equal to the sine of theta divided by the cosine of theta. This relationship can be rearranged to find the sine of theta by multiplying both sides by the cosine of theta.
How can you find the sine of theta given the tangent of theta and the cosine of theta?
-You can find the sine of theta by multiplying the tangent of theta (which is five) by the cosine of theta (which is one over the square root of 26), resulting in sine of theta equals 5 over the square root of 26.
What is the final expression for the sine of theta in terms of the tangent and cosine of theta?
-The final expression for the sine of theta, after rationalizing the numerator, is 5 root 26 over 26.
Outlines
📚 Introduction to Pythagorean Identities
This paragraph introduces the concept of Pythagorean identities in trigonometry. It explains that there are three basic identities and that these can be rearranged to derive additional identities. The first identity presented is the fundamental sine squared plus cosine squared equals one. The paragraph then discusses how this identity can be rearranged to express sine squared or cosine squared in terms of one minus the other. This sets the stage for exploring further identities involving secant, tangent, cosecant, and cotangent.
🔍 Applying Pythagorean Identities to Solve for Sine and Cosine
In this paragraph, the script demonstrates the application of Pythagorean identities to solve for sine and cosine of an angle when given the tangent and the condition that cosine is positive. The process begins by using the secant squared identity to find the secant of theta, which is the reciprocal of cosine. After finding the secant squared to be 26, the secant of theta is determined to be the square root of 26. The cosine of theta is then found by taking the reciprocal of the positive square root of 26. The paragraph continues by using the tangent identity to solve for sine of theta, which is the product of tangent and cosine of theta. The final result for sine is 5 times the square root of 26 divided by 26, and the video concludes with this solution.
Mindmap
Keywords
💡Pythagorean Identities
💡sine squared of theta
💡cosine squared of theta
💡secant squared of theta
💡tangent squared of theta
💡cosecant squared of theta
💡cotangent squared of theta
💡reciprocal identities
💡tangent of theta
💡quotient identity
💡rationalizing
Highlights
Introduction to Pythagorean identities with three basic forms and their rearranged versions.
First Pythagorean identity: sine squared of theta plus cosine squared of theta equals one.
Rearranging the first identity to find sine squared of theta equals one minus cosine squared of theta.
Rearranging the first identity to find cosine squared of theta equals one minus sine squared of theta.
Second Pythagorean identity: secant squared of theta is equal to one plus tangent squared of theta.
Rearranging the second identity to find tangent squared of theta equals secant squared of theta minus one.
Rearranging the second identity to find one equals secant squared of theta minus tangent squared of theta.
Third Pythagorean identity: cosecant squared of theta is equal to one plus cotangent squared of theta.
Rearranging the third identity to find cotangent squared of theta equals cosecant squared of theta minus one.
Rearranging the third identity to find one equals cosecant squared of theta minus cotangent squared of theta.
Using identities to solve problems, starting with finding sine and cosine of theta given tangent of theta is five.
Using the identity involving secant squared of theta to find secant squared of theta equals 26.
Calculating secant of theta as the square root of 26.
Determining cosine of theta using the reciprocal identity and focusing on the positive root.
Finding cosine of theta as one over the square root of 26.
Using the quotient identity involving tangent to find sine of theta.
Calculating sine of theta as five over the square root of 26.
Rationalizing the expression for sine of theta to get 5 root 26 over 26.
Transcripts
Browse More Related Video
5.1.4 Odd-Even Identities

Trig - 0.4 Fundamental Trig Identities
Proving Trigonometric Identities | Fundamental Trigonometric Identities | Formulas | Part 2
5.4.1 Double-Angle Identities
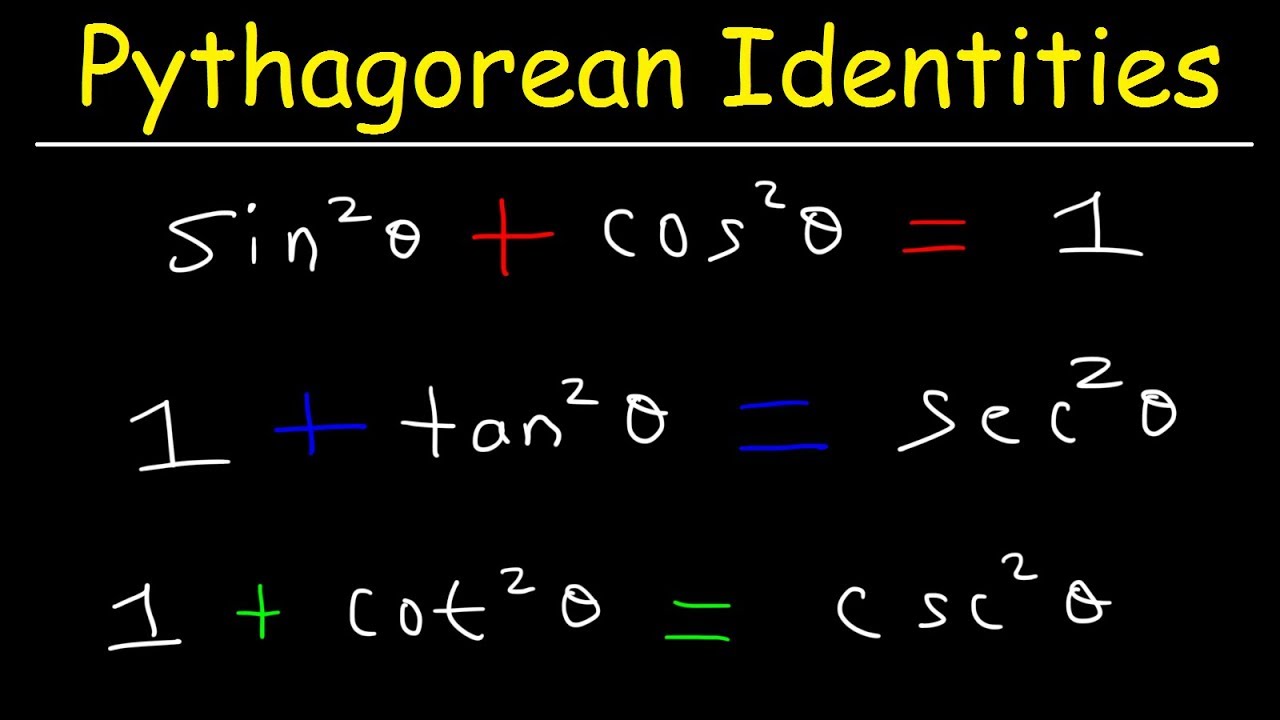
Pythagorean Identities - Examples & Practice Problems, Trigonometry
Quotient Identities - Evaluating Tangent and Cotangent Functions
5.0 / 5 (0 votes)
Thanks for rating: