The Most Beautiful Equation in Math

Carnegie Mellon University
14 Mar 201603:49
EducationalLearning
32 Likes 10 Comments

TLDRThe video script introduces the audience to Euler's identity, touted as the most beautiful equation in mathematics. It explains the significance of three fundamental mathematical constants: Pi (π), the imaginary unit (i), and the base of natural logarithms (e). The script humorously touches on the concept of complex numbers and the intriguing ways these constants interrelate, culminating in the equation e^(iπ) + 1 = 0. The discussion also includes a light-hearted joke about Pi and i, highlighting the playful side of mathematics.

Takeaways
  • 📌 The most beautiful equation in math is often associated with a combination of key mathematical constants.
  • 🤔 Pi (π) is a well-known constant approximately equal to 3.14159, representing the ratio of a circle's circumference to its diameter.
  • 🌀 The imaginary unit 'i' is the square root of -1, introducing the concept of complex numbers.
  • 📈 'e' is another important mathematical constant, approximately equal to 2.71828, and is the base of the natural logarithm.
  • 🎂 'e' can be related to probabilities, such as the probability of every chocolate returning to the wrong spot in a box of chocolates.
  • 🔢 Euler's identity combines the numbers e, i, and π with 1 and 0 to form the equation e^(iπ) + 1 = 0, showcasing the harmony of mathematics.
  • 👕 The script mentions a humorous T-shirt joke involving π and i, highlighting the cultural impact of these mathematical concepts.
  • 💡 The T-shirt joke's punchline 'e should say join me and we'll be one' reflects the unifying aspect of Euler's identity, resulting in -1.
  • 🌟 The beauty of Euler's identity lies in the unification of different branches of mathematics through fundamental constants.
  • 🎓 The discussion of the equation and the joke demonstrates the accessibility and appeal of complex mathematical concepts to a general audience.
  • 🚀 The script serves as an engaging introduction to complex numbers and transcendental numbers, sparking interest in further mathematical exploration.
Q & A

  • What is the significance of the equation shared in the transcript?

    -The equation shared in the transcript is known as Euler's identity, which is considered the most beautiful equation in mathematics because it elegantly combines five fundamental constants: e, i, π, 1, and 0, into a simple and profound relationship.

  • What does the 'I' in the equation represent?

    -In the equation, 'I' represents the imaginary unit, which is the square root of -1. It is a concept in complex numbers that allows for the inclusion of numbers that cannot be represented on the real number line.

  • What is the mathematical constant 'e'?

    -The constant 'e' is a mathematical transcendental number, approximately equal to 2.71828. It is the base of the natural logarithm and appears in various mathematical contexts, including growth and decay processes and calculus.

  • How is the number 'e' related to the probability example given in the transcript?

    -The number 'e' is related to the probability example in that it represents the probability that every chocolate in a box of 100 chocolates would end up in the wrong spot if dropped and randomly placed back into the box. This probability approaches 1 over 'e' as the number of chocolates increases.

  • What is Euler's identity?

    -Euler's identity is an equation that expresses the relationship e^(iπ) + 1 = 0, where 'e' is the base of the natural logarithm, 'i' is the imaginary unit, and 'π' is Pi. This identity is celebrated for its simplicity and the profound union of different mathematical concepts it represents.

  • What does the equation e^(iπ) + 1 = 0 demonstrate?

    -The equation e^(iπ) + 1 = 0 demonstrates a remarkable convergence of the most fundamental numbers in mathematics: the transcendental numbers e and π, the imaginary unit i, and the integers 1 and 0. It shows that when 'e' is raised to the power of iπ, the result, when added to 1, equals zero, which is a profound and aesthetically pleasing result.

  • How does the concept of complex numbers allow for the existence of 'i'?

    -Complex numbers extend the real number system by introducing a second dimension, allowing for numbers that have a real part and an imaginary part. This extension permits the existence of 'i', the square root of -1, which would not be possible within the confines of real numbers alone.

  • What is the significance of Pi (π) in the equation?

    -Pi (π) is a mathematical constant that represents the ratio of a circle's circumference to its diameter. In Euler's identity, π is one of the fundamental constants that, when combined with 'e', 'i', 1, and 0, creates a simple yet profound equation that showcases the interconnectedness of these mathematical concepts.

  • What is the joke mentioned in the transcript about Pi and 'i'?

    -The joke mentioned in the transcript is a play on words between Pi (π) and 'i', where Pi says, 'Get real,' and 'i' says, 'Be rational.' It's a humorous exchange that highlights the characteristics of these numbers, with Pi being an irrational number and 'i' representing an imaginary number. The punchline suggested is that 'e' should join them, and they would become one, which humorously results in the number -1.

  • How does the concept of Euler's identity showcase the beauty of mathematics?

    -Euler's identity showcases the beauty of mathematics by demonstrating how seemingly disparate mathematical concepts can come together in a simple and elegant equation. It unites fundamental constants and complex numbers in a way that is both aesthetically pleasing and intellectually stimulating, highlighting the harmony and interconnectedness within mathematical ideas.

  • What is the approximate value of the mathematical constant 'e'?

    -The approximate value of the mathematical constant 'e' is 2.71828. It is an irrational number with a non-repeating, non-terminating decimal expansion.

Outlines
00:00
📚 Introduction to Beautiful Mathematical Equations

The speaker introduces the concept of the most beautiful equation in mathematics, which can be found when searching on Google. They mention the use of a board to illustrate the equation and introduce three fundamental mathematical constants: Pi (π), the imaginary unit (i), which is the square root of -1, and 'e', which is a transcendental number approximately equal to 2.71828. The speaker explains that 'e' is related to probabilities, such as the unlikely chance of placing 100 chocolates back in the wrong spots after dropping them.

Mindmap
Keywords
💡Pi (π)
Pi, denoted by the symbol π, is a mathematical constant that represents the ratio of a circle's circumference to its diameter. It is an irrational number, meaning it cannot be expressed as a simple fraction and its decimal representation goes on infinitely without repeating. In the video, Pi is introduced as one of the 'crazy numbers' that, when combined with other mathematical constants, forms the basis of Euler's identity, which is celebrated as one of the most beautiful equations in mathematics.
💡Imaginary numbers
Imaginary numbers are a class of complex numbers that, when squared, result in a negative number. The imaginary unit 'i' is defined as the square root of -1. These numbers extend the real number system and are essential in various fields of mathematics, including geometry, engineering, and physics. In the video, the concept of imaginary numbers is introduced to explain the 'I' in Euler's identity, emphasizing the mathematical flexibility that allows for the creation of complex numbers.
💡E (e)
The number 'e' is a mathematical constant approximately equal to 2.71828 and is the base of the natural logarithm. It is an irrational and transcendental number, similar to Pi, and appears in various mathematical contexts, including exponential growth and decay processes, and compound interest calculations. In the video, 'e' is presented as one of the fundamental numbers that, when combined with Pi and 'i', forms the basis of Euler's identity.
💡Euler's identity
Euler's identity is an equation that succinctly combines five fundamental mathematical constants: 'e', 'i', Pi (π), 1, and 0. The equation is e^(iπ) + 1 = 0, which showcases the profound interconnection between these constants. It is often hailed as one of the most beautiful equations in mathematics due to its elegance and simplicity. In the video, the speaker highlights Euler's identity as the culmination of the discussion on the beautiful interplay of numbers.
💡Complex numbers
Complex numbers are numbers that consist of two parts: a real part and an imaginary part, where the imaginary part is a multiple of the imaginary unit 'i'. They extend the concept of real numbers by allowing for the square root of negative numbers and are crucial in various mathematical and physical applications. In the video, the speaker introduces complex numbers as a way to understand the number 'i' and its role in forming Euler's identity.
💡Transcendental numbers
Transcendental numbers are numbers that are not the root of any non-zero polynomial equation with rational coefficients. They are not algebraic and their decimal representation is non-repeating and non-terminating. Pi (π) and 'e' are both examples of transcendental numbers. In the video, the speaker mentions transcendental numbers to emphasize the unique and infinite nature of numbers like Pi and 'e', which are integral to Euler's identity.
💡Probability
Probability is a measure of the likelihood that a particular event will occur, expressed as a number between 0 and 1. It is a fundamental concept in mathematics and statistics, used to predict and analyze uncertain events. In the video, the speaker uses a humorous example of chocolates being dropped and the probability of each one returning to the wrong spot to illustrate how this probability approaches 1 over 'e', thereby connecting 'e' to a real-world scenario.
💡Jokes
Jokes are a form of entertainment that typically involve a punchline and are intended to provoke laughter. In the context of the video, the speaker references a joke involving Pi and 'i', where Pi says 'Get real' and 'i' says 'Be rational', which is a humorous play on the mathematical concepts of real numbers and rational numbers. The speaker then extends the joke by suggesting that 'e' should join in and say 'Join me and we'll be one', leading to the punchline of negative one.
💡T-shirts
T-shirts are a common form of casual attire, often used as a canvas for displaying messages, designs, or jokes. In the video, the speaker mentions t-shirts with mathematical jokes related to Pi and 'i', indicating the cultural impact and popularity of these mathematical concepts. The speaker also suggests an additional punchline for the joke on the t-shirt, showcasing the integration of mathematics into everyday humor and merchandise.
💡Interconnections
Interconnections refer to the relationships or links between different elements or concepts. In the context of the video, the speaker emphasizes the interconnections between various mathematical constants and their roles in forming Euler's identity, highlighting the beauty of how these seemingly disparate elements come together in a single, elegant equation.
Highlights

The most beautiful equation in math is introduced.

Pi (π) is a well-known mathematical constant.

The concept of 'i' as the square root of -1 is explained, introducing complex numbers.

The number 'e' is described as another fundamental mathematical constant, similar to Pi.

The approximate value of 'e' is given as 2.71828, with an extended precision value mentioned.

The number 'e' has applications in probability, such as the chocolate box example.

Three seemingly unrelated numbers (Pi, i, and e) are combined to form Euler's identity.

Euler's identity is presented as the most beautiful equation in math due to its combination of fundamental constants.

A humorous joke involving Pi and 'i' is discussed, highlighting their cultural impact.

The joke is expanded with a new punchline involving 'e', showcasing creativity in mathematical humor.

The significance of Euler's identity lies in its simplicity and the profound impact of combining fundamental mathematical concepts.

The discussion emphasizes the interconnectedness of mathematical concepts.

The presentation style is engaging, using analogies and humor to explain complex mathematical ideas.

The transcript provides a unique perspective on the beauty and practicality of mathematics.

The narrative is a blend of mathematical education and entertainment.

The transcript showcases the relevance of mathematics in everyday scenarios, like the chocolate box analogy.

The discussion touches on the historical aspect of mathematical constants and their discovery.

The transcript emphasizes the universality of mathematical concepts, as they apply across different fields and situations.

Transcripts

Browse More Related Video

Proving the Most Beautiful Equation Bob Ross Style

Polynomial approximation of functions (part 7)

Euler's formula & Euler's identity | Series | AP Calculus BC | Khan Academy

Cambridge Mathematician Reacts to 'Animation vs Math'

14 - What is Euler's Number 'e', Ln(x) - Natural Log & e^x Functions?

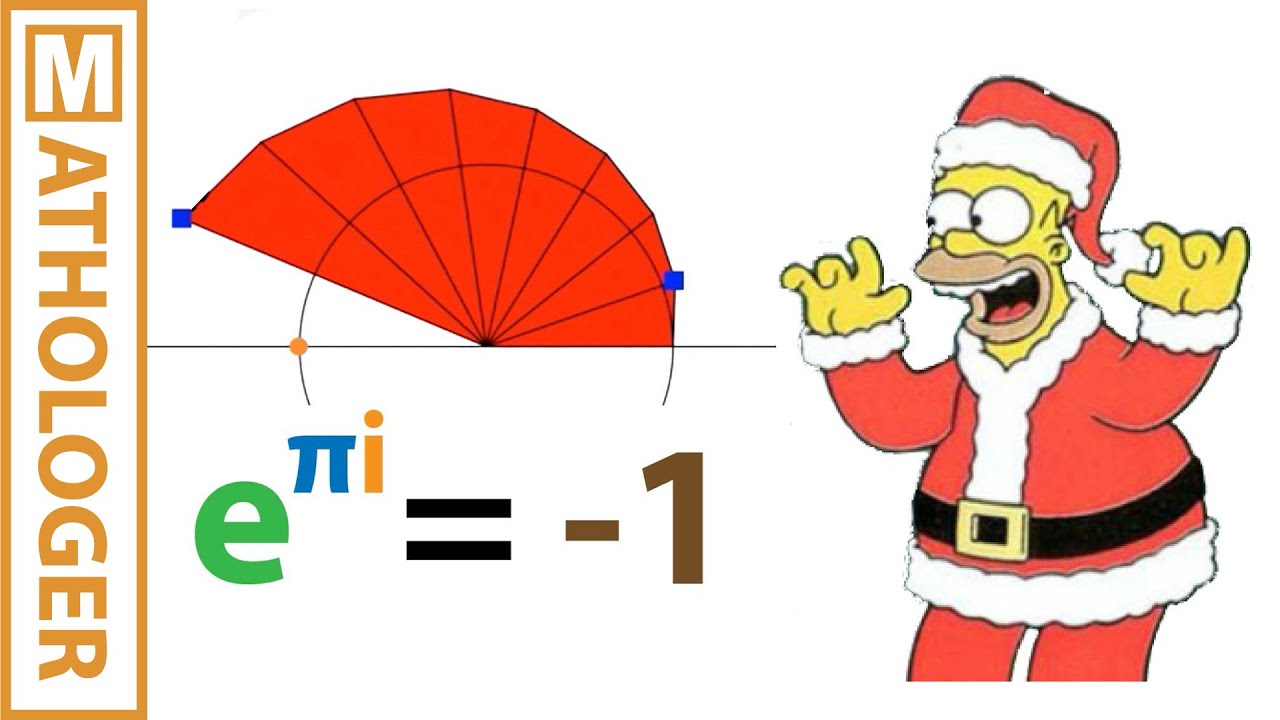
e to the pi i for dummies

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsEuler's IdentityComplex NumbersTranscendental NumbersImaginary NumbersPi and eMathematical BeautyEducational ContentHumor in MathT-shirt Jokes