Precalc 5.2 Verifying Trig Identities

SupernovaMath
11 Dec 201613:19
EducationalLearning
32 Likes 10 Comments

TLDRThis video tutorial covers the process of verifying trigonometric identities, emphasizing the importance of working with one side of the equation and using algebraic skills to simplify expressions. It guides viewers through various steps, including factoring, using fundamental identities, and converting all terms to sines and cosines. The presenter demonstrates how to solve several problems, turning complex trigonometric expressions into simpler forms, ultimately verifying the identities.

Takeaways
  • 📚 The lesson is about verifying trigonometric identities, which is different from simplifying expressions where the value is not known.
  • 📝 There are specific guidelines to follow when verifying trig identities, starting with working on one side of the equation only, typically the left side.
  • 🔍 Look for opportunities to simplify expressions using algebraic skills such as factoring, adding fractions, and using fundamental identities.
  • 🧩 Fundamental trigonometric identities can be used to pair functions effectively, like sine and cosine, secant and tangent, and cosecant and cotangent.
  • 🔄 If stuck, consider converting all terms to sines and cosines, which are the basic trigonometric functions.
  • 🤔 Encouragement to always try something, as even unsuccessful attempts can provide insights and lead to new approaches.
  • 🎯 The goal is to simplify the left side of the equation to match the right side, which is often a simplified or standard trigonometric expression.
  • 📉 The process involves recognizing and using Pythagorean identities to convert expressions into a form that matches the desired outcome.
  • 🔄 Demonstrated examples show the step-by-step simplification process, including converting to and from sine and cosine, and using reciprocal identities.
  • 📐 The transcript includes a detailed walkthrough of several problems, showing how to apply the guidelines and algebraic manipulation to verify identities.
  • 🧩 The importance of having a list of trigonometric identities handy while working through the problems is emphasized for quick reference.
  • 😄 The instructor expresses enthusiasm for the topic, likening the process of verifying trig identities to solving puzzles, which can be enjoyable.
Q & A

  • What is the main difference between section 5.1 and section 5.2 in the context of trigonometry?

    -Section 5.1 focuses on simplifying expressions using fundamental trigonometric identities, while section 5.2 is about verifying trigonometric identities by showing that one side of an equation equals the other.

  • What is the first step in verifying trigonometric identities according to the video?

    -The first step is to work with only one side of the equation, typically the left side, and manipulate it to match the right side without altering the right side.

  • What algebraic skills are recommended to use when simplifying trigonometric expressions in the video?

    -Skills such as factoring, adding fractions, squaring binomials, and creating monomial denominators are recommended to simplify expressions as much as possible.

  • How can the fundamental trigonometric identities be used in verifying identities?

    -Fundamental identities can be used by noting which functions are in the final expression and finding identities that pair well, such as sine and cosine, secant and tangent, or cosecant and cotangent.

  • What should you do if the initial guidelines for verifying identities do not help?

    -If the initial guidelines do not help, try converting all terms to sines and cosines, as these are the basic trigonometric functions.

  • Why is it important to always try something when verifying trigonometric identities?

    -Even if an attempt leads to a dead end, it can provide insights and sometimes it may be necessary to start over to find the correct path to simplify the expression.

  • What is the significance of having fun with trigonometric puzzles as mentioned in the video?

    -Having fun with trigonometric puzzles helps maintain engagement and encourages a problem-solving mindset, which is crucial for understanding and applying trigonometric identities.

  • How does the video demonstrate the simplification of the expression involving tangent, cosecant, and secant of theta?

    -The video shows that by breaking down tangent, cosecant, and secant in terms of sine and cosine, the expression simplifies to 1, verifying the identity.

  • In the example involving sine squared minus cotangent times tangent over cotangent times sine, how is the expression simplified?

    -By recognizing that cotangent and tangent are reciprocals and using the Pythagorean identity, the expression is simplified to negative cosine of theta.

  • What identity is used in the video to simplify the expression involving cosecant squared minus cotangent squared over tangent times cosine?

    -The identity one plus cotangent squared equals cosecant squared is used, and by manipulating it, the expression is simplified to cosecant of X.

  • How does the video approach the problem of simplifying the expression sine of X plus cosine of X cotangent of X?

    -By converting cotangent to sine over cosine and simplifying the fraction, the expression is simplified to cosecant of X using the Pythagorean identity.

  • What mistake is corrected in the video when simplifying the expression 1 over sine of X minus 1 over cosecant of X?

    -The mistake is in the initial simplification, where the expression is incorrectly simplified. The correct approach is to recognize that 1 over sine of X minus sine of X simplifies to cosecant of X minus sine of X.

Outlines
00:00
📚 Introduction to Verifying Trig Identities

The video introduces the concept of verifying trigonometric identities, distinguishing it from simplifying expressions in the previous section. It outlines a six-step process for verification, emphasizing working with one side of the equation, using algebraic skills to simplify, applying fundamental trig identities, converting all terms to sines and cosines if needed, and the importance of trying different approaches. The presenter uses the example of simplifying an equation involving tangent, cosecant, and secant to illustrate the process, successfully reducing it to the identity of 1.

05:01
🔍 Detailed Walkthrough of Trig Identity Simplification

This paragraph delves deeper into the process of simplifying trigonometric identities, using specific examples to demonstrate the application of the guidelines. The presenter simplifies expressions involving sine squared, cotangent, and tangent, and then moves on to examples with cosecant squared, cotangent squared, and tangent. Each step is carefully explained, showing how to manipulate the expressions using trig identities and algebraic manipulations, ultimately achieving the goal of simplifying the left side of the equation to match the right side, which is often an identity of 1 or a simplified trigonometric function.

10:02
🎓 Conclusion and Encouragement for Trig Identity Puzzles

The final paragraph wraps up the lesson on verifying trigonometric identities, highlighting the presenter's enthusiasm for the subject. It emphasizes the trial-and-error nature of working with trigonometric puzzles and encourages viewers to try different approaches, even if they lead to dead ends, as they can provide valuable insights. The presenter concludes by reiterating the goal of simplifying the left side of the equation to match the right side, using the process outlined in the video.

Mindmap
Keywords
💡Trig Identities
Trig identities are fundamental equations involving trigonometric functions that are true for all values of the variable where the functions are defined. In the video, the focus is on verifying these identities, meaning proving that one side of an equation equals the other by manipulating expressions using known identities.
💡Fundamental Identities
Fundamental identities are the basic trigonometric identities such as sin²(x) + cos²(x) = 1. These identities are used as tools to simplify and manipulate trigonometric expressions. In section 5.2, students use these identities to verify more complex trigonometric equations.
💡Manipulate
To manipulate in this context means to algebraically rearrange and simplify expressions. The video emphasizes manipulating the left side of an equation using algebraic techniques and trigonometric identities to prove it equals the right side.
💡Algebraic Skills
Algebraic skills refer to the ability to perform algebraic operations such as factoring, adding fractions, and simplifying expressions. These skills are crucial for verifying trig identities as they help transform and simplify the expressions involved.
💡Sines and Cosines
Sines and cosines are the basic trigonometric functions. The video suggests converting all terms to sines and cosines if stuck, as these functions are foundational and often simplify the verification process.
💡Pythagorean Identities
Pythagorean identities are trigonometric identities derived from the Pythagorean theorem, such as sin²(x) + cos²(x) = 1. These identities are frequently used in the video to simplify expressions and verify trigonometric equations.
💡Reciprocals
Reciprocals in trigonometry refer to functions that are multiplicative inverses of each other, like sine and cosecant or tangent and cotangent. The video demonstrates using these relationships to simplify and verify trigonometric expressions.
💡Simplify
To simplify means to reduce an expression to its simplest form. In the context of the video, simplifying involves using algebraic operations and trigonometric identities to make one side of an equation look like the other.
💡Cosecant
Cosecant is the reciprocal of the sine function (csc(x) = 1/sin(x)). The video shows how to convert other trigonometric functions into cosecant to help simplify and verify identities.
💡Trial and Error
Trial and error is a problem-solving method involving testing various approaches until finding one that works. The video encourages using trial and error in verifying trig identities, as even failed attempts can provide insights into solving the problem.
Highlights

Introduction to verifying trigonometric identities as opposed to simplifying expressions.

Guidelines for verifying trig identities with steps to follow for the process.

Emphasis on working with only one side of the equation, specifically the left side.

Use of algebraic skills to simplify expressions, including factoring and combining like terms.

Utilization of fundamental trigonometric identities to simplify expressions.

Conversion of all terms to sines and cosines as a strategy when stuck.

The importance of trying different approaches even if they lead to dead ends for insights.

Encouragement to have fun with the trigonometric puzzles.

Example problem walkthrough: Simplifying the left side of an equation to equal 1.

Demonstration of breaking down tangent, cotangent, and secant in terms of sine and cosine.

Simplification of an expression involving sine squared, cotangent, and tangent.

Use of the Pythagorean identity to convert sine squared into a form involving cosine.

Another example problem: Simplifying an expression to achieve a negative cosine of theta.

Manipulation of identities to express cosecant squared in terms of cotangent squared.

Conversion of cotangent to sine over cosine for further simplification.

Final simplification of an expression to match the goal of cosecant of X.

Example of simplifying an expression involving sine, cosine, and cotangent to cosecant.

Common mistake made during simplification and the process of backtracking to correct it.

Final simplification of an expression to match the goal of 1 over sine of X, which is cosecant.

Conclusion of the lesson emphasizing the trial and error nature of working with trigonometric identities.

Transcripts

Browse More Related Video

5.2.1 Proving Trigonometric Identities

Tips to verifying trigonometric identities

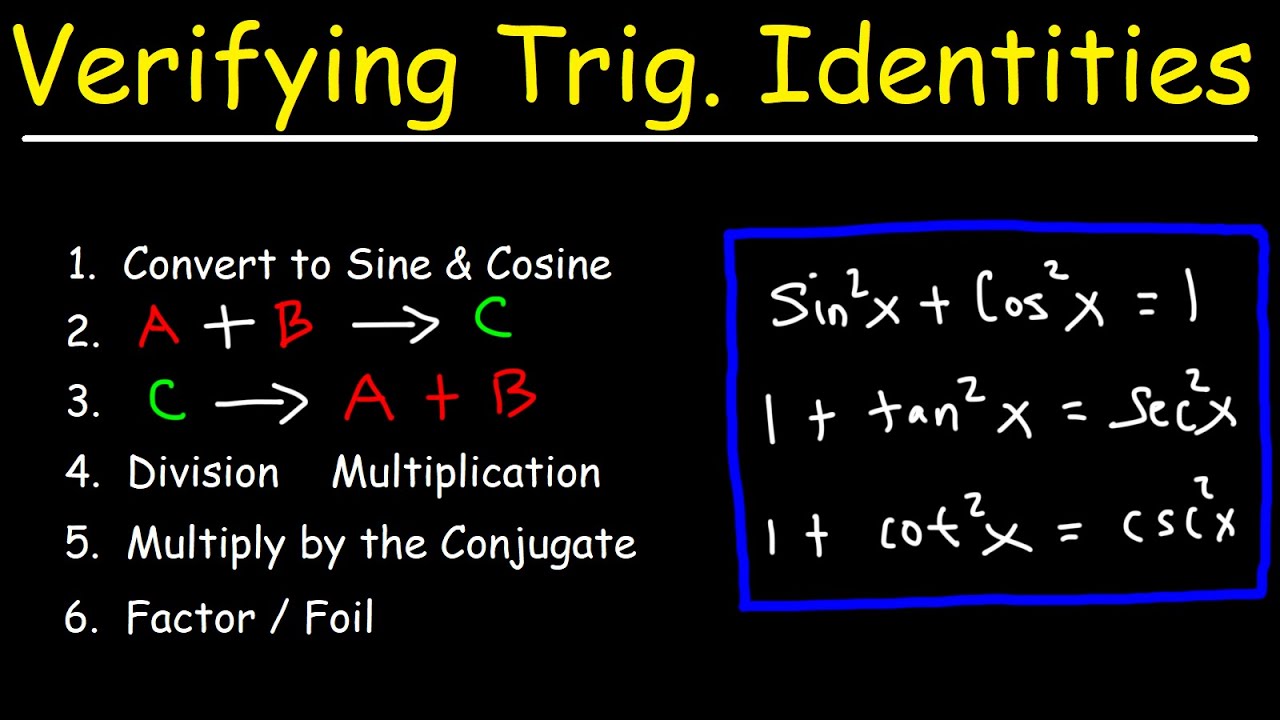
Verifying Trigonometric Identities

How to Prove Trigonometric Identities (Precalculus - Trigonometry 24)

Introduction to Using Trigonometric Identities (Precalculus - Trigonometry 23)

Precalc 5.1 Using Fundamental Trig Identities

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
TrigonometryIdentitiesMath TutorialAlgebraic SkillsEducationalPuzzle SolvingSimplificationFundamental IdentitiesPythagoreanProblem-Solving