4/21 Algebra Zoom class
TLDRThe teacher guides students through a lesson on completing the square for quadratic equations. The session focuses on solving problems, understanding the process, and simplifying radicals. Students are instructed to work on the odd-numbered problems, with the teacher demonstrating a few even-numbered examples. Emphasis is placed on showing work and checking answers using the provided answer key. Questions from students are addressed, ensuring clarity on specific steps, especially when dealing with fractions and simplifying radicals. The session concludes with group work and additional guidance for more challenging problems.
Takeaways
- 📝 This class session focuses on practicing completing the square.
- 🎥 Students have video notes and homework to watch three videos and complete a guide on the quadratic formula.
- 📄 The worksheet for today involves solving ten odd-numbered problems.
- ✅ The answer key is provided to check work and ensure accuracy.
- 👨🏫 The teacher demonstrates solving even-numbered problems to clarify the process.
- ✍️ Key steps include adding terms to both sides, simplifying, and ensuring the equation is in standard form.
- 🔍 Specific problems like number 12 and number 18 are worked through in detail to illustrate the method.
- 📚 Students are encouraged to ask questions if they encounter difficulties, especially with fractions and square roots.
- 🤝 Students are divided into groups to work on problems, with the teacher available for assistance.
- 🖥️ Technical difficulties with the whiteboard are addressed, and problem-solving continues effectively.
Q & A
What is the primary focus of the class activity described in the transcript?
-The primary focus of the class activity is practicing completing the square for quadratic equations.
What specific instruction is given for homework?
-Students are instructed to watch three videos and complete the guide for additional notes on the quadratic formula.
What is the purpose of the answer key provided in the worksheet?
-The answer key is provided so students can check their work and ensure they are solving the problems correctly.
Which problems from the worksheet are the students assigned to work on individually?
-Students are assigned to work on the odd-numbered problems individually.
What method is the teacher using to explain the problems during the class?
-The teacher is demonstrating how to solve a few even-numbered problems together with the class to ensure students understand the process.
What should students do if their answers do not match the provided answers?
-Students should go back and check their steps to find and correct any mistakes.
How does the teacher plan to use the whiteboard during the lesson?
-The teacher plans to copy problems onto the whiteboard and solve them there, sharing the whiteboard with the class.
What does the teacher do when a student has a question during the lesson?
-The teacher addresses the student's question and clarifies the concept or problem they are unsure about.
How does the teacher suggest handling coefficients in quadratic equations when completing the square?
-The teacher suggests dividing the entire equation by the coefficient to simplify the quadratic term to have a coefficient of one.
What additional steps are taken if the quadratic equation involves a fraction?
-The teacher explains how to create a common denominator and simplify the fraction, then solve the equation by completing the square.
Outlines
📚 Final Practice Day on Completing the Square
The instructor introduces the final day of practice for the class, focusing on completing the square, applicable to fourth, fifth, and sixth periods. Students are informed about video notes and homework involving three videos, guide completion, and extra guided notes on the quadratic formula. The instructor also mentions leaving the answer key on the worksheet for students to check their work, emphasizing the importance of understanding the process and steps, not just the final answers.
🔍 Detailed Walkthrough of Completing the Square
The instructor provides a step-by-step guide on how to complete the square, starting with moving variable terms to one side of the equation and constants to the other. The process involves creating a perfect square trinomial and simplifying square roots. The example given is a quadratic equation where the instructor demonstrates adding and subtracting terms to both sides to complete the square, simplifying the square root of 63 into 3 times the square root of 7, and solving for the variable n.
🤔 Addressing Student Questions on Completing the Square
The instructor addresses student questions about the method of completing the square, specifically on simplifying square roots and dealing with equations where the first term coefficient is not one. The discussion includes the importance of simplifying radicals when possible and handling fractions that result from the process. The instructor also clarifies the process for equations with terms like '2 plus x' and how to adjust for them.
📝 Dealing with Fractions and Coefficients in Completing the Square
The instructor tackles a complex problem involving fractions and coefficients different from one. The process involves dividing all terms by the coefficient to simplify the equation, moving fractions to the other side, and dealing with the resulting complications. The instructor emphasizes the importance of maintaining a common denominator and the method of multiplying by a fraction to take half of a term, as seen in the example where 13/9 is multiplied by 1/2 to find half of the term.
🧩 Completing the Square with Fractions and Irrational Numbers
The instructor continues the process of completing the square with a focus on handling fractions and irrational numbers. The example provided involves creating a common denominator, simplifying the equation, and taking the square root of non-perfect squares. The instructor simplifies the square root of 925 by factoring out the square root of 25, resulting in an irrational number that cannot be simplified further.
📉 Breaking Down Complex Fractions in Quadratic Equations
The instructor works through the final steps of solving a complex quadratic equation with fractions, emphasizing the importance of simplifying the radical and rationalizing the denominator. The process includes factoring down the equation into a binomial format, taking the square root of both sides, and simplifying the resulting expression. The instructor confirms the correctness of the solution and relates it to the answer key.
👥 Group Work and Individual Assistance
The instructor organizes the class into groups for further work on the problems, offering individual assistance as needed. There is a focus on problem numbers 13 and 15, which are identified as potentially difficult. The instructor moves between rooms to help students with their work, answering questions, and ensuring understanding of the concepts taught.
🔄 Clarifying Steps and Rationalizing Denominators
The instructor clarifies the steps for completing the square, particularly the process of rationalizing the denominator. Students are shown how to adjust terms and simplify equations, with an emphasis on the correct format for the final answer. The discussion includes the rationale behind changing the format of the answer to match the answer key.
🤝 Final Review and Assistance for Persistent Students
The session concludes with the instructor offering final assistance to students who stayed beyond the scheduled time. The instructor agrees to solve an additional problem, number 15, as a reward for the students' dedication. There is a sense of camaraderie as the instructor thanks the students for their hard work and invites them to join the main room for the final review.
Mindmap
Keywords
💡Completing the Square
💡Quadratic Formula
💡Standard Form
💡Perfect Square Trinomial
💡Simplifying Radicals
💡Factoring
💡Binomial
💡Radical
💡Coefficients
💡Order of Operations
Highlights
Today, we're focusing on completing the square for quadratic equations. This is our last practice session.
Homework assignment: Watch three videos and complete the guided notes on the quadratic formula.
The worksheet includes ten problems, focusing on odd-numbered questions. We'll do a few even-numbered problems together.
The answer key is provided so you can check your work. If your answer doesn't match, review your steps.
Detailed explanation of how to complete the square for a quadratic equation by converting it to standard form.
Example problem: Converting the equation -3n^2 + 4n - 59 = 0 to standard form and solving it by completing the square.
Important step: Adding the square of half the middle term to both sides to form a perfect square trinomial.
Simplifying square roots: Review of breaking down square roots into simpler factors.
For complex problems, use fraction multiplication to handle coefficients and maintain common denominators.
Simplifying radicals: The importance of identifying perfect square factors for further simplification.
Handling variable terms: Moving variable terms to one side and clearing constants for easier solving.
Group work setup: Students will break into groups to practice solving the problems together.
Additional practice: Focusing on challenging problems that involve non-integer coefficients.
Use of the whiteboard for collaborative problem-solving and visualizing steps.
Q&A session: Addressing students' questions and clarifying steps for solving quadratic equations.
Transcripts
Browse More Related Video
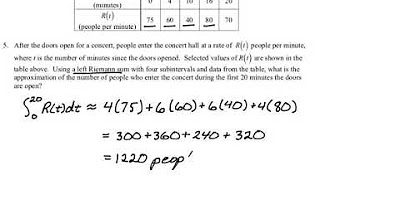
AP Calc AB & BC Practice MC Review Problems #1

[LIVE] zoom Math Calculus For Business | Integrals by substitution #byparts #partialfractionmethod
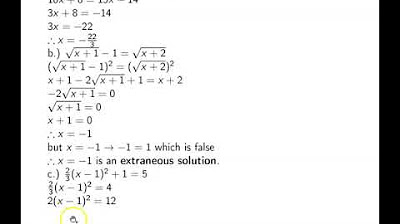
Ch. 1.5 Equations
2022 Live Review 4 | AP Calculus AB | Integrals & Using the Fundamental Theorem of Calculus

Ch. 1.7 Modeling with Equations
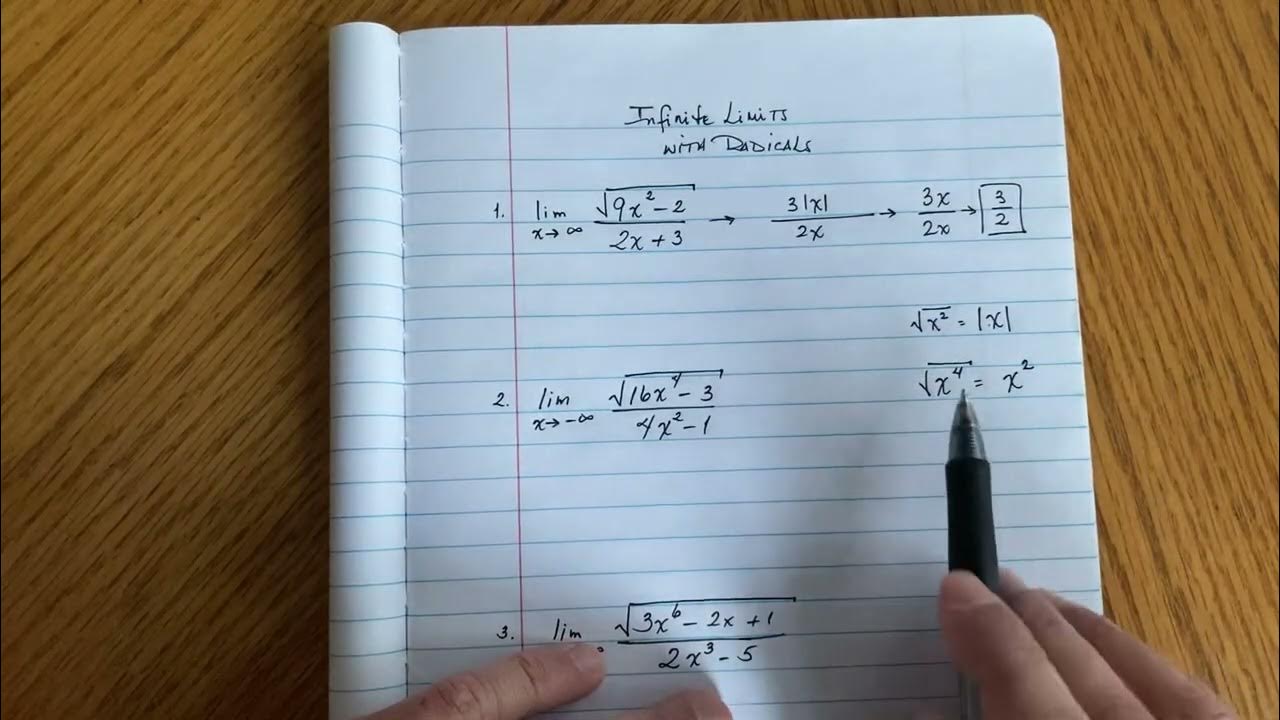
Infinite Limits With Radicals
5.0 / 5 (0 votes)
Thanks for rating: