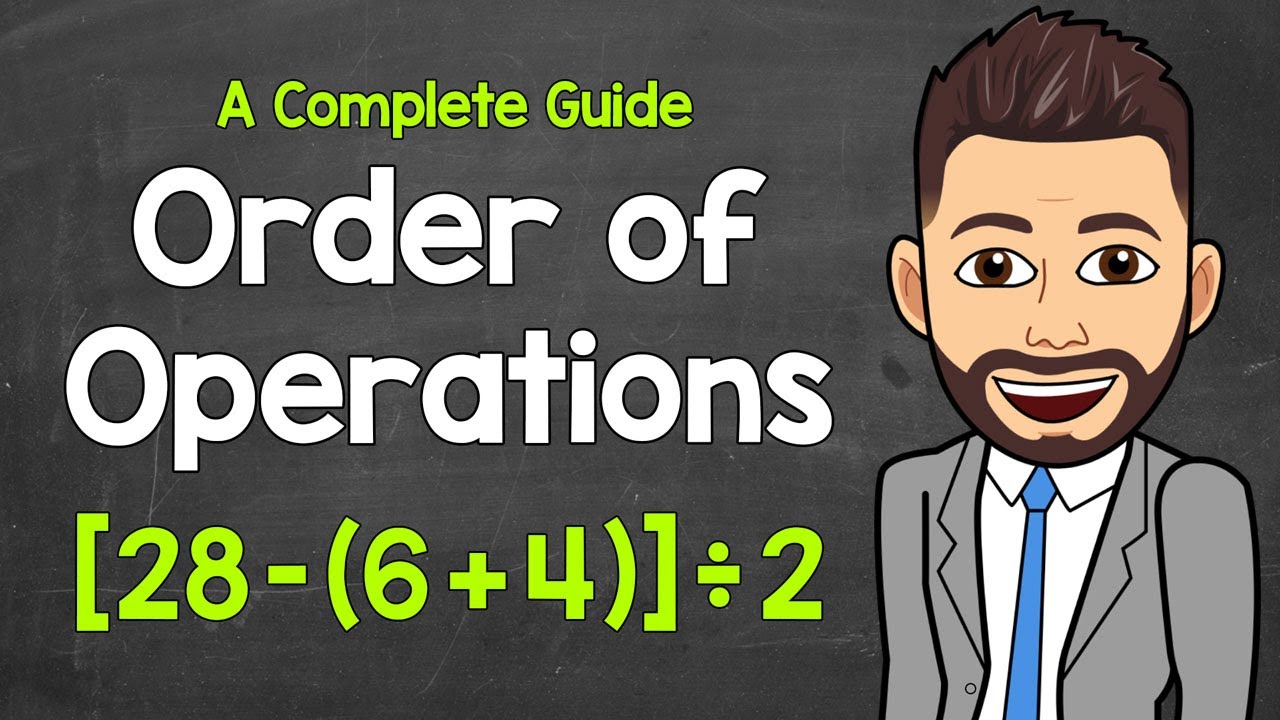
How To Evaluate Expressions With Variables Using Order of Operations

The Organic Chemistry Tutor
25 Jun 201711:35
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video script delves into the intricacies of evaluating mathematical expressions involving variables, fractions, and exponents. It emphasizes the importance of the order of operations, using the acronym PEMDAS to guide viewers through the correct sequence: Parentheses, Exponents, Multiplication and Division (from left to right), and Addition and Subtraction (from left to right). The script provides step-by-step examples to illustrate how to handle complex expressions and demonstrates the process of evaluating algebraic expressions by substituting given values for variables. It also highlights the impact of operation order on the final result, ensuring viewers understand the fundamental rules of mathematical computation.

Takeaways
  • 📚 The importance of order of operations is emphasized, with the acronym PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction) guiding the sequence of mathematical operations.
  • 🔢 Multiplication and division are of equal priority and should be performed from left to right when they appear in an expression without parentheses.
  • ➕ Addition and subtraction also have equal priority and are executed from left to right in an expression.
  • 👉 Parentheses take precedence over all other operations and should be calculated first.
  • 💡 Exponents represent repeated multiplication and are calculated before multiplication and division.
  • 🤔 The script provides examples to illustrate the correct order of operations, emphasizing the difference in outcomes based on the order in which operations are performed.
  • 📉 In expressions with variables and exponents, the order of operations must still be followed, with exponents calculated first, followed by multiplication and division, and finally addition and subtraction.
  • 📌 The video script includes examples with variables, demonstrating how to substitute values for variables and then perform the operations in the correct order.
  • 📘 The process of evaluating algebraic expressions is explained step by step, with a focus on correctly applying the order of operations.
  • 📊 The script also covers expressions with fractions, showing how to convert them into multiplication and division for easier calculation.
  • 📚 The final examples in the script involve more complex expressions, reinforcing the need to follow the order of operations to arrive at the correct answer.
Q & A

  • What is the correct order of operations in mathematics?

    -The correct order of operations is given by the acronym PEMDAS, which stands for Parentheses, Exponents, Multiplication and Division (from left to right), Addition and Subtraction (from left to right).

  • Why does the order of operations matter in evaluating expressions?

    -The order of operations matters because it determines the sequence in which calculations are performed, which can lead to different results if not followed correctly.

  • What is the result of the expression 7 + 4 * 3 when evaluated using the order of operations?

    -Following the order of operations, you first multiply 4 by 3 to get 12, then add 7 to get a result of 19.

  • How does the order of operations affect the expression 36 - 12 / 3?

    -According to PEMDAS, division has priority over subtraction, so you divide 12 by 3 to get 4, and then subtract that from 36 to get 32.

  • What is the result of the expression 24 / 6 * 2 if you perform the operations from left to right?

    -When you divide 24 by 6 first, you get 4, and then multiplying that by 2 gives you a result of 8.

  • How do you evaluate the expression 8 * 5 / 4?

    -You can simply multiply from left to right: 8 times 5 gives 40, and then dividing that by 4 gives a result of 10.

  • What is the result of the expression 24 + 12 / 10 - 4?

    -First, you add 24 and 12 to get 36, then subtract 4 from 10 to get 6, and finally divide 36 by 6 to get a result of 6.

  • How do you evaluate the expression involving the sum and product within parentheses: 4 * (3 + 5) - 7 * 2?

    -First, calculate the sum inside the parentheses (3 + 5 = 8), then multiply by 4 to get 32, and subtract the product of 7 and 2 (14) from 32 to get a result of 18.

  • What is the result of the complex expression 3 * 4 - 2 * (3^3 - 2^4) + 8 * 2?

    -First, calculate the exponents: 3^3 is 27 and 2^4 is 16. Then perform the operations inside the parentheses (27 - 16 = 11), multiply by 2 to get 22, subtract that from 4, and continue with the rest of the expression to get a final result of -38.

  • How do you evaluate an algebraic expression with variables like 4x + 2y - 3z given specific values for x, y, and z?

    -Substitute the given values for x, y, and z into the expression. For example, if x=2, y=3, and z=-4, you would calculate 4*2 + 2*3 - 3*(-4), which simplifies to 8 + 6 + 12, resulting in 26.

  • What is the result of the expression x^2 + 3y^3 / (2z + 1) given x=4, y=2, and z=4.5?

    -First, calculate the squares and cubes: x^2 is 16 and y^3 is 8. Then multiply 3 by 8 to get 24. Next, calculate the denominator: 2z + 1 is 2*4.5 + 1, which is 9 + 1. Finally, divide (16 + 24) by 10 to get a result of 4.

  • How do you evaluate the expression 4x + y - z / 3 with x=3, y=4, and z=12?

    -First, add x and y to get 3 + 4 = 7. Then divide z by 3 to get 12 / 3 = 4. Multiply these results to get 4 * 7 = 28, and subtract the division result to get 28 - 4, resulting in 24.

Outlines
00:00
🧮 Understanding Order of Operations

This paragraph introduces the concept of order of operations, emphasizing the importance of following the correct sequence when performing mathematical calculations. It explains the acronym PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction) as a guide to remember the hierarchy of operations. The paragraph provides examples to illustrate how the order affects the outcome, such as calculating '7 + 4 * 3' which results in 19, not 33, due to multiplication being performed before addition. It also discusses the equal priority of multiplication and division, and addition and subtraction, and how to proceed when these operations are adjacent.

05:04
📚 Advanced Mathematical Expressions with PEMDAS

The second paragraph delves into more complex mathematical expressions involving variables, fractions, and exponents, while still adhering to the order of operations. It demonstrates how to evaluate expressions with parentheses and exponents, such as '3 * 4 - 2 * 3^3 - 2^4 + 8 * 2', by first addressing the exponents and then proceeding with the rest of the operations in the correct order. The paragraph also covers how to handle expressions with variables, like '4x + 2y - 3z', by substituting the given values for x, y, and z before performing the calculations.

10:04
🔢 Evaluating Algebraic Expressions with Variables

The final paragraph focuses on evaluating algebraic expressions that include variables and operations. It provides step-by-step instructions on how to substitute values for variables within an expression and then carry out the necessary calculations. Examples given include expressions with squared and cubed terms, as well as division by a variable. The paragraph concludes with a straightforward example of '4x + y - z / 3', where specific values for x, y, and z are substituted to arrive at the final answer, reinforcing the process of evaluating expressions by following the order of operations and performing substitutions.

Mindmap
Keywords
💡Order of Operations
The 'Order of Operations' is a fundamental mathematical principle that dictates the sequence in which operations should be performed in an expression. It is crucial for correct evaluation and is often remembered by the acronym PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction). In the video, it is used to explain why certain operations are performed before others, such as multiplying before adding in the expression '7 + 4 * 3', which results in 19 rather than 33.
💡Parentheses
Parentheses are symbols used in mathematical expressions to indicate that the operations within them should be performed first. This is part of the 'Order of Operations' rule. In the script, it is mentioned that parentheses have the highest priority, and an example is given where the sum of 'three plus five' is calculated before being multiplied by four.
💡Exponents
Exponents, also known as powers, are used to denote repeated multiplication of a number by itself. For example, 'three to the third power' means multiplying three by itself three times, which equals 27. In the video, exponents are explained as part of the PEMDAS rule and are used in a complex expression to demonstrate their priority in calculation.
💡Multiplication
Multiplication is one of the basic arithmetic operations that involves combining groups of equal size. In the context of the video, multiplication is shown to have higher priority than addition according to the PEMDAS rule, as demonstrated in the expression '7 + 4 * 3', where multiplication is performed before addition.
💡Division
Division is the arithmetic operation of splitting a quantity into a number of equal parts. The script uses the example '36 - 12 / 3' to illustrate that division should be performed before subtraction, following the order of operations.
💡Addition
Addition is the process of combining two or more numbers to find their total or sum. In the video, addition is shown to have the same priority as subtraction, and it is performed from left to right when they appear in sequence, as seen in the expression '24 / 6 * 2'.
💡Subtraction
Subtraction is the arithmetic operation of taking one quantity away from another. The video script explains that subtraction has the same priority as addition and is performed from left to right, as demonstrated in the expression '24 + 12 / 10 - 4'.
💡Variables
Variables are symbols, often letters, that represent unknown or changeable quantities in mathematics. In the script, variables are used in algebraic expressions like '4x + 2y - 3z', and specific values for x, y, and z are substituted to evaluate the expression.
💡Algebraic Expression
An algebraic expression is a combination of variables and constants joined by arithmetic operations. The video provides examples of algebraic expressions and demonstrates how to evaluate them by substituting values for the variables, such as in the expression '4x + 2y - 3z' where x=2, y=3, and z=-4.
💡Fractions
Fractions represent a part of a whole and are expressed as the ratio of two numbers. In the video, fractions are used in the context of division, such as '24 / 6', which simplifies to 4, and are integral to understanding how to evaluate expressions involving division.
Highlights

The importance of understanding the order of operations (PEMDAS) in performing mathematical calculations is emphasized, highlighting the need to prioritize operations based on their position in an expression.

The correct order of operations for the expression '7 plus 4 times 3' is multiplication before addition, resulting in 19 instead of 33.

The expression '36 minus 12 divided by 3' demonstrates the priority of division over subtraction, leading to the correct answer of 32.

The concept of PEMDAS is further explained, with multiplication and division having equal priority and addition and subtraction having the same priority, but multiplication and division are performed from left to right.

The expression '24 divided by 6 multiplied by 2' illustrates the importance of the order of operations, with multiplication and division having the same priority, leading to different answers depending on the order.

The example '24 plus 12 divided by 10 minus 4' is used to show that evaluating algebraic expressions involves substituting values and performing the operations from left to right.

The expression 'three multiplied by four minus two times three to the third power minus two to the fourth power plus eight times two' demonstrates the need to work inside parentheses and handle exponents correctly.

The calculation '4x plus 2y, minus 3z' with x=2, y=3, and z=-4 shows how substitution of values into an algebraic expression leads to the correct answer.

The expression 'x squared plus three y cubed divided by two z plus one' with x=4, y=2, and z=4.5 is used to illustrate the process of evaluating expressions with variables, resulting in 9.

The final example '4 times x plus y, minus z divided by 3' with x=3, y=4, and z=12 is used to demonstrate the process of evaluating an expression with variables, leading to the correct answer of 24.

Transcripts

Browse More Related Video

Order of Arithmetic Operations: PEMDAS

Math Antics - Order Of Operations

a very simple algebra question , but 97% FAILED

Order of Operations Explained | PEMDAS | A Complete Guide | Math with Mr. J

Algebra Basics: Solving 2-Step Equations - Math Antics

How to Rearrange Equations in Chemistry

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsExpressionsVariablesFractionsExponentsOrder of OperationsPEMDASAlgebraCalculusEducationalTutorial