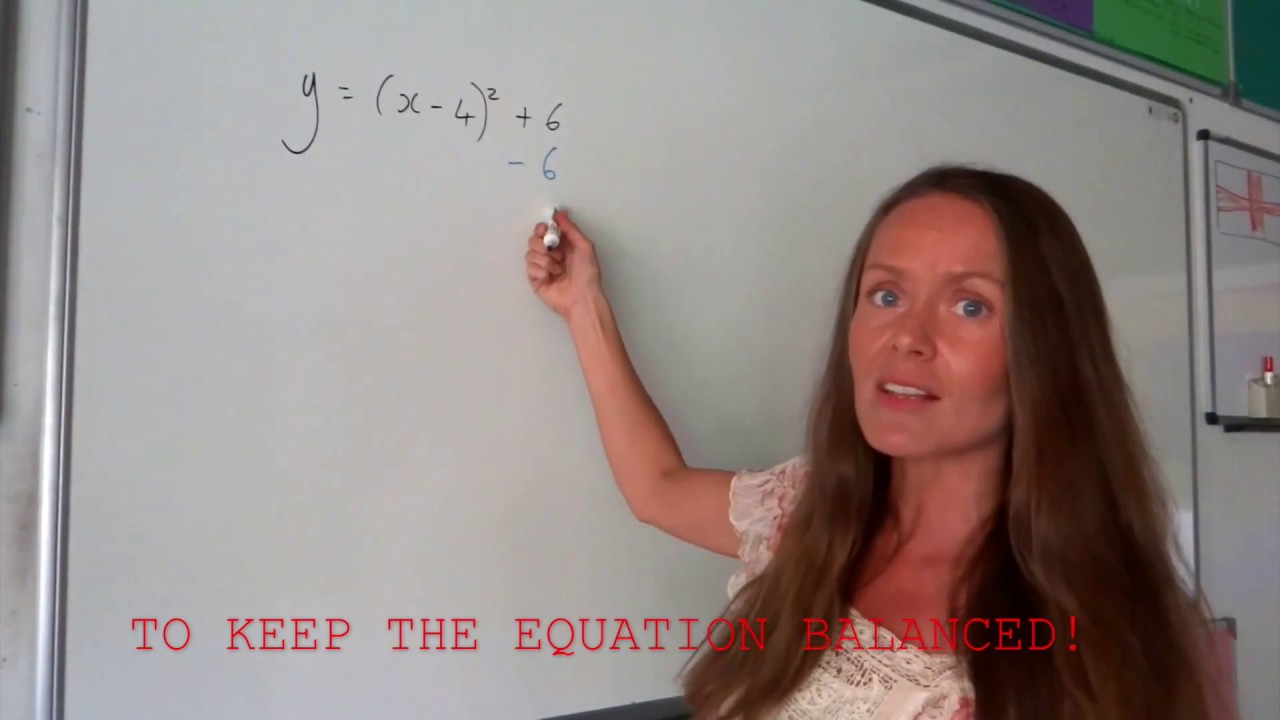How to Rearrange Equations in Chemistry
TLDRThe video script offers a comprehensive guide on how to effectively rearrange chemical formulas to solve for different variables. It emphasizes the importance of understanding algebraic rules and applying them to manipulate formulas, rather than memorizing each formula. The guide covers basic operations such as addition, subtraction, multiplication, and division, and extends to more complex concepts like dealing with fractions, roots, and powers. It demonstrates how to isolate variables by performing opposite operations and using tricks like multiplying diagonally to eliminate fractions. The script provides step-by-step solutions for various examples, including thermochemistry formulas and the ideal gas law, highlighting common mistakes students make. It concludes with a challenging problem from Bohr's model, showcasing the process of isolating a variable through a series of algebraic steps. The video encourages viewers to practice these skills with provided problems to enhance their understanding and proficiency in chemistry.
Takeaways
- 📚 **Understanding Algebra**: It's crucial to grasp algebraic rules for rearranging formulas, which can simplify chemistry problems.
- 🔍 **Rearranging Formulas**: To isolate a variable, perform the opposite operation on the terms that are not allowing the variable to be alone.
- 🧮 **Basic Algebra Review**: Reviewing basic algebra operations is essential before tackling more complex chemistry formulas.
- ➕ **Subtraction for Addition**: When a variable is added with something else, subtract that something to isolate the variable.
- ➖ **Addition for Subtraction**: If a variable is being subtracted, add it back to both sides to isolate the variable.
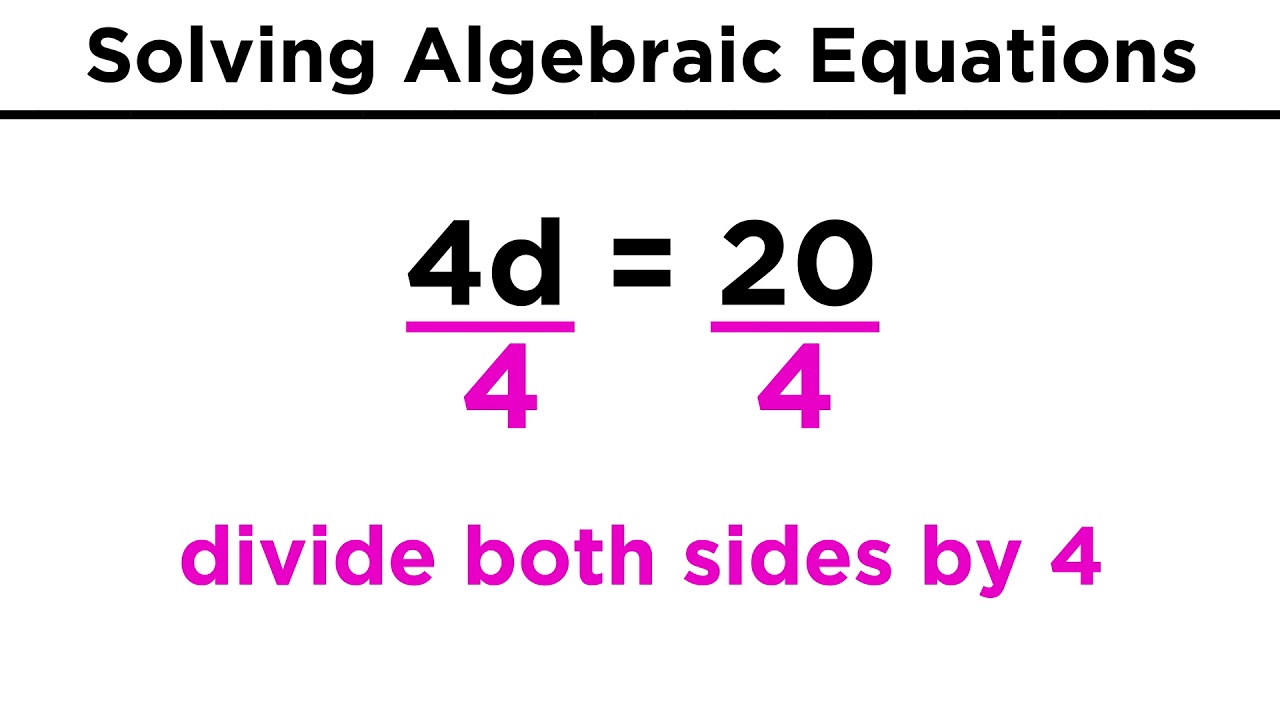
- 🔄 **Multiplication and Division**: To isolate a variable multiplied or divided by another, perform division or multiplication respectively by the opposite number.
- 📏 **Diagonal Multiplication**: For equations with two fractions set equal to each other, multiply diagonally to eliminate fractions.
- ⛰ **Powers and Roots**: To eliminate a power, take the root of both sides, and to eliminate a root, raise both sides to the power.
- 🔥 **Thermochemistry Formulas**: Practice rearranging formulas in thermochemistry to isolate different variables like 'q'.
- 🌌 **Ideal Gas Law**: When solving for variables in the ideal gas law, identify what's preventing the variable from being alone and perform the opposite operation.
- 🔗 **Combined Gas Law**: For combined gas law problems, use diagonal multiplication to eliminate fractions and isolate the desired variable.
- 🤔 **Bohr's Model Challenge**: When solving complex formulas like those from Bohr's model, eliminate terms outside parentheses first, then isolate the variable step by step.
Q & A
What is a variable in the context of chemistry and algebra?
-A variable is a symbol or letter that represents a value in chemistry and algebra. It is used to stand in for a quantity that can change.
Why is it beneficial to understand how to rearrange formulas in chemistry?
-Understanding how to rearrange formulas allows you to solve for different variables, making it easier to apply the formulas to various problems without needing to memorize each one solved for every possible variable.
What is the basic rule for moving a variable to the opposite side of a formula in algebra?
-To move a variable to the opposite side of a formula, you must perform the opposite operation. If the variable is being added, you subtract it; if it is being subtracted, you add it. This is done for both sides of the formula to maintain equality.
How do you deal with fractions set equal to each other in algebra?
-When dealing with two fractions set equal to each other, you can multiply diagonally. This means multiplying the numerator of the first fraction by the denominator of the second fraction and setting it equal to the product of the numerator of the second fraction and the denominator of the first fraction.
What is the process to get rid of a power in an algebraic expression?
-To get rid of a power, you raise both sides of the equation by the same root. For example, to eliminate a power of 2, you would take the square root of both sides.
How do you remove a root from an equation?
-To remove a root from an equation, you raise both sides to the power that corresponds to the root. For instance, to eliminate a square root, you would square both sides of the equation.
What is the ideal gas law formula?
-The ideal gas law formula is PV = nRT, where P is the pressure, V is the volume, n is the number of moles, R is the ideal gas constant, and T is the temperature in Kelvin.
How do you solve for n in the ideal gas law formula?
-To solve for n in the ideal gas law formula, you would divide both sides of the equation by RT (R and T together), which cancels out the R and T on the left side, leaving you with n = PV/RT.
What is the combined gas law in chemistry?
-The combined gas law is derived from Boyle's Law, Charles's Law, and Avogadro's Law. It is expressed as (P1V1/T1) = (P2V2/T2), which relates the pressure and volume of a gas at two different temperatures and pressures.
How do you solve for V1 in a gas law that has V1 in the denominator?
-To solve for V1, you would multiply both sides of the equation by T1 (the denominator causing V1 not to be alone), which cancels out the T1 on the left side, leaving you with V1 = (PV2/(T2) * T1).
What is Bohr's model and how is it used in chemistry?
-Bohr's model is a theory that describes the behavior of electrons in atoms, suggesting that electrons orbit the nucleus in quantized paths or shells. It is used to calculate the energy levels of electrons and predict the spectral lines of the hydrogen atom.
How do you solve for the initial quantum number (n initial) in Bohr's model?
-To solve for n initial, you would first eliminate anything outside the parentheses by performing the opposite operation (division if it's multiplication). Then, you would isolate n initial by dividing both sides by the remaining terms in the parentheses and taking the square root of both sides.
Outlines
🔍 Understanding Algebraic Formula Manipulation in Chemistry
This paragraph introduces the concept of algebraic manipulation in chemistry, emphasizing the importance of understanding how to rearrange formulas to solve for different variables. It explains the use of algebraic rules to move variables to the opposite side of an equation by performing opposite operations. The paragraph also covers the process of solving for a variable in the presence of addition, subtraction, multiplication, and division, as well as dealing with fractions and roots. It concludes with an example of applying these principles to a thermochemistry formula to solve for 'q'.
🧮 Advanced Algebraic Techniques and Gas Law Applications
The second paragraph delves into more advanced algebraic techniques, including the manipulation of fractions and roots in equations. It demonstrates how to eliminate fractions by multiplying diagonally and how to deal with powers and roots by raising both sides of an equation to the same power or taking the root. The paragraph then applies these techniques to solve for variables in the ideal gas law and other gas law formulas, illustrating common mistakes students make. It concludes with a complex example using Bohr's model to solve for 'n initial', showcasing the process of isolating a variable and emphasizing the importance of algebraic manipulation for solving chemistry problems.
Mindmap
Keywords
💡Formula
💡Variable
💡Algebra Rules
💡Opposite Operation
💡Multiplication and Division
💡Fractions
💡Roots and Powers
💡Thermochemistry
💡Ideal Gas Law
💡Gas Law Formulas
💡Bohr's Model
💡Practice Problems
Highlights
Understanding how to rearrange formulas is crucial in Chemistry, and it can simplify memorization.
Variables in formulas represent values and can be rearranged using algebra rules.
To move a variable to the opposite side of a formula, perform the opposite operation on both sides.
Reviewing basic algebra rules is essential before tackling more complex formulas.
Subtracting or adding terms to isolate a variable x is a fundamental technique.
Multiplication and division in formulas require the opposite operation to isolate a variable.
When dealing with fractions set equal to each other, multiply diagonally to eliminate them.
To remove a power from an equation, raise both sides to the same root.
Raising both sides to the power of a root eliminates the root from the equation.
Thermochemistry formulas can be rearranged to solve for different variables like q.
Common mistakes with gas law formulas can be avoided by correctly applying algebraic operations.
The ideal gas law can be rearranged to solve for the number of moles (n) by dividing by RT.
For another gas law, multiplying by the denominator allows solving for volume (V1).
Diagonal multiplication is a trick to eliminate fractions in equations with equal fractions.
The combined gas law can be rearranged to solve for temperature (T2) by eliminating fractions and canceling terms.
Bohr's model formula can be rearranged to solve for the initial quantum number (n initial) by isolating and applying algebraic operations.
Practicing rearrangement of formulas with given variable values is a key skill for solving Chemistry problems.
Completing practice problems and watching further videos in the playlist can enhance understanding and proficiency in formula rearrangement.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: