Order of Operations Explained | PEMDAS | A Complete Guide | Math with Mr. J

Math with Mr. J

3 Aug 202351:15

EducationalLearning

32 Likes 10 Comments

TLDRIn this comprehensive guide by Mr. Jay, the importance of the order of operations (PEMDAS) in mathematics is emphasized for ensuring consistent problem-solving and avoiding confusion. The video illustrates the process with various examples, starting with simple expressions and progressively incorporating parentheses, exponents, and complex scenarios like nested parentheses and fraction bars. It also covers how to handle positive and negative integers, reinforcing the need for a standardized approach to achieve the same results across different problems.

Takeaways

📚 The video is a comprehensive guide on the order of operations, also known as PEMDAS (Parentheses, Exponents, Multiplication and Division, and Addition and Subtraction).
🔢 The purpose of the order of operations is to ensure everyone solves math problems in the same way, leading to the same answers and avoiding confusion.
🔄 An example given in the video demonstrates that without a standard order, simple problems like 12 - 5 * 2 can have different answers when calculated in different orders.
👉 The video uses the acronym PEMDAS to help remember the sequence of operations that should be followed when solving mathematical expressions.
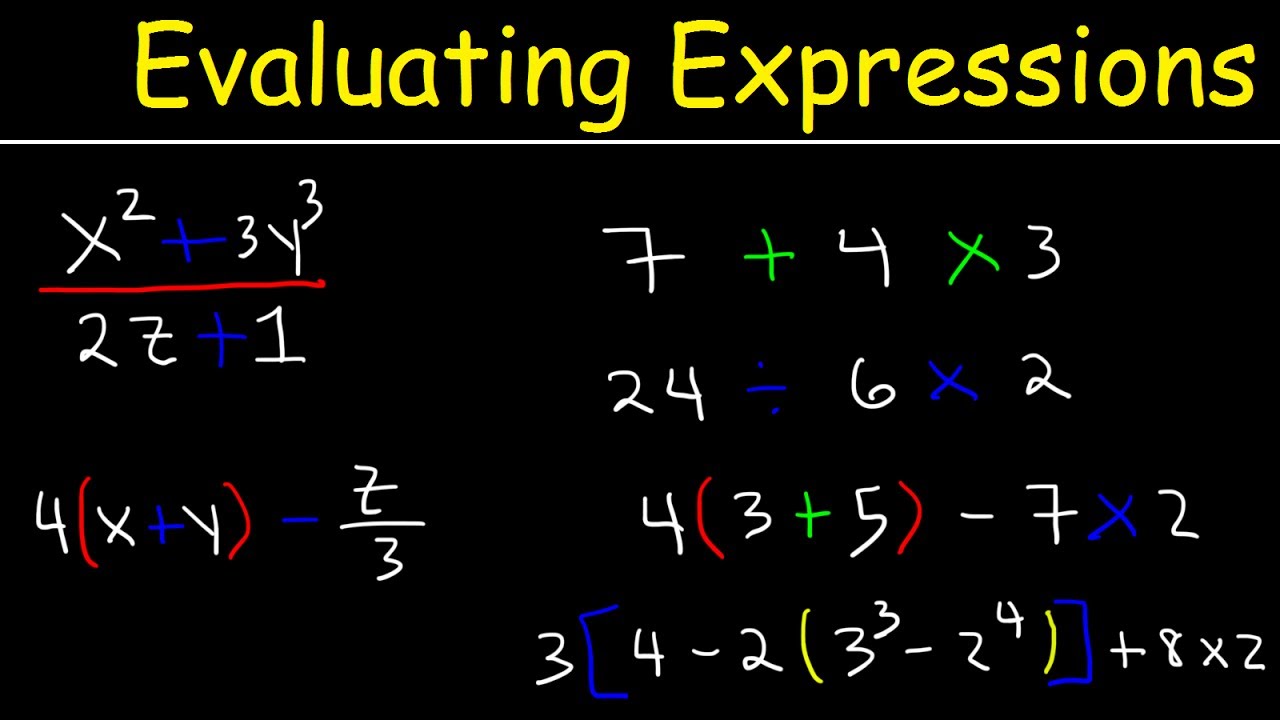
📐 The video provides a series of examples to illustrate how to apply the order of operations, starting with solving expressions within parentheses first.
📈 The script explains that multiplication and division are of equal priority and should be performed from left to right, as should addition and subtraction.
📝 The video script includes examples with multiple grouping symbols like parentheses, brackets, and braces, emphasizing that calculations inside the innermost group should be done first.
🎯 The guide also covers problems involving fraction bars, which act as grouping symbols requiring the numerator to be divided by the denominator.
✅ The video demonstrates that even complex problems can be solved step by step by following the order of operations, including those with fraction bars and mixed operations.
🤔 The importance of keeping track of negative signs and the impact of parentheses on the outcome of exponentiation is highlighted in examples involving negative numbers.
📊 The script concludes with a reminder that the order of operations is essential for consistency and correctness in mathematical problem-solving.

Q & A

What is the purpose of the order of operations in mathematics?
-The order of operations ensures that everyone works through math problems in the same way, leading to the same answers and avoiding confusion about the process.
What does PEMDAS stand for and what does it represent?
-PEMDAS stands for Parentheses, Exponents, Multiplication and Division (from left to right), and Addition and Subtraction (from left to right). It represents the standard order in which operations should be performed in mathematical expressions.
Why is it important to follow the order of operations?
-Following the order of operations is crucial because it leads to consistent and correct answers. Without it, even simple problems can have different answers depending on the approach taken.
What is an example of how different approaches can lead to different answers without the order of operations?
-The script provides the example of calculating '12 - 5 * 2'. If one starts by subtracting 12 - 5 first, the result is 14. However, if multiplication is done first (5 * 2), the result is 2, showing that the order greatly affects the outcome.
How does the order of operations relate to standardization in other areas, such as traffic rules or measurement units?
-Just as traffic rules and measurement units are standardized to avoid confusion and ensure everyone is on the same page, the order of operations serves as a standardized set of rules in mathematics to ensure consistent results.
What is the correct answer to the problem 12 - 5 * 2 when following the order of operations?
-Following the order of operations, which dictates that multiplication comes before subtraction, the correct answer is 2 (12 - (5 * 2)).
Can you explain the process of solving the expression 16 - 5 * 3 + 12 using the order of operations?
-First, perform the multiplication: 5 * 3 = 15. Then, perform the subtraction from left to right: 16 - 15 = 1, and finally, add the remaining numbers: 1 + 12 = 13. The final answer is 13.
How should you approach a problem with multiple grouping symbols like parentheses, brackets, and braces?
-When encountering multiple grouping symbols, always start with the innermost group and work your way outward. This means solving expressions within the innermost parentheses first, then moving to brackets, and finally braces.
What is the role of a fraction bar in mathematical expressions?
-A fraction bar acts as a grouping symbol that separates the numerator (top part) from the denominator (bottom part). It instructs to calculate the result of the numerator and denominator separately and then divide the numerator by the denominator.
Can you provide an example of simplifying a fraction obtained from an order of operations problem?
-The script provides an example where 6/18 is simplified by dividing both the numerator and the denominator by their greatest common factor, which is 6, resulting in the simplified fraction 1/3.
How does the order of operations apply when dealing with negative numbers?
-The order of operations remains the same regardless of whether the numbers are positive or negative. The key is to correctly apply the rules of arithmetic with negative numbers, keeping track of the signs throughout the calculation.
What is the final answer to the problem involving negative numbers: (-4 - 4)^2 + (-3)^3 / 9?
-The final answer to the problem is 61. The process involves squaring -8 (resulting in 64), cubing -3 (resulting in -27), and then dividing -27 by 9 to get -3, and finally adding 64 and -3 to arrive at 61.

Outlines

00:00

📚 Introduction to Order of Operations

Mr. Jay introduces the concept of order of operations, emphasizing its importance for standardized problem-solving. He explains the acronym PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction) and illustrates the necessity of a uniform approach with an example: calculating 12 - 5 * 2 in two different ways, resulting in different answers without the order of operations. This highlights the need for a set of rules to ensure everyone arrives at the same solution.

05:01

🔢 Order of Operations: Acronym PEMDAS and Examples

The video script explains the acronym PEMDAS and its significance in remembering the order of operations. It delves into example problems, demonstrating step-by-step solutions while adhering to the PEMDAS rule set. Problems involving parentheses, exponents, multiplication, division, addition, and subtraction are worked through, showing the process of solving mathematical expressions methodically and correctly.

10:01

📘 Multiplication and Division Prioritization

This section of the script focuses on the prioritization of multiplication and division over addition and subtraction, as per the PEMDAS rule. It provides an example problem involving the expression 7 squared minus 14 times 2, which is solved by first addressing the exponent and then proceeding with multiplication and subtraction in the correct order.

15:04

📙 Parentheses and Exponents in Order of Operations

The script discusses how to handle expressions with parentheses and exponents, emphasizing that operations within parentheses take precedence over exponents. An example is given where the expression 13 plus 5 times the result of (2 cubed plus 4) divided by 2 is calculated step by step, showing the correct application of the order of operations.

20:07

📔 Working Through Complex Problems with Multiple Grouping Symbols

The script presents a complex problem involving multiple grouping symbols like parentheses, brackets, and braces. It explains the strategy of addressing the innermost group first and then working outwards. An example is provided where the expression involves nested parentheses within brackets, and the viewer is guided through the process of solving it correctly.

25:07

📒 Fraction Bars as Grouping Symbols

This part of the script introduces fraction bars as grouping symbols, which require calculating the numerator and denominator separately before division. The viewer is shown how to work through problems with fraction bars, either by solving the numerator and denominator sequentially or side by side, ensuring the correct application of the order of operations.

30:07

📕 Complex Fractions and Simplification

The script addresses complex problems involving fraction bars where the result is a fraction that may need simplification. An example is given where the numerator is 5 squared minus 19 and the denominator is 3 times 6. After calculating the result as 6 over 18, the fraction is simplified by dividing both the numerator and the denominator by their greatest common factor, resulting in the simplified fraction of one-third.

35:10

📖 Handling Positive and Negative Integers

This section of the script covers problems involving positive and negative integers. It explains how to work through expressions with negative numbers, such as negative 18 divided by negative 2 times the result of negative 11 plus 6. The script emphasizes the importance of keeping track of negative signs and demonstrates how to correctly apply the order of operations to arrive at the final answer.

40:12

😃 Conclusion of the Order of Operations Guide

The script concludes with a summary of the complete guide to the order of operations. It reiterates the importance of following the PEMDAS rule set for consistent and accurate mathematical problem-solving. The viewer is thanked for watching, and the video ends on a friendly note, inviting them to watch more educational content in the future.

Mindmap

Keywords

💡Order of Operations

The 'Order of Operations' is a fundamental mathematical principle that dictates the sequence in which operations must be performed to correctly solve an expression. In the video, it is explained as PEMDAS, which stands for Parentheses, Exponents, Multiplication and Division (from left to right), and Addition and Subtraction (from left to right). The concept is central to the video's theme, as it provides a standardized approach to solving mathematical problems and preventing confusion, as illustrated in the example of 12 minus 5 times 2, which can be solved in two different ways without the order of operations.

💡Parentheses

Parentheses are symbols used in mathematical expressions to indicate that the operations within them should be performed first due to their highest priority in the order of operations. In the script, Mr. Jay emphasizes the importance of addressing expressions within parentheses before moving on to other operations, as seen in examples such as '13 minus 8 in parentheses' and 'six plus four in parentheses', which are solved before any other operations in the given problems.

💡Exponents

Exponents, often referred to as 'power' or 'order', are a way of indicating the number of times a base number is multiplied by itself. In the video, exponents are mentioned as the second level of priority in the order of operations after parentheses. An example from the script is '2 cubed', which means 2 multiplied by itself three times (2 * 2 * 2), resulting in 8, and is performed before any multiplication or division.

💡Multiplication and Division

Multiplication and division are arithmetic operations that are considered to have the same level of priority in the order of operations. The video script mentions that when both operations are present in an expression, they should be performed from left to right. An example given is 'five times two', which equals ten, and '18 divided by nine', which equals two, both of which are performed before addition or subtraction.

💡Addition and Subtraction

Addition and subtraction are basic arithmetic operations that involve combining or taking away quantities, respectively. According to the order of operations explained in the video, they have the same priority level and should be performed from left to right after multiplication and division have been completed. An instance from the script is '16 minus 15 plus 12', where subtraction and addition are carried out sequentially from left to right.

💡PEMDAS

PEMDAS is an acronym used to remember the order of operations in mathematics. It stands for Parentheses, Exponents, Multiplication and Division (from left to right), and Addition and Subtraction (from left to right). The video uses PEMDAS as a mnemonic device to help viewers recall the sequence of operations. The script mentions PEMDAS as a way to represent the order of operations and to guide viewers through solving various mathematical examples.

💡Fraction Bar

A fraction bar, also known as a fraction line, is a symbol used to separate the numerator and the denominator in a fraction, indicating that the numerator should be divided by the denominator. In the video, the fraction bar acts as a grouping symbol, and the script explains that expressions above and below the fraction bar should be grouped and solved separately before performing the division. An example provided is '10 plus 5 times 6 above the fraction bar' and '12 divided by 3 below the fraction bar', resulting in the fraction 40/4, which simplifies to 10.

💡Nested Parentheses

Nested parentheses refer to a situation where parentheses are found within other grouping symbols such as brackets or braces. The video script discusses how to handle expressions with multiple grouping symbols by starting with the innermost group first. An example given in the script is 'six plus four in parentheses within brackets', where the inner parentheses are solved before addressing the brackets.

💡Grouping Symbols

Grouping symbols in mathematics, such as parentheses, brackets, and braces, are used to indicate that the expressions within them should be evaluated before anything outside. The video script explains that all grouping symbols take priority in the order of operations and that when multiple grouping symbols are present, the innermost group should be calculated first. The script uses examples with parentheses within brackets to illustrate this concept.

💡Simplification

Simplification in mathematics refers to the process of making a complex expression or fraction more straightforward or easier to understand. In the context of the video, simplification is shown when a fraction is reduced to its simplest form by dividing the numerator and the denominator by their greatest common factor. The script demonstrates this with the fraction 6/18, which simplifies to 1/3 after dividing both the numerator and the denominator by their greatest common factor, which is 6.

Highlights

Introduction to the concept and importance of the order of operations (PEMDAS).

Explanation of why a standardized order of operations is necessary to avoid confusion and ensure everyone arrives at the same answer.

Illustration of the impact of not following the order of operations using the example 12 - 5 * 2.

Clarification on the correct answer for the example problem using the order of operations.

Analogy used to explain the importance of a standard set of rules in operations, similar to traffic lights.

Introduction to the acronym PEMDAS and its role in remembering the order of operations.

Detailed walkthrough of solving the first example problem involving parentheses and division.

Demonstration of solving an expression with multiplication and addition, emphasizing the left-to-right rule.

Example of working through an expression with exponents and multiplication, highlighting the order of operations.

Solution to a problem with nested parentheses and multiplication, showing the step-by-step approach.

Explanation of how to handle expressions with multiple grouping symbols like parentheses, brackets, and braces.

Example problem involving fraction bars and the process of grouping the numerator and denominator before division.

Complex problem-solving demonstration with a fraction bar, including handling parentheses and exponents.

Approach to simplifying fractions when the result is a proper fraction, as shown in an example.

Handling of expressions with positive and negative integers, including division and multiplication of negative numbers.

Detailed explanation of working with negative numbers and exponents, with an emphasis on keeping track of signs.

Final summary of the complete guide to the order of operations and the importance of following these rules for accurate problem-solving.

Transcripts

Browse More Related Video

Order of Arithmetic Operations: PEMDAS

How To Evaluate Expressions With Variables Using Order of Operations

Math Antics - Order Of Operations

Algebra Basics: Solving 2-Step Equations - Math Antics

How to Use Your Scientific Calculator

Exponents Explained!

Related Tags

Math Education Order of Operations PEMDAS Rules Problem Solving Algebra Basics Math Strategies Educational Content Math Tutorial Fractions Integers