Algebra Basics: Solving 2-Step Equations - Math Antics

mathantics
23 Oct 201510:29
EducationalLearning
32 Likes 10 Comments

TLDRIn this Math Antics video, Rob teaches viewers how to solve two-step algebraic equations that involve a combination of addition/subtraction and multiplication/division operations. He emphasizes the importance of following the reverse Order of Operations to undo the steps in the correct sequence. Rob illustrates the process with examples, highlighting the impact of how equations are grouped, especially with parentheses and fraction lines, which can change the order in which operations must be undone. The video aims to simplify the process of solving more complex equations by practicing with various problems and paying attention to the grouping of terms.

Takeaways
  • 📚 The video teaches how to solve two-step equations involving one addition/subtraction and one multiplication/division operation.
  • 🔄 To solve these equations, you need to 'undo' two operations, which can be more complex due to the various combinations and the order of operations.
  • 🛠 The Order of Operations (PEMDAS/BODMAS) is crucial for knowing the sequence of operations, but when solving equations, you apply these rules in reverse.
  • 👉 The reverse Order of Operations suggests undoing addition before multiplication, as multiplication comes before addition in the standard order.
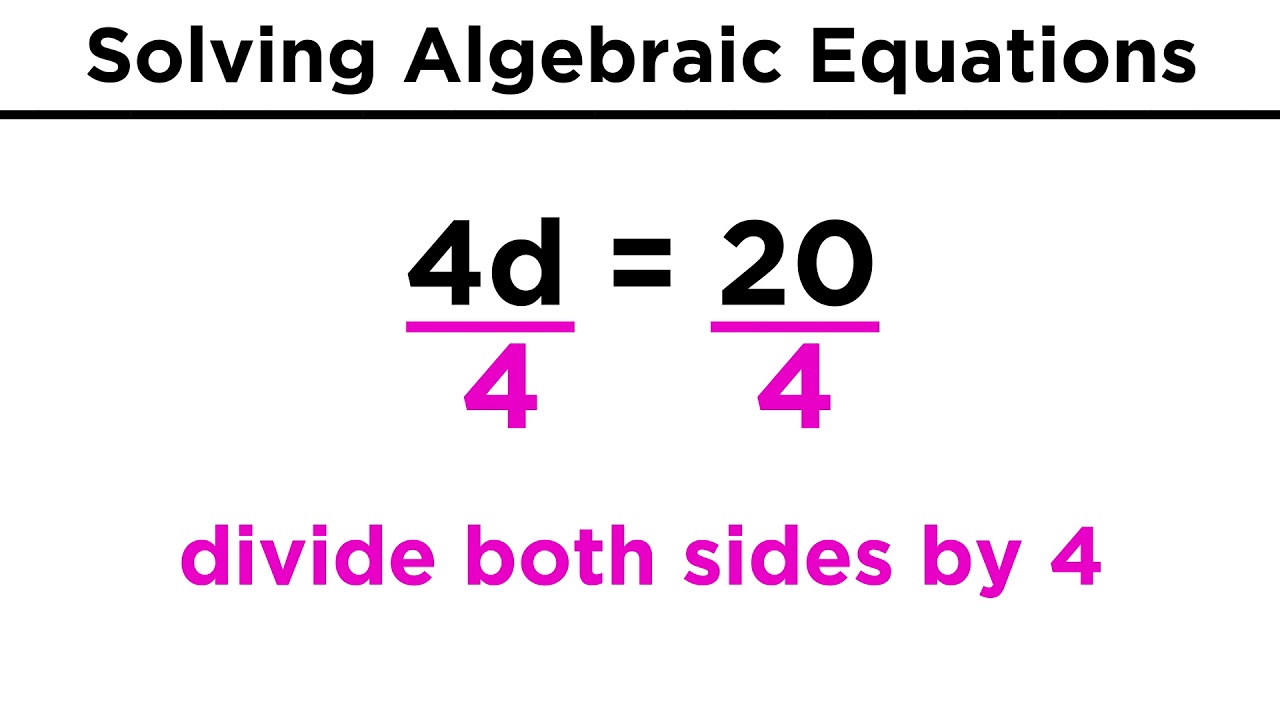
  • ⚡ Example 1: Solving '2x + 2 = 8' involves subtracting 2 from both sides first, then dividing by 2 to isolate x, resulting in x = 3.
  • 🌟 Example 2: For 'x/2 - 1 = 4', add 1 to both sides first, then multiply by 2 to solve for x, which gives x = 10.
  • 🔍 'Groups' in equations, such as those created by parentheses, dictate that operations within these groups are performed first and last when undoing.
  • 📐 The fraction line in algebraic expressions automatically creates groups for terms above and below it, which should be considered when solving.
  • 🎯 In equations with groups, like '2(x + 2) = 8', undo the outer operation last, leading to the solution x = 2.
  • 🧩 Understanding how equations are grouped can lead to different solutions, as demonstrated by the variations of 'x/2 - 1 = 4' and '(x - 1)/2 - 1 = 4'.
  • 📈 Practice is essential for solving two-step equations effectively, as there are many combinations and groupings to consider.
Q & A

  • What is the main topic of the Math Antics video?

    -The main topic of the video is solving two-step equations that involve one addition or subtraction operation and one multiplication or division operation.

  • Why are two-step equations more complicated to solve than simple equations?

    -Two-step equations are more complicated because they have more possible combinations of operations and require deciding the order in which to undo the operations.

  • What is the purpose of the Order of Operations rules when solving equations?

    -The Order of Operations rules guide the order in which operations should be undone when solving equations, helping to isolate the unknown variable.

  • How can the reverse Order of Operations help in solving two-step equations?

    -Using the reverse Order of Operations helps determine the order in which to undo the operations in a two-step equation, making it easier to isolate the unknown variable.

  • What is the first step in solving the two-step equation 2x + 2 = 8?

    -The first step is to undo the addition by subtracting 2 from both sides of the equation.

  • How does the presence of parentheses affect the order in which operations are undone in an equation?

    -Parentheses create a group, and according to the Order of Operations, operations within groups should be undone last.

  • What is an implied group in Algebra?

    -In Algebra, an implied group is a set of terms that are considered as a single unit, often found above or below a fraction line without explicit parentheses.

  • In the equation x/2 - 1 = 4, what changes when the '1' is moved above the fraction line?

    -When the '1' is moved above the fraction line, it is only subtracted from 'x' and not the entire 'x/2' term, creating a different implied group and changing the solution process.

  • What is the final answer for the equation (2)(x + 2) = 8 after following the reverse Order of Operations?

    -The final answer for the equation is x = 2, after undoing the multiplication and then the addition.

  • Why is it important to practice solving various two-step equations?

    -Practicing various two-step equations helps to understand the different combinations of operations and grouping methods, making it easier to solve more complex problems in the future.

Outlines
00:00
🔢 Introduction to Solving Two-Step Equations

Rob introduces the concept of two-step equations, which involve both addition/subtraction and multiplication/division operations. He explains that solving these equations requires reversing the Order of Operations, which typically involves performing operations inside parentheses first, followed by exponents, then multiplication and division, and finally addition and subtraction. Rob illustrates the process with a simple equation, 2x + 2 = 8, and demonstrates how to undo the operations in reverse order to isolate the variable x. He emphasizes the importance of understanding the order in which to undo operations and how grouping affects the solution process.

05:04
📚 Mastering Two-Step Equations with Groups

This paragraph delves into the complexities introduced by 'groups' in equations, such as those formed by parentheses. Rob explains that operations within groups must be undone last, according to the reverse Order of Operations. He contrasts solving an equation with and without grouping, showing how the presence of parentheses changes the solution. Rob uses the equation (x + 2) * 2 = 8 to illustrate the process and emphasizes the importance of recognizing implied groups, such as those created by a fraction line in algebraic expressions. He reinforces the strategy of undoing operations in reverse order and paying attention to how variables are grouped.

10:04
📘 Importance of Practice in Solving Two-Step Equations

In the final paragraph, Rob stresses the importance of practice in solving two-step equations due to their varied nature and the need to recognize different grouping scenarios. He encourages viewers to tackle a multitude of problems to become proficient. Rob wraps up the video with a reminder to visit www.mathantics.com for more learning resources and looks forward to the next session, ensuring that viewers have grasped the fundamentals of solving two-step equations and the significance of the reverse Order of Operations in tackling them.

Mindmap
Keywords
💡Two-step equations
Two-step equations are mathematical expressions that require two different operations to isolate the variable. In the video, these equations are central to the theme as they illustrate the complexity that arises when dealing with multiple operations. For example, the script discusses solving equations like '2x + 2 = 8' and 'x/2 - 1 = 4', which involve both addition/subtraction and multiplication/division.
💡Arithmetic operations
Arithmetic operations refer to the basic mathematical operations of addition, subtraction, multiplication, and division. The video emphasizes the importance of understanding these operations to solve two-step equations. The script mentions that equations can have a variety of combinations of these operations, which adds to the complexity of solving them.
💡Order of Operations
The Order of Operations is a set of rules that dictate the sequence in which operations in an expression should be performed. In the context of the video, the Order of Operations is crucial for both performing and undoing operations when solving equations. The script humorously points out that while the rules tell us the order to do operations, they also guide us in the reverse order to undo them.
💡Inverse operations
Inverse operations are mathematical operations that 'undo' or reverse the effect of another operation. For instance, subtraction is the inverse of addition, and division is the inverse of multiplication. The video script uses the concept of inverse operations to explain how to isolate the variable in two-step equations, such as subtracting before dividing.
💡Parentheses
Parentheses are used in mathematics to group expressions together, indicating that the operations within them should be performed first according to the Order of Operations. In the video, the script explains how parentheses change the approach to solving equations, as seen in the equation '2(x + 2) = 8', where the parentheses create a group that affects the order of operations.
💡Groups
Groups in mathematics refer to expressions or terms that are enclosed in parentheses or other brackets, or separated by a fraction line, which should be evaluated as a single unit. The video script discusses how groups change the order in which operations are undone, emphasizing the importance of dealing with groups last when solving equations.
💡Fraction line
A fraction line, also known as a vinculum, is the horizontal line in a fraction that separates the numerator from the denominator. In algebra, as mentioned in the script, the fraction line is used to automatically group the terms above and below it. This concept is highlighted in the video when solving equations involving fractions, such as '(x - 1)/2 = 4'.
💡Implied groups
Implied groups are groups of terms that are understood to be grouped together without the explicit use of parentheses or brackets. In the video, the script explains that in algebra, terms above or below a fraction line are considered implied groups. This concept is crucial when solving equations, as it affects the order in which operations are undone.
💡Multiplication and division
Multiplication and division are two of the four basic arithmetic operations. The video script discusses how these operations, when part of two-step equations, require specific strategies for solving. For example, the script explains that when solving '2x + 2 = 8', multiplication is undone before addition.
💡Addition and subtraction
Addition and subtraction are fundamental arithmetic operations that are also key to solving two-step equations. The video script uses these operations to illustrate the process of undoing operations in reverse order. For instance, in the equation 'x/2 - 1 = 4', subtraction is undone first by adding '1' to both sides.
Highlights

Introduction to solving equations with two arithmetic operations.

Explanation of why two-step equations are more complicated than single-step equations.

The importance of the Order of Operations in solving equations.

How to reverse the Order of Operations to undo operations in equations.

Solving a simple two-step equation: 2x + 2 = 8.

The concept of undoing operations in reverse order to isolate the unknown variable.

Using subtraction to undo addition in the equation 2x + 2 = 8.

Using division to undo multiplication in the equation 2x + 2 = 8.

Solving another two-step equation with division and subtraction: x/2 - 1 = 4.

Undoing subtraction before division in the equation x/2 - 1 = 4.

The role of parentheses in grouping operations in equations.

Solving an equation with parentheses to group x + 2.

The impact of grouping on the solution of an equation.

Understanding implied groups in algebraic fractions.

Solving an equation with an implied group above the fraction line.

The necessity of practicing to solve various two-step equations.

Final advice on using the reverse Order of Operations for solving equations.

Transcripts

Browse More Related Video

Algebra Basics: Solving Basic Equations Part 2 - Math Antics

Algebraic Equations and Their Solutions

Algebra Basics: Solving Basic Equations Part 1 - Math Antics

Algebraic Equations With Variables on Both Sides

a very simple algebra question , but 97% FAILED

Math Antics - Number Patterns

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
AlgebraEquationsOrder of OperationsSolving StrategiesMath EducationEducational VideoMath AnticsTwo-Step EquationsMathematicsLearning