PROBLEM SOLVING INVOLVING CIRCLES
TLDRThis educational video script introduces the concept of a circle defined as all points in a plane equidistant from a central point. It then solves three mathematical problems involving circles: finding the equation of a circle given its center and a point on the circumference, calculating the height of a semicircular tunnel with a specified width and radius, and determining the horizontal distance from the center of a Ferris wheel for a car at a certain altitude. Each problem is methodically approached using geometric formulas and the Pythagorean theorem, providing clear steps and final answers, enhancing viewers' understanding of circle-related geometry.
Takeaways
- 📚 The script introduces a series of mathematical problems involving circles.
- 🔍 It defines a circle as all points in a plane that are a fixed distance from a given point, known as the center.
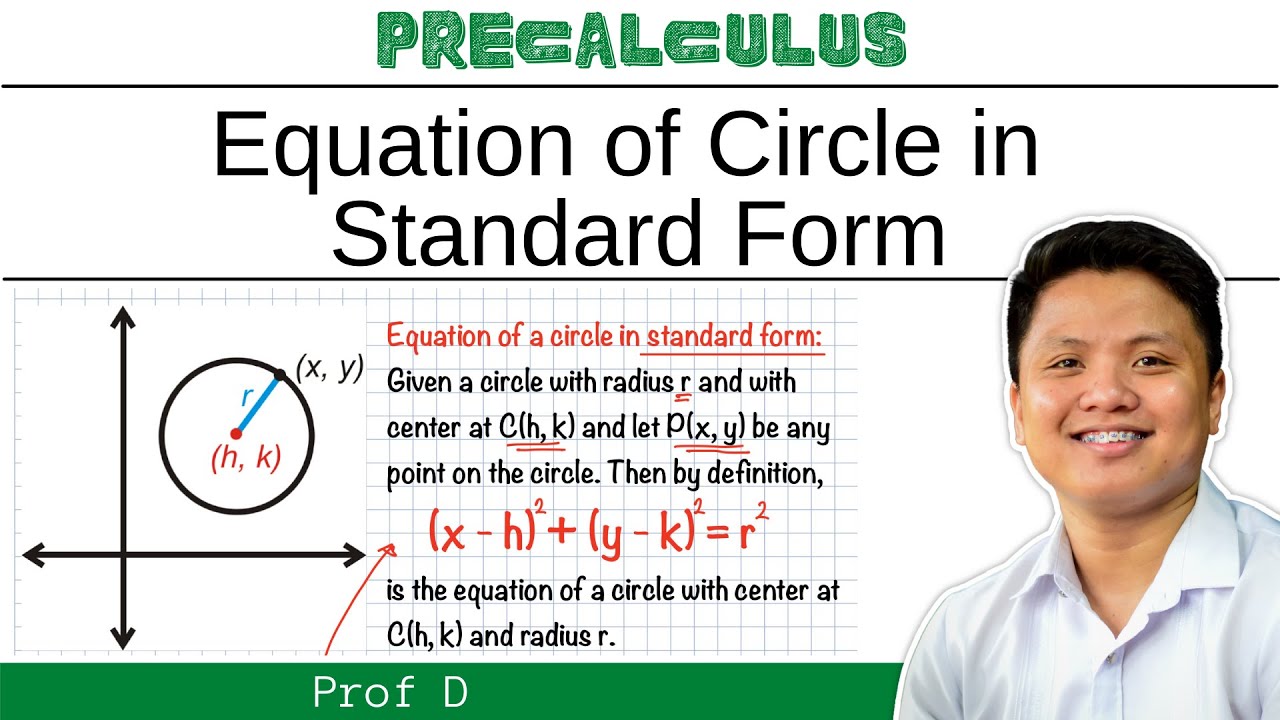
- 📐 The standard equation of a circle is given as (x - h)² + (y - k)² = r², where (h, k) is the center and r is the radius.
- 🧩 Problem 1 involves finding the equation of a circle with a given center and a point on the circle.
- 📝 The midpoint formula is used to determine the coordinates of a point on the circle in Problem 1.
- 📏 The distance formula is applied to find the radius of the circle in Problem 1.
- 🚧 Problem 2 discusses a semicircular tunnel with a specific width and radius, and calculates the height at the edge of the lane.
- 🎡 Problem 3 relates to a Ferris wheel, calculating the horizontal distance from the center when the car is at a certain altitude.
- 📈 The Pythagorean theorem is utilized in Problem 3 to find the horizontal distance from the center of the Ferris wheel.
- 📊 The script provides step-by-step solutions to the problems, including algebraic manipulation and application of geometric formulas.
- 🎓 The video aims to educate viewers on solving circle-related problems with clear explanations and mathematical reasoning.
Q & A
What is the definition of a circle according to the video?
-A circle is defined as all points in a plane that are a fixed distance from a given point in the plane. The given point is called the center, denoted as (h, k), and the fixed distance is called the radius, denoted as r.
What is the standard equation used to represent a circle?
-The standard equation of a circle is (x - h)² + (y - k)² = r², where (h, k) is the center of the circle and r is the radius.
How does the video determine the coordinates of a point on the circle in problem number one?
-The video uses the midpoint formula to find the coordinates of a point on the circle. It sets the midpoint to be the average of the x-coordinates and y-coordinates of the center and the point on the circle, respectively.
What is the equation of the circle in problem number one after substituting the values?
-The equation of the circle in problem number one is (x - 5)² + (y + 2)² = 232.
In problem number two, how is the height of the tunnel at the edge of each lane calculated?
-The height of the tunnel at the edge of each lane is calculated using the Pythagorean theorem, where the radius of the semicircle is 9 feet and the width of the lane is 5 feet.
What is the final height of the tunnel at the edge of each lane in problem number two?
-The final height of the tunnel at the edge of each lane is 7.48 feet.
What is the radius of the Ferris wheel in problem number three?
-The radius of the Ferris wheel in problem number three is 15 meters, which is half of the diameter (30 meters).
How is the horizontal distance of the car from the center of the Ferris wheel calculated in problem number three?
-The horizontal distance is calculated using the Pythagorean theorem, where the vertical distance from the center is 8 meters (24 meters altitude minus 15 meters radius) and the radius is 15 meters.
What is the final horizontal distance of the car from the center of the Ferris wheel when it is at an altitude of 24 meters?
-The final horizontal distance of the car from the center of the Ferris wheel is 12.69 meters.
What is the purpose of the video script presented?
-The purpose of the video script is to explain and solve mathematical problems involving circles, including finding the equation of a circle and calculating distances and heights related to circular shapes.
Outlines
📚 Introduction to Circle Problems
The video script begins with an introduction to problems involving circles. The presenter defines a circle as all points in a plane that are at a fixed distance from a given point, known as the center, with the fixed distance being the radius. The first problem presented is to find the equation of a circle with a given center at (5, -2) and a point on the circle at (-2, 1). Using the midpoint formula and the distance formula, the presenter calculates the radius and then derives the equation of the circle as \((x - 5)^2 + (y + 2)^2 = 232\).
🏗️ Calculating the Height of a Semi-circular Tunnel
In the second paragraph, the script discusses a problem related to a semi-circular tunnel with a radius of 9 feet and a 10-foot-wide lane running through it. The presenter calculates the height of the tunnel at the edge of the lane by setting up the equation \(x^2 + y^2 = R^2\) with \(R = 9\) feet. After dividing the lane width by 2 to find the x-coordinate, the presenter solves for y to find the height of the tunnel, which is determined to be 7.48 feet at each edge.
🎡 Determining the Horizontal Distance on a Ferris Wheel
The final paragraph of the script addresses a problem involving a Ferris wheel. Given that the wheel is 1 meter above the ground at its lowest point and 31 meters above the ground at its highest point, the presenter calculates the diameter and radius of the wheel. Using the Pythagorean theorem, the presenter determines the horizontal distance from the center to a car at an altitude of 24 meters, which is found to be 12.69 meters. The video concludes with a thank you message and a hope that viewers have learned from the content.
Mindmap
Keywords
💡Circle
💡Center (h, k)
💡Radius (R)
💡Equation of a Circle
💡Midpoint Formula
💡Distance Formula
💡Semicircular Tunnel
💡Ferris Wheel
💡Pythagorean Theorem
💡Altitude
💡Diameter
Highlights
Group 9 presents exercises on problems involving circles.
A circle is defined as all points in a plane a fixed distance from a given point, the center.
The standard equation of a circle is (x-h)² + (y-k)² = r², where (h,k) is the center and r is the radius.
Problem 1 involves finding the equation of a circle with a given center and a point on the circle.
The midpoint formula is used to find the coordinates of a point on the circle.
The distance formula is applied to calculate the radius of the circle.
The final equation of the circle is derived using the standard form and the calculated radius.
Problem 2 discusses a semicircular tunnel with a specific width and radius.
The height of the tunnel at the edge of a lane is calculated using the radius and the width of the lane.
The Pythagorean theorem is utilized to find the height of the tunnel.
The final height of the tunnel at each edge is given as 7.48 feet.
Problem 3 examines a Ferris wheel with a car at different altitudes above the ground.
The diameter and radius of the Ferris wheel are determined from the given altitudes.
The vertical distance from the center at a specific altitude is calculated.
The Pythagorean theorem is again used to find the horizontal distance from the center at a given altitude.
The final horizontal distance from the center when the car is at an altitude of 24 meters is 12.69 meters.
The video concludes with a summary of the problems and an invitation to learn more.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: