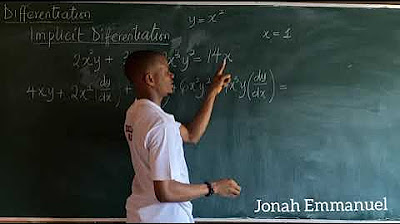
How to use implicit differentiation on an equation for dy dx

Brian McLogan
5 Nov 201603:51
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the process of differentiation with respect to the variable X in the context of equations involving X and Y. The presenter explains the steps of taking the derivative of both sides of an equation, emphasizing that Y is the dependent variable. The equation given involves terms like Y cubed, Y squared, and linear terms in X and Y. The derivative of each term is calculated, and the terms are then rearranged to isolate the derivative of Y with respect to X on one side. The presenter factors out the derivative term, dy/dx, resulting in a simplified equation where dy/dx is equal to 2x divided by an expression involving Y. This summary captures the essence of the differentiation process as presented in the script, providing a clear and concise explanation that is likely to engage viewers interested in calculus.

Takeaways
  • 📚 The process involves differentiating both sides of an equation with respect to X, focusing on equations involving X and Y variables.
  • 🔑 The independent variable is X, and the dependent variable is Y.
  • 🧮 The differentiation involves taking the derivative of each term on both sides of the equation.
  • ⚖️ The differentiation process requires understanding when variables match up and when they do not with respect to X.
  • 🔍 Terms that do not match up with respect to X are differentiated normally, such as 3y^2 and 2y.
  • 📉 The term -5y matches up with respect to X, so its derivative is simply -5.
  • 🧷 The derivative of x^2 with respect to X is 2x.
  • 🔗 After differentiating, terms involving dy/dx are collected on one side of the equation.
  • 📝 The remaining terms are placed on the other side of the equation, and 2x is added to this side.
  • 📐 Factor out dy/dx from the terms on the left side, as it is a common factor.
  • 🟡 The resulting equation is 3y^2 + 2y - 5 = 2x, which is derived from the product rule.
  • ✅ To solve for dy/dx, the equation is rearranged so that dy/dx is isolated, resulting in dy/dx = 2x / (3y^2 + 2y - 5).
Q & A

  • What is the main topic of the transcript?

    -The main topic of the transcript is the process of differentiating equations with respect to X, involving variables X and Y.

  • What does the term 'independent variable' refer to in the context of this transcript?

    -In the context of this transcript, the independent variable is X, which is the variable with respect to which the differentiation is being performed.

  • What does the term 'dependent variable' refer to in the context of this transcript?

    -In the context of this transcript, the dependent variable is Y, which is the variable that changes in response to changes in X.

  • What is the initial step in differentiating the given equation?

    -The initial step is to take the derivative of both sides of the equation with respect to X, which involves applying the derivative operator D/DX to each term.

  • What is the purpose of taking the derivative of the equation?

    -The purpose of taking the derivative is to find the rate of change of the dependent variable Y with respect to the independent variable X.

  • What does it mean when variables do not match up with respect to X in the derivative?

    -When variables do not match up with respect to X, it means that the derivative of the term does not involve the variable X, and thus, the term remains as it is without the derivative operator applied to it.

  • What is the next step after taking the derivative of the equation?

    -The next step is to collect all terms involving the derivative dy/dx on one side of the equation, typically the left side, and all other terms on the opposite side.

  • Why is it necessary to factor out dy/dx from the equation?

    -Factoring out dy/dx is necessary to isolate the derivative and solve for it, which represents the rate of change of Y with respect to X.

  • What is the final expression for the derivative of Y with respect to X, as given in the transcript?

    -The final expression for the derivative of Y with respect to X is dy/dx = 2x / (3y^2 + 2y - 5).

  • What does the term 'dy/dx' represent in the context of the transcript?

    -In the context of the transcript, 'dy/dx' represents the derivative of Y with respect to X, which is the rate at which Y changes as X changes.

  • What is the final step in the process described in the transcript?

    -The final step is to solve for dy/dx by dividing both sides of the equation by the expression in the denominator, which results in the derivative of Y with respect to X.

  • What could be a potential source of confusion in the transcript?

    -A potential source of confusion could be the moment when the transcript mentions '3 y^2' being lost in the process, indicating a possible error or oversight in the step-by-step explanation.

Outlines
00:00
🧮 Derivative Calculation and Solving for dy/dx

The paragraph explains a process of differentiation with respect to X in a mathematical context. It begins with a discussion on differentiating both sides of an equation involving X and y, where y is the dependent variable. The differentiation involves taking the derivative of terms like y cubed, y squared, and linear terms with respect to X. The paragraph then addresses the need to match up variables with respect to X and to take the derivative accordingly. It proceeds to show the steps of collecting terms involving dy/dx on one side and other terms on the opposite side, followed by factoring out dy/dx to isolate it. Finally, it demonstrates how to solve for dy/dx by dividing both sides of the equation by the expression obtained after factoring out dy/dx. The paragraph ends with an invitation for questions, indicating an interactive educational setting.

Mindmap
Keywords
💡Differentiate
Differentiate refers to the process of finding the derivative of a function with respect to a variable, which is a fundamental concept in calculus. In the video, the speaker is discussing how to differentiate both sides of an equation with respect to 'x', which is a key step in solving differential equations. An example from the script is 'taking D over DX of both sides'.
💡Equations
Equations are mathematical statements that assert the equality of two expressions. In the context of the video, equations are used to represent relationships between variables 'x' and 'y'. The script discusses dealing with equations that involve 'x' and 'y', which are central to the theme of solving for derivatives.
💡Independent and Dependent Variables
In mathematics, an independent variable is one that can be freely manipulated, while a dependent variable is determined by the independent variable. In the video, 'x' is referred to as the independent variable, and 'y' is the dependent variable. This distinction is crucial for understanding how to apply differentiation to find the rate of change of 'y' with respect to 'x'.
💡Derivative
A derivative in calculus represents the rate at which a function changes with respect to its variable. The video focuses on taking derivatives, which is shown when the speaker says 'still take the derivative'. Derivatives are essential for understanding how one quantity changes in relation to another.
💡Sum
In mathematics, a sum refers to the result of adding two or more numbers or expressions. The script mentions 'our sum', which likely refers to a summation of terms within an equation. This concept is important when differentiating terms within an equation, as each term's derivative must be considered.
💡Product Rule
The product rule is a fundamental theorem used to find the derivatives of products of two functions. Although not explicitly named in the script, the concept is implied when the speaker discusses factoring out 'dy/dx' from an equation. The product rule would be used to differentiate a product of 'y' and 'x' in the given context.
💡Factoring
Factoring is the process of breaking down a polynomial or expression into a product of its factors. In the video, the speaker factors out 'dy/dx' to simplify the equation, as shown in 'factor out the dy/dx'. This step is crucial for isolating the derivative and solving for 'y' with respect to 'x'.
💡Solving for dy/dx
Solving for dy/dx means finding the derivative of 'y' with respect to 'x', which is the main goal of the video. The script illustrates this process when the speaker isolates 'dy/dx' on one side of the equation to find its value. This is a common technique in differential calculus.
💡Rate of Change
The rate of change is a measure of how one quantity changes in relation to another. In the context of the video, finding the derivative represents determining the rate at which 'y' changes with respect to 'x'. This concept is central to the theme of the video, as it is the underlying purpose of differentiating the given equation.
💡Variables
Variables are symbols used to represent values in mathematical expressions or equations. In the video, 'x' and 'y' are the primary variables, with 'x' being independent and 'y' being dependent. The script discusses how these variables relate to each other through differentiation.
💡Contextual Understanding
Contextual understanding refers to comprehending the meaning of terms or concepts within a specific situation or narrative. In the video, the speaker ensures that the audience understands how each mathematical concept applies to the process of differentiating an equation. This is important for grasping the overall message and applying the knowledge practically.
Highlights

Differentiating both sides of an equation with respect to X involves dealing with X and y variables.

The independent variable is X, while the dependent variable is Y.

Taking the derivative of both sides of the equation using D/DX.

The sum rule is applied when differentiating Y cubed plus Y squared minus 5Y minus X squared.

The derivative of a constant is zero, as seen with the derivative of four being zero.

Variables do not match up with respect to X of Y, which requires taking the derivative of each term separately.

The derivative of Y with respect to X is represented as Dy/DX.

Terms with matching variables are collected on one side of the equation.

The process involves adding 2X to the other side of the equation.

Factoring out Dy/DX from the equation is a key step in solving it.

The resulting equation after factoring is 3y^2 + 2y - 5 = 2X.

The goal is to solve for the derivative of Y with respect to X.

To solve for Dy/DX, the equation is divided by the factored term on both sides.

The final expression for the derivative of Y with respect to X is Dy/DX = 2X / (3y^2 + 2y - 5).

The process requires understanding when variables match up and when they do not with respect to X.

The derivative of each term is taken separately if the variables do not match up.

The derivative of a term without a variable is simply taken as is.

The final solution involves isolating Dy/DX on one side of the equation.

The method demonstrates the application of differentiation rules in solving equations.

The transcript provides a step-by-step guide to differentiating equations involving X and Y variables.

Transcripts

Browse More Related Video

Implicit Differentiation for Calculus - More Examples, #1

Implicit Differentiation

Implicit Differentiation Method

Second derivatives (implicit equations): find expression | AP Calculus AB | Khan Academy

Implicit Differentiation for Calculus - More Examples #2

Math 11 - Section 3.8

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusDifferentiationDependent VariableIndependent VariableMathematicsDerivativesEducational ContentProblem SolvingX and Y VariablesMathematical AnalysisLearning Resource