The ARCTIC CIRCLE THEOREM or Why do physicists play dominoes?
TLDRThe Mathologer's 2020 Christmas video explores the fascinating world of domino tilings, starting with the classic mutilated chessboard puzzle and delving into the Arctic Circle Theorem. It explains how physicists use tilings as models for natural phenomena and introduces Kasteleyn's formula for calculating the number of tilings on rectangular boards. The video also uncovers the magical square dance for generating random tilings of Aztec diamonds and their simple yet mysterious tiling formula, concluding with a mini master class on determinants and their role in counting tilings.
Takeaways
- 😲 The video introduces the concept of domino tiling puzzles and their significance in mathematics and physics.
- 🧩 It discusses the 'mutilated chessboard puzzle', explaining why a chessboard with two opposite corners removed cannot be tiled with dominoes.
- 🔍 The script highlights a parity argument showing that if the number of black and green squares on a chessboard is unequal, it cannot be tiled with dominoes.
- 🎯 The video presents a method to tile a chessboard by removing one black and one green square, proving that any such board can always be tiled.
- 📚 The script explains the importance of domino tilings in physics, as a model for certain natural phenomena, like atoms forming molecules.
- 📉 It introduces Kasteleyn's formula for calculating the number of tilings of a rectangular board, which involves complex numbers and trigonometric functions.
- 🔢 The video demonstrates how to use the determinant of a matrix to calculate the number of tilings for certain 'mutilated' chessboards.
- 💃 The 'magic square dance' is introduced as a method to grow tilings of Aztec diamonds from a small starter diamond, providing a visual and intuitive understanding of the process.
- ❄️ The 'arctic circle theorem' is explained, which describes the appearance of a circular pattern in random tilings of large Aztec diamond boards.
- 🔮 The video explores the number of tilings of Aztec diamonds with a surprisingly simple formula, contrasting with the complexity of Kasteleyn's formula for rectangular boards.
- 🎁 The script concludes with a puzzle about tiling hexagonal boards with dominoes shaped to cover two equilateral triangles, hinting at a 3D interpretation of these tilings.
Q & A
What is the main theme of the 2020 Mathologer Christmas video?
-The main theme of the video is exploring the love of physicists and mathematicians for domino tilings, with a special focus on the 'arctic circle theorem' and the 'mutilated chessboard puzzle'.
What is the 'mutilated chessboard puzzle'?
-The 'mutilated chessboard puzzle' involves removing two opposite corners from a standard chessboard and attempting to tile the remaining board with dominoes that cover two squares each.
Why can't a mutilated chessboard with two opposite corners removed be tiled with dominoes?
-A mutilated chessboard with two opposite corners removed cannot be tiled because doing so would leave an unequal number of black and green squares, which is impossible since each domino must cover one of each color.
What is the significance of the parity argument in the context of the mutilated chessboard puzzle?
-The parity argument shows that any chessboard, whether standard or mutilated, can only be tiled with dominoes if there is an equal number of black and green squares. This is significant because it provides a simple and powerful method to determine whether a board can be tiled without attempting all possible configurations.
How does the video demonstrate that a chessboard with one black and one green square removed can always be tiled?
-The video shows that by creating a round trip path that visits every square of the chessboard exactly once and removing the two squares, the remaining path can be divided into two parts that can be tiled with dominoes along their lengths.
What is the connection between domino tilings and physics mentioned in the video?
-Domino tilings are connected to physics as they serve as a simple model for certain natural phenomena. The squares of a grid can be thought of as atoms that form two-atom molecules (dominoes), which then tile the grid in a random pattern, potentially revealing interesting structures.
Who published the formula for the number of tilings of a rectangular board, and what is its significance?
-Piet Kasteleyn published the formula for the number of tilings of a rectangular board in 1961. This formula is significant because it provides a mathematical way to calculate the number of possible domino tilings for any given rectangular board.
What is the 'Aztec diamond' and how does it relate to the arctic circle theorem?
-The 'Aztec diamond' refers to a series of chessboard-like figures obtained by cutting the corners of square boards with even side lengths. The arctic circle theorem is related to these figures as it describes the phenomenon where random tilings of large Aztec diamonds feature a roughly circular chaotic region in the middle, known as the 'arctic circle'.
What is the 'magic square dance' mentioned in the video, and how does it help in understanding the number of tilings of Aztec diamonds?
-The 'magic square dance' is a method for growing all tilings of all Aztec diamonds from a starter diamond by using a series of moves that involve sliding and filling in two-by-two squares. This method helps in understanding the number of tilings of Aztec diamonds by providing a systematic way to generate and count all possible tilings.
How does the video explain the surprisingly simple formula for the number of tilings of Aztec diamonds?
-The video explains the simple formula for the number of tilings of Aztec diamonds by using the concept of induction and the 'magic square dance'. It starts with the smallest Aztec diamond, shows that it has two tilings, and then demonstrates how each subsequent diamond's tilings can be derived from the previous one, leading to the general formula of 2^(n*(n+1)/2).
What is the determinant trick mentioned in the video, and how does it apply to the tiling of mutilated chessboards?
-The determinant trick is a mathematical method used to calculate the number of tilings of a mutilated chessboard. By creating a matrix that represents the possible pairings of squares on the board and calculating its determinant, one can find the number of ways the board can be tiled with dominoes. The trick applies to boards where the determinant formula yields a real integer result.
Outlines
🎄 Introduction to the Christmas Mathologer Video
The script opens with a warm welcome to the 2020 Mathologer Christmas video, acknowledging the unusual year and promising a fittingly eccentric video to close it. The host introduces the theme of dominoes, a favorite among physicists and mathematicians, and teases an enigmatic 'Arctic Circle Theorem'. The video kicks off with the classic 'mutilated chessboard' puzzle, challenging viewers to tile a chessboard with two opposite corners removed using dominoes. After several unsuccessful attempts, the host reveals a common pattern: an unmatchable pair of black squares, leading to a discussion on the impossibility of tiling due to an unequal number of black and green squares.
🧩 Domino Tiling and the Mutilated Chessboard Puzzle
This paragraph delves deeper into the logic behind the impossibility of tiling a mutilated chessboard with an odd number of squares of one color. The host explains that a full chessboard can be easily tiled because it has an equal number of black and green squares. However, removing two opposite corners disrupts this balance, leaving an unmatchable pair of black squares. The script also explores the scenario of removing one black and one green square, proving that such a board can always be tiled. The host introduces a 'round trip' method to visualize this tiling process, emphasizing the simplicity and elegance of the argument.
🔢 The Mathematics of Domino Tiling
The script transitions to the mathematical significance of domino tiling, likening the grid's squares to atoms and dominoes to molecules, forming a model for natural phenomena. It introduces the formula for calculating the number of tilings of a rectangular board, first published by physicist Piet Kasteleyn in 1961. The host guides viewers through the application of Kasteleyn's formula using an 8x8 chessboard as an example, highlighting the surprising involvement of trigonometric functions and the cancellation of irrational numbers to yield an integer result, which represents the number of possible tilings.
📚 Advanced Domino Tiling Concepts
This paragraph introduces more advanced concepts in domino tiling, such as the determinant method for calculating the number of tilings of any mutilated chessboard. The host demonstrates how to create a matrix representing the neighboring relationships between squares and how to calculate the determinant to find the number of tilings. The explanation includes a step-by-step guide on how to label the board, create the matrix, and interpret the result, even for boards with complex shapes.
🌐 The Arctic Circle Theorem and Aztec Diamonds
The script shifts focus to the Arctic Circle Theorem and Aztec diamond boards, which are square boards with corner squares removed. The host discusses the emergence of 'frozen regions' in the corners of large Aztec diamonds when tiled at random, and the central 'chaotic region' that resembles an arctic circle. The video showcases animations of random tilings of Aztec diamonds, illustrating the theorem's effects and the prevalence of circular patterns in nature.
🎲 Random Tiling and the Growth of Aztec Diamonds
This paragraph explores the method of generating random tilings of Aztec diamonds through a process described as a 'magic square dance'. The host explains how to grow tilings from the smallest 2x2 board to larger diamonds by sliding and filling in 2x2 sections with dominoes. The script emphasizes the efficiency and elegance of this method, which can be used to create random tilings and understand the distribution of tilings for large Aztec diamonds.
📉 The Formula for Aztec Diamond Tilings
The host presents the surprisingly simple formula for calculating the number of tilings of Aztec diamonds, which is a sum of powers of two. The explanation uses mathematical induction to prove the formula, starting with the base case of the smallest Aztec diamond and extending the argument to larger diamonds. The script provides a clear and logical progression of the proof, highlighting the elegance of the mathematical reasoning.
❄️ The Arctic Circle Phenomenon
This paragraph delves into the 'arctic circle' phenomenon observed in random tilings of large Aztec diamonds, where a circular pattern emerges in the center. The host uses the 'magic square dance' to argue that the regularly tiled regions in the corners, or 'frozen regions', are almost certain to appear in most tilings. The script provides a detailed explanation of why these patterns occur and how they relate to the overall structure of the tiling.
🔺 Hexagonal Boards and Domino Tiling
The script introduces a new type of board subdivided into equilateral triangles and a corresponding type of domino that covers two triangles. The host discusses the determinant formula for calculating the number of tilings for these boards and presents the concept of 'arctic circles' on hexagonal boards. The video includes a puzzle for viewers to ponder regarding the equal numbers of differently oriented tiles in any tiling of a regular hexagon board.
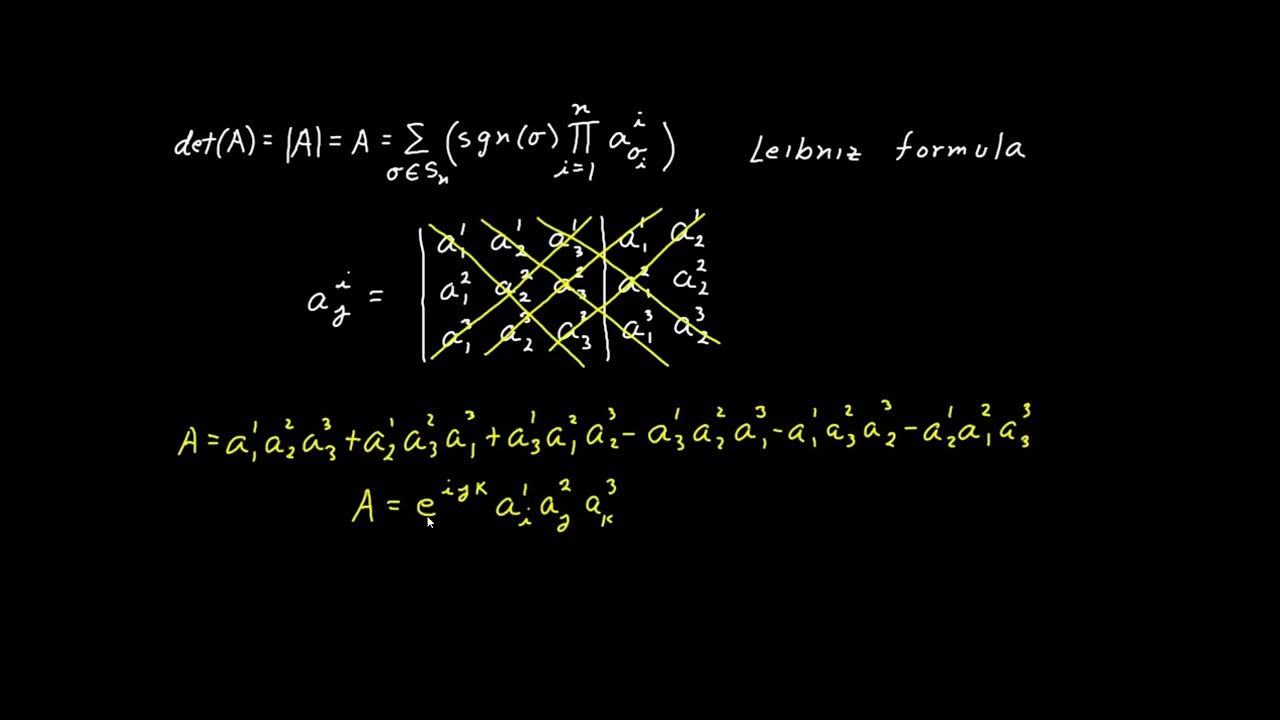
🏆 Mini Master Class on Determinants
In this final paragraph, the host offers a 'mini master class' for those interested in a deeper understanding of determinants and their role in calculating the number of tilings. The explanation covers the basics of 2x2 and 3x3 determinants, the significance of permutations, and how the determinant formula can be applied to mutilated boards. The script provides a step-by-step guide to understanding the determinant's mechanics and its relevance to the problem of domino tiling.
📝 Conclusion and Determinant Trick Derivation
The script concludes with a summary of the determinant trick's application to Kasteleyn's formula and a note on the broader implications of the mathematical concepts discussed. The host encourages feedback on the video's content and expresses a desire for a break after the year's events. There's also a mention of an article by Jim Propp for those interested in the derivation of Kasteleyn's formula using determinants, offering a deeper dive into the mathematical intricacies.
Mindmap
Keywords
💡Dominoes
💡Mutilated Chessboard Puzzle
💡Tiling
💡Parity Argument
💡Kasteleyn's Formula
💡Determinant
💡Aztec Diamond
💡Arctic Circle Theorem
💡Iterated Shuffling
💡Eigenvalues
💡Frozen Regions
Highlights
Introduction to the concept of domino tiling and its appeal to physicists and mathematicians.
The arctic circle theorem is introduced as a mysterious and intriguing topic.
The mutilated chessboard puzzle is presented, sparking interest in tiling problems.
Demonstration of failed attempts to tile a mutilated chessboard, leading to an important observation.
The parity argument is explained, showing why a mutilated chessboard cannot be tiled when black and green squares differ.
A surprising result: any chessboard with an equal number of black and green squares can be tiled, even if it's mutilated.
The concept of domino tilings as a model for natural phenomena in physics is introduced.
Kasteleyn's formula for the number of tilings of a rectangular board is presented, highlighting its complexity and significance.
An explanation of how to use the determinant of a matrix to calculate the number of tilings of a mutilated board.
The challenge for viewers to calculate the number of tilings for small boards and to spot patterns in the results.
The exploration of random tilings of Aztec diamond boards and the emergence of 'frozen regions' and the 'arctic circle'.
The simple and beautiful formula for the number of tilings of Aztec diamonds is introduced.
The 'magic square dance' method for generating all tilings of Aztec diamonds is explained.
The proof of the formula for the number of tilings of Aztec diamonds using induction and the magic square dance.
An explanation of the 'arctic circle theorem' and its implications for the shape of random tilings.
The extension of tiling concepts to boards subdivided into equilateral triangles, introducing a new type of 'arctic circle'.
Final challenge to the viewers involving equal numbers of differently oriented tiles in hexagonal boards.
A mini master class explaining the mathematical reasoning behind the determinant trick for counting tilings.
A summary of the key points and a call to action for feedback on the video content.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: