Physics 15 Torque Fundamentals (8 of 13) How to Calculate a Torque (Basic Example 2)

Michel van Biezen
5 May 201603:07
EducationalLearning
32 Likes 10 Comments

TLDRThe video script presents a classic physics problem involving the calculation of torque. It features a beam, 2 meters in length, inclined at a 60-degree angle to the horizontal, with a 10-kilogram mass hanging from it via a cable. The force exerted by the mass, equivalent to its weight (mass times gravitational acceleration, G), acts along the line of the beam. The torque is determined by multiplying this force by the perpendicular distance from the line of action of the force to the pivot point, which in this case is the length of the beam times the cosine of the angle. The resulting torque is calculated to be 98 Newton meters, with a clockwise direction, denoted as negative in vector notation. The problem is solved using trigonometric principles and provides a clear understanding of how torque is calculated and its directional implications.

Takeaways
  • πŸ“ The problem involves calculating the torque caused by a mass hanging from a cable attached to a beam.
  • πŸ“ The beam is 2 meters long and makes a 60-degree angle with the horizontal.
  • πŸ“š The mass of the object is 10 kilograms, and the force acting on the beam is equal to the weight of the object (mass times gravity).
  • πŸ”΅ The force's line of action can be extended to find the perpendicular distance from the force to the pivot point, which is key for torque calculation.
  • πŸ“ Torque is calculated as the force times the perpendicular distance from the force's line of action to the pivot point.
  • πŸ“‰ The adjacent side to the 60-degree angle in the triangle formed by the beam, the force, and the ground represents this perpendicular distance.
  • πŸ“Œ The formula for torque in this context is the weight of the object (mass times gravity) times the length of the beam times the cosine of the angle.
  • πŸ”’ Plugging in the values, the torque is calculated as 10 kg Γ— 9.8 m/sΒ² Γ— 2 m Γ— cos(60Β°), which equals 98 Newton meters.
  • ➑️ The direction of the torque is clockwise, which is considered negative in this context.
  • βš™οΈ The magnitude of the torque is 98 Newton meters, and when considering direction, it is represented as a vector pointing into the negative Z direction.
  • πŸ“ Torque can be expressed both as a scalar (magnitude only) or as a vector quantity (magnitude and direction).
Q & A

  • What is the mass of the object hanging from the cable?

    -The mass of the object is 10 kilograms.

  • What is the length of the beam?

    -The length of the beam is 2 meters.

  • What is the angle the beam makes with the horizontal?

    -The beam makes an angle of 60 degrees with the horizontal.

  • What is the force acting on the beam due to the hanging object?

    -The force acting on the beam is the weight of the object, which is the mass times the acceleration due to gravity (10 kg * 9.8 m/s^2).

  • What is the formula for torque in this context?

    -The torque is equal to the force (weight of the object) times the perpendicular distance from the line of action of the force to the pivot point.

  • How is the perpendicular distance calculated in this scenario?

    -The perpendicular distance is calculated as the length of the beam (L) times the cosine of the angle (cosine of 60 degrees).

  • What is the value of the torque calculated in the script?

    -The torque is calculated to be 98 Newton meters.

  • In which direction would the beam rotate if it was subjected to the force alone?

    -The beam would rotate in a clockwise direction.

  • What is the term used to describe a clockwise torque?

    -A clockwise torque is also known as a negative torque.

  • How can the direction of the torque be represented in vector notation?

    -The direction of the torque can be represented as a vector quantity with a negative sign indicating the direction into the board, which is the negative Z direction.

  • What is the acceleration due to gravity (G) used in the calculation?

    -The acceleration due to gravity (G) used in the calculation is 9.8 m/s^2.

  • What is the significance of considering the force's line of action in calculating torque?

    -Considering the force's line of action is significant because it helps determine the perpendicular distance from the force to the pivot point, which is essential for calculating the torque.

Outlines
00:00
πŸ” Calculating Torque on a Hanging Mass

The video introduces a classic physics problem involving a beam with a hanging object, aiming to calculate the torque exerted by the object's weight. The object has a mass of 10 kilograms, and the beam is 2 meters long, making a 60-degree angle with the horizontal. The weight of the object is used as the force, and a right triangle is formed to determine the perpendicular distance from the force's line of action to the pivot point. The torque formula is presented as the force (weight of the object) times the perpendicular distance (L times cosine of the angle). After substituting the given values, the torque is calculated to be 98 Newton meters. The direction of the torque is determined to be clockwise, which is considered negative in vector notation, and is represented as -98 Newton meters towards the negative Z direction.

Mindmap
Keywords
πŸ’‘Torque
Torque is the rotational equivalent of linear force. It is a measure of the force that can cause an object to rotate around an axis and is calculated as the product of the force applied and the perpendicular distance from the axis of rotation to the line of action of the force. In the video, torque is central to understanding the effect of the hanging mass on the beam, with the formula for torque being force times the length of the beam times the cosine of the angle (60 degrees in this case).
πŸ’‘Beam
A beam is a horizontal or angled structural element that primarily resists loads applied along its length by bending. In the video, the beam is the structure from which the object is hanging, creating a torque that the video aims to calculate. The beam's length and the angle it makes with the horizontal are critical parameters in the torque calculation.
πŸ’‘Mass
Mass is a measure of the amount of matter in an object, typically measured in kilograms. In the context of the video, the mass of the hanging object (10 kilograms) is a key factor in determining the weight of the object, which in turn affects the torque exerted on the beam.
πŸ’‘Weight
Weight is the force exerted on an object due to gravity and is calculated as the mass of the object multiplied by the acceleration due to gravity (G). In the video, the weight of the 10-kilogram object is found by multiplying its mass by 9.8 m/s^2, which is the standard acceleration due to gravity on Earth.
πŸ’‘Angle
An angle is the space between two lines or planes that intersect. In the video, the angle of 60 degrees made by the beam with the horizontal is a crucial component in the torque calculation, as it determines the cosine value used in the formula.
πŸ’‘Pivot Point
The pivot point is the point around which a body rotates orζ‰­εŠ¨ (twists). In the video, the pivot point is the axis about which the torque is being calculated. The perpendicular distance from the line of action of the force to the pivot point is a key factor in determining the torque.
πŸ’‘Force
Force is any interaction that causes a change in the motion of an object. In the video, the force is the weight of the hanging object, which is the product of its mass and the acceleration due to gravity. This force acts on the beam and is responsible for creating the torque that the video aims to calculate.
πŸ’‘Perpendicular Distance
Perpendicular distance is the shortest distance from a point to a line in a plane, measured at a right angle to the line. In the context of the video, the perpendicular distance is the length of the beam times the cosine of the angle, which is used to calculate the torque.
πŸ’‘Cosine
Cosine is a trigonometric function that describes the ratio of the adjacent side to the hypotenuse in a right-angled triangle. In the video, the cosine of the 60-degree angle is used to find the perpendicular distance from the force's line of action to the pivot point, which is necessary for calculating the torque.
πŸ’‘Newton
A Newton is the International System of Units (SI) derived unit of force. It is defined as the force needed to accelerate a one-kilogram mass by one meter per second squared. In the video, the weight of the object is expressed in Newtons, which is then used to calculate the torque in Newton meters.
πŸ’‘Direction of Torque
The direction of torque indicates the axis about which the torque tends to cause rotation. In the video, the torque is described as being clockwise, which is also referred to as a negative torque. This is important for understanding the rotational effect of the force on the beam.
πŸ’‘Vector Quantity
A vector quantity is a quantity that has both magnitude and direction. In the video, the torque is described not only by its magnitude (98 Newton meters) but also by its direction (clockwise or negative Z direction), making it a vector quantity.
Highlights

The example involves calculating the torque caused by a mass hanging from a cable attached to a beam.

The mass of the object is 10 kilograms.

The length of the beam is 2 meters.

The beam makes an angle of 60 degrees with the horizontal.

The force acting on the beam is equal to the weight of the object times gravitational acceleration (G).

A triangle is formed, allowing for the use of trigonometry to find the torque.

The line of action of the force can be extended to find the perpendicular distance to the pivot point.

The torque is calculated as the force times the perpendicular distance from the line of action of the force to the pivot point.

The hypotenuse of the triangle is the length of the beam (L), and the adjacent side is the perpendicular distance (D).

The torque formula is derived as the weight of the object times G, times the length of the beam, times the cosine of the angle.

The numerical values used in the calculation are mass (10 kg), gravitational acceleration (9.8 m/s^2), and length of the beam (2 m).

The cosine of 60 degrees is used in the calculation, which equals 1/2.

The calculated torque is 98 Newton meters.

The direction of the torque is clockwise, also known as a negative torque.

Clockwise torque implies a rotation in the clockwise direction.

The torque can be represented in vector notation as a negative 98 Newton meters in the Z direction.

The magnitude and direction of the torque are both important for a complete understanding of the effect on the beam.

Transcripts

Browse More Related Video

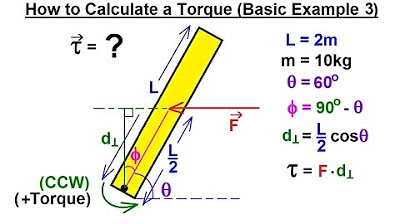
Physics 15 Torque Fundamentals (9 of 13) How to Calculate a Torque (Basic Example 3)

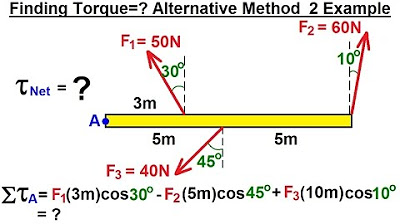
Physics 15 Torque (9 of 27) Alternative Method 1 & 2: Example Torque=?

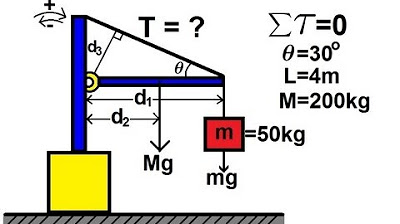
Physics 15 Torque Example 1 (1 of 7) Mass on Rod and Cable

Physics 15 Torque (3 of 27) Tension=? in the Cable

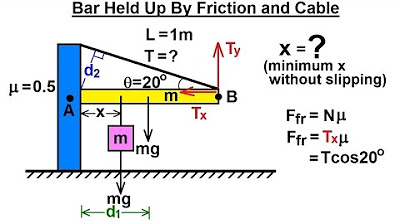
Physics 15 Torque (4 of 27) Bar Held Up by Friction

Physics 15 Torque Fundamentals (5 of 13) How to Calculate a Torque (Method 2)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsTorqueBeam MechanicsCalculationEngineeringForce AnalysisWeight CalculationGeometryTrigonometryDirection Vector