Physics 15 Torque Fundamentals (5 of 13) How to Calculate a Torque (Method 2)
TLDRThe video script explains an alternative method for calculating torque, which is the force that causes an object to rotate around a pivot point. It describes how to determine the torque by multiplying the force's magnitude by the perpendicular distance from the force's line of action to the pivot point. The script illustrates this with a right triangle, where the perpendicular distance is found by multiplying the object's length (hypotenuse) by the cosine of the angle between the force's direction and the pivot. An example is provided using a force of 20 Newtons, an object length of 4 meters, and a 30-degree angle, resulting in a torque of approximately 69 Newton-meters. The method is emphasized as a valuable approach in certain situations where it simplifies the calculation process.
Takeaways
- 📐 **Torque Calculation Method**: Torque can be calculated by multiplying the force magnitude by the perpendicular distance from the force's line of action to the pivot point.
- 🔍 **Line of Action**: The line of action is the direction in which the force is applied to the object, and a line drawn from the pivot to this line perpendicularly defines the perpendicular distance.
- ➡️ **Perpendicular Distance**: The perpendicular distance is the length from the pivot point to the line of action of the force, forming a right triangle with the force's line of action.
- 📐 **Right Triangle Relation**: The perpendicular distance is equal to the hypotenuse (length L of the object) times the cosine of the angle (theta) between the force's line of action and the object.
- 🔢 **Torque Equation**: The torque is equal to the magnitude of the force times the length of the object times the cosine of the angle theta.
- 📈 **Cosine Function**: The cosine function is used to find the perpendicular distance when the angle and the length of the object are known.
- 📚 **Consistency in Equations**: The method presented yields the same torque equation as previous methods, just approached differently.
- 🛠️ **Practical Application**: This method can be easier in certain situations, depending on the setup of the problem and the diagram provided.
- 📏 **Example Calculation**: Given a length of 4 meters, an angle of 30 degrees, and a force of 20 Newtons, the torque can be calculated as 20 N * 4 m * cos(30°), resulting in approximately 69 Newton-meters.
- 🔧 **Situational Advantage**: Sometimes calculating torque using the perpendicular distance can be more straightforward, depending on the specific problem configuration.
- ✅ **Same Result, Different Approach**: The final result remains the same regardless of the method used to calculate torque, emphasizing the importance of understanding multiple approaches.
Q & A
What is the concept of torque in physics?
-Torque is the measure of the force that can cause an object to rotate about an axis, and it is calculated by multiplying the force's magnitude by the perpendicular distance from the pivot point to the line of action of the force.
How is the line of action of a force defined?
-The line of action of a force is a line in the same direction as the force is acting and is used to determine the point at which the force is applied.
What is the perpendicular distance in the context of calculating torque?
-The perpendicular distance is the shortest distance from the pivot point to the line of action of the force, measured at a right angle to the line of action.
How does one find the perpendicular distance to the line of action of the force?
-The perpendicular distance can be found by drawing a line from the pivot point to the line of action of the force, ensuring it is perpendicular. This forms a right triangle, where the perpendicular distance is the adjacent side, and the hypotenuse is the length of the object (L).
What is the relationship between the perpendicular distance and the length of the object?
-The perpendicular distance is related to the length of the object (L) and the angle (theta) between the force and the object by the equation: perpendicular distance = L * cos(theta).
What is the formula for calculating torque using the magnitude of force, the length of the object, and the cosine of the angle?
-The formula for torque is given by: Torque = Magnitude of force * Length of the object (L) * Cosine of the angle (theta).
How does the method of calculating torque by finding the perpendicular distance differ from other methods?
-This method involves directly calculating the perpendicular distance using geometry and trigonometry, rather than working with the cosine of the angle directly, which can sometimes be more straightforward depending on the setup of the problem.
In the example given, what are the values for the length of the object, the angle, and the magnitude of force?
-In the example, the length of the object is 4 meters, the angle is 30 degrees, and the magnitude of force is 20 Newtons.
What is the calculated torque in the example provided, if we round it off?
-The calculated torque in the example is approximately 69 Newton-meters when rounded off.
Why might this method of calculating torque sometimes be easier than others?
-This method might be easier when the situation or the diagram of the system being analyzed is more conducive to geometric interpretation and when the perpendicular distance can be clearly identified and measured.
What is the significance of using different methods to calculate torque?
-Different methods can offer alternative perspectives and can be more convenient or simpler to apply in certain scenarios, providing flexibility and ensuring accuracy in a variety of situations.
How does the cosine function play a role in the calculation of torque?
-The cosine function is used to relate the angle between the force vector and the lever arm (the perpendicular distance from the pivot to the line of action of the force), allowing for the calculation of torque even when the force is not directly aligned with the lever arm.
Outlines
🔧 Understanding Torque Calculation with Perpendicular Distance
This paragraph explains an alternative method for calculating torque. It involves an object with a length L that can pivot around one end and a force applied at the other end. Torque is calculated by multiplying the force's magnitude by the perpendicular distance from the force's line of action to the pivot point. The line of action is the direction in which the force is applied, and the perpendicular distance is found by drawing a line from the pivot point to this line of action. The paragraph also explains how to find this distance by creating a right triangle, where the perpendicular distance is equal to the hypotenuse (length L) times the cosine of the angle theta. The torque formula is then derived as the force magnitude times the object's length times the cosine of theta. An example calculation is provided using a force of 20 Newtons, a length of 4 meters, and an angle of 30 degrees, resulting in a torque of approximately 69 Newtons.
Mindmap
Keywords
💡Torque
💡Pivot Point
💡Line of Action
💡Perpendicular Distance
💡Magnitude of Force
💡Right Triangle
💡Hypotenuse
💡Cosine of the Angle
💡Newtons
💡Length of the Object
💡Angle Theta
Highlights
Introduction to a different method for calculating torque.
Torque is calculated by multiplying the force's magnitude by the perpendicular distance from the force's line of action to the pivot point.
Demonstration of drawing the line of action of the force.
Explanation of the perpendicular distance as the distance from the force's line of action to the pivot point.
Formation of a right triangle to find the perpendicular distance.
The perpendicular distance is equal to the hypotenuse times the cosine of the angle theta.
Torque formula involving the magnitude of force, object length, and cosine of theta.
Comparison of this method to previous methods, emphasizing its practicality in certain situations.
Example calculation using the new method with given values: length (4m), angle (30 degrees), and force (20 Newtons).
Calculation result of torque using the example values, yielding 69 Newtons.
Emphasis on the method's utility despite its complexity in certain scenarios.
Highlighting the value of approaching torque calculation in this manner for specific setups.
Explanation of how the cosine of theta is integrated into the calculation without directly working with the angle.
Illustration of the process to find the torque by drawing the line from the pivot point to the line of action.
Clarification that the method simplifies to the same torque equation as previous methods.
Advantage of this method in situations where the setup makes it more straightforward to find the perpendicular distance.
Use of a calculator to perform the example calculation, emphasizing the practical application of the method.
Final summary of the method's effectiveness and its place in the context of torque calculation.
Transcripts
Browse More Related Video
Physics 15 Torque Fundamentals (4 of 13) How to Calculate a Torque (Method 1)
Physics 15 Torque Fundamentals (9 of 13) How to Calculate a Torque (Basic Example 3)
Physics 15 Torque (9 of 27) Alternative Method 1 & 2: Example Torque=?
Calculating Torque
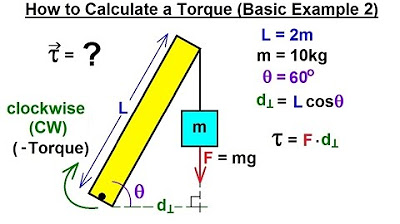
Physics 15 Torque Fundamentals (8 of 13) How to Calculate a Torque (Basic Example 2)
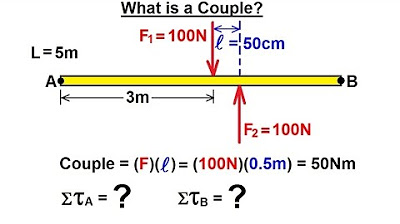
Physics 15 Torque (6 of 27) What is a Couple?
5.0 / 5 (0 votes)
Thanks for rating: