Rotational Equilibrium Introduction (and Static Equilibrium too!!)
TLDRIn this engaging educational video, the concept of rotational equilibrium is introduced and explained through a dialogue between Mr. P and his students, Bo, Bobby, and Billy. The discussion begins with a review of translational equilibrium, where an object is in equilibrium when the net force acting on it equals zero, resulting in no acceleration and either a state of rest or constant velocity. The conversation then transitions to rotational equilibrium by drawing parallels with Newton's Second Law of Motion, emphasizing that when the net torque equals zero, an object experiences no angular acceleration, meaning it is either not rotating or rotating at a constant velocity. The video uses visual examples, such as a dog at rest and a moving car, to illustrate these principles. It also distinguishes between static equilibrium, where an object is at rest and not rotating, and dynamic equilibrium, where forces and torques balance but motion continues. The video concludes with a reminder of the importance of identifying the correct axis of rotation and objects when analyzing equilibrium, setting the stage for further exploration of static equilibrium in future lessons.
Takeaways
- 📚 **Translational Equilibrium**: An object is in translational equilibrium when the net force acting on it equals zero.
- 🏃 **Physical Meaning**: An object in translational equilibrium is not necessarily at rest; it could also be moving with a constant velocity.
- 🔄 **No Acceleration**: If the net force is zero, the acceleration of the object is zero, meaning it has no linear acceleration.
- 🚗 **Examples**: Buster the dog at rest and a car moving at a constant velocity are both in translational equilibrium.
- 🔧 **Rotational Equilibrium**: An object is in rotational equilibrium when the net torque acting on it equals zero.
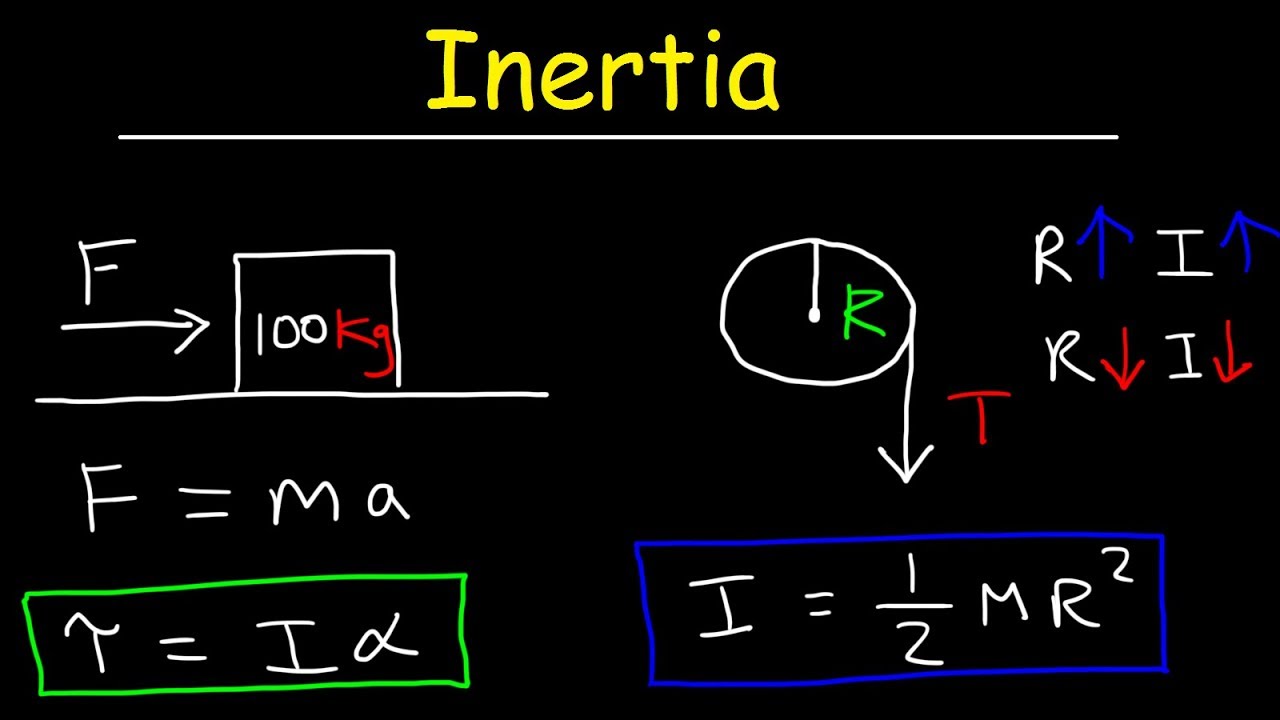
- ⚙️ **Rotational Inertia & Angular Acceleration**: The net torque equals rotational inertia times angular acceleration; in equilibrium, angular acceleration is zero.
- 🌀 **Constant Angular Velocity**: An object rotating at a constant angular velocity is in rotational equilibrium because its angular acceleration is zero.
- 📐 **Summing Forces & Torques**: When analyzing equilibrium, identify the object, forces, direction for force summation, and axis of rotation for torque summation.
- 🔩 **Static Equilibrium**: An object is in static equilibrium when it is at rest and in both translational and rotational equilibrium, with net torque equaling zero about any axis.
- 🏢 **Analyzing Structures**: The concept of static equilibrium is crucial for analyzing static structures, which will be covered in more depth in future lessons.
- 🥤 **Jinx Moment**: A light-hearted moment in the script where a character owes a soda due to repeating what someone else just said.
Q & A
What is translational equilibrium?
-An object is in translational equilibrium when the net force acting on it equals zero, which means the object is not accelerating and could either be at rest or moving with a constant velocity.
How does Newton's Second Law of Motion relate to translational equilibrium?
-According to Newton's Second Law, the net force on an object is equal to the mass of the object times its acceleration. When the net force is zero, the acceleration is also zero, indicating translational equilibrium.
What does it mean for an object to be in rotational equilibrium?
-An object is in rotational equilibrium when the net torque acting on it equals zero. This results in zero angular acceleration, meaning the object is either not rotating or rotating at a constant angular velocity.
What is the difference between static equilibrium and rotational equilibrium?
-Static equilibrium refers to a state where an object is at rest and in both translational and rotational equilibrium simultaneously. In this case, the net torque equals zero about any axis of rotation.
Why is it important to identify the axis of rotation when calculating torque?
-Identifying the axis of rotation is crucial because the torque, which is a measure of the force that can cause rotational motion, depends on the choice of the axis. Different axes can yield different torque values for the same force.
Can an object moving at a constant velocity be considered to be in translational equilibrium?
-Yes, an object moving at a constant velocity is in translational equilibrium because the net force acting on it is zero, resulting in no acceleration.
What does it mean for a ceiling fan to be in rotational equilibrium when it is rotating at a constant speed?
-When a ceiling fan is rotating at a constant speed, the net torque acting on it is zero because the torque from the motor is balanced by the torque due to internal friction and air resistance on the fan blades.
What is the relationship between angular acceleration and rotational equilibrium?
-An object is in rotational equilibrium when its angular acceleration is zero. This means that the object's angular velocity is constant, indicating no change in the rate of rotation.
What is the significance of visualizing forces and torques in understanding equilibrium?
-Visualization helps to understand the concept of equilibrium by showing how the sum of forces or torques can result in a state of no acceleration (translational) or no change in the rate of rotation (rotational), making it easier to grasp abstract physical principles.
Why do we consider both translational and rotational equilibrium when analyzing static structures?
-Considering both types of equilibrium is important for a complete analysis of static structures because it accounts for all possible types of motion or lack thereof. It ensures that the structure is stable and not subject to any form of acceleration or rotation.
What does it imply if the net torque on an object equals zero about any axis of rotation?
-If the net torque on an object equals zero about any axis of rotation, it implies that the object is in static equilibrium. This means the object is at rest and not undergoing any form of rotational motion around any axis.
How does the concept of equilibrium help in analyzing physical systems?
-The concept of equilibrium is fundamental in physics for analyzing physical systems. It allows us to determine the conditions under which an object or a system remains in a state of rest or uniform motion, which is essential for predicting the behavior of structures and mechanisms under various forces and torques.
Outlines
📚 Introduction to Translational and Rotational Equilibrium
In the first paragraph, Mr. P introduces the concept of rotational equilibrium, following a review of translational equilibrium. Bo explains that an object is in translational equilibrium when the net force acting on it is zero, which means no linear acceleration and the object could either be at rest or moving at a constant velocity. Billy and Bobby further clarify that while an object at rest is in translational equilibrium, an object in equilibrium is not necessarily at rest. Mr. P uses the example of his dog Buster to illustrate an object at rest in equilibrium and his car to represent an object in motion with no acceleration. The paragraph concludes with a transition to rotational equilibrium, asking Billy to apply the principles of translational equilibrium to rotational motion.
🌀 Understanding Rotational Equilibrium and Static Equilibrium
The second paragraph delves into rotational equilibrium, where Billy suggests starting with the rotational form of Newton's Second Law of Motion and setting net torque to zero. This leads to the conclusion that an object in rotational equilibrium has zero angular acceleration, meaning it is either not rotating or rotating at a constant angular velocity. Mr. P uses the example of a ceiling fan to illustrate both a fan at rest and one rotating at a constant velocity, both in rotational equilibrium due to the net torque being zero. The paragraph also emphasizes the importance of identifying the correct object and axis of rotation when summing forces and torques. The concept of static equilibrium is introduced as a state where an object is at rest and in both translational and rotational equilibrium, with the net torque equaling zero about any axis. The paragraph concludes with a note on the practical applications of static equilibrium in analyzing static structures, which will be further explored in future lessons.
Mindmap
Keywords
💡Translational Equilibrium
💡Net Force
💡Acceleration
💡Rotational Equilibrium
💡Torque
💡Angular Acceleration
💡Static Equilibrium
💡Axis of Rotation
💡Newton's Second Law of Motion
💡Rotational Inertia
💡Linear Acceleration
Highlights
An object is in translational equilibrium when the net force acting on it equals zero.
An object in translational equilibrium is not necessarily not moving; it could be at rest or moving with a constant velocity.
The net force equals mass times acceleration, which is the translational form of Newton's Second Law of Motion.
If the net force equals zero, the acceleration of the object equals zero, meaning it is not accelerating.
An object in rotational equilibrium has a net torque of zero, which equals rotational inertia times angular acceleration.
An object that is not rotating or rotating at a constant angular velocity is in rotational equilibrium.
Rotational equilibrium is analogous to translational equilibrium but applies to rotational motion.
When the net torque acting on an object equals zero, its angular acceleration is zero, indicating no change in angular velocity.
Visualizing translational equilibrium with examples like a dog at rest and a car moving at a constant velocity.
A ceiling fan at rest with zero torque acting on it is in rotational equilibrium.
A fan rotating at a constant angular velocity has a net torque of zero due to balanced forces.
When summing forces or torques, it's important to identify the object, direction, and axis of rotation.
Static equilibrium occurs when an object is at rest and in both translational and rotational equilibrium simultaneously.
In static equilibrium, the net torque equals zero about any axis of rotation.
Static equilibrium is crucial for analyzing static structures and will be covered in more depth in future lessons.
The concept of static equilibrium is helpful for understanding the balance of forces in stationary structures.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: