Introduction to Static Equilibrium
TLDRThis tutorial introduces the concept of static equilibrium, focusing on objects that are not moving or are in a state of balance where all forces acting on them are equal and cancel each other out. The video explains the importance of understanding vectors and forces, and how to apply equilibrium principles to determine forces such as tension in a system. It uses examples, including an object on a table and a scenario with forces acting in different directions, to illustrate how to resolve vectors and calculate the magnitude and direction of forces. The tutorial also works through a problem involving a massless pulley system to find the tension forces and the mass of an object, emphasizing the need to consider vector components and direction when analyzing equilibrium.
Takeaways
- 📚 The concept of static equilibrium refers to objects that are not moving or are in a state of balance, where all acting forces are equal or balanced.
- ⚖️ To achieve equilibrium, the sum of all forces acting on an object must be zero when considering both magnitude and direction.
- 📈 Vectors play a crucial role in understanding forces because they have both magnitude and direction, which must be accounted for when summing forces.
- 🔄 When resolving vectors, it's necessary to break down forces into components that act along the same axis to ensure accurate summation towards equilibrium.
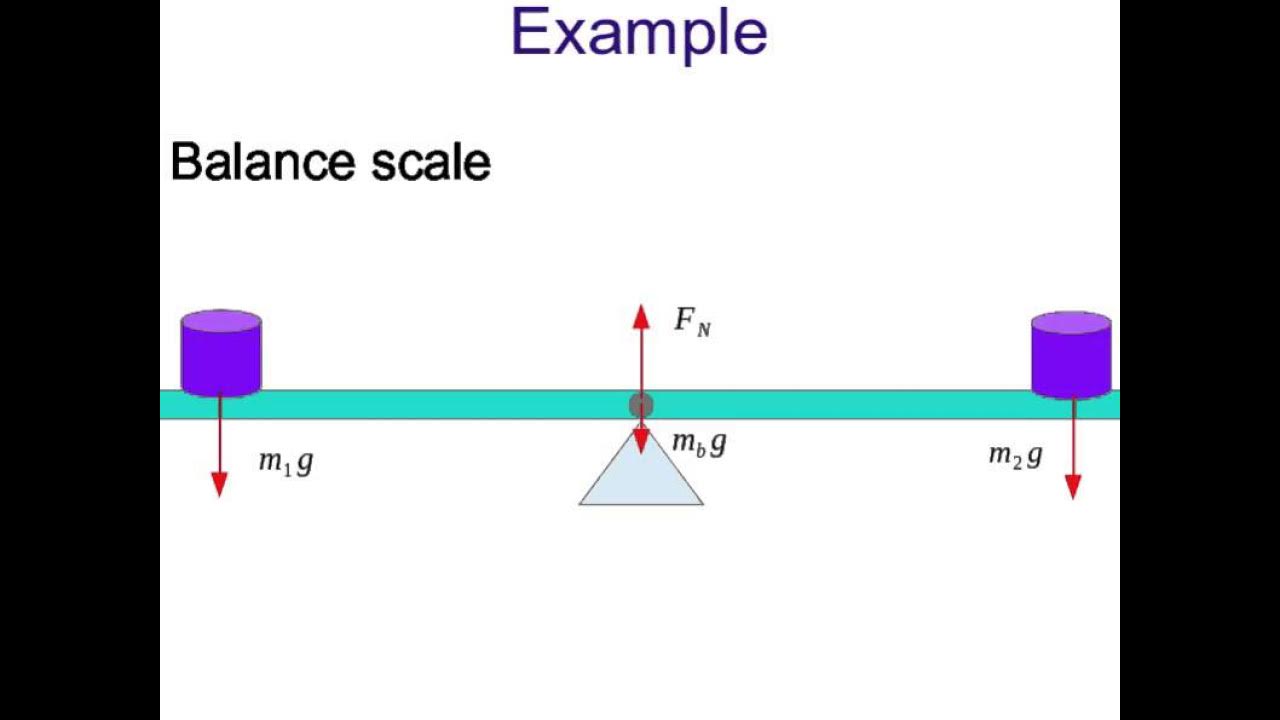
- 🧮 The normal force is an example of a force that opposes the weight (mg) of an object, allowing it to remain at rest on a surface.
- 🔢 In a scenario with two forces acting on an object in equilibrium, the forces must be equal in magnitude and opposite in direction to sum to zero.
- 📐 Resolving vectors involves using trigonometric functions like cosine and sine to find the components of forces acting at angles to the axis of interest.
- ⚙️ A massless pulley, as mentioned in the example, does not alter the tension in the string or rope, maintaining the tension throughout its length.
- 🤔 Understanding the direction of forces is essential, as forces acting in opposite directions on the same axis can cancel each other out when summed vectorially.
- 📐 The process of resolving vectors is demonstrated through an example problem, where forces are broken down into components and then summed to find equilibrium.
- 📝 In solving for unknowns like tension forces (T1, T2) or mass (m2), it's important to apply the equilibrium condition that the sum of forces in each direction equals zero.
- 💡 The video script emphasizes the importance of understanding vector resolution and equilibrium for students who are new to these concepts in physics.
Q & A
What does static equilibrium mean in the context of this tutorial?
-In this tutorial, static equilibrium refers to situations where all the forces acting on an object are balanced, meaning the sum of the forces is zero, indicating that the object is either not moving or is in a state of balance.
What is the normal force in the context of an object on a table?
-The normal force is the force that opposes the weight of an object resting on a surface, such as a table. It acts perpendicular to the surface and is equal in magnitude to the weight of the object when the object is in equilibrium.
How does the direction of a force affect its vector representation?
-The direction of a force is crucial in its vector representation. Vectors have both magnitude and direction, so when forces are added together, their directions must be taken into account. Forces in opposite directions on the same axis can cancel each other out, resulting in a net force of zero.
What is the significance of resolving vectors when dealing with forces?
-Resolving vectors is essential when dealing with forces because it allows us to break down forces into components that act along specific axes. This is necessary to accurately calculate the net force along each axis, which is crucial for determining if the system is in equilibrium.
How is the weight of an object related to its mass and the acceleration due to gravity?
-The weight of an object is the force due to gravity acting on its mass. It can be calculated using the formula W = m * g, where m is the mass of the object and g is the acceleration due to gravity.
What is the assumption made about the pulley in the given example?
-The assumption made about the pulley in the example is that it is massless. This means that the tension in the rope remains the same throughout, as the pulley does not affect the tension due to its own mass or rotation.
How do you find the components of a force acting at an angle to the horizontal?
-To find the components of a force acting at an angle to the horizontal, you use trigonometric functions. The horizontal component is found using the cosine of the angle (F_x = F * cos(theta)), and the vertical component is found using the sine of the angle (F_y = F * sin(theta)).
What is the process to find the tension T1 in the given example?
-To find the tension T1, you consider the forces acting on mass m1 in the vertical direction. The weight of m1 (m1g) and the tension T1 are the two forces. Since the system is in equilibrium, these forces must sum to zero, leading to the equation T1 - m1g = 0. Solving for T1 gives T1 = m1g.
How do you calculate the mass m2 of the second object in the system?
-To calculate the mass m2, you consider the forces in the vertical direction (y-axis), which include T1y, T2y, and m2g. Since the system is in equilibrium, the sum of these forces is zero. By substituting known values and solving the equation, you can find the unknown mass m2.
What is the importance of considering the direction of forces when summing them in a system?
-Considering the direction of forces is important because forces are vectors, which means they have both magnitude and direction. When summing forces, you must consider their direction to determine if they will add or subtract from each other. This is essential for accurately calculating the net force and determining if a system is in equilibrium.
How does the masslessness of the pulley simplify the calculation of tensions in the system?
-The masslessness of the pulley simplifies the calculation because it implies that there is no additional force required to move the pulley itself. This means that the tension in the string (T1) remains constant throughout, eliminating the need to account for variations in tension due to the pulley's mass.
Outlines
📚 Introduction to Static Equilibrium
This paragraph introduces the concept of static equilibrium, which refers to objects that are not moving or are in a state of balance where all the forces acting on the object cancel each other out. The tutorial emphasizes the importance of understanding vectors and forces. It also explains how to apply the concept of equilibrium to an object on a table, illustrating the opposing forces of weight (mg) and the normal force (Fn), which must sum to zero in vector form. The directionality of forces is highlighted as a critical aspect of vector addition.
📐 Vectors and Force Equilibrium in Different Scenarios
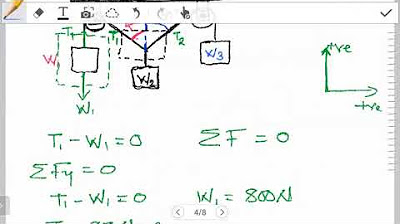
The second paragraph delves into the application of static equilibrium with examples involving horizontal forces acting on an object. It demonstrates how to calculate the net force when multiple forces are involved, emphasizing the need to consider the direction of each force as vectors. The concept of resolving vectors into their components along the same axis for addition is introduced, and the importance of specifying both magnitude and direction for each force is reiterated. An example involving a pulley system is presented to illustrate these principles, with a focus on the assumption of a massless pulley and its implications on tension in the system.
🔍 Resolving Vectors and Calculating Tensions
This paragraph focuses on the process of resolving vectors into their components and using this technique to solve for unknown forces in a system. It provides a step-by-step approach to finding the components of forces acting at various angles using trigonometric functions. The paragraph also covers how to set up and solve equations for forces in equilibrium, specifically detailing the calculation of tension forces (T1 and T2) in a given scenario. The importance of considering the direction of the forces when resolving vectors is emphasized.
🧮 Calculation of T1 and T2 Using Trigonometry
The fourth paragraph continues the example from the previous one, focusing on the calculation of the tension forces T1 and T2 using trigonometric relationships in right-angled triangles. It explains how to find the horizontal (T1x) and vertical (T1y) components of the tension force T1 using cosine and sine functions for angles given in the problem. The paragraph also outlines the process of finding the components of T2, noting the need to consider the direction of the force components when applying them to the equilibrium equations.
📐 Summing Forces in the X and Y Axes for Equilibrium
In this paragraph, the focus shifts to summing the forces acting on the system along the x and y axes to ensure equilibrium. It demonstrates how to set up and solve equations for the forces in the x-axis (T1x and T2x) and y-axis (T1y, T2y, and the weight of m2, m2g). The process involves substituting the resolved components of the forces into the equilibrium equations and solving for the remaining unknowns, such as T2 and the mass m2. The paragraph concludes with the results of T2 being 115 newtons and m2 being 16.4 kg.
🎓 Conclusion and Invitation for Feedback
The final paragraph wraps up the tutorial by summarizing the quantities that were solved for: T1 (98 newtons), T2 (115 newtons), and m2 (16.4 kg). It invites feedback from viewers, particularly those who are new to vectors, and asks for topics they would like to be emphasized in the next video. The tutor also hints at the next video, which will cover another example using the same concepts, and encourages viewers to attempt a related problem before the next session.
Mindmap
Keywords
💡Static Equilibrium
💡Vectors
💡Forces
💡Normal Force
💡Tension
💡Mass
💡Weight
💡Resolving Vectors
💡Components of a Vector
💡Pulley System
💡Equation of Equilibrium
Highlights
Introduction to the concept of static equilibrium and its application.
Explanation of equilibrium as a state where all forces acting on an object are balanced or equal.
Importance of understanding vectors and forces to grasp the topic of equilibrium.
The necessity to consider the direction of forces when summing them in vector form.
Example of an object on a table, illustrating the balance between weight and the normal force.
Use of the normal force (Fn) to oppose the weight (mg) of an object in equilibrium.
Demonstration of how to calculate forces in a system with multiple forces acting on an object.
Emphasis on the vector nature of forces, requiring both magnitude and direction for accurate calculations.
Procedure for resolving vectors into their components along different axes.
Explanation of how to handle forces acting in different directions when summing them to zero.
Detailed walkthrough of a problem involving a massless pulley system to find tensions (T1, T2) and mass (m2).
Assumption that the pulley is massless, which implies no change in tension across the pulley.
Calculation of tension T1 using the weight of mass m1 and the concept of equilibrium.
Resolution of vector T2 into its x and y components using trigonometric functions.
Final calculation of mass m2 by summing forces in the y-axis and applying equilibrium conditions.
The importance of considering the direction of each force component when summing them vectorially.
Offer to create a video on resolving vectors for those who are struggling with the concept.
Invitation for feedback on the difficulty of understanding vectors for first-time learners.
Promise of another example in the next video to reinforce understanding of static equilibrium.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: