Math 1325 Lecture 12 2
TLDRThis lecture delves into the intricacies of calculus, specifically focusing on the power rule for integrals in the context of business and social science. The script explains the standard power rule for integration, which involves increasing the power by one and multiplying by one over the new power. It then explores the application of this rule to composite functions, where the derivative of the inner function is also considered. The lecture provides a step-by-step guide on how to apply the power rule to composite functions, including the use of substitution and the importance of including both the inner function and its derivative in the integrand. Several examples are worked through, illustrating the process of identifying the inner function, finding its derivative, and applying the composite rule to find the integral. The script also touches on the limitations of the method when dealing with variable terms rather than constants and suggests alternative approaches, such as foiling and separating terms for easier integration. The lecture concludes with a reminder of the importance of checking solutions by differentiating the result to ensure it matches the original integrand.
Takeaways
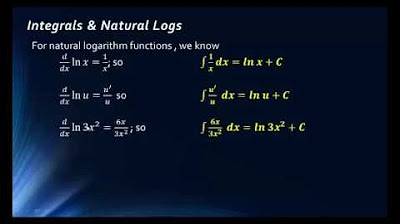
- 📚 The integral of a power function x^n is x^{n+1}/(n+1) + C, where C is the constant of integration.
- 🔍 When dealing with composite functions, the integral requires considering both the inner function and its derivative.
- 📈 For composite functions, an alternative form of the power rule is used, which includes the entire function raised to the power of n+1 divided by n+1, plus the constant of integration.
- 🔑 The substitution method is a useful technique for integrating composite functions, where you substitute the inner function with a new variable, u.
- 🧮 The derivative notation Dy/Dx can be manipulated to help rewrite integrals in a form that is easier to solve.
- ✅ After finding an integral, it's good practice to check the result by differentiating the solution to see if it matches the original integrand.
- 📉 When integrating a constant times a function, the constant can be factored out of the integral, but this does not apply to variable terms.
- 🤔 Some integrals may not be solvable using the substitution method if the necessary parts of the integrand are not present, and alternative methods like foiling and separating terms may be required.
- 📝 It's essential to identify the inner function and its derivative when using substitution, as these are the parts that will be substituted into the integral.
- 🔗 The integral of a function raised to a power can be solved using the composite rule, which involves raising the inner function to the power of n+1 and dividing by n+1.
- 📌 Remember that the integral of a function is the reverse process of differentiation, and understanding this relationship is crucial for solving integrals.
Q & A
What is the power rule for integration in calculus?
-The power rule for integration states that the integral of a function raised to the power of n is obtained by increasing the power by one, dividing the function by the new power, and adding a constant of integration. Mathematically, it's represented as ∫x^n dx = (x^(n+1))/(n+1) + C, where n ≠ -1.
How does the integration of composite functions differ from simple functions?
-When integrating composite functions, one must consider both the outer function and the derivative of the inner function. This is essential because the integration needs to reconstruct the original function from its derivative, accounting for the chain rule used in differentiation.
What is meant by 'composite function' in calculus?
-A composite function is a function made up of two or more functions, where the output of one function becomes the input of another. In calculus, dealing with composite functions often involves applying the chain rule during differentiation and a modified power rule during integration.
Can you explain the substitution method used in the integration of composite functions?
-The substitution method in integration involves replacing a part of the integral with a new variable, usually to simplify the integration process. This involves setting a part of the function (often the inner function in a composite function) as 'u', finding du by differentiating u with respect to x, and then replacing these in the integral.
What does 'dx' represent in an integral?
-'dx' represents a differential element of the variable x in an integral, indicating the variable with respect to which the function is being integrated. It is part of the notation used in the integral to define the integration process over x.
Why is it necessary to include the derivative of the inner function in the integrand when integrating a composite function?
-Including the derivative of the inner function in the integrand is necessary due to the chain rule of calculus. This rule is applied during differentiation of composite functions and must be considered in reverse during integration to correctly reconstruct the original function.
What happens if a constant is factored out during the integration process?
-When a constant is factored out during the integration process, it is placed outside the integral sign. This is based on the linear property of integrals, which allows constants to be separated from the function being integrated, simplifying the calculation.
How do you verify the correctness of an integral?
-To verify the correctness of an integral, one can differentiate the resulting function and compare it to the original integrand. If the differentiation of the integrated function yields the original function (accounting for the constant of integration), the integral is correct.
What is the purpose of the constant of integration?
-The constant of integration represents an indefinite number added to the function during integration. It accounts for the fact that the antiderivative of a function is not unique but rather a family of functions differing only by a constant.
How does the integration of a function using substitution differ from integration without substitution?
-Integration using substitution typically involves changing the variable and the differential part of the integral to simplify the function into a more manageable form. This is particularly useful for composite functions or when the integrand involves complex expressions. Without substitution, the integral is performed directly on the original variables and expression, which can be more complex or unwieldy.
Outlines
📚 Power Rule for Calculus in Business and Social Science
This paragraph introduces the power rule for calculus, specifically for business and social science. It explains the integral or anti-derivative of a power function, which is to increase the power by one and multiply by one over the new power. The paragraph also discusses the application of the power rule to composite functions, where the derivative of the inner function is multiplied by the outer function's derivative. An example is given where the integral of (12x * (3x^2 + 2)) is calculated using substitution and the power rule. The importance of including both the inner function and its derivative in the integrand to revert to the original function is emphasized.
🧮 Composite Functions and Integration by Substitution
The second paragraph delves into the process of integrating composite functions using substitution. It demonstrates how to identify the power function and its derivative within the integral. The paragraph provides a step-by-step guide on how to perform substitution, apply the composite rule, and then substitute back to find the final solution. It also includes a method to check the solution by differentiating the result to see if it matches the original integrand. Two examples are given, one with a radical and one with a polynomial, to illustrate the process. The paragraph highlights the importance of correctly identifying the inner function and its derivative for successful integration.
🚫 Limitations of Integration by Substitution
The third paragraph addresses the limitations of integration by substitution when dealing with variable terms rather than constants. It explains that while constants can be pulled out of the integral, variable terms cannot be treated in the same way. The paragraph provides an example where the integrand (x^2 + 4) raised to the power of two cannot be solved using the substitution method due to the absence of a mechanism to pull a variable outside of an integral. Instead, the paragraph suggests an alternative approach by foiling the polynomial and integrating each term separately, which simplifies the process. The effectiveness of this method is demonstrated by taking the derivative of the result and verifying that it reverts to the original integrand.
Mindmap
Keywords
💡Integral
💡Anti-derivative
💡Power Function
💡Composite Function
💡Constant of Integration
💡Derivative
💡Substitution
💡Exponent
💡Inner Function
💡Power Rule
💡Differentiation
Highlights
The integral of a power function is derived by increasing the power by one and multiplying by one over the new power.
When dealing with composite functions, the integral includes both the inner function and its derivative.
An alternative form of the power rule is introduced for composite functions.
Substitution is used to simplify the integration of composite functions.
The integral of a composite function raised to a power includes the function to the power of n + 1 over n + 1.
Derivatives can be thought of in a rational notation, aiding in the understanding of calculus problems.
The change in y (Dy) equals the derivative of y with respect to x (f'(x)) times the differential of x (dx).
Examples are provided to illustrate the application of the power rule for composite functions.
Integration can be checked by differentiating the result to see if it matches the original function.
Radicals can be rewritten as powers, simplifying integration.
The use of substitution is demonstrated in solving integrals, with U representing the inner function.
Constants can be factored out of integrals, simplifying the integration process.
The importance of including both the inner function and its derivative in the integrand is emphasized.
The process of foiling and separating terms is shown as an alternative method for integrating polynomials.
Integration of a function can sometimes be simplified by multiplying out and then integrating each part.
The method of adding missing factors and offsetting them with reciprocals is explained for solving certain integrals.
The integral of a function can be checked by differentiating to ensure the result is correct.
The transcript provides a comprehensive guide on applying the power rule for calculus in business and social science contexts.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: