MATH 1325 Lecture 12 3 Integrals of Exponents and Logarithms
TLDRThe video lecture provides an in-depth exploration of integral calculus involving exponential and logarithmic functions. It begins with a review of the natural exponential function, emphasizing its unique property where the derivative equals the function itself, leading to the antiderivative also being the exponential function plus a constant. The lecture then delves into the integration of exponential functions with a variable exponent, using substitution methods and the chain rule. A step-by-step approach, referred to as the 'EDICT' method, is introduced for solving such integrals, which involves identifying the inner function, its derivative, and performing substitution to simplify the integral to a form that can be easily solved. The method is demonstrated with several examples, including a practical application to model the market value of a house over time. The second half of the lecture focuses on the integration of natural logarithmic functions, highlighting the derivative of the natural log and its integral form. The process for integrating composite functions involving natural logs is also explained, using substitution similar to the exponential case. The lecture concludes with a reminder to check answers by differentiating the result and ensuring it matches the original integrand, emphasizing the importance of accuracy in mathematical procedures.
Takeaways
- 📚 The derivative of the natural exponential function e^x is itself, e^x, and its antiderivative is also e^x + C.
- 🔄 When integrating exponential functions of the form e^u where u is a function of x, use substitution with u as the exponent and du as the derivative of the exponent.
- 📈 For composite functions, the integral of e^u * du is e^u + C, which simplifies the integration process.
- 🤔 The method for integrating exponential functions with a compound exponent is called the 'edict' method, involving steps like identifying the inner function, finding its derivative, and substitution.
- 🏡 In word problems, such as modeling the value of a house, the integral of the rate of change with respect to time can find the original function, which represents the value over time.
- 🧮 To find an unknown constant like C in an integral, use a reference point provided in the problem, like the initial value of a house in a given year.
- ✍️ Always check the answer by differentiating it to ensure it matches the original integrand, which helps to catch mathematical errors.
- 🚫 Be cautious with the natural log integrals where the argument is a function of x; ensure the numerator is the derivative of the denominator to apply the natural log rule.
- 🔑 The integral of 1/u * du is the natural log of u + C, which is key for integrating quotients where the numerator is the derivative of the denominator.
- 🔢 For problems involving rates of change, like the value of a house over time, integrate the given derivative to find the original function, then apply initial conditions to solve for constants.
- 📈 When applying the 'edict' method, remember to factor out constants and use reciprocals to match the integral's form to the natural log or exponential integral form.
- 💡 Practice is essential; after learning the method, attempt problems independently and then check your work against the provided solutions to reinforce understanding.
Q & A
What is the derivative of the natural exponential function e^x?
-The derivative of the natural exponential function e^x is e^x itself, which is a unique property where the slope of the function at every point is equal to the function value at that point.
What is the antiderivative of e^x?
-The antiderivative of e^x is e^x plus a constant C, which is derived from the fundamental theorem of calculus stating that integrals are antiderivatives.
How do you integrate an exponential function with a variable exponent?
-When integrating an exponential function with a variable exponent, represented as e^u where u is a function of x, you use substitution, treating u as the inner function and du/dx as the derivative of the inner function. The integral becomes e^u + C.
What is the 'EDICT' method mentioned in the transcript?
-The 'EDICT' method is a mnemonic for solving integrals involving exponential functions with variable exponents. It stands for Exponent, Differentiate, Identify, Check, and Transform, and is a systematic approach to performing the substitution method for such integrals.
How do you find the integral of a natural logarithm function with a variable argument?
-To find the integral of a natural logarithm function with a variable argument, you use the chain rule for integration. If the argument is a function of x, you set u equal to the argument, find du/dx, and then integrate u'/u du to get ln(u) + C.
What is the integral of 1/x dx?
-The integral of 1/x dx is the natural logarithm of the absolute value of x plus a constant, ln|x| + C.
What is the significance of finding the value of the constant C in an integral?
-The constant C represents an arbitrary constant of integration. Finding its value is significant because it allows you to determine the specific function that passes through a given point, which is often provided in word problems.
How do you determine the value of the constant C in an integral?
-You determine the value of the constant C by using a reference point given in the problem, such as an initial condition or a specific value of the function at a particular point.
What is the integral of e^(ax^2) dx?
-The integral of e^(ax^2) dx is e^(ax^2) plus a constant C, where a is a constant and x is the variable. This is derived using substitution with u = ax^2 and du/dx = 2ax.
What is the EDICT method's first step when solving an integral with an exponential function of x?
-The first step in the EDICT method is to identify the inner function, which is the exponent of the exponential function in the integral.
How does the EDICT method handle constants within the integral?
-The EDICT method allows adding a constant to the integral as long as its reciprocal is also included outside the integral to maintain the equality, which helps in matching and substituting parts of the integral.
What is the process for integrating a function like (2x + 1)e^(x^2 + 2x) dx?
-The process involves setting the inner function u equal to the exponent (x^2 + 2x), finding its derivative du/dx, and then using substitution to transform the integral into a form that can be solved, resulting in e^u + C after integration.
Outlines
📚 Introduction to Exponential and Logarithmic Integrals
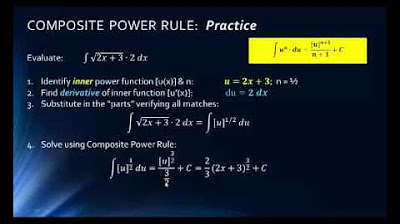
This paragraph introduces the topic of integrals involving exponential and logarithmic functions. It begins with a review of the properties of the natural exponential function, emphasizing the unique relationship between its derivative and the function itself. The integral of e^x is shown to be e^x + C. The concept of substitution, represented by 'u' for a function of x, is introduced for more complex integrals. An example is provided where the exponent is a function of x, specifically 3x^2, and the integral is solved using the chain rule. The paragraph also outlines a method called the 'edict' method for solving integrals with exponential composite functions, which includes steps like identifying the inner function, finding its derivative, and performing substitution to simplify the integral.
🔍 The Edict Method for Exponential Integrals
This paragraph delves deeper into the 'edict' method for solving exponential integrals. It explains the process of substitution by matching parts of the integral with the derivative of the inner function. An example is given where the inner function is x^2 + 2x, and the method is demonstrated step by step, including the correction of a typo in the process. The paragraph emphasizes the importance of checking the answer by taking the derivative and ensuring it matches the original integrand. It also discusses the direct integration approach for simpler exponential functions without a compound exponent.
🧮 Integrating Exponential Functions with Negative Exponents
This paragraph discusses the integration of exponential functions with negative exponents. It explains how to handle integrals with a negative exponent by moving the exponent to the denominator and adjusting the integral accordingly. An example is provided where the inner function is -x^3, and the integral is solved using substitution and the addition of a constant to balance the equation. The paragraph also covers the process of reversing the substitution to obtain the final answer and emphasizes the importance of converting the answer back to its original form for clarity.
📈 Integration of Natural Logs and Composite Functions
This paragraph focuses on the integration of natural logarithms, particularly when the argument of the log is a function of x. It explains the derivative of the natural log and how it leads to the integral of 1/x dx being the natural log of x plus C. The concept of a chain rule is applied when the argument of the natural log is a function of x. The paragraph provides an example of integrating the natural log of a function and outlines a method for integrating quotients where the numerator is the derivative of the denominator. It also discusses how to identify and solve such integrals using substitution, with examples and a reminder to check the solution by differentiating.
🏠 Applying Integration to a Real-World Problem: House Value
This paragraph presents a word problem involving the market value of a house over time. It provides a function for the rate of change of the house value and asks to find the function expressing the value of the house in terms of time. The paragraph demonstrates how to use integration to find the original function from its derivative, applying the 'edict' method with the exponential function as the inner function. It also shows how to determine the constant of integration using a reference point, such as the house value in 2015. The paragraph concludes with solving for the predicted value of the house in 2025, emphasizing the importance of units and careful calculation.
💡 Finding the Constant of Integration and Practical Applications
The final paragraph emphasizes the importance of finding the constant of integration, 'C', in problems where a reference point is provided or when common sense can be applied to determine it. It discusses how to approach word problems and real-world applications by understanding the context and using logical reasoning to find the value of 'C'. The paragraph provides examples of common reference points in different types of functions, such as revenue and fixed costs, and advises not to overthink the process.
Mindmap
Keywords
💡Exponential Functions
💡Derivatives
💡Integrals
💡Chain Rule
💡Natural Logarithm
💡Antiderivatives
💡Substitution Method
💡Composite Function
💡Integration by Parts
💡U-Substitution
💡Word Problems
Highlights
Derivative of the natural exponential function e^x is e^x, which is unique as the slope equals the function value at every point.
The integral (antiderivative) of e^x is e^x + C, where C is the constant of integration.
When integrating e^u where u is a function of x, apply the chain rule by taking the derivative of e^u (e^u) and multiplying by the derivative of u (u').
The integral of e^u * u' du is e^u + C, which is found by recognizing the integral as an application of the chain rule.
Example given for integrating e^(3x^2) which results in e^(3x^2) + C after applying the chain rule.
The 'EDICT' method is introduced as a memorable acronym for integrating exponentials with u as the exponent.
The inner function u is always the exponent in the integral of e^u * du.
The derivative of the inner function u (u') is found by differentiating the exponent in the integral.
The substitution method is used in the 'go fish' step to match and substitute parts of u and du into the integral.
The integral of 1/x dx is the natural logarithm ln(x) + C, which is a direct result of the derivative of ln(x) being 1/x.
For integrals with ln(u) where u is a function of x, use the chain rule by differentiating 1/u * u'.
The integral of u'/u du results in ln(u) + C, which is derived from the derivative of ln(u).
When the numerator is the derivative of the denominator in a fraction, it indicates a natural logarithm composite function integral.
The substitution method is also applicable to natural logarithm integrals, following a similar process to exponential integrals.
The reference point for the constant C in an integral is often given in word problems, such as the initial value of a function.
In word problems, apply the integral to find the original function when given its derivative, and use reference points to solve for constants.
For exponential growth models, such as the value of a house over time, integrate the rate of change to find the value function.
Use the initial condition to solve for the constant of integration in exponential growth models.
Predict future values using the integrated function by substituting future time points.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: