4.5 - Integration Techniques - Substitution
TLDRThe video script offers an in-depth exploration of the integration technique known as substitution, which is instrumental in integrating more complex functions derived from the chain rule. The process involves identifying an inner and outer function within a composite function, ensuring the derivative of the inner function is present. Substitution simplifies the function by replacing the inner function with a new variable, 'u', and adjusting the differential accordingly. The technique is adept at reversing the differentiation process to find anti-derivatives. The script walks through several examples, illustrating how to apply substitution to a variety of functions, including those involving natural logarithms and exponentials. It emphasizes the importance of resubstitution to revert to the original variable and highlights the verification of anti-derivatives by differentiation. The script also addresses the calculation of definite integrals using substitution, with a reminder to apply limits of integration only after reverting to the original variable.
Takeaways
- 📚 **Integration by Substitution**: A technique used to integrate complex functions that are the result of differentiation using the chain rule.
- 🔄 **Chain Rule Review**: When differentiating a composed function, the derivative is the product of the derivative of the outer function and the derivative of the inner function.
- 🔑 **Identifying Inner and Outer Functions**: To integrate using substitution, one must identify the inner and outer functions and ensure the derivative of the inner function is present in the integral.
- 🔍 **Substitution Strategy**: The substitution variable, often 'u', is set equal to the inner function, and the integral is then solved in terms of 'u'.
- 🧬 **Manipulating Differentials**: The differential 'dx' must be expressed in terms of the new variable 'u', which requires finding 'du/dx' and solving for 'du' and 'dx'.
- ↔️ **Resubstitution**: After integrating with respect to 'u', the original variable 'x' must be substituted back in to find the final antiderivative.
- 📐 **Definite Integrals**: When evaluating definite integrals, the limits of integration are applied after resubstitution to the original variable.
- 🔗 **Function and Derivative Relationship**: For successful substitution, look for a function and its derivative within the integral; if found, use the function as the substitution variable.
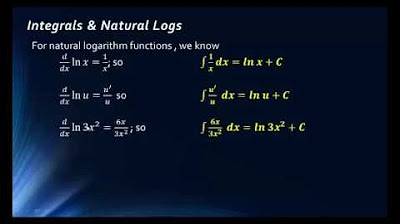
- 📉 **Natural Logarithm Cases**: Special attention is needed when dealing with natural logarithms; the derivative of the log function can be used for substitution even if it's not the inner function.
- 🤔 **Logarithm Rules**: Logarithm properties, such as pulling out exponents, can simplify the integral and make it more suitable for substitution.
- 📈 **Exponential Functions**: When the inner function is an exponential, the substitution can involve the entire exponent, not just the base, especially when the derivative is present in the integral.
Q & A
What is the main topic discussed in the video?
-The video discusses the integration technique known as substitution, which is used to integrate more complex functions, particularly those resulting from differentiation using the chain rule.
How does the substitution technique relate to the chain rule in differentiation?
-The substitution technique works backwards from the chain rule in differentiation. It allows us to find the anti-derivative of functions that were derived using the chain rule by substituting the inner function with a new variable and adjusting the differential accordingly.
What is the first step in applying the substitution technique?
-The first step is to identify the inner and outer functions of the composite function you are trying to integrate.
Why is it necessary to have the derivative of the inner function present when using substitution?
-The derivative of the inner function must be present because it is a factor in the function that is being integrated. This allows for the simplification of the integral into a form that can be more easily solved using substitution.
How do you determine the differential 'du' when making a substitution?
-You determine 'du' by differentiating the substitution expression with respect to 'x', which gives you an expression involving 'du/dx'. You then solve for 'du' by isolating it, typically by multiplying both sides of the equation by 'dx'.
What is the purpose of resubstitution in the substitution technique?
-Resubstitution is the process of replacing the substitution variable 'u' back with the original inner function to express the integral in terms of the original variable after the integration with respect to 'u' has been performed.
How does the substitution technique help in evaluating definite integrals?
-The substitution technique simplifies the function within the integral, allowing for easier computation. After finding the anti-derivative with respect to the new variable, the original limits of integration are applied once the function is resubstituted back in terms of the original variable.
What is the role of the constant of integration 'c' in the context of the substitution technique?
-The constant of integration 'c' is added after performing the integration to account for the constant that would result from indefinite integration. It represents an arbitrary constant that can appear in the antiderivative of a function.
Why is it important to verify the answer when using the substitution technique?
-Verifying the answer by differentiating the found antiderivative ensures that the technique was applied correctly and that the resulting integral matches the original function, providing confidence in the solution's accuracy.
Can the substitution technique be applied to any complex integral, or are there conditions that must be met?
-The substitution technique cannot be applied to just any complex integral. It is specifically used for integrals that are composite functions, where the derivative of the inner function is a factor in the integral, or when there is a constant multiple of the derivative present.
How does the presence of a natural logarithm function affect the choice of substitution?
-When a natural logarithm function is present, the choice of substitution might not be immediately clear. It may require rewriting the expression to highlight a function and its derivative, or using logarithm rules to simplify the expression before applying the substitution technique.
Outlines
😀 Introduction to Integration by Substitution
The video begins by introducing the concept of integration by substitution, a technique used to integrate more complex functions, particularly those derived from the chain rule in differentiation. The chain rule is reviewed, emphasizing the composition of inner and outer functions and their derivatives. The focus is on reversing this process to find anti-derivatives of functions resulting from chain rule differentiation.
📝 Substitution Process and Differentials
The paragraph explains the substitution process in integration by detailing how to replace the inner function with a new variable, u, and subsequently adjusting the differential, dx, to reflect the change in the variable of integration. It also discusses strategies for isolating the differentials du and dx and substituting them into the integral.
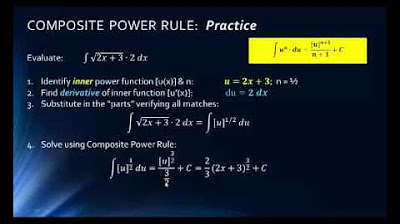
🔍 Identifying Inner and Outer Functions
This section focuses on identifying the inner and outer functions in a composite function and determining the appropriate substitution based on the derivative of the inner function. It provides examples of different functions and how to find their differentials du and dx, which are crucial for the substitution technique.
🧮 Substitution for Exponential and Logarithmic Functions
The video covers how to apply substitution to more complex functions, such as exponential and logarithmic ones. It demonstrates the process of differentiating these functions, finding the appropriate differentials, and simplifying the integral through substitution.
🔗 Substitution Technique Review and Application
A review of the substitution technique is presented, emphasizing the need to identify a nested function and its derivative within the integral. The process of substitution is reiterated, including replacing the inner function with a variable and adjusting the differential. The importance of resubstitution to regain the original variable is highlighted.
🧠 Solving Integrals Using Substitution
The paragraph delves into solving several integrals using the substitution technique. It illustrates how to identify the appropriate substitutions, perform the integration with respect to the new variable, and resubstitute to obtain the final antiderivative in terms of the original variable.
📐 Definite Integrals and Substitution
The video concludes with an example of calculating a definite integral using substitution. It explains that while the strategy remains the same, the limits of integration are applied only after resubstitution to the original variable. The process of evaluating the definite integral by substituting the limits and calculating the difference is demonstrated.
Mindmap
Keywords
💡Substitution Integration Technique
💡Chain Rule
💡Nested Function
💡Anti-Derivative
💡Derivative
💡Constant Multiple
💡Natural Logarithm
💡Definite Integral
💡Differential
💡Power Rule
💡Resubstitution
Highlights
Integration by substitution allows integrating complex functions resulting from differentiation via the chain rule.
The technique works backwards from the derivative to find the original function's anti-derivative.
Identifying the inner and outer functions of a composed function is crucial for successful substitution.
The derivative of the inner function must be present in the integral for substitution to be applicable.
Substitution simplifies the integral by changing the variable of integration and the differential.
After integrating with respect to the new variable, resubstitution is required to revert to the original variable.
For definite integrals, limits of integration are applied after resubstitution and only to the original variable.
The process involves differentiating the substitution expression to find the relationship between du and dx.
Substitution can simplify complex integrals into a form that allows for easier application of anti-derivative rules.
The natural log function can be a nested function where the derivative is also present in the integral, allowing for substitution.
Logarithm rules can be applied to simplify the integral before considering a substitution strategy.
When the derivative of the inner function is a constant multiple of what's present in the integral, substitution is still valid.
The constant of integration is omitted when evaluating definite integrals as it cancels out upon applying limits.
Substitution can be applied to a wide range of integrals, especially those involving exponential and logarithmic functions.
Practicing substitution is essential for recognizing suitable integrals and mastering the technique.
The technique is powerful for integrating new functions that were previously difficult to approach.
The process requires recognizing the structure of the integral, finding the correct substitution, and applying the appropriate rules.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: