Business Calculus - Section 3.5 - Applied Optimization Problems
TLDRThe video script delves into the critical business calculus topic of optimization, focusing on applied optimization problems. It outlines a strategic approach to solving optimization issues, which involves reading the problem, drawing a diagram, defining variables, formulating equations, reducing the equation to a single variable, and using calculus to find the extreme value. The script illustrates this process through several examples, including maximizing the area of a rectangular pen with a fixed perimeter, determining the dimensions and area of the largest possible garden within a budget, and finding the optimal ticket price for a concert to maximize revenue. Additionally, it discusses inventory control, aiming to minimize the total cost of storage and production by finding the optimal order frequency for napkins in a fast-food restaurant. The script provides a comprehensive understanding of optimization, emphasizing its relevance in business decision-making.
Takeaways
- 📈 **Optimization in Business Calculus**: The process of finding the absolute extrema (maximum or minimum) of a situation, often related to profit, cost, or other business metrics.
- 📝 **General Strategy for Optimization**: Follow a step-by-step approach that includes reading the problem, drawing a picture, creating and describing variables, formulating equations, reducing to one variable, finding extreme values, and interpreting results.
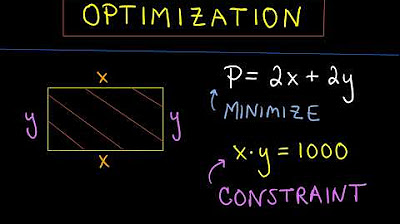
- 🔍 **Variables and Constraints**: Define variables for the dimensions of the problem (like length and width) and identify constraints (such as available fencing or budget).
- 🧵 **Perimeter and Area**: Use the perimeter to relate the length and width of a rectangle, and then express the area in terms of a single variable to optimize.
- 📐 **Second Derivative Test**: Utilize the second derivative to determine if a critical point is a maximum or minimum by checking if the second derivative is negative (concave down) or positive (concave up).
- 💰 **Cost Function Simplification**: Simplify cost functions by combining like terms and expressing them in terms of a single variable to find the optimal solution.
- 📉 **Revenue Maximization**: Adjust variables such as ticket prices to maximize revenue, taking into account the relationship between price and demand.
- 👥 **Inventory Control**: Balance the costs of ordering and storing inventory to minimize total costs, considering factors like demand, storage costs, and ordering fees.
- 🔄 **Uniform Annual Demand**: Account for consistent usage rates when calculating inventory needs, which simplifies the process of determining average inventory levels.
- 📦 **Average Inventory Level**: Calculate the average inventory level by considering the total inventory ordered and the rate of usage.
- 📊 **Cost Function Derivatives**: Use derivatives to find the minimum cost by setting the first derivative of the cost function to zero and verifying with the second derivative.
- ⏱️ **Ordering Frequency**: Determine the optimal frequency of orders to minimize costs by using the derived equations from the cost function and constraints.
Q & A
What is the main topic discussed in the provided transcript?
-The main topic discussed in the transcript is optimization in business calculus, specifically applied optimization problems and how to solve them using calculus.
What is the general strategy for solving an optimization problem?
-The general strategy for solving an optimization problem includes reading the problem, drawing a picture if appropriate, creating variables and describing them, creating the equation to optimize and any constraint equations, reducing the optimization equation to one independent variable, finding the extreme value using the extreme value theorem or the second derivative test, and interpreting the results in terms of the problem.
In the context of the farmer's fencing problem, what is the constraint?
-In the farmer's fencing problem, the constraint is the total amount of fencing available, which is 60 feet. This limits the size of the rectangular pen that can be built.
How does the area of the rectangular pen relate to its length and width?
-The area of the rectangular pen is calculated by multiplying its length (X) by its width (Y), represented by the equation A = X * Y.
What is the maximum area of the pen that the farmer can build with 60 feet of fencing?
-The maximum area of the pen that the farmer can build with 60 feet of fencing is 225 square feet.
In the second example, what are the two different types of fencing costs mentioned?
-In the second example, the two different types of fencing costs mentioned are $12 per foot for the special electric fencing and $4 per foot for the regular barbed wire fencing.
What is the purpose of using calculus to find the optimal ticket price for a concert?
-The purpose of using calculus to find the optimal ticket price for a concert is to maximize the revenue from ticket sales, balancing the price increase with the estimated decrease in attendance.
What is inventory control, and why is it important for a company?
-Inventory control is the management of the balance between the frequency of ordering supplies and the costs associated with storage versus the costs of ordering too frequently or not having enough on hand. It is important for a company to minimize the total cost involved in the ordering and storage process.
How does the fast-food restaurant's inventory control problem relate to the cost of storage and production of napkins?
-The fast-food restaurant's inventory control problem involves finding the optimal number of times per year to order napkins to minimize the total cost of storage and production. This involves balancing the cost of storing napkins with the cost of placing orders for them.
What is the formula for calculating the cost function C in the fast-food restaurant's inventory control problem?
-The formula for calculating the cost function C in the fast-food restaurant's inventory control problem is C = (Storage cost * (Average number of napkins on hand)) + (Ordering cost * Number of orders per year), which simplifies to C = (5 * (1/2 * X)) + (50 * Y), where X is the number of napkins per order and Y is the number of times per year the restaurant orders napkins.
How often should the fast-food restaurant order napkins to minimize the total cost?
-The fast-food restaurant should order napkins approximately 24.5 times per year, which equates to about every 15 days, to minimize the total cost of storage and production.
Outlines
📈 Optimization in Business Calculus
The video begins by emphasizing the significance of optimization in business calculus. It introduces the concept of optimization as finding the absolute extrema (maximum or minimum) of a situation, which is crucial for business applications like maximizing profit or minimizing cost. The presenter outlines a seven-step strategy for solving optimization problems, which includes reading the problem, drawing a diagram, creating variables, formulating equations, reducing the equation to one independent variable, finding the extreme value, and interpreting the results. An example problem is presented where a farmer aims to maximize the area of a rectangular pen using 60 feet of fencing.
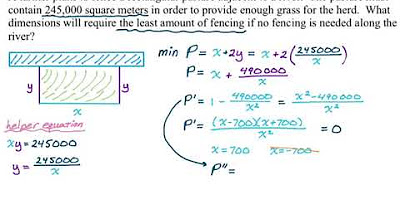
🧵 Cost and Area Optimization for a Rectangular Garden
The second paragraph delves into a problem where a farmer has a budget of $6,000 to build a rectangular garden fence, with one side using special electric fencing costing $12 per foot and the other sides using regular barbed wire at $4 per foot. The goal is to find the dimensions and area of the largest possible garden within the budget. The presenter guides through setting up the cost function, simplifying it, and using calculus to find the critical values that represent the dimensions of the garden. The solution involves finding the maximum area by differentiating the cost function and applying the second derivative test to confirm the maximum.
🎟️ Maximizing Revenue with Variable Ticket Pricing
The third example addresses a scenario where a promotions company is trying to maximize revenue from concert ticket sales. The company estimates that for every $2 increase in ticket price, 12 fewer people will attend. The presenter creates a table to visualize the relationship between price changes, the number of attendees, and revenue. Using calculus, the presenter finds the optimal ticket price that maximizes revenue, taking into account the initial price, the number of price changes, and the resulting number of attendees. The process involves setting up a revenue function, differentiating it, and solving for the critical value to find the maximum revenue and the corresponding number of attendees.
📚 Inventory Control for a Fast-Food Restaurant
The fourth paragraph discusses inventory control, focusing on a fast-food restaurant that needs to manage its annual demand for 12,000 boxes of napkins. The presenter explains the costs associated with storage and ordering, and how inventory control aims to balance these costs. The goal is to minimize the total cost by determining the optimal number of times the restaurant should order napkins per year. The presenter sets up an equation based on the annual demand and the costs, and uses calculus to find the minimum cost, which involves finding the critical point and verifying it as a minimum using the second derivative.
🔍 Detailed Analysis of Inventory Ordering Frequency
The final paragraph provides a detailed analysis of how often the restaurant should order napkins to minimize costs. The presenter interprets the numbers related to the napkins' annual demand and the costs of storage and ordering. The constraint equation is established, and the presenter calculates the average number of napkins on hand. The cost function, comprising storage and ordering costs, is formulated. By solving for one variable in terms of the other using the constraint, the presenter finds the critical value that minimizes the total cost. The second derivative test confirms that this value indeed represents a minimum, leading to the conclusion of how frequently the restaurant should order napkins.
Mindmap
Keywords
💡Optimization
💡Calculus
💡Constraint
💡Perimeter
💡Area
💡Cost Function
💡Revenue
💡Second Derivative Test
💡Inventory Control
💡Uniform Annual Demand
💡Critical Value
Highlights
Optimization is defined as the process of finding the absolute extrema of a situation, which is crucial in business calculus.
Applied optimization problems are solved using calculus to address real-world scenarios like inventory control.
The general strategy for solving optimization problems involves seven steps, from reading the problem to interpreting results.
A farmer uses 60 feet of fencing to build a rectangular pen, aiming to maximize the area with optimization techniques.
The relationship between the length (X) and width (Y) of the pen is derived from the perimeter constraint.
The area function is simplified to a function of a single variable (X) by substituting the relationship between X and Y.
The second derivative test is used to find the extreme value of the optimization equation, indicating a maximum or minimum.
For the fencing problem, the maximum area of 225 square feet is achieved when the length (X) is 15 feet.
A second example involves a farmer with a $6,000 budget for fencing, aiming to maximize the area of a rectangular garden with different fencing costs.
The cost function for the garden is derived from the cost of electric and barbed wire fencing, leading to a constraint equation.
The largest possible garden is found by optimizing the area function, resulting in dimensions and area calculations.
A promotions company uses optimization to find the optimal ticket price for a concert to maximize revenue, considering variable attendance.
A table is created to visualize the relationship between ticket price changes, attendance, and revenue.
The revenue function is maximized using calculus, revealing the optimal ticket price and the corresponding maximum revenue.
Inventory control problems, such as a fast-food restaurant's napkin ordering, are addressed to minimize the total cost of storage and ordering.
The restaurant's napkin ordering problem is solved by finding the optimal number of orders per year, balancing storage and ordering costs.
The cost function for the napkin ordering includes both storage and ordering costs, which are optimized to find the minimum total cost.
The solution to the napkin problem suggests ordering approximately every 15 days to minimize costs, assuming storage costs are higher than ordering costs.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: