Using Factoring to Solve Quadratics (Precalculus - College Algebra 18)
TLDRThe video script provides an in-depth exploration of solving quadratic equations, focusing on the factoring method to find x-intercepts. The presenter introduces the 'diamond method', a technique for factoring quadratics that involves identifying two numbers that multiply to 'ac' (where 'a' is the coefficient of the x^2 term and 'c' is the constant term) and add up to the coefficient of the x term ('b'). The script emphasizes that factoring is not always possible, but when it is, it offers a fast and efficient way to find real solutions. The presenter also discusses the limitations of the square root method and when it's applicable, as well as the concept of the zero product property. The video aims to clarify common misunderstandings about factoring quadratics and equips viewers with a reliable method to find x-intercepts, ensuring a solid grasp of the topic.
Takeaways
- 📚 Start by setting the quadratic equation equal to zero to find x-intercepts, which are the points where the parabola crosses the x-axis.
- 🔍 If the quadratic is in order and the first term is positive, it's ready for factoring.
- ✅ Attempt to factor out the greatest common factor (GCF) first, as this can simplify the equation and sometimes reveal additional solutions.
- 🅰️ Use the diamond method to find two numbers that add up to the coefficient of the x term (B) and multiply to the product of the coefficients of x² and the constant term (AC).
- 💡 If the GCF is not present, the diamond method helps in splitting the middle term to apply factoring by grouping.
- ⛔ The square root method isn't always applicable; it works when a perfect square trinomial can be isolated.
- 🔁 Changing the sign of the leading coefficient to make it positive does not affect the x-intercepts because reflecting over the x-axis doesn't alter the x-values.
- 📉 For a quadratic equation in the form of ax² + bx + c = 0, if 'a' is not 1, you can't directly apply the shortcut method of changing signs to find solutions.
- 📈 The complete shortcut involves dividing the numbers found using the diamond method by the coefficient 'a' of x², then changing the sign to find solutions.
- 🤔 Always be cautious of sign errors when factoring and applying the zero product property; ensure the correct signs are maintained throughout the process.
- ✨ The technique demonstrated is a powerful method for quickly finding x-intercepts without having to use more complex methods like completing the square or the quadratic formula.
Q & A
What is the focus of this video?
-The focus of this video is to teach how to solve quadratics and find x-intercepts using factoring techniques, specifically the diamond method.
Why is it important to set the quadratic equation to zero when finding x-intercepts?
-Setting the quadratic equation to zero is important because it allows us to find the x-values where the function intersects the x-axis, which are the x-intercepts or roots of the quadratic.
What is the zero product property mentioned in the video?
-The zero product property states that if a product of two factors equals zero, then at least one of the factors must be zero. This property is used to find the solutions to the quadratic equation once it is factored.
How does the diamond method help in factoring quadratics?
-The diamond method is a graphic organizer that helps visualize the numbers needed to factor a quadratic. It aids in finding two numbers that add up to the coefficient of the x-term and multiply to the product of the coefficient of the x^2 term and the constant term.
Why might the square root method not be applicable to every quadratic equation?
-The square root method may not be applicable if the quadratic equation cannot be simplified to have all x terms raised to the power of 2 on one side and a constant on the other side.
What is the shortcut mentioned for finding x-intercepts if the leading coefficient (a) is 1?
-If the leading coefficient (a) is 1, you can directly find the x-intercepts by changing the signs of the numbers found through the diamond method, as these numbers represent the solutions to the quadratic equation.
What is the significance of the parabola's direction (upward or downward opening) in relation to the x-intercepts?
-The direction of the parabola indicates whether the parabola will cross the x-axis at one or two points (x-intercepts). An upward-opening parabola will cross the x-axis twice, while a downward-opening parabola will also cross twice but at different x-values.
How does the video address the issue of shortcuts not working all the time?
-The video emphasizes that a good shortcut should work all the time. It discusses why certain shortcuts may fail in specific scenarios and provides a complete shortcut that works for any quadratic equation when factoring is possible.
What is the role of the greatest common factor (GCF) in factoring quadratics?
-The GCF, if present, should be factored out first as it simplifies the quadratic equation. This can sometimes reveal additional x-intercepts, such as when the GCF includes an x-term leading to an x-intercept of zero.
Why is it suggested to have the first term of the quadratic positive when factoring?
-Having the first term positive simplifies the factoring process and makes it easier to apply certain techniques, such as the diamond method, which relies on the signs of the numbers that are found.
What is the complete shortcut for finding the factors or solutions of a quadratic equation with a leading coefficient of 1?
-The complete shortcut involves finding two numbers that add up to the middle coefficient (B) and multiply to the product of the leading coefficient (A) and the constant term (C), then dividing these numbers by the leading coefficient (which is 1 in this case), and finally changing the signs to find the solutions.
Outlines
😀 Introduction to Factoring Quadratics
The video begins with an introduction to solving quadratic equations by focusing on factoring. The presenter emphasizes that there are multiple ways to factor quadratics, but they will present the most effective method they've encountered. The purpose is to find x-intercepts or the points where the parabola crosses the x-axis. The video also mentions that factoring won't yield imaginary solutions and may require the use of the quadratic formula or completing the square for such cases.
🔍 Setting up Quadratics for Factoring
The presenter explains the importance of setting up the quadratic equation properly for factoring. This involves ensuring the equation is set equal to zero, organizing terms in descending order (x^2, x, constant), and starting with a positive leading term. The video also introduces the 'diamond method' as a means to visualize and find the correct numbers to facilitate factoring.
📚 Factoring by Grouping and Zero Product Property
The video delves into the process of factoring by grouping, which involves splitting the middle term to create four terms instead of three, allowing for grouping and factoring. The zero product property is also discussed, stating that if a product of factors equals zero, at least one of the factors must be zero. This leads to finding the x-intercepts where the parabola crosses the x-axis.
🚫 Limitations of the Square Root Method
The presenter discusses the limitations of the square root method for solving quadratics, explaining that it is not always applicable. The method requires a term raised to the second power containing all x's, which is not present in the given example. The video suggests that if the square root method is not feasible, the next step is to try factoring.
🔢 Factoring with Greatest Common Factor (GCF)
The video highlights the importance of checking for a greatest common factor (GCF) before attempting to factor a quadratic. Factoring out the GCF can simplify the quadratic and sometimes reveal additional solutions. The presenter demonstrates how to factor out the GCF and proceed with factoring the remaining expression.
🤔 Understanding the Diamond Method for Factoring
The presenter continues to elaborate on the diamond method, emphasizing the need to find two numbers that add up to the coefficient of the x term and multiply to the product of the leading coefficient and the constant term. The video clarifies that these numbers must be found carefully, as incorrect numbers will lead to an unsuccessful factoring attempt.
📉 Applying Factoring to Downward-Opening Parabolas
The video addresses the process of factoring quadratics that represent downward-opening parabolas. The presenter demonstrates how to rearrange the equation to have a positive leading term and how to apply the factoring techniques previously discussed. The concept of x-intercepts and their relation to the parabola's intersection with the x-axis is reiterated.
🔁 Factoring and the Zero-Product Property
The presenter explains that the zero-product property allows for solving quadratics by setting each factor equal to zero. This approach is valid for finding x-intercepts and provides real solutions if the quadratic is factorable. The video also touches on the concept of double roots, where a parabola touches the x-axis at the same point, resulting in the same solution being found twice.
📐 Factoring and the Complete Shortcut
The video introduces a complete shortcut for factoring quadratics, which involves dividing the numbers found using the diamond method by the leading coefficient (a) and then changing the signs to find the solutions. The presenter assures that this shortcut will always yield the correct factors and solutions, providing a fast and efficient method for solving quadratics.
🎯 Factoring Special Cases and Relating Techniques
The presenter discusses special cases in factoring, such as when the quadratic equation does not factor easily or when it represents a parabola that has a double root. The video emphasizes the importance of understanding how different factoring techniques relate to one another and how they can be adapted to various situations. The concept of a parabola being shifted and its impact on the x-intercepts is also explained.
✅ Wrapping Up Factoring Techniques
The video concludes with a summary of the factoring techniques presented and their effectiveness. The presenter expresses hope that the new technique shared will be beneficial for the viewers and sticks with them. An overview of the next topic, completing the square, is provided, with a promise to explain why it works and to offer an easy method for it in the next video.
Mindmap
Keywords
💡Quadratics
💡Factoring
💡X-intercepts
💡Square Root Method
💡Completing the Square
💡Zero Product Property
💡Greatest Common Factor (GCF)
💡Diamond Method
💡Parabola
💡Vertex
💡Difference of Squares
Highlights
The video introduces a technique for solving quadratics by focusing on factoring, specifically the 'Diamond Method'.
The presenter emphasizes the importance of setting the quadratic equal to zero and having terms in order for factoring to work effectively.
It is highlighted that factoring will not yield imaginary solutions, suggesting the use of the quadratic formula or completing the square for such cases.
The video demonstrates how to determine if the square root method is applicable to a given quadratic before attempting to factor.
Completing the square is introduced as an alternative technique to make a quadratic factorable when the square root method is not applicable.
The Diamond Method simplifies the process of finding two numbers that add up to the coefficient of the x term and multiply to the product of a and c.
The presenter uses the Diamond Method to transform a trinomial into a set of binomials that can be factored by grouping.
The video clarifies that the Diamond Method relies on the zero product property to find x-intercepts of quadratic equations.
The shortcut for factoring quadratics when 'a' is one is explained, showing how to quickly find factors and solutions.
The video addresses the misconception that some quadratics are not factorable and demonstrates that they can still be factored using square roots.
The presenter cautions against using shortcuts that do not work all the time and advocates for a complete understanding of factoring techniques.
A complete shortcut is introduced that works for all quadratics, involving dividing by 'a' and simplifying to find x-intercepts.
The video shows how to handle quadratics that have a double root, indicating the vertex of the parabola touches the x-axis at a single point.
Different ways to express the factors of a quadratic are discussed, including variations like 'X minus 3 halves' and '2x minus 3'.
The concept of shifting parabolas and how it affects x-intercepts is explained, tying together graphing, vertex, and x-intercepts.
The video concludes with a discussion on factoring quadratics that are not perfect squares over integers but can still be factored using square roots.
The presenter assures viewers that all quadratics can be factored and provides encouragement for applying the techniques learned to solve a variety of quadratic problems.
Transcripts
Browse More Related Video

The Square Root Method in Solving Quadratics (Precalculus - College Algebra 17)
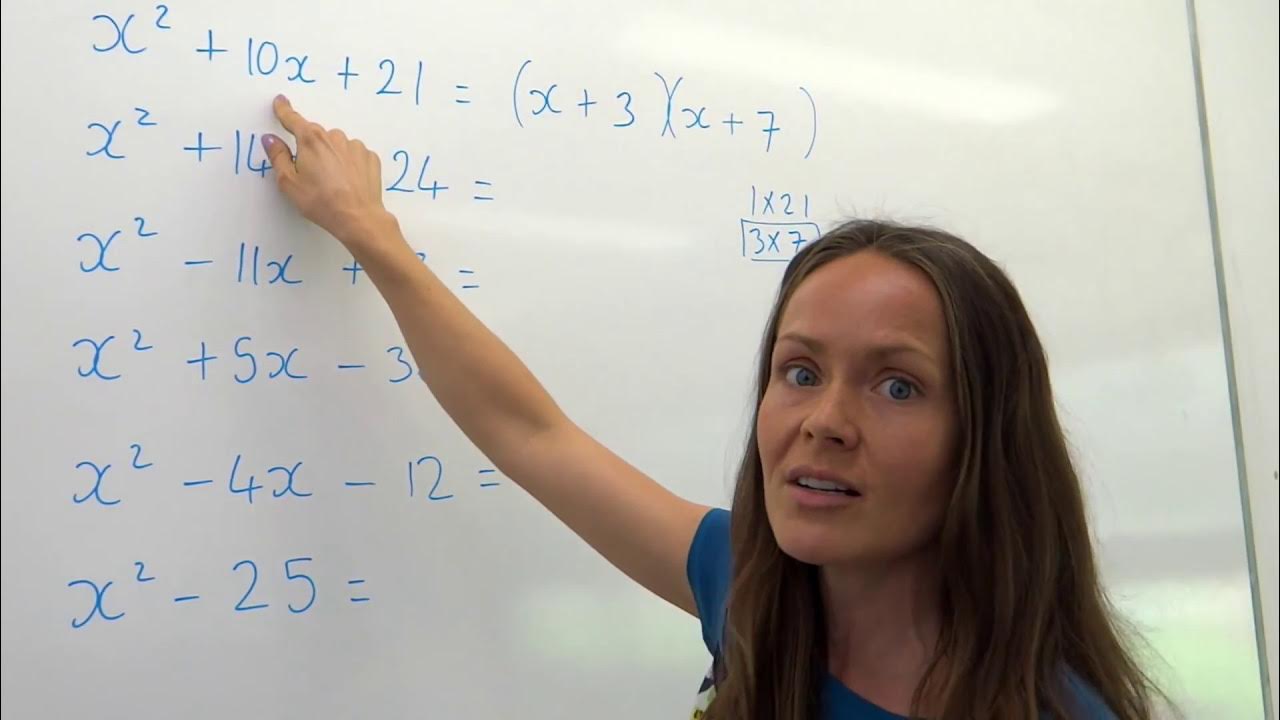
The Maths Prof: Factorising Quadratics (putting into double brackets)

Introduction to Solving Quadratics (Precalculus - College Algebra 16)

Factoring Trinomials - Quick & Simple
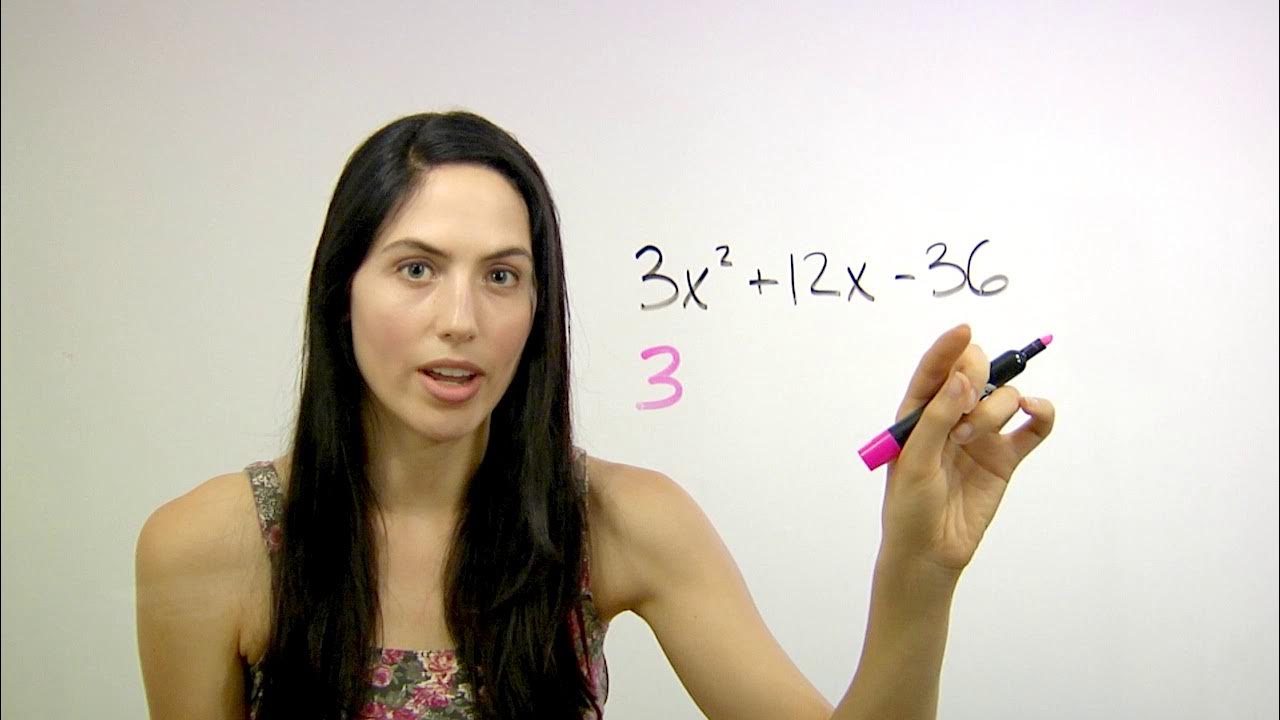
Factoring Quadratics... How? (NancyPi)
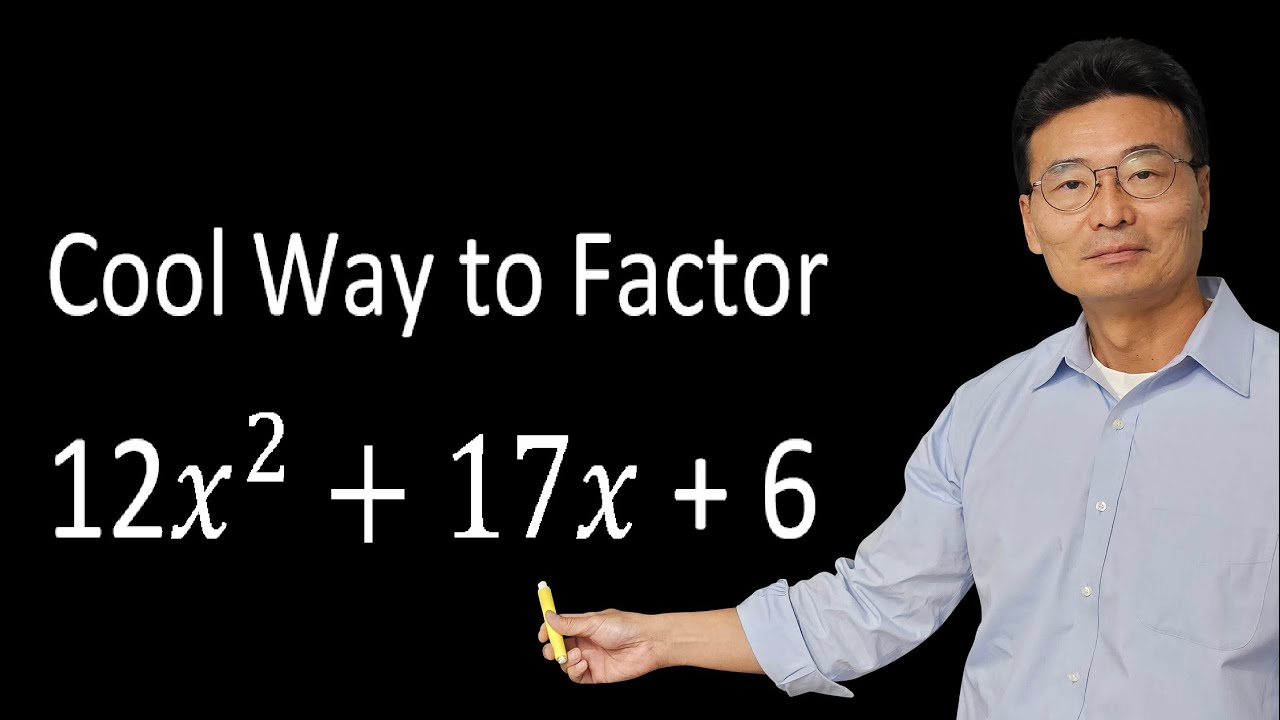
Factoring Trinomials
5.0 / 5 (0 votes)
Thanks for rating: