Calculating gravitational potential energy | Modeling energy | High school physics | Khan Academy

Khan Academy
12 Jul 202104:32
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video delves into the concept of gravitational potential energy, using a 5 kg mass positioned 10 meters above the Earth's surface as an example. It explains how the potential energy, calculated as mass times the gravitational constant (g) times height, amounts to 490 joules in this scenario. The video illustrates the concept by asking viewers to consider the work required to lift the mass against gravity and how this energy is stored and can be released to do work, such as lifting another mass. The emphasis is on understanding the relationship between force, work, and energy in the context of a gravitational field.

Takeaways
  • 🌟 Energy is the capacity to do work, with various types including kinetic and potential energy.
  • 📈 Potential energy is related to an object's position, specifically its height in a gravitational field.
  • 🌐 Gravitational potential energy depends on the object's position relative to Earth's surface and the gravitational constant (g).
  • 🔢 The formula for calculating gravitational potential energy is mass (kg) times gravitational field constant (g) times height (m).
  • 🎯 The value of g is approximately 9.8 meters per second squared near the Earth's surface.
  • 💡 Gravitational potential energy is measured in joules, the same unit used for work and force times distance.
  • 🏋️ Lifting an object against gravity requires work, equivalent to the object's weight (mass times g) times the height.
  • 🔄 The work done to lift an object can be stored as potential energy and later converted back to work.
  • 🔄 An example of energy conversion is using a pulley system where a lifted mass can do work on another object.
  • 📚 Understanding the concept of gravitational potential energy helps in grasping the relationship between force, work, and energy.
  • 🌍 The value of g can change with distance from Earth, but it is considered constant near the surface for simplification in calculations.
Q & A

  • What is the definition of energy as discussed in the script?

    -Energy is defined as the capacity to do work.

  • What are the two types of energy mentioned in the script?

    -The two types of energy mentioned are kinetic energy, which is energy due to motion, and potential energy, which is energy due to position.

  • What is gravitational potential energy?

    -Gravitational potential energy is the potential energy an object has due to its position in a gravitational field.

  • How is the height related to gravitational potential energy?

    -The height of an object in a gravitational field contributes to its potential energy. The higher the object, the greater its potential energy.

  • What is the formula for calculating gravitational potential energy?

    -The formula for calculating gravitational potential energy is mass (m) times the gravitational field constant (g) times height (h), or mgh.

  • What is the approximate value of g for Earth's gravitational field near the surface?

    -The approximate value of g for Earth's gravitational field near the surface is 9.8 meters per second squared.

  • What units are used to express energy and work in the script?

    -Energy and work are expressed in joules.

  • How does the script illustrate the concept of work in relation to potential energy?

    -The script illustrates this by explaining that lifting an object against gravity requires work, which is equal to the object's weight times the height. This work done is stored as potential energy.

  • What is the relationship between the mass of an object, its height, and the work done to lift it?

    -The work done to lift an object is directly proportional to its mass and the height it is lifted. The greater the mass and the higher the height, the more work is required.

  • How can the potential energy of an object be converted back into work?

    -The potential energy can be converted back into work by allowing the object to fall; the object's weight times the distance fallen will result in an equivalent amount of work being done.

  • What is the significance of the pulley system example in the script?

    -The pulley system example demonstrates how potential energy can be transferred and used to do work. When one mass falls, its potential energy is converted into work, which lifts another mass by the same height.

Outlines
00:00
🌟 Understanding Gravitational Potential Energy

This paragraph introduces the concept of gravitational potential energy, which is the potential energy an object possesses due to its position within a gravitational field. The instructor uses the example of a 5-kilogram mass positioned 10 meters above the Earth's surface to explain how to calculate the difference in potential energy compared to when the object is on the surface. The formula for gravitational potential energy is given as mass times the gravitational field constant (g) times height (h). The value of g is approximated as 9.8 meters per second squared, and the calculation results in the potential energy being measured in joules, which is also the unit for work. The instructor reinforces the concept by discussing the work required to lift the mass against gravity and how this energy is then stored and can be used to do work, such as lifting another mass through a pulley system.

Mindmap
Keywords
💡Energy
Energy is defined as the capacity to do work, and it is a fundamental concept in physics. In the context of the video, it is used to describe the potential for a physical system to perform work, specifically through the motion or position of an object. The video discusses various types of energy, emphasizing the focus on gravitational potential energy as it relates to an object's position within a gravitational field, such as that of the Earth.
💡Kinetic Energy
Kinetic energy is the energy that an object possesses due to its motion. It is directly proportional to the mass of the object and the square of its velocity. In the video, kinetic energy is introduced as one of the multiple types of energy discussed, contrasting it with potential energy, which is not dependent on motion but rather on an object's position or condition.
💡Potential Energy
Potential energy is the stored energy of an object due to its position relative to other objects or forces acting upon it. In the video, potential energy is highlighted by discussing its relationship with an object's position, specifically in a gravitational field. Gravitational potential energy is the focus, which is the energy an object has because of its elevated position relative to the Earth's surface.
💡Gravitational Potential Energy
Gravitational potential energy is a specific type of potential energy that an object possesses because of its position in a gravitational field, such as that of the Earth. It is dependent on the object's mass, the acceleration due to gravity, and its height relative to a reference point. The video delves into the calculation of gravitational potential energy and how it relates to the work done in lifting an object against gravity.
💡Mass
Mass is a measure of the amount of matter in an object, and it is a fundamental property that determines the strength of its gravitational interaction with other objects. In the context of the video, mass is a crucial factor in calculating both kinetic and potential energies, particularly gravitational potential energy, as it influences the force of gravity acting upon the object.
💡Height
Height refers to the vertical distance of an object above a reference point, such as the Earth's surface. In the video, height is a key factor in determining the amount of gravitational potential energy an object has. The higher the object, the greater the potential energy it possesses due to its elevated position in the gravitational field.
💡Gravitational Field
A gravitational field is the region around a massive object, such as the Earth, where the force of gravity acts upon other objects. The strength of the gravitational field near the Earth's surface is represented by the acceleration due to gravity (g). The video explains how gravitational potential energy is influenced by the position of an object within this field.
💡Work
Work is a measure of energy transfer that occurs when a force is applied over a distance. In the context of the video, work is related to the energy required to move an object against the force of gravity, which is equivalent to the gravitational potential energy when the object is lifted to a certain height.
💡Joules
Joules are the unit of measurement for work and energy in the International System of Units (SI). They represent the amount of work done when a force of one newton displaces an object by one meter in the direction of the force. In the video, the unit joules is used to quantify both the work done and the potential energy of the object in the gravitational field.
💡Acceleration Due to Gravity (g)
Acceleration due to gravity (g) is the acceleration that an object experiences when it is in free fall near the Earth's surface, approximately 9.8 meters per second squared. It is a constant value used in the calculation of gravitational potential energy, as it represents the gravitational force acting on an object per unit mass.
💡Weight
Weight is the force exerted on an object due to gravity. It is calculated as the product of an object's mass and the acceleration due to gravity (g). In the video, the weight of the object is related to the force that must be applied to lift it against gravity and is directly tied to the concept of gravitational potential energy.
Highlights

The concept of energy as the capacity to do work is introduced.

Multiple types of energies are discussed, including kinetic and potential energy.

Potential energy is defined as energy due to an object's position.

Gravitational potential energy is explored, which is related to an object's position in a gravitational field.

An example is given of a 5 kg mass 10 meters above the Earth's surface to illustrate the concept.

The formula for gravitational potential energy is presented as mass times gravitational field constant times height.

The value of g, the gravitational field constant, is approximated as 9.8 meters per second squared.

Calculating the potential energy involves multiplying the mass by the gravitational constant and the height.

The units of energy and work are discussed, which are expressed in joules.

The concept of work is related to lifting an object against gravity and the force required.

The weight of an object is defined as its mass times the gravitational field constant.

The potential energy of an object lifted in a gravitational field is equal to the work done to lift it.

A practical example is given of how the potential energy can be visualized and utilized through a pulley system.

The potential energy calculation for a 5 kg mass lifted 10 meters is worked out to be 490 joules.

The concept of an object's potential energy being relative to its position is emphasized.

The idea that potential energy can be converted into work is clarified through the example of the mass and pulley system.

The importance of understanding the relationship between an object's weight, height, and the work done is highlighted.

Transcripts

Browse More Related Video

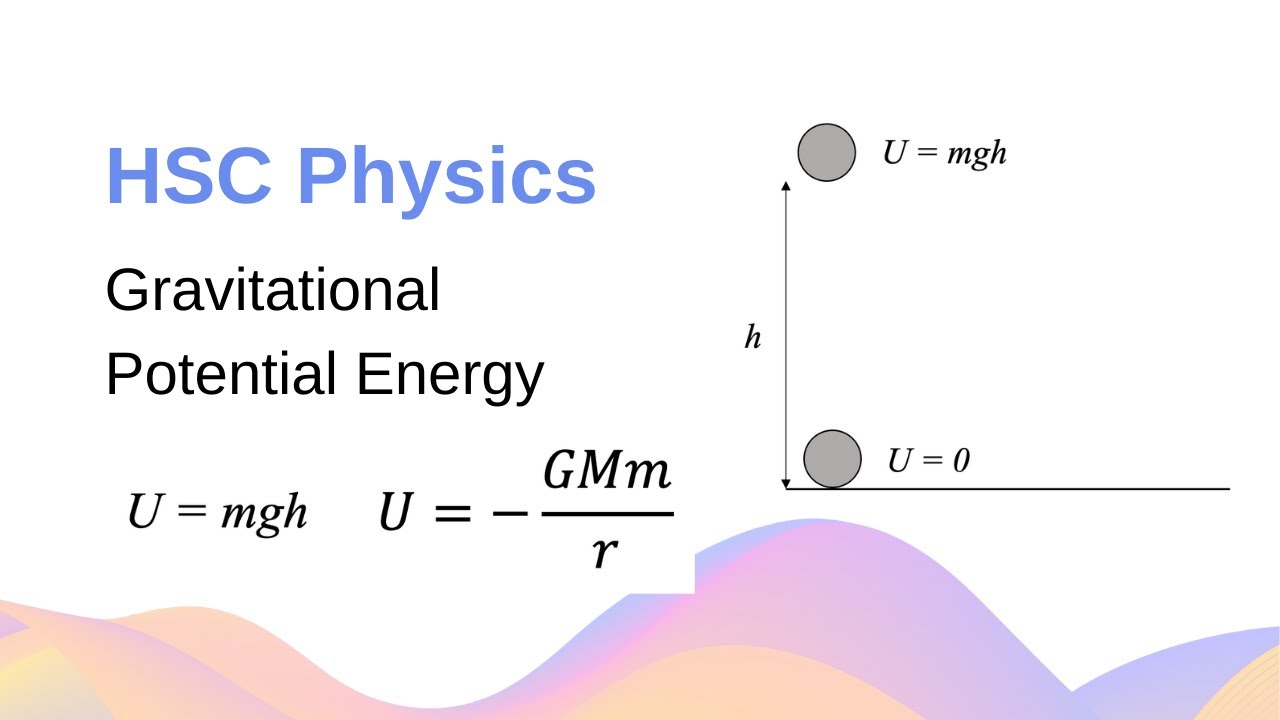
Gravitational Potential Energy & Work Done + Calculation Example // HSC Physics

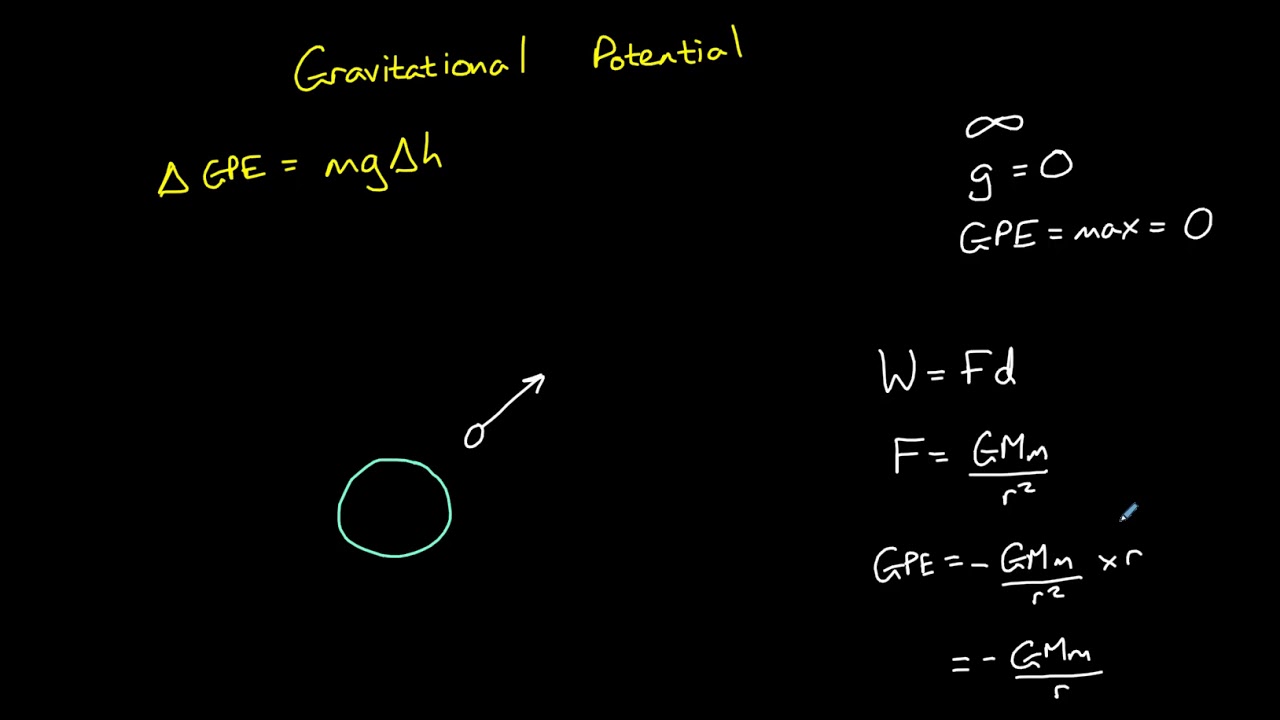
Gravitational Potential and Gravitational Potential Energy

Gravitational Potential Energy Calculations (GPE) - Mass x Gravity x Height OR GPE=mgh

13.3a Gravitational Potential Theory | A2 G-Fields | Cambridge A Level Physics

GCSE Physics - Gravity, Weight and GPE #3

Gravitational Potential Questions | A Level Physics | Formulas, Key Words, Graphs

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Energy ConceptsPhysics EducationGravitational FieldWork and EnergyKinetic vs PotentialScientific CalculationsMechanical WorkEarth's GravityPhysics PrinciplesLifting ObjectsEnergy Storage