How to Remember/Derive the Kinematics Equations

Tangerine Education
2 Feb 201810:01
EducationalLearning
32 Likes 10 Comments

TLDRThis script offers an insightful breakdown of the four fundamental kinematic equations used in physics, focusing on how to memorize and derive them effectively. It explains the relationship between velocity, acceleration, displacement, and time, using clear examples and graphical representations. The video simplifies complex concepts by demonstrating how to rearrange and combine the equations to solve for various variables, ultimately emphasizing the importance of understanding these principles for a solid grasp of kinematics.

Takeaways
  • 📚 The four fundamental kinematic equations are essential for understanding physics.
  • 🧠 Memorizing these equations can be facilitated by understanding and applying pre-existing knowledge.
  • 🔄 The first kinematic equation relates final velocity (v) to initial velocity (u), acceleration (a), and time (t): v = u + at.
  • 📈 Acceleration is the slope of the velocity vs. time graph, which can be expressed as a = (v - u) / t.
  • 🔄 The second equation describes displacement (Δx) as the average velocity (v_avg) times time (t): Δx = v_avg * t, where v_avg = (u + v) / 2.
  • 🏠 Displacement can also be visualized as the area under the velocity vs. time graph, calculated as (base * height) / 2.
  • 🔄 The third equation connects displacement (Δx) with initial velocity (u), acceleration (a), and time (t): Δx = u * t + 1/2 * a * t^2.
  • 🔄 By substituting known equations, we can derive that the final velocity squared (v^2) equals the initial velocity squared (u^2) plus twice the product of acceleration and displacement (2aΔx).
  • 🔄 The fourth equation is derived by eliminating the time variable and is expressed as: (v^2 - u^2) = 2aΔx.
  • 📊 The kinematic equations can be visualized and proven using graphs, such as the velocity vs. time graph.
  • 💡 Understanding and applying these kinematic equations is crucial for solving problems in physics involving motion and displacement.
Q & A

  • What are the four kinematic equations discussed in the transcript?

    -The four kinematic equations discussed are: 1) Final velocity = initial velocity + acceleration × time, 2) Displacement (ΔX) = (V₀ + Vₕ) / 2 × time (T), 3) ΔX = V₀ × T + 0.5 × a × T², and 4) Vₕ² = V₀² + 2a(ΔX).

  • How is acceleration defined in the context of the first kinematic equation?

    -Acceleration is defined as the slope of a velocity versus time graph, or equivalently, as the change in velocity over the change in time (acceleration = ΔV / ΔT).

  • What does the second kinematic equation indicate about average velocity?

    -The second kinematic equation indicates that displacement is equal to the average velocity times time. The average velocity is calculated as (initial velocity + final velocity) / 2.

  • How can you derive the third kinematic equation from the first two?

    -By eliminating the final velocity (Vₕ) from the first two equations and rearranging the terms, you can derive the third equation: ΔX = V₀ × T + 0.5 × a × T².

  • What is the significance of the fourth kinematic equation, Vₕ² = V₀² + 2a(ΔX)?

    -The fourth kinematic equation relates the final velocity squared to the initial velocity squared, acceleration, and displacement. It is useful for solving problems where the relationship between these quantities is needed.

  • How does the transcript explain the concept of change in a variable?

    -The transcript explains the concept of change as the difference between the final and initial values of a variable, such as change in velocity (ΔV) being final velocity minus initial velocity.

  • What is the method used in the transcript to help memorize the kinematic equations?

    -The method used is to understand and derive the equations using known concepts and relationships, such as the slope of a graph and the average of two numbers, to build a deeper understanding and facilitate memorization.

  • How can the area under a velocity vs. time graph be used to find displacement?

    -The area under the velocity vs. time graph can be used to find displacement by calculating the area of the shape formed by the graph, the time axis, and the initial velocity line. For example, if the shape is a triangle, the displacement is equal to (base × height) / 2.

  • What is the role of the distributive property in simplifying the third kinematic equation?

    -The distributive property is used to simplify the third kinematic equation by breaking it down into simpler terms, which allows for easier manipulation and substitution to derive the final form of the equation (ΔX = V₀T + 0.5aT²).

  • How does the transcript demonstrate the process of solving for time in the second kinematic equation?

    -The transcript demonstrates solving for time by isolating it on one side of the equation. By dividing both sides of the equation ΔX = (V₀ + Vₕ) / 2 × T by (V₀ + Vₕ) / 2, time (T) is found to be equal to 2ΔX / (V₀ + Vₕ).

  • What is the final step in deriving the fourth kinematic equation?

    -The final step in deriving the fourth kinematic equation is to add initial velocity to both sides of the derived equation (Vₕ² - V₀² = 2aΔX) to get the final form: Vₕ² = V₀² + 2aΔX.

Outlines
00:00
📚 Introduction to Kinematics Equations

This paragraph introduces the four fundamental kinematics equations essential for understanding physics. It emphasizes the importance of memorizing these equations and presents a method to derive them using known variables. The first equation discussed is the final velocity equation, which relates initial velocity, acceleration, and time. The explanation includes the concept of acceleration as the slope of a velocity-time graph and the derivation of the equation through rearranging known relationships.

05:05
📈 Derivation and Application of Displacement Equations

The second paragraph delves into the derivation and application of displacement equations. It explains the concept of average velocity and how it relates to displacement, using a velocity-time graph for illustration. The paragraph provides a step-by-step breakdown of how displacement can be calculated as the area under the velocity-time curve. It also demonstrates how to derive the equation for displacement in terms of initial velocity, acceleration, and time by combining and simplifying the previously discussed equations.

Mindmap
Keywords
💡Kinematics
Kinematics is the branch of physics that deals with the motion of objects without considering the forces that cause the motion. In the context of the video, it is the foundation for understanding the equations that describe the relationship between different variables like velocity, acceleration, time, and displacement. The script discusses four key kinematic equations that are essential for analyzing motion in physics problems.
💡Velocity
Velocity is a physical quantity that describes the rate of change of an object's position with respect to time. It is a vector quantity, meaning it has both magnitude and direction. In the video, velocity is used to describe the speed at which an object is moving at different points in time, and it is a key variable in the kinematic equations that are being discussed.
💡Acceleration
Acceleration is the rate of change of velocity of an object with respect to time. It is a vector quantity that describes how quickly an object speeds up, slows down, or changes direction. In the video, acceleration is crucial for understanding how the velocity of an object changes over time and is a component of the kinematic equations that relate velocity, time, and displacement.
💡Time
Time is a fundamental physical quantity that measures the duration of events and the intervals between them. In the context of the video, time is a variable that is used in conjunction with velocity and acceleration to calculate displacement and other motion-related quantities. It is a crucial factor in the kinematic equations that describe how an object's state of motion changes over a period.
💡Displacement
Displacement refers to the change in position of an object and is a vector quantity that has both magnitude and direction. It is different from distance traveled, as it takes into account the direction of motion. In the video, displacement is one of the key variables used in the kinematic equations to describe the overall change in position of an object over a period of time.
💡Average Velocity
Average velocity is defined as the total displacement divided by the total time taken for that displacement. It provides a measure of the average speed of an object over a given time interval or distance. In the video, average velocity is used to simplify the calculation of displacement and is a key concept in understanding the relationship between velocity, time, and displacement.
💡Change in Velocity
Change in velocity, often denoted as ΔV, refers to the difference between the final velocity (Vₕ) and the initial velocity (V₀) of an object. It is a measure of how much an object's velocity has changed over a given time period and is a fundamental concept in kinematics, as it is directly related to acceleration.
💡Slope
In mathematics, the slope of a line is a measure of its steepness or gradient. In the context of the video, the slope of a velocity versus time graph is used to define acceleration, as the slope represents the rate of change of velocity with respect to time.
💡Graph
A graph is a visual representation of data or mathematical functions, where individual data points or function values are plotted on a coordinate system. In the video, graphs are used to illustrate the relationships between physical quantities such as velocity, acceleration, and time, making it easier to visualize and understand the concepts of kinematics.
💡Area Under the Line
The area under a line on a graph represents the accumulated value over the interval defined by the line. In the context of the video, the area under a velocity-time graph corresponds to the displacement of an object, as the area represents the total change in position over time.
💡Distributive Property
The distributive property is a fundamental arithmetic rule that states that the product of a number and the sum of two other numbers is equal to the sum of the products of the number and each addend. In the video, this property is used to simplify and rearrange the kinematic equations, making them easier to understand and apply.
Highlights

Four kinematics equations are discussed, providing a foundational understanding for physics.

The method to memorize kinematics equations efficiently is introduced, building on pre-existing knowledge.

The first kinematics equation relates final velocity, initial velocity, acceleration, and time.

Acceleration is defined as the slope of a velocity-time graph, offering a geometric interpretation.

Change in velocity is quantified as the difference between final and initial velocities.

The second kinematics equation connects displacement, average velocity, and time, with a practical example provided.

Displacement is calculated as the area under the velocity-time graph, demonstrated with a specific example.

The third kinematics equation is derived by eliminating the final velocity, showcasing algebraic manipulation.

The relationship between displacement, initial velocity, acceleration, and time is further explored and simplified.

The fourth kinematics equation is derived by eliminating the time variable, highlighting the power of substitution and algebraic simplification.

A comprehensive understanding of kinematics equations is achieved through the derivation and application of these fundamental relationships.

The video content serves as a valuable resource for anyone needing to understand or review kinematics equations.

The use of graphical representations, such as the velocity-time graph, aids in the visualization and comprehension of the concepts.

The practical application of these equations is emphasized through the use of real-world examples and scenarios.

The video provides a step-by-step approach to deriving and understanding the kinematics equations, making it accessible to a wide range of learners.

The final equation derived, relating final velocity squared to initial velocity squared, acceleration, and displacement, encapsulates the essence of the kinematics discussion.

The transcript serves as a detailed guide for anyone looking to deepen their understanding of the fundamental principles of physics.

Transcripts

Browse More Related Video

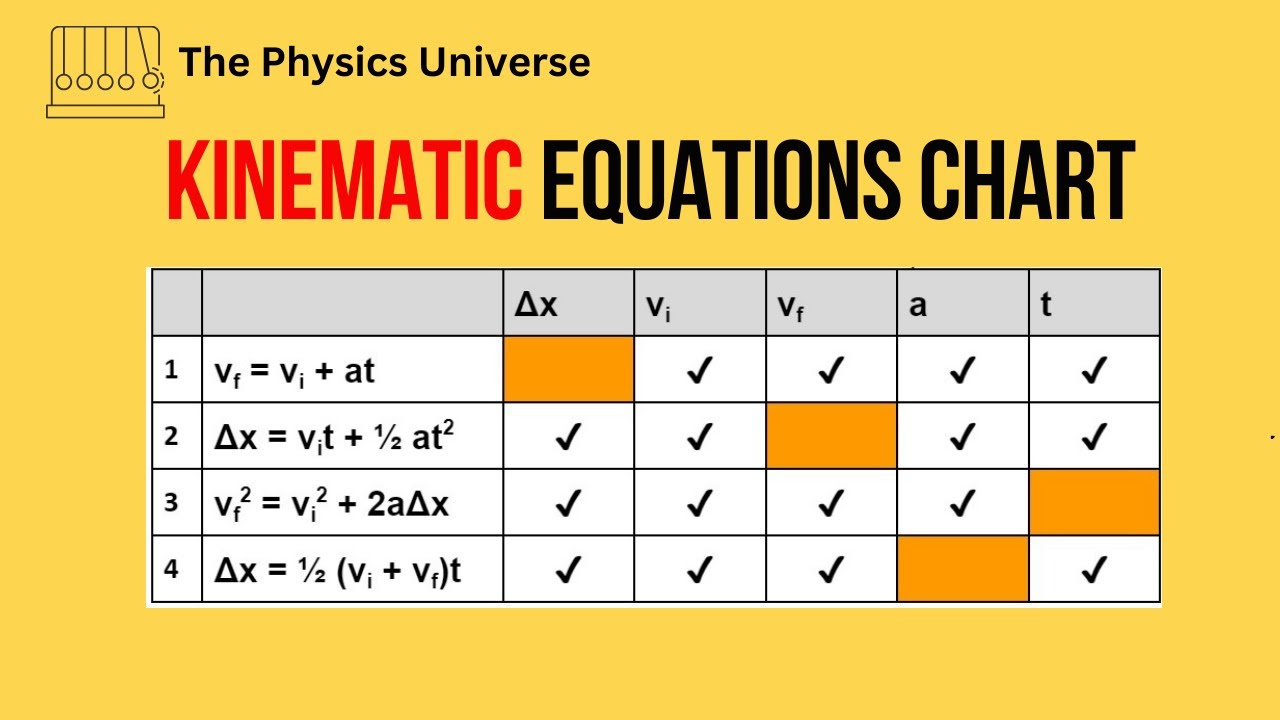
Creating And Using Kinematic Equations Chart - Kinematics - Physics

AP Physics 1 Kinematics Review

Deriving Kinematic Equations (UPDATED) - Kinematics - Physics

How to Cram Kinematics in 1 hour for AP Physics 1

Position/Velocity/Acceleration Part 2: Graphical Analysis

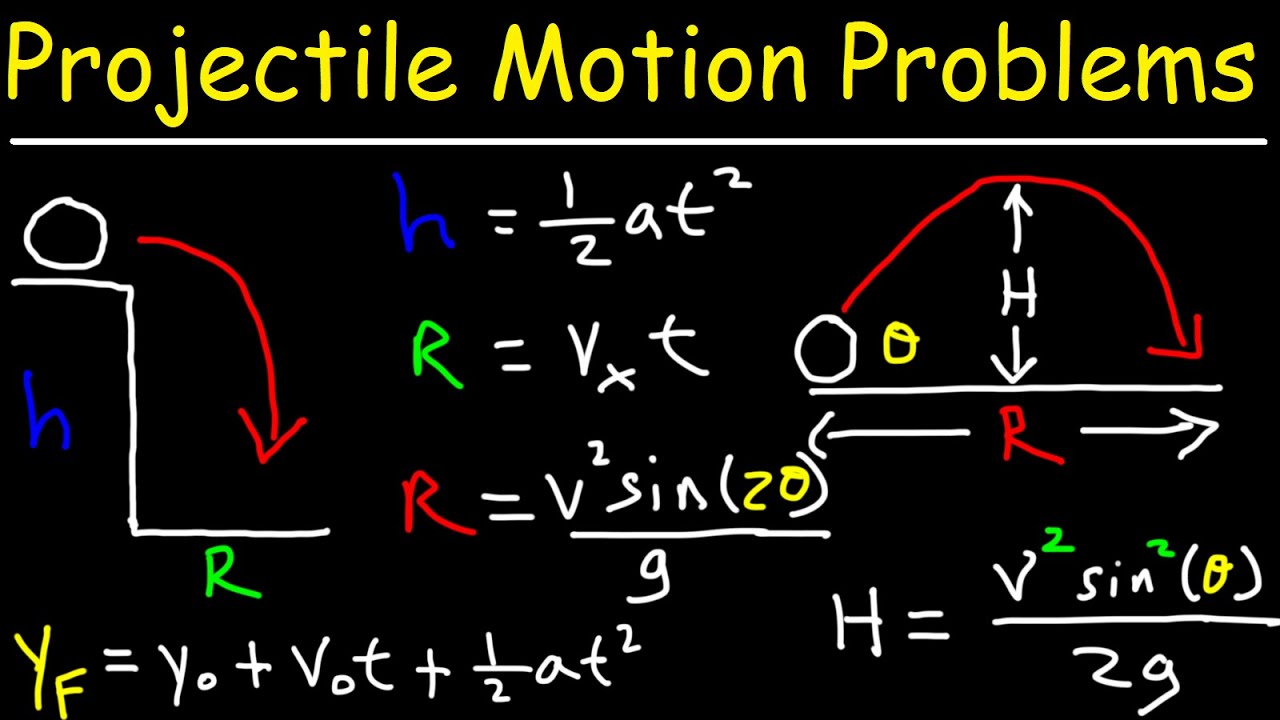
How To Solve Projectile Motion Problems In Physics

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Physics EducationKinematics FormulasVelocity EquationsAcceleration ConceptsDisplacement CalculationLearning TechniquesPhysics ProblemsMathematical DerivationsEducational ContentScience Tutorial