What is a DIFFERENTIAL EQUATION?? **Intro to my full ODE course**
TLDRThis video introduces the concept of differential equations, powerful tools for modeling the physical world, emphasizing their relevance in various scenarios like bank accounts, pandemics, and Newton's second law. The video explains that differential equations often have multiple solutions, which can be determined with initial conditions. It also highlights the importance of calculus in understanding rate of change and the potential for future exploration of solving these equations.
Takeaways
- 📚 Differential equations are powerful tools for modeling and understanding the physical world.
- 🎓 The video is part of a playlist accompanying a university course on differential equations with calculus as a prerequisite.
- 🌐 A free and open-source textbook is available, corresponding to the video series.
- 🔄 Differential equations relate the rate of change of a function to the function itself, often expressed as y' = f(y).
- 💰 An example of a differential equation is the continuous compound interest model, where the rate of change of an amount y is proportional to y itself.
- 📈 The exponential growth function y(t) = e^(0.03t) is a solution to the continuous compound interest model differential equation.
- 🤔 Differential equations often have an infinite family of solutions, depending on a constant c, which can be determined by initial conditions.
- 🚀 Another example is the motion of a ball thrown vertically, modeled by a second-order differential equation involving gravity and initial conditions.
- 🧮 The solutions to differential equations can be found by integrating the equations, as demonstrated with the ball's motion equation.
- 🔍 Further videos will explore when and how to find solutions to differential equations, as well as the theory behind their solutions.
Q & A
What is the primary purpose of differential equations?
-The primary purpose of differential equations is to model and understand the physical world by describing how various quantities change over time.
What is a differential equation?
-A differential equation is an equation that includes derivatives, representing the rates of change of quantities, which helps in modeling the dynamics of various systems.
How does the concept of a derivative relate to differential equations?
-Derivatives are used in differential equations to represent the rate of change of a function, which is essential for modeling how different aspects of the world change over time.
What is the significance of the exponential growth model in the context of differential equations?
-The exponential growth model is significant because it represents a type of differential equation that can be used to model scenarios like bank account interest accumulation and the spread of pandemics, where growth is proportional to the current size.
How does an initial condition help in solving a differential equation?
-An initial condition provides the necessary starting point or value for a variable at a specific time, which helps to determine the unique solution from the infinite family of solutions that a differential equation may have.
What is the role of Newton's second law in the formation of a differential equation related to a ball thrown vertically?
-Newton's second law, which states that the force on an object is equal to the mass times its acceleration, is used to derive a second-order differential equation that describes the motion of a ball thrown vertically, taking into account the force of gravity.
Why are there often multiple solutions to a single differential equation?
-There are often multiple solutions to a single differential equation because the equations typically contain integration constants, which can take on different values, resulting in a family of solutions corresponding to different initial conditions.
What is the relationship between the order of a differential equation and the number of initial conditions needed to solve it?
-The order of a differential equation (the highest derivative present) determines the number of initial conditions needed to find a unique solution. For example, a second-order differential equation requires two initial conditions to specify a unique solution.
How does the concept of a solution to a differential equation differ from other types of mathematical solutions?
-A solution to a differential equation is a function that satisfies the differential equation, as opposed to solutions to algebraic equations which are typically specific values. Differential equation solutions can be part of a family of functions, each corresponding to different initial conditions or constants.
What are some of the key questions that will be explored in future videos of the differential equations playlist?
-Future videos will explore questions such as when differential equations have solutions, the existence of a unique solution versus multiple solutions, and the methods or procedures for finding solutions to given differential equations.
What is the significance of the quadratic behavior in the solution of the differential equation for a ball thrown vertically?
-The quadratic behavior in the solution represents the parabolic trajectory of the ball, showing how the ball's position changes over time under the influence of gravity, starting from an initial position and velocity.
Outlines
📚 Introduction to Differential Equations
This paragraph introduces the concept of differential equations as a powerful tool for modeling and understanding the physical world. It highlights the connection between calculus and differential equations, emphasizing how calculus can be used to find the rate of change of functions. The video series is mentioned as a companion to a university course on differential equations, with a free and open-source textbook available. The main idea is that differential equations often involve knowing the rate of change rather than the exact state of a system at all times, as exemplified by a bank account with a continuous 3% interest rate. The solution to this differential equation is e to the 0.03t, demonstrating that there can be an infinite family of solutions depending on a constant (c).
🚀 Applying Differential Equations to Real-World Scenarios
This paragraph delves into applying differential equations to real-world scenarios, such as bank accounts, pandemics, and bacterial growth, showing how the rate of change is proportional to the original value. It introduces the concept of an initial condition, which, when combined with a differential equation, can yield a specific solution. The example of a bank account growing exponentially is used to illustrate this point. The paragraph also discusses the motion of a ball thrown straight up and falling back down, using Newton's second law to derive a second-order differential equation. The solution to this equation is a quadratic function, and the importance of initial conditions in determining the specific path of the ball is emphasized.
🔍 Exploring the Theory and Solutions of Differential Equations
The final paragraph discusses the theoretical aspects of differential equations, questioning when solutions exist and how many there might be. It acknowledges that while the examples provided offered solutions, there may be cases where the equations are more complex. The paragraph also touches on the methods for finding solutions, noting that while some can be computed directly, others may require learning specialized procedures. The video ends with an invitation to follow the playlist and textbook for further exploration of differential equations, promising more math in the upcoming videos.
Mindmap
Keywords
💡Differential Equations
💡Derivatives
💡Models
💡Rate of Change
💡Initial Conditions
💡Exponential Growth
💡Continuous Compounding
💡Newton's Second Law
💡Integration
💡Kinematic Equations
💡Open Source Textbook
Highlights
Differential equations are powerful tools for modeling and understanding the physical world.
This video is part of a playlist on differential equations accompanying a university course.
Differential equations are often used to model changes in objects, such as movement, fluid flow, and stock prices.
Calculus is a prerequisite for understanding differential equations, particularly the concept of derivatives.
Differential equations can be used to find the original functions from their rates of change.
An example of a differential equation is the model for a bank account with a continuous 3% interest rate.
The solution to a differential equation can be found by using the function e to the power of a constant times t.
Differential equations often have an infinite family of solutions, not just a single solution.
An initial condition is needed to find the specific value of the constant in a differential equation's solution.
The concept of differential equations is relevant in various scenarios like bank accounts, pandemic growth, and bacterial population growth.
A second example of a differential equation is modeling the motion of a ball thrown straight up and falling back down.
Newton's second law leads to a second-order differential equation for the motion of the ball.
The solution to the ball's motion differential equation is a quadratic function of time.
For second-order differential equations, two initial conditions are needed to specify the constants in the solution.
The video introduces the concept of differential equations and their applications in a clear and accessible manner.
The video encourages viewers to explore the playlist and the accompanying open-source textbook for further learning.
The video poses questions about the existence and uniqueness of solutions to differential equations, which will be explored in future videos.
The video provides a foundational understanding of differential equations and their practical applications.
Transcripts
Browse More Related Video

Differential Equations with Velocity and Acceleration (Differential Equations 7)

Simple Differential Equations

Introduction to Differential Equations (Differential Equations 2)
Calculus AB Homework 7.1 Differential Equations

AP Calculus AB: Lesson 7.2 Slope Fields
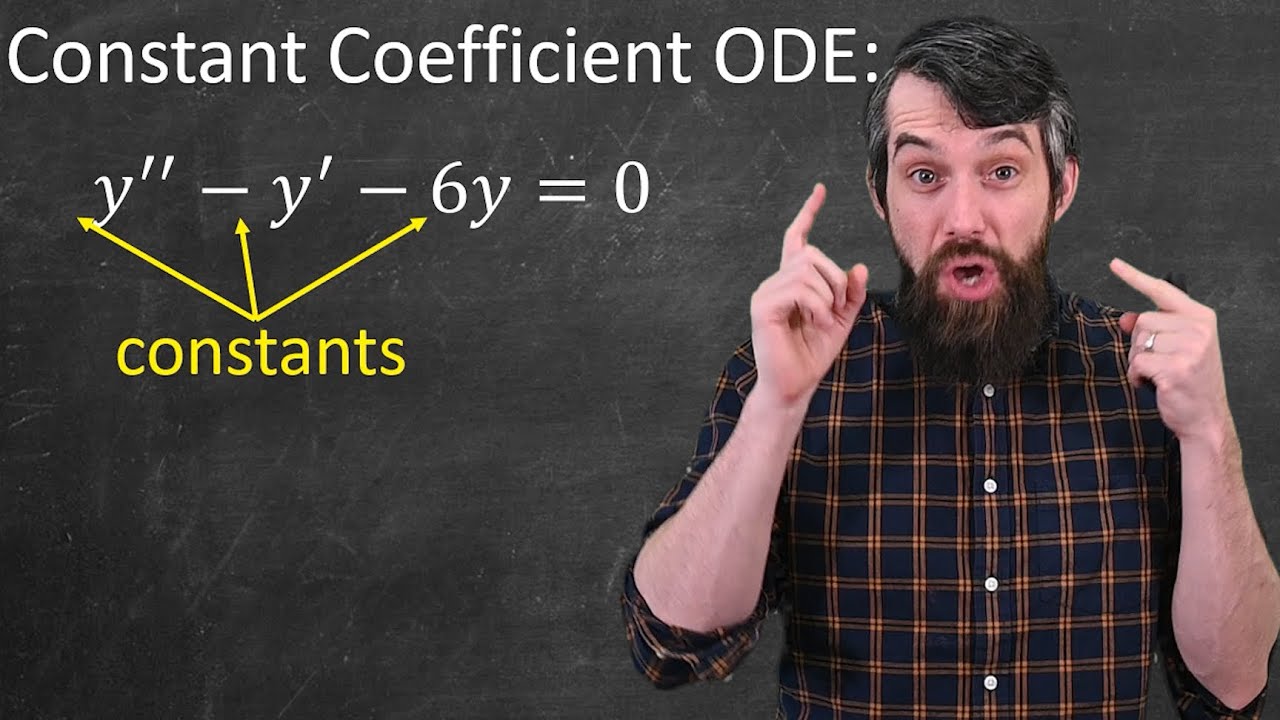
How to Solve Constant Coefficient Homogeneous Differential Equations
5.0 / 5 (0 votes)
Thanks for rating: