Calculate the pH of Acids and Bases Given the Concentration of a Solution
TLDRThe video script is an educational guide on calculating pH values for both acidic and basic solutions. The presenter explains the pH formula, demonstrates how to calculate pH for a 0.03 M HCl solution, and then moves on to a more complex H2SO4 example. The video also covers how to calculate pH for bases, using hydroxide ion concentration, and provides examples with detailed calculations. The presenter emphasizes the importance of understanding the number of ions and significant figures in accurate pH calculation.
Takeaways
- 📚 The pH scale measures the acidity or alkalinity of a solution, with lower pH values indicating stronger acids and higher pH values indicating stronger bases.
- 🧪 To calculate pH, use the formula pH = -log[H+], where [H+] is the hydrogen ion concentration.
- 💧 For strong acids with a 1:1 ratio of hydrogen ions to anions, the molarity of the acid directly represents the [H+].
- 🔄 For strong acids with more than one hydrogen ion per molecule (e.g., H2SO4), multiply the given molarity by the number of hydrogen ions.
- 📈 When dealing with bases, use the relationship Kw = [H+][OH-] = 1×10^-14 to find the [H+] from the hydroxide ion concentration.
- 🧬 The concentration of hydroxide ions in a basic solution can be found by dividing the given molarity by the number of hydroxide ions in the base.
- 🌟 The pH of a basic solution can be determined using the hydroxide ion concentration and the ion product constant for water (Kw).
- 🔢 For solutions where the ratio of hydrogen ions is not 1:1, adjust the molarity to account for the correct number of ions.
- 📊 When calculating pH, significant figures (sig figs) are important to ensure accuracy in the results.
- 👨🏫 It is essential to follow the instructions of your teacher or professor regarding the number of significant figures or decimal places to report.
- 🎥 The video provides step-by-step examples of calculating pH for both acids and bases, demonstrating the use of the pH formula and ion product constant.
Q & A
What is the pH formula?
-The pH formula is pH = -log[H+], where [H+] represents the hydrogen ion concentration of the solution.
How do you calculate the pH of a 0.03 M HCl solution?
-For a 0.03 M HCl solution, since HCl is a strong acid and dissociates completely, the hydrogen ion concentration is equal to the molarity of the acid. Therefore, pH = -log(0.03), which calculates to approximately 1.5 when keeping two significant figures.
What is the significance of the 1:1 ratio in the HCl pH calculation?
-The 1:1 ratio indicates that for each molecule of HCl that dissociates in water, one hydrogen ion (H+) and one chloride ion (Cl-) are produced. This means the concentration of hydrogen ions is equal to the initial molarity of the HCl solution.
How does the pH calculation change when dealing with a polyprotic acid like H2SO4?
-For a polyprotic acid like H2SO4, which dissociates to produce two hydrogen ions for every molecule, the concentration of hydrogen ions is twice the molarity of the acid. Thus, in the pH calculation, you would use 2 * [H2SO4 concentration] as the [H+] value.
What is the significance of the 1:2 ratio in the pH calculation of a solution like Ca(OH)2?
-The 1:2 ratio in Ca(OH)2 indicates that for each molecule of Ca(OH)2, two hydroxide ions (OH-) are produced. This means the concentration of hydroxide ions is twice the molarity of the base, and this must be used in the calculation of the hydrogen ion concentration and subsequently the pH.
How do you calculate the pH of a basic solution?
-For a basic solution, you first calculate the hydroxide ion (OH-) concentration using the given molarity of the base. Then, using the relationship [H+] * [OH-] = 1 * 10^-14, you solve for the hydrogen ion concentration [H+]. Finally, you use this value in the pH formula to find the pH of the solution.
What is the relationship between pH and the strength of an acid?
-The lower the pH value, the stronger the acid. A strong acid will have a pH value closer to 0, indicating a higher concentration of hydrogen ions in the solution.
What is the general rule for the pH of a solution in relation to 7?
-A pH less than 7 indicates an acidic solution, while a pH greater than 7 indicates a basic (alkaline) solution. A pH of exactly 7 is considered neutral.
How do you round the pH value to significant figures?
-You round the pH value to the number of significant figures required by your calculation context or as instructed by your teacher or professor. Typically, two or three significant figures are used.
What is the importance of using a calculator in pH calculations?
-A calculator is essential in pH calculations because it allows for precise computation of logarithms and division, which are necessary to determine the hydrogen ion concentration and the final pH value.
How does the concentration of hydroxide ions affect the pH calculation in basic solutions?
-In basic solutions, the pH is calculated based on the hydroxide ion concentration. The higher the concentration of hydroxide ions, the lower the hydrogen ion concentration, and thus the higher the pH value of the solution.
Outlines
🧪 Introduction to Calculating pH of Acidic Solutions
This paragraph introduces the topic of calculating pH for acidic solutions. The speaker begins by expressing excitement about covering chemistry problems requested by viewers, specifically those related to pH calculation. The first problem involves a 0.03 Molar HCl solution. The speaker explains the pH formula (pH = -log[H+]) and how to apply it to find the pH of the given solution. The process includes identifying the ratio of hydrogen to chloride ions, which in this case is 1:1, and using the molarity value in the pH formula. The calculation is demonstrated with the use of a calculator, resulting in a pH value of approximately 1.528. The speaker also discusses the importance of significant figures (sig figs) in reporting the final pH value, noting that most pH measuring devices only display two decimal places.
🧪 Calculating pH of Solutions with Non-1:1 Ion Ratios
This paragraph delves into calculating the pH of solutions where the ion ratio is not 1:1. The example provided involves sulfuric acid (H2SO4), which has a 2:1 ratio of hydrogen ions to sulfate ions. The speaker emphasizes the need to adjust the molarity value to account for the extra hydrogen ions, doubling the given molarity to 0.06 M for the calculation. The pH formula is reapplied, and the new molarity value is used to find the pH, which is approximately 1.22. The speaker reiterates the importance of adhering to the number of significant figures as per the instructions from the viewers' professors or teachers.
🧪 Understanding the Concept of Acidic and Basic Solutions
This paragraph introduces the concept of acidic and basic solutions by comparing two acids with different pH values. The speaker explains that a lower pH indicates a stronger acid, while a higher pH indicates a stronger base. The pH scale is briefly reviewed, with acids ranging from 1 to just below 7, bases from above 7 to 14, and a neutral pH of 7. The speaker then transitions to discussing bases, using a hypothetical solution with a given concentration. The importance of recognizing the nature of the compound (acid or base) is highlighted, with a focus on the presence of OH- ions to identify bases.
🧪 Calculating pH of Basic Solutions with Hydroxide Ions
This paragraph focuses on calculating the pH of basic solutions, specifically those containing hydroxide ions. The speaker presents a problem involving a 0.05 M solution of a base and explains the need to use a different approach since the solution does not contain hydrogen ions directly. The relationship between hydrogen ion concentration [H+] and hydroxide ion concentration [OH-] is introduced, with the sum of all acidic and basic substances in the solution being 1 x 10^-14. The calculation involves solving for the hydrogen ion concentration using algebraic manipulation and then applying the pH formula. The resulting pH is approximately 12.697, with a discussion on the appropriate number of significant figures to report.
🧪 Final pH Calculation for a Basic Solution with Calcium and Hydroxide Ions
The final paragraph of the script focuses on calculating the pH of another basic solution, this time with a 0.05 M molarity of calcium and hydroxide ions. The speaker explains the need to double the hydroxide ion concentration due to the 2:1 ratio of hydroxide to calcium ions. Using the same relationship between [H+] and [OH-] as in the previous base calculation, the speaker sets up the equation and solves for the hydrogen ion concentration. The resulting [H+] is 1 x 10^-13, which is then used in the pH formula to find the pH value of exactly 13. The speaker concludes by reiterating the importance of following the guidance of teachers or professors regarding the number of significant figures to include in the final pH value.
Mindmap
Keywords
💡pH calculation
💡Hydrogen ion concentration
💡Acid
💡Base
💡Molarity
💡Ion product constant
💡Logarithm
💡Significant figures
💡Sulfuric acid
💡Potassium hydroxide
💡Calcium hydroxide
Highlights
The video is about calculating pH of solutions using molarity of acids and bases.
The pH formula is introduced as pH = -log[H+], where [H+] is the hydrogen ion concentration.
For a 0.03 M HCl solution, the pH is calculated with a 1:1 ratio of H+ to Cl- ions.
The pH of the 0.03 M HCl solution is found to be 1.528748, rounded to 1.5 with two significant figures.
The video presents a problem involving H2SO4 (sulfuric acid), which has a 2:1 ratio of H+ to SO4^2- ions.
The pH of the 0.03 M H2SO4 solution is calculated to be 1.2 with two significant figures.
A comparison between the two acids shows that the lower the pH, the stronger the acid.
The video then discusses calculating pH for bases, starting with a solution of KOH.
The relationship between [H+] and [OH-] is given by the formula [H+] * [OH-] = 1 * 10^-14.
For the KOH solution, the [H+] is found by dividing 1 * 10^-14 by the given [OH-] concentration.
The pH of the KOH solution is calculated to be 12.7, rounded to 13 as per the calculator reading.
Another base, Ca(OH)2, is used in the last problem, with a 1:2 ratio of Ca^2+ to OH- ions.
The pH of the 0.05 M Ca(OH)2 solution is determined to be exactly 13.
The video emphasizes the importance of understanding the number of ions and their ratios in acid-base reactions.
The presenter advises students to write down the entire number before applying significant figures.
The video concludes by reiterating the method for calculating pH from molarity of acidic or basic solutions.
Transcripts
Browse More Related Video
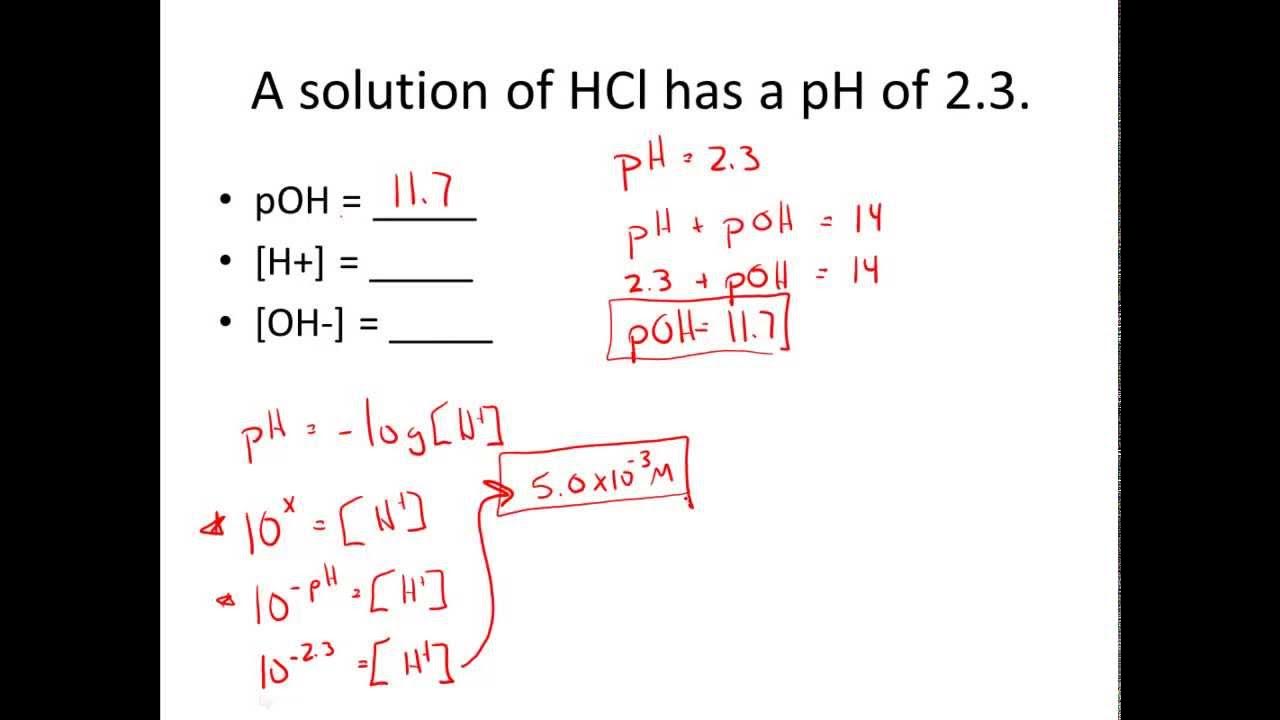
Given pH & pOH, Solve for [H+] & [OH-] Practice Problems
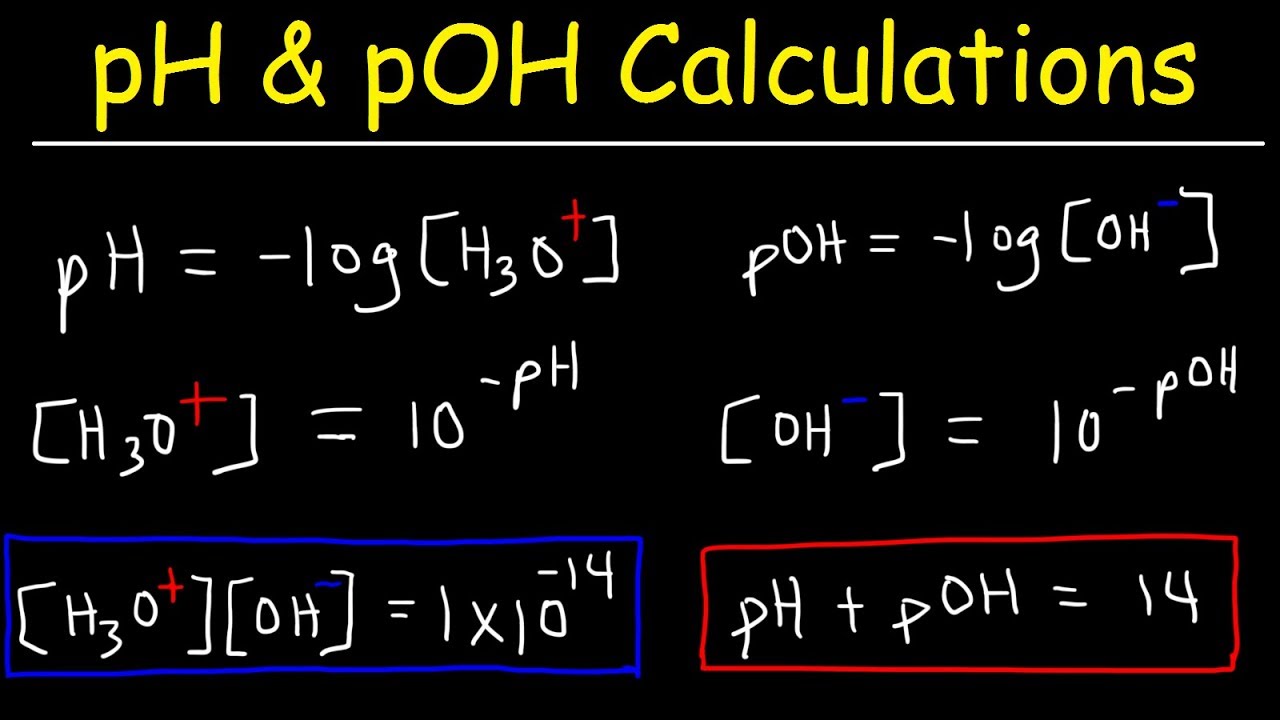
pH, pOH, H3O+, OH-, Kw, Ka, Kb, pKa, and pKb Basic Calculations -Acids and Bases Chemistry Problems

ALEKS - Interconverting pH and Hydronium Ion Concentration

What is pH ; How to Calculate pH (3 examples) - Chemistry
Acids And Bases Salts And pH Level - What Are Acids Bases And Salts - What Is The pH Scale Explained

pH, pOH of strong acids and bases | Chemistry | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: