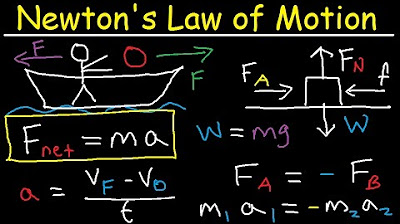
AP Physics 1 Dynamics (Forces and Newton’s Laws) Review
TLDRThis video script offers a comprehensive review of dynamics in AP Physics 1, focusing on force diagrams and Newton's Laws of Motion. It explains the absence of a forward force on a box moving at constant velocity, illustrating Newton's first law. The script further discusses static and kinetic friction, and the conditions for equilibrium. It clarifies the application of Newton's second law in calculating acceleration and the identification of action-reaction pairs. The video also addresses the concept of forces on an inclined plane, emphasizing the correct use of sine and cosine in these scenarios.
Takeaways
- 📚 The video discusses force diagrams and the application of Newton's Laws of Motion in the context of AP Physics 1.
- 🎯 When an object is moving at constant velocity on a horizontal surface, there is no net force acting on it horizontally, which aligns with Newton's First Law.
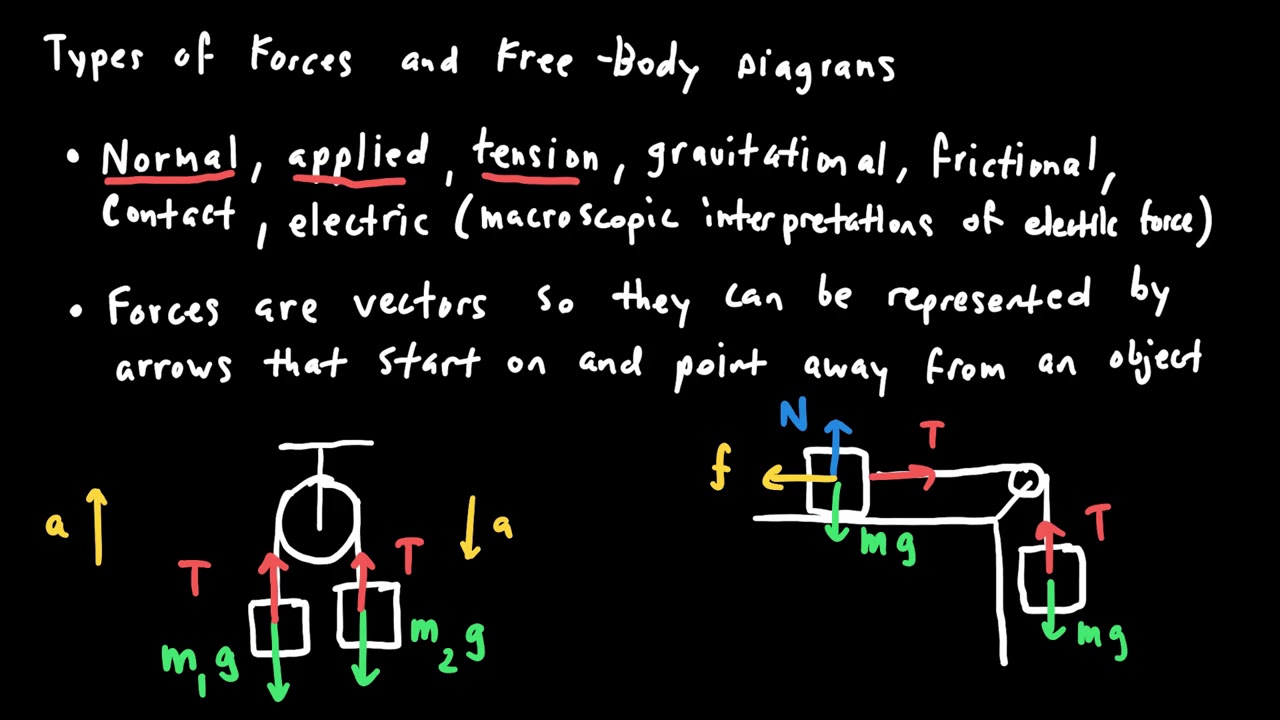
- 🔍 Identifying incorrect force diagrams is an important skill; in the example given, a forward force was incorrectly included when there should be none.
- ⚖️ Static and dynamic equilibrium both involve a net force of zero, but differ in that static equilibrium refers to an object at rest, while dynamic equilibrium involves constant velocity motion.
- 🤔 Newton's Second Law is used to analyze the acceleration of objects, stating that the net force on an object is equal to its mass times its acceleration (F = ma).
- 🌐 When analyzing a system of connected objects, like a pulley system, the acceleration of each object is the same because they are connected.
- 🔧 Newton's Third Law is about action and reaction forces, which are equal in magnitude and opposite in direction, acting on different objects.
- 📐 The correct use of trigonometric functions (sine and cosine) is crucial when dealing with inclined planes, as they determine the components of gravitational force.
- 🚫 Friction, both static and kinetic, is a force that must be considered in real-world scenarios and is calculated differently for each type.
- 🌍 The example of the Earth and a rock illustrates that while action and reaction forces are equal, the effects on different masses can vary greatly due to the difference in mass and resulting acceleration.
- 📌 When pushing two objects together that are accelerating, the forces between them are internal and the system's acceleration is the same for both objects, which simplifies the analysis.
Q & A
What is the first force mentioned in the script that acts on an object with mass?
-The first force mentioned is the gravitational force, which acts downward on any object with mass on Earth.
What is the significance of Newton's first law in the context of the box moving at constant velocity?
-Newton's first law, also known as the law of inertia, states that an object at rest will stay at rest, and an object in motion will continue moving at a constant velocity unless acted upon by a net external force. In the context of the box, since it is moving at a constant velocity, it indicates that there is no net force acting on it, meaning the forces are balanced.
What are the two possibilities when the net force equals zero?
-The two possibilities when the net force equals zero are static equilibrium, where the object is at rest, and dynamic equilibrium, where the object is moving at a constant velocity.
How does Newton's second law help in analyzing the forces acting on an accelerating at-Machine?
-Newton's second law states that the acceleration is equal to the net force divided by the mass (a = F/m). This law helps in analyzing the forces on an accelerating at-Machine by allowing us to calculate the net force based on the observed or known acceleration and the mass of the system.
What is the relationship between the tension force (ft) and the gravitational force (fgb) when an at-Machine is accelerating?
-When the at-Machine is accelerating, the tension force (ft) is not equal to the gravitational force (fgb). The gravitational force is the weight of the object (B), and if the system is accelerating, the tension force must be greater than the gravitational force to create a net force causing the acceleration.
How does the script illustrate Newton's third law with the forces acting on the at-Machine?
-The script illustrates Newton's third law by showing that the tension force (ft) and the gravitational force (fgb) are not action-reaction pairs because they act on the same object (A). The correct action-reaction pairs would involve forces acting on different objects, such as the force exerted by the Earth on an object (action) and the force exerted by the object on the Earth (reaction).
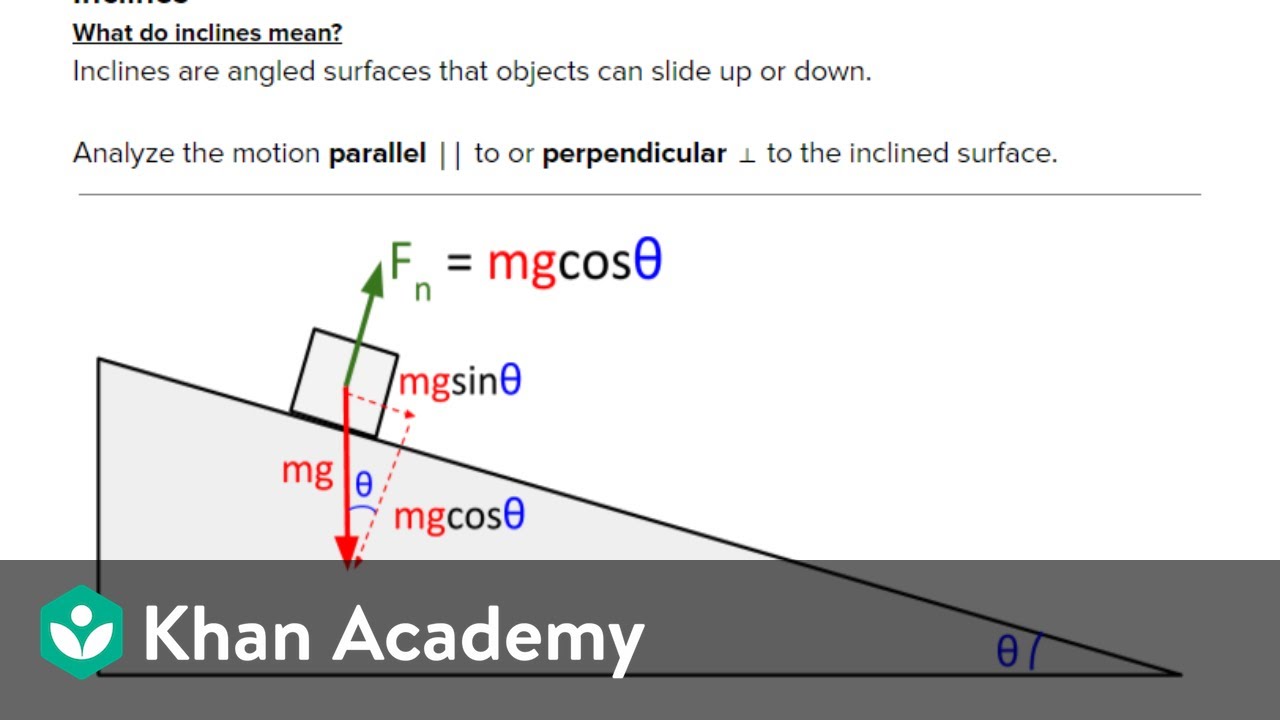
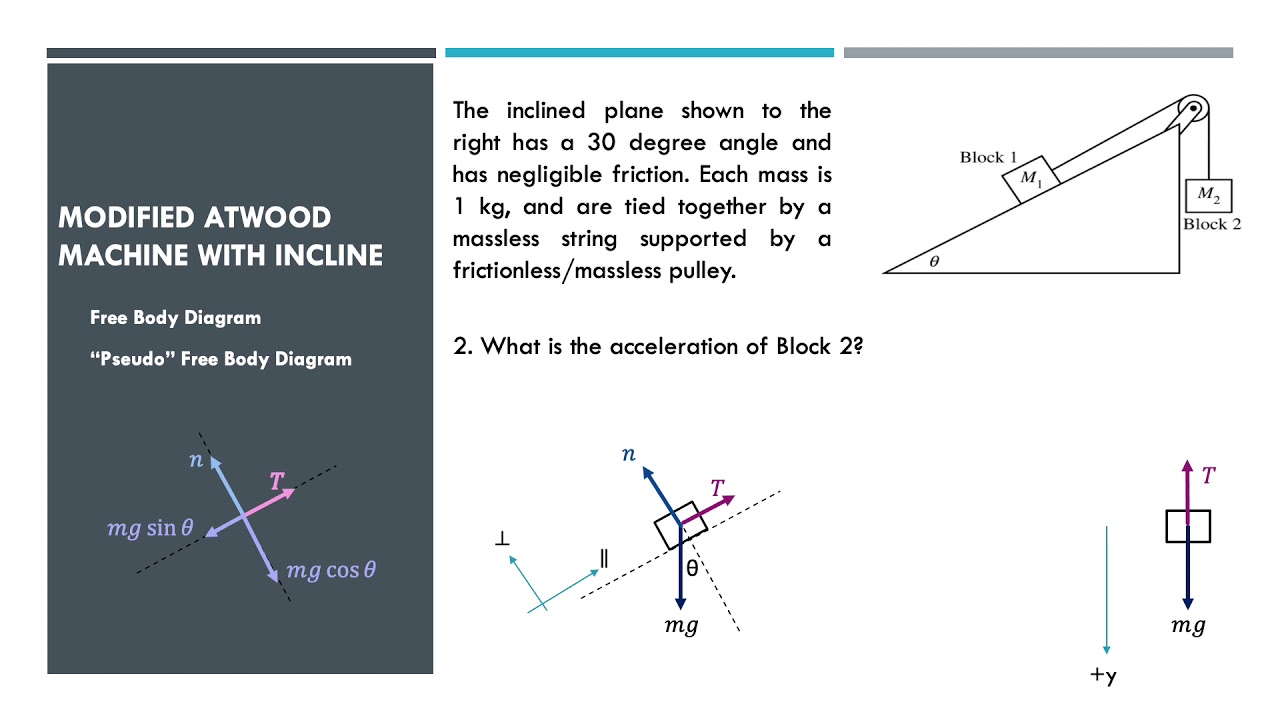
What is the correct way to resolve gravitational force into components on an inclined plane?
-On an inclined plane, the gravitational force is resolved into two components: one perpendicular to the surface (FG cosine Theta) and one parallel to the surface (FG sine Theta). The perpendicular component is equal to the gravitational force times the cosine of the incline angle, and the parallel component is equal to the gravitational force times the sine of the incline angle.
How does the presence of friction affect the forces acting on an object sliding down an inclined plane?
-If there is friction and the object is sliding down the inclined plane, the gravitational force is still present, but now there is also a normal force (FN) perpendicular to the surface, and a kinetic friction force that acts opposite to the direction of motion. The kinetic friction force is equal to the coefficient of friction times the normal force.
What is the maximum static friction force, and how does it relate to the applied force?
-The maximum static friction force is equal to the coefficient of static friction (μs) times the normal force (FN). It is the maximum force that can be applied to an object without causing it to move relative to the surface it's on. The static friction force will match the applied force up to this maximum value, preventing the object from starting to slide.
How can static friction cause an object to move, even though it's not moving relative to the surface?
-Static friction can cause an object to move if it is applied in a way that overcomes the object's inertia but does not cause it to slide relative to the surface it's on. For example, if you push a box on the bottom of a stack, the static friction between the boxes causes the top box to move along with the bottom box without sliding on the surface.
In the scenario where two boxes are pushed together, what is the key concept to remember about their acceleration?
-The key concept is that both boxes will have the same acceleration because they are moving together as one system. This means that when analyzing the forces, you can set the acceleration of box A equal to the acceleration of box B, which simplifies the calculations and helps in solving the problem.
Outlines
📚 Dynamics for AP Physics - Force Analysis
This paragraph introduces a review of Dynamics from AP Physics, focusing on force analysis. The video begins with a box on a smooth surface moving at a constant velocity to the right. The speaker then draws forces acting on the box, including gravitational force and normal force, and asks viewers to identify any incorrect forces. It emphasizes the absence of a forward force, aligning with Newton's first law, which states that an object in motion will continue to move at a constant velocity unless acted upon by an external force. The speaker corrects the force diagram, removing the unnecessary forward force, and explains the concepts of static and dynamic equilibrium, both characterized by a net force of zero. The video continues with an example of a modified atwood machine, illustrating the mistake of assuming equal tension forces (ft and fgb) in an accelerating system. It corrects this by applying Newton's second law, explaining that for an object to accelerate, the net force must be in the direction of the acceleration, and the larger force (fgb) must be greater than the smaller force (ft). The speaker also discusses how to calculate the acceleration of object B and the tension force, emphasizing the importance of considering the system's mass when applying Newton's laws.
🔍 Newton's Laws - Action and Reaction
In this paragraph, the focus shifts to Newton's third law of motion, which states that for every action, there is an equal and opposite reaction. The speaker uses examples to illustrate this law, such as the force between the Earth and a rock, and clarifies that while the forces are equal in magnitude, their effects differ due to the masses of the objects involved. The speaker then addresses a common mistake in force diagrams for objects on an inclined plane, correcting the use of sine and cosine for the gravitational force components. It explains the difference between static and kinetic friction, noting that kinetic friction is proportional to the coefficient of friction and the normal force, while static friction can vary up to a maximum value based on the applied force. The paragraph concludes with an example of pushing two boxes together, emphasizing that both boxes will have the same acceleration and that the forces between them are internal forces within the system.
🚀 Applying Newton's Laws - Systems in Motion
The final paragraph delves into applying Newton's laws to analyze systems in motion. The speaker uses the example of two boxes being pushed together to demonstrate how to calculate the forces and accelerations involved. It reiterates that both objects in the system will share the same acceleration and that the internal forces between them do not affect this acceleration. The speaker also discusses the concept of static friction, explaining that it can cause an object to move as long as there is no relative motion between the object and the surface it is on. The paragraph highlights the importance of understanding the relationship between applied forces, frictional forces, and the mass of the objects when analyzing physical systems. By using Newton's second law, the speaker shows how to determine the net force and acceleration of the system, providing a comprehensive understanding of the dynamics at play.
Mindmap
Keywords
💡Dynamics
💡Gravitational Force
💡Normal Force
💡Newton's First Law
💡Static Equilibrium
💡Dynamic Equilibrium
💡Newton's Second Law
💡Friction
💡Pulley System
💡Inclination
💡Action-Reaction Pairs
Highlights
Reviewing Dynamics for AP Physics 1, starting with a box on the ground.
The box is moving towards the right at constant velocity on a smooth surface.
Identifying the correct force diagram for the box with no forward force acting on it.
Application of Newton's first law in explaining the constant velocity motion of the box.
Explaining the two possibilities when the net force equals zero: Static equilibrium and Dynamic equilibrium.
Analyzing forces on a modified at-machine, identifying the mistake in the force diagram.
Using Newton's second law to calculate the net force and the system's mass.
Determining the tension force by isolating object A and focusing on its horizontal motion.
Applying Newton's third law to identify action-reaction pairs in the force diagram.
Drawing a force diagram for an object on an inclined plane and correcting the errors.
Explaining the use of sine and cosine in determining the forces acting on the inclined plane.
Describing the characteristics of kinetic friction and its relationship with the coefficient of friction.
Discussing static friction, its properties, and how it can cause an object to move without relative motion.
Analyzing a situation with two boxes being pushed together, emphasizing the same acceleration for both boxes.
Using the agent-victim notation to clearly define the forces acting between two objects.
Understanding that static friction doesn't prevent an object from moving, but it prevents relative motion.
Calculating the maximum static friction and its relationship with the applied force.
Explaining how the friction force changes when an object starts sliding and the concept of kinetic friction.
Discussing the effect of the Earth's large mass on the acceleration of objects in its gravitational pull.
Concluding with the importance of understanding the shared acceleration in connected systems.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: