Strategy in finding limits | Limits and continuity | AP Calculus AB | Khan Academy
TLDRThe video script discusses various strategies for finding limits in calculus, emphasizing the importance of understanding when to directly substitute values and when to apply special techniques for dealing with discontinuities or indeterminate forms. It introduces the concept of vertical asymptotes and illustrates methods such as factoring, multiplying by conjugates, and using trigonometric identities to simplify expressions and find limits. The video also briefly mentions L'hospital's Rule and the use of approximation as a last resort.
Takeaways
- 📈 The video discusses strategies for finding limits of functions, emphasizing the importance of choosing the right technique.
- 📊 When approaching a limit, the first step is to try substituting the value of 'a' into the function and evaluate if it yields a real number.
- 🔄 It's noted that the limit and the function value at a point may differ, especially in cases of discontinuities.
- 🤔 For plain vanilla functions like polynomials, rational, and trigonometric expressions, evaluating the function at 'a' is usually sufficient to find the limit.
- ⚠️警惕特殊函数情况, such as piecewise-defined functions or those with jump discontinuities, where direct evaluation may not suffice.
- 📉 Encountering an expression that results in zero over zero or infinity over infinity indicates an indeterminate form that requires further analysis.
- 🌟 Factoring and simplifying expressions can help resolve indeterminate forms and lead to the discovery of the actual limit.
- 🔢 For rational radical expressions, multiplying by a conjugate can simplify the expression and help in finding the limit.
- 📐 Trigonometric identities can be used to transform indeterminate forms involving trigonometric functions, allowing for easier limit determination.
- 🔍 If standard techniques fail, numerical approximation can be a last resort to estimate the limit by testing values very close to the point of interest.
Q & A
What is the primary goal of the video?
-The primary goal of the video is to discuss strategies for determining which technique to use when finding limits of functions.
What is the first step recommended by the flowchart for finding the limit of a function?
-The first step recommended by the flowchart is to substitute the value of 'a' into the function and evaluate f(a) to see if it yields a real number.
Why is it important to be cautious when the function is continuous at a point and you evaluate it at that point?
-It is important to be cautious because the limit and the function value can be different, especially in cases where the function has a point discontinuity or a jump discontinuity.
What does encountering an expression that results in 'number divided by zero' indicate?
-Encountering an expression that results in 'number divided by zero' indicates that you are likely dealing with a vertical asymptote.
How can you verify the presence of a vertical asymptote?
-You can verify the presence of a vertical asymptote by trying out values near the point of interest, plotting the function, or using algebraic techniques to find where the denominator becomes zero.
What is the significance of the indeterminate form 'zero over zero'?
-The indeterminate form 'zero over zero' indicates that the function is not defined at that point, and further algebraic manipulation, such as factoring or application of L'Hopital's Rule (in calculus), may be needed to find the limit.
What is the basic strategy for dealing with an indeterminate form involving a rational expression?
-The basic strategy for dealing with an indeterminate form involving a rational expression is to factor the expression and simplify it to evaluate the limit.
How can you handle an indeterminate form involving radical expressions?
-For indeterminate forms involving radical expressions, you can multiply the expression by its conjugate to eliminate the radical and simplify the expression for limit evaluation.
What is the role of trigonometric identities in solving limits?
-Trigononometric identities can be used to simplify expressions involving trigonometric functions, allowing you to find limits by canceling out terms or rewriting the expression in a more manageable form.
What is the last resort technique for finding limits when other methods fail?
-The last resort technique for finding limits when other methods fail is approximation, where you try values very close to the point of interest and observe the behavior of the function.
Why is it important to consider different scenarios when finding limits?
-Considering different scenarios when finding limits is important because it ensures that you account for discontinuities, special cases, and the behavior of the function near the point of interest, leading to a more accurate and thorough understanding of the limit.
Outlines
📚 Introduction to Finding Limits
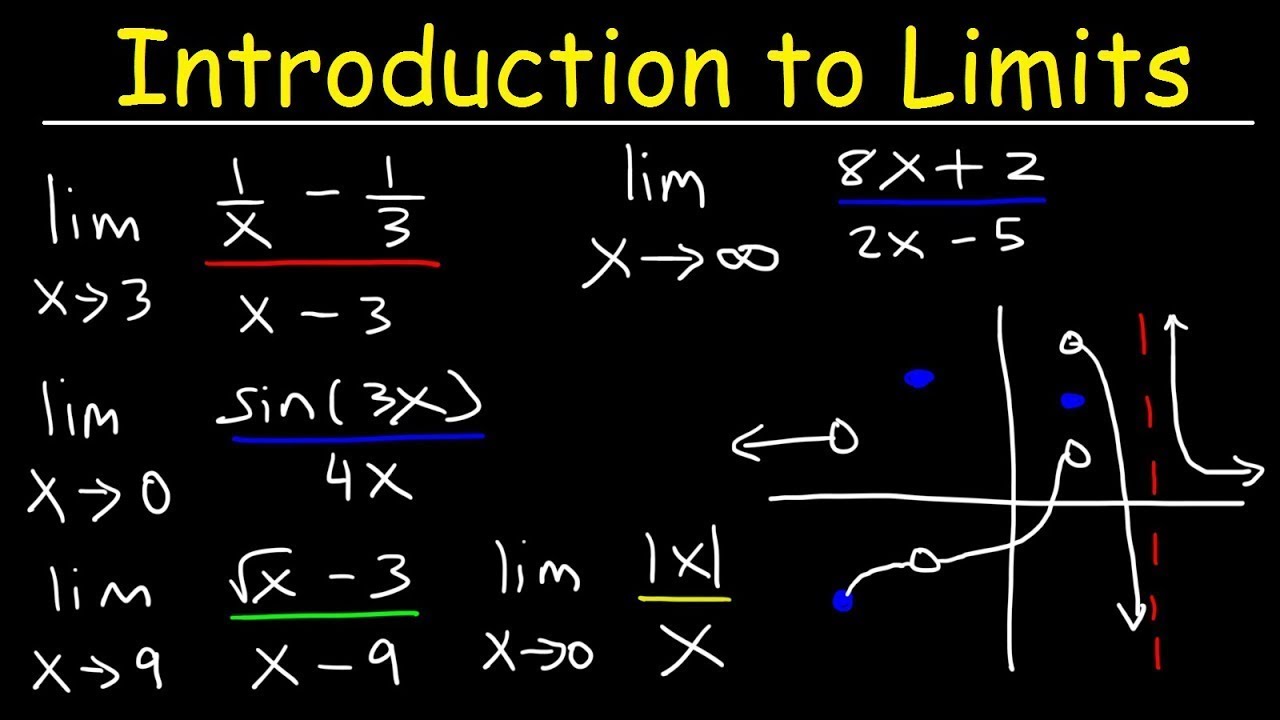
This paragraph introduces the concept of finding limits in calculus, emphasizing the importance of strategies in determining the appropriate technique to use. The instructor references a flowchart from Khan Academy and explains the initial steps of attempting to substitute the value of 'a' into the function to find the limit. It highlights the distinction between the limit and the function's value, especially in cases of discontinuities. The paragraph also touches on the idea of evaluating functions at 'a' for simple functions like polynomials, rational, and trigonometric expressions, and what to consider when dealing with piecewise-defined functions or discontinuities, such as vertical asymptotes.
📈 Dealing with Indeterminate Forms and Special Cases
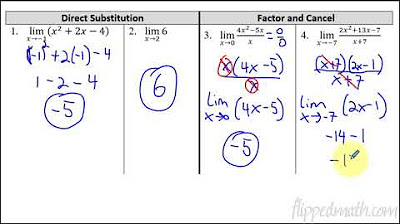
The second paragraph delves into techniques for handling indeterminate forms that arise when evaluating limits. It introduces the concept of factoring, particularly for rational expressions, as a method to simplify and find the limit. The paragraph provides a detailed example of factoring and simplifying a rational expression to determine the limit as x approaches -1, resulting in a value of three fourths. It also mentions the use of L'hospital's Rule for more advanced cases involving calculus. Additionally, the paragraph discusses other techniques such as multiplying by a conjugate for radical expressions and the application of trigonometric identities to resolve indeterminate forms.
🌟 Advanced Techniques and Numerical Approximations
The final paragraph focuses on advanced techniques for finding limits, particularly in cases where previous methods fail. It discusses the handling of point discontinuities through simplification and the use of trigonometric identities to transform expressions and find limits. The example provided involves the limit of a trigonometric expression as x approaches zero, which is resolved using trigonometric identities to yield a limit of one half. The paragraph concludes with a brief mention of numerical approximation as a last resort method for finding limits, suggesting the use of very close values to the point of discontinuity to estimate the limit.
Mindmap
Keywords
💡Limit
💡Flowchart
💡Continuous Function
💡Vertical Asymptote
💡Indeterminate Form
💡Factoring
💡Rational Expression
💡Trigonometric Expressions
💡L'Hopital's Rule
💡Conjugate
💡Trig Identities
💡Approximation
Highlights
The video discusses various strategies for finding limits in calculus, providing a comprehensive approach to tackling different types of limit problems.
A flowchart developed by Khan Academy is introduced as a tool to help determine which technique to use when finding limits.
The first step in finding a limit is to try substituting the value of 'a' into the function and evaluating it to see if the result is a real number.
It's important to note that the limit of a function at a point may not always equal the value of the function at that point, especially in cases of discontinuities.
For basic functions like polynomials and trigonometric expressions, evaluating the function at 'a' usually gives the limit if it results in a real number.
Piecewise-defined functions or those with discontinuities at 'a' require more careful analysis and may not be directly evaluable at 'a'.
If the function evaluation results in a division by zero, it might indicate a vertical asymptote, which needs further investigation.
The concept of a vertical asymptote is explained with an example, showing how to identify and handle such cases in limit problems.
Special cases where the function is undefined, like 1/x - x, do not have a vertical asymptote and require different handling.
The video introduces the concept of indeterminate forms, such as 0/0, and outlines methods for dealing with them, including factoring and simplifying expressions.
Factoring is a key technique for resolving indeterminate forms, as demonstrated by the example of simplifying a rational expression to find the limit.
The video also discusses the use of algebraic and trigonometric techniques to tackle indeterminate forms, such as multiplying by a conjugate for radical expressions.
Trigonometric identities are used to simplify and find limits in cases where the original expression results in an indeterminate form, like sine functions.
The video emphasizes the importance of being mathematically precise, even when simplifying expressions, to ensure the correct evaluation of limits.
As a last resort, numerical approximation can be used to estimate limits by trying values very close to the point of interest.
The video provides a structured approach to finding limits, starting with direct substitution and moving through various techniques for handling more complex scenarios.
Understanding the behavior of functions around points of discontinuity is crucial for accurately determining limits.
The video offers a practical guide to using factoring and simplification techniques to resolve indeterminate forms and find limits.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: