Calculus - Working with position, velocity, and acceleration
TLDRIn this engaging video, the secret math tutor introduces viewers to the concepts of motion, specifically focusing on position, velocity, and acceleration. The video uses a position function to illustrate how derivatives can be used to determine these aspects of motion. The tutor demonstrates how to find the velocity and acceleration of a particle by taking the first and second derivatives, respectively, of the position function. The video also explores the concept of 'jerk' as the third derivative. Practical examples are given, including calculating the velocity at a specific time and determining when the acceleration is zero. The tutor further explains how to find the total distance traveled by a particle, emphasizing the importance of considering direction changes. The video concludes with a reminder to be cautious when interpreting derivatives and solving equations, as this can significantly impact the results. For more informative content, viewers are encouraged to visit the tutor's website.
Takeaways
- 📐 The position function represents an object's height over time, and its derivatives provide information about velocity and acceleration.
- 🔢 The first derivative of the position function gives the velocity, the second derivative gives the acceleration, and the third derivative gives the jerk.
- ⏱️ To find the velocity of a particle at a specific time, take the first derivative of the position function and evaluate it at that time.
- ⏰ The acceleration can be found by taking the second derivative of the position function or the first derivative of the velocity function.
- 🔍 Setting the acceleration equal to zero helps find when the particle changes direction, which is crucial for determining the total distance traveled.
- 🔁 When the acceleration is zero, the velocity function must be evaluated at that time to understand the particle's speed at that instant.
- 📉 To find when the particle changes direction, look at where the velocity is zero and check the sign of the velocity around those points.
- 🚫 Be cautious when interpreting derivatives; a solution for time does not always directly translate to a physical quantity like velocity.
- 📏 The total distance traveled is the sum of the distances covered in each direction, which requires considering the direction changes.
- 🔢 For calculating total distance, if the particle changes direction, break down the problem into segments between direction changes.
- ➗ The position function alone might not give the total distance directly; it's necessary to consider the path's direction and any reversals.
Q & A
What is the relationship between the position function of an object and its velocity and acceleration?
-The position function of an object describes its location over time. The first derivative of the position function gives the velocity, which indicates how fast the object is moving. The second derivative of the position function, or the derivative of the velocity, gives the acceleration, which measures how quickly the velocity is changing.
What is the position function given in the video?
-The position function given in the video is T cubed minus 12 T squared minus 45 T, where T represents time in seconds and the function gives the position of a particle in meters.
How do you find the velocity of the particle after T equals zero?
-To find the velocity after T equals zero, you first take the first derivative of the position function to get the velocity function. Then, you substitute T with zero into the velocity function to calculate the velocity at that specific time.
What is the acceleration of the particle after one second?
-To find the acceleration after one second, you first find the derivative of the velocity function (which is the second derivative of the position function) to get the acceleration function. Then, you substitute T with one into the acceleration function to calculate the acceleration at that time, which is -18 meters per second squared.
When does the acceleration of the particle equal zero?
-The acceleration of the particle equals zero when T equals four seconds. This is found by setting the acceleration function (the derivative of the velocity function) equal to zero and solving for T.
What is the velocity of the particle when the acceleration equals zero?
-When the acceleration equals zero at T equals four seconds, the velocity of the particle is found by substituting T with four into the velocity function. The resulting velocity is -3 meters per second.
How do you determine the total distance traveled by the particle from T equals 0 to 4?
-To determine the total distance traveled, you need to consider the direction of the particle's movement. You find the points where the velocity is zero and check the sign of the velocity around these points to determine if the particle has changed direction. Then, you calculate the distance traveled before and after these points separately and sum them up.
At what times does the particle change direction?
-The particle changes direction at T equals three and T equals five seconds. This is determined by analyzing the sign of the velocity function around the points where the velocity is zero.
What is the distance traveled by the particle from T equals 0 to 3?
-The distance traveled by the particle from T equals 0 to 3 is 54 meters. This is calculated by finding the difference in the position function values at T equals 0 and T equals 3.
What is the distance traveled by the particle from T equals 3 to 4?
-The distance traveled by the particle from T equals 3 to 4 is 2 meters. This is determined by finding the difference in the position function values at T equals 3 and T equals 4.
What is the total distance traveled by the particle from T equals 0 to 4?
-The total distance traveled by the particle from T equals 0 to 4 is 56 meters. This is the sum of the distance traveled from 0 to 3 (54 meters) and the distance traveled from 3 to 4 (2 meters).
Why is it important to consider the direction of the particle's movement when calculating the total distance traveled?
-It is important to consider the direction of the particle's movement because the total distance traveled is the magnitude of the path taken, regardless of direction. If the particle changes direction, the distance traveled in one direction is added to the distance in the opposite direction to get the total distance.
Outlines
📐 Understanding Motion: Position, Velocity, and Acceleration
The video begins with an introduction to the concepts of motion, specifically focusing on position, velocity, and acceleration. The presenter explains that the position of an object can be described by a function of time, and its derivatives provide the velocity and acceleration. The video emphasizes the importance of recognizing these terms and knowing which derivative to take for a given problem. An example is given with a position function, and the process of finding the velocity and acceleration by taking the first and second derivatives, respectively, is demonstrated. The concept of jerk as the third derivative is also briefly mentioned.
🚀 Calculating Velocity and Acceleration for a Particle
The presenter continues with a detailed example involving a position function representing the position of a particle in meters after T seconds. The first task is to find the velocity of the particle at T equals zero by taking the first derivative of the position function and evaluating it at T=0. The acceleration is then found by taking the derivative of the velocity function. The video also explores the scenario where the acceleration is zero and how to find the corresponding velocity at that time by substituting the time value into the velocity function. The complexity of the problem is highlighted by the need to understand the context of the motion, including when the particle changes direction.
🔢 Determining Total Distance Traveled by a Particle
The final part of the video script addresses the calculation of the total distance traveled by the particle from T equals 0 to T equals 4. The presenter clarifies that this is not as straightforward as finding the difference in position between the two times, as the particle may change direction during its motion. To solve this, the velocity function is analyzed to find when it equals zero, indicating potential changes in direction. By examining the sign of the velocity before and after these points, it is determined that the particle changes direction at T equals 3 and T equals 5. The total distance is then calculated by breaking the problem into segments: from 0 to 3 seconds and from 3 to 4 seconds. The position at these times is found by substituting the values into the position function, and the distances are summed to find the total distance traveled, which is 56 meters.
Mindmap
Keywords
💡Motion
💡Position
💡Velocity
💡Acceleration
💡Jerk
💡Derivative
💡Power Rule
💡Distance Traveled
💡Direction Change
💡Factoring
💡Sign of the Velocity
Highlights
The video focuses on solving problems involving motion, specifically position, velocity, and acceleration.
The position function can represent the height of an object over time, with its derivative providing velocity and the second derivative providing acceleration.
The third derivative of the position function is known as jerk, which is another important term when working with motion problems.
The velocity of a particle after T seconds is found by taking the first derivative of the position function.
Acceleration is found by taking the second derivative of the position function or the first derivative of the velocity function.
The velocity of the particle after T equals zero is calculated by substituting zero into the velocity function.
The acceleration at any given time is found by taking the derivative of the velocity function.
To find the velocity when acceleration is zero, the second derivative (acceleration function) is set to zero and solved for T.
The total distance traveled is not simply the difference in position at two times; it must account for any changes in direction.
The velocity function is used to determine when the particle changes direction by finding when it equals zero and checking the sign around these points.
The total distance traveled from T equals 0 to 4 is calculated by breaking the interval into segments where the direction changes.
The position function is used to find the distance traveled in each segment by calculating the difference in position at the endpoints.
The particle's direction change at T equals 3 and 5 is identified by analyzing the sign of the velocity function around these points.
The total distance traveled from 0 to 4 is the sum of the distances traveled in each segment, accounting for direction changes.
Care must be taken when interpreting derivatives to ensure that the correct physical quantity (velocity, acceleration, etc.) is being solved for.
The importance of understanding the context of the problem and the meaning of the derivatives in relation to the motion of the particle is emphasized.
The video concludes with a reminder to be cautious when solving these types of problems to ensure accurate interpretation of the derivatives.
Transcripts
Browse More Related Video
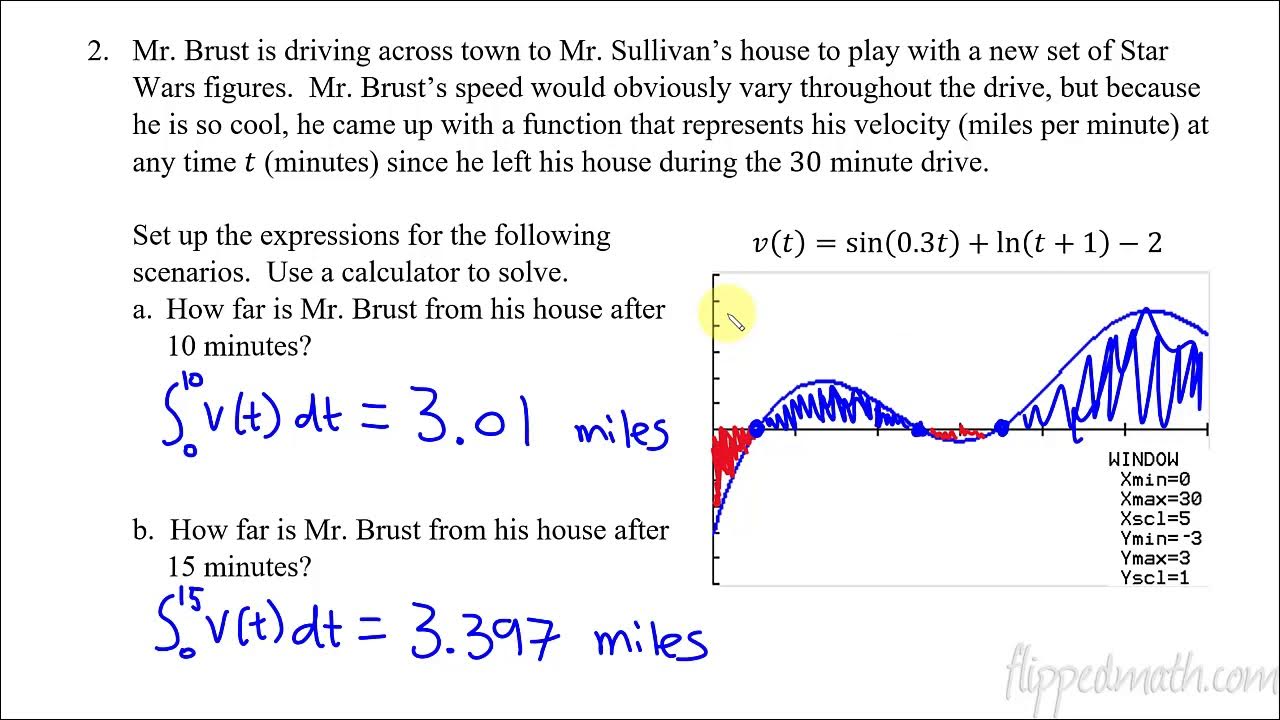
Calculus AB/BC – 8.2 Connecting Position, Velocity, and Acceleration of Functions Using Integrals
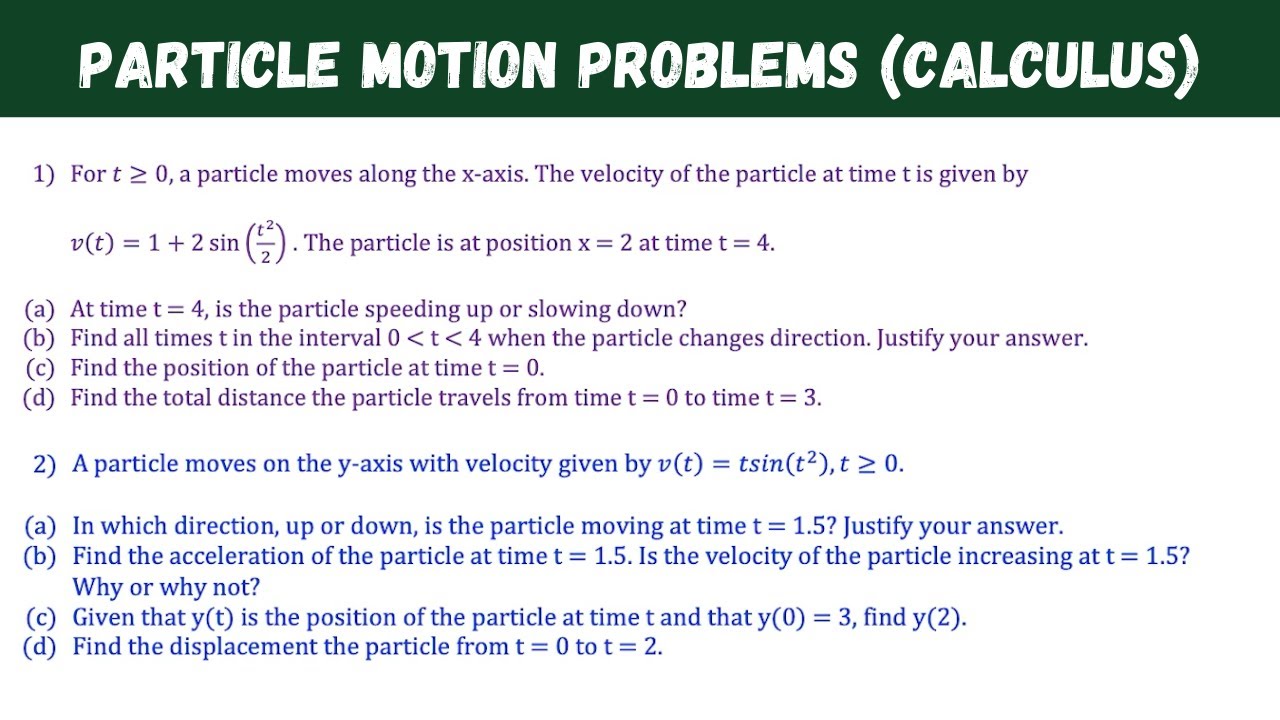
Particle motion problems - Calculus
AP Calculus AB - Straight Line Motion

Particle MotionDay1

2011 Calculus BC free response #1a | AP Calculus BC solved exams | AP Calculus BC | Khan Academy
Motion problems: when a particle is speeding up | AP Calculus AB | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: