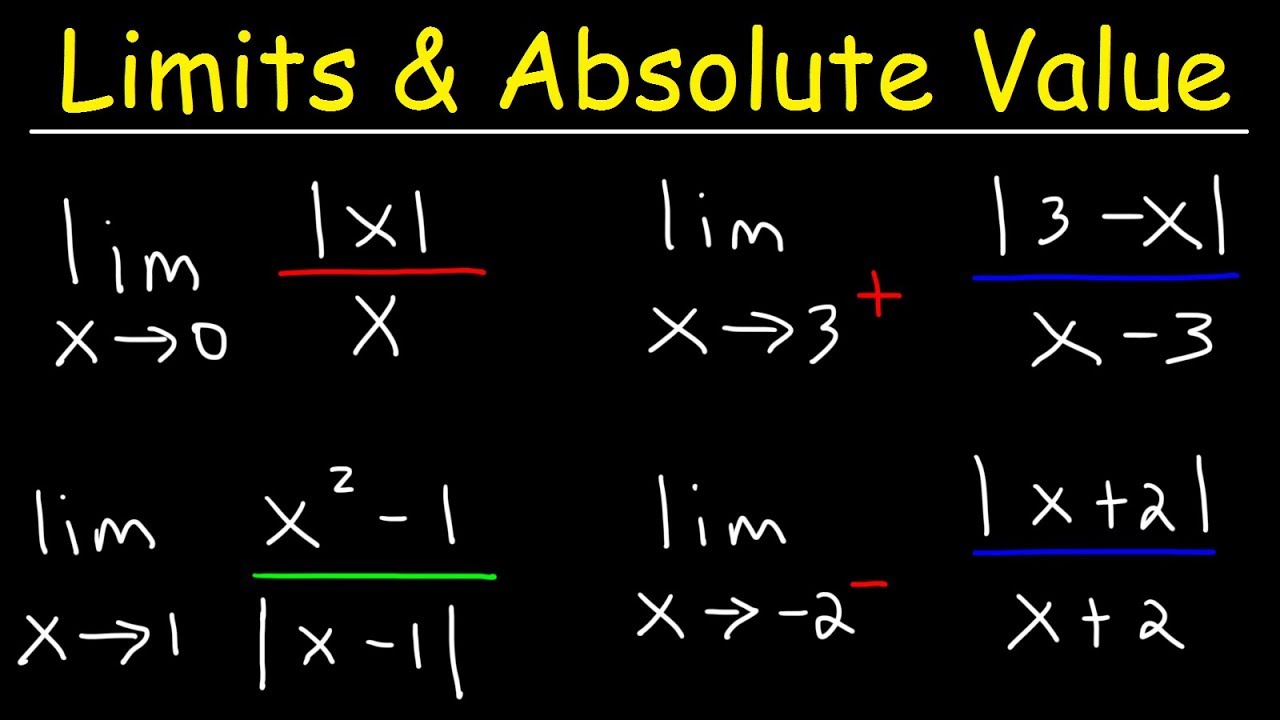Calculus AB - Unit 1 Review
TLDRThis video tutorial explores various calculus problems, focusing on limit calculations. It demonstrates how to handle indeterminate forms, factor expressions to simplify limits, and determine continuity using piecewise functions. The script also covers the intermediate value theorem and identifying discontinuities. Practical examples, such as finding horizontal and vertical asymptotes, are provided, along with a step-by-step approach to solving limit problems, ensuring a comprehensive understanding of the concepts.
Takeaways
- 📚 The video discusses solving limits problems from a math unit review, starting with simplifying expressions to avoid indeterminate forms.
- 🔍 When direct substitution results in an indeterminate form like 0/0, algebraic simplification is necessary to find the limit.
- 📉 For limits involving functions that approach a certain value from the left or right, it's important to consider the behavior of the function as it approaches that value.
- 🤔 The concept of continuity is explored, with a function being continuous at a point if the limit as x approaches that point equals the function's value at that point.
- 📈 The video demonstrates how to find the limit of a function as h approaches zero, which involves simplifying the expression and canceling out the h term.
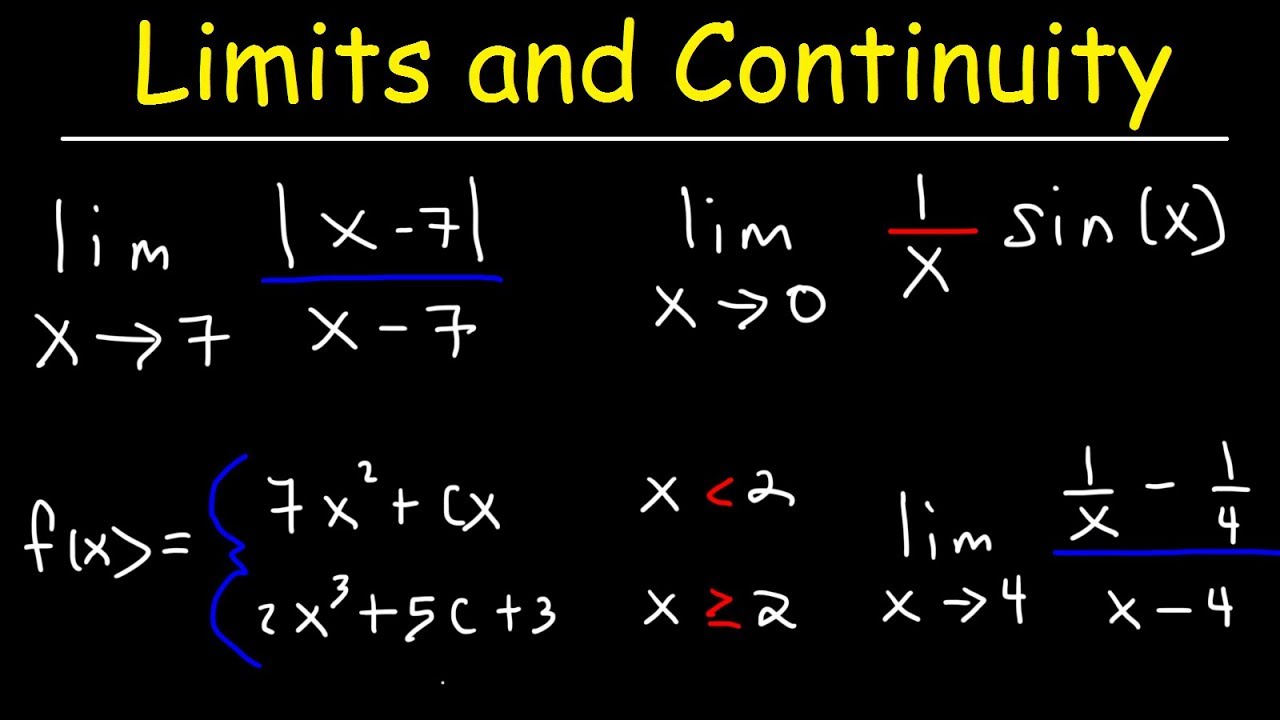
- 📌 The piecewise function's continuity is analyzed by checking if the function's value at a certain point equals the limit from both sides.
- 📊 The script explains how to determine the value of constants in a function to ensure continuity at specific points by solving equations derived from the continuity condition.
- 📐 The intermediate value theorem is mentioned, which guarantees the existence of at least one solution within an interval if the function is continuous and the values at the interval's endpoints are known.
- 📉 The presence of vertical and horizontal asymptotes in a function's graph is identified, with vertical asymptotes occurring where the denominator of a rational function equals zero, and horizontal asymptotes being determined by the behavior of the function as x approaches infinity or negative infinity.
- 📝 The video script also addresses how to determine the reasons for discontinuity in a function, such as when the limit as x approaches a certain point does not equal the function's value at that point.
- 🔢 The use of calculators is suggested for finding specific values or solving equations that cannot be easily done by hand, such as finding the exact point where a function equals a certain value within a given interval.
Q & A
What is the first problem discussed in the video, and what is the indeterminate form encountered?
-The first problem is finding the limit as X approaches 0 of a given function. The indeterminate form encountered is 0 over 0, which occurs when direct substitution is attempted.
How is the indeterminate form in the first problem resolved?
-The indeterminate form is resolved by simplifying the expression algebraically, factoring out an X cubed from both the numerator and the denominator, and then finding the limit by substitution, which results in a limit of 2.
What is the second problem in the video about, and what is the issue with direct substitution?
-The second problem is about finding the limit as X approaches 2 from the left for a given function. Direct substitution results in a non-zero number divided by zero, indicating an approach to either positive or negative infinity.
How does the video determine the limit of the second problem?
-The video determines the limit by considering the behavior of the function as X approaches 2 from the left, noting that the denominator approaches zero from the left, resulting in a positive infinity limit.
What is the definition of continuity used in the video for the third problem?
-The video uses the three-part definition of continuity, which states that for a function to be continuous at a point, the function value at that point must equal the limit of the function as X approaches that point from both the left and the right.
How is the value of K determined in the fourth problem to ensure continuity?
-The value of K is determined by setting the function value at X equals 2 equal to the limit of the function as X approaches 2. By solving the equation, it is found that K must equal 3 for the function to be continuous at X equals 2.
What is the horizontal asymptote of the function in the fifth problem, and how is it determined?
-The horizontal asymptote of the function in the fifth problem is y equals 2. It is determined by observing the behavior of the function as X approaches both positive and negative infinity, as well as by comparing the leading coefficients of the numerator and denominator.
How does the video justify the existence of a vertical asymptote in the fifth problem?
-The existence of a vertical asymptote is justified by identifying the values of X that make the denominator zero (in this case, X equals plus or minus three) and confirming that the numerator does not also become zero at these points.
What are the conditions for the function G in the sixth problem to be continuous at X equals 3?
-For the function G to be continuous at X equals 3, the left-hand limit, right-hand limit, and the function value at X equals 3 must all be equal. The video shows that the left and right-hand limits are equal, and thus the function is continuous at this point.
What is the reason given in the seventh problem for the function G not being continuous at X equals 3?
-The reason given for the function G not being continuous at X equals 3 is that the limit as X approaches 3 of G does not equal G of 3. The left and right-hand limits are equal to each other, but they are not equal to the value of the function at X equals 3.
What does the intermediate value theorem guarantee in the eighth problem, and which statement reflects this?
-The intermediate value theorem guarantees that if a function is continuous on a closed interval and takes two values, then it must take every value between those two. Statement 1 is the one that reflects this, as it states that f of X equals 13, which is between f of 2 and f of 4, indicating the existence of at least one solution on the interval.
In the ninth problem, how is the limit as X approaches 1 from the right of a given expression found?
-The limit is found by using the properties of limits to split the expression into the product of two limits, evaluating each limit separately, and then combining the results to find the overall limit.
What is the condition for a vertical asymptote in the tenth problem, and how is it justified?
-A vertical asymptote occurs where the denominator of a rational function is zero. In the tenth problem, the condition is justified by showing that the limit as X approaches the value that makes the denominator zero results in either positive or negative infinity.
How are horizontal asymptotes determined in the tenth problem?
-Horizontal asymptotes are determined by taking the limit as X approaches both positive and negative infinity of the function. If these limits result in a finite value, it indicates the existence of a horizontal asymptote at that value.
What does the intermediate value theorem guarantee in the eleventh problem, and how is the value of C found?
-The intermediate value theorem guarantees the existence of a value C in the interval from 1.5 to 2.5 such that H of C equals a given value (-4 in this case). The value of C is found by setting the function equal to -4 and using a graphing calculator to find the point of intersection, which is approximately 2.354.
Outlines
📚 Calculus Limit Problems
This paragraph discusses solving calculus limit problems from a unit review. The speaker begins by simplifying a limit expression with an indeterminate form of 0/0, factoring out x^3 and finding the limit to be 2. Next, the limit as x approaches 2 from the left for a function is analyzed, leading to the conclusion of positive infinity due to the behavior of the function around x=2. The third problem involves finding the derivative of a function at x=0, simplifying the expression and canceling terms to find the limit. The fourth problem examines the continuity of a piecewise function at x=2, determining the value of K for continuity. The fifth problem involves analyzing the behavior of a rational function with a horizontal asymptote and vertical asymptotes, finding the values of A and B that ensure the function's continuity and behavior at infinity.
📘 Evaluating Limits and Continuity
The second paragraph continues the theme of limits and continuity, addressing the existence of limits and the continuity of functions at specific points. It starts with an analysis of the limit of a function as x approaches 2 and 3, confirming the existence of these limits and their values. The paragraph then discusses the continuity of a function at x=3, using the definition of continuity to determine that the function is indeed continuous at this point. The speaker also evaluates the truth of several statements regarding the existence of limits and continuity based on the provided graph and function definitions.
🔍 Intermediate Value Theorem and Discontinuity Analysis
This paragraph delves into the application of the intermediate value theorem and the reasons for discontinuity in functions. It starts by examining the truth of several statements related to the behavior of a function on a given interval, using the theorem to confirm the existence of a value within the interval where the function equals a specific y-coordinate. The speaker then identifies reasons for discontinuity in a piecewise function at x=3, concluding that the limit does not equal the function's value at that point. Lastly, the paragraph discusses the conditions under which the intermediate value theorem guarantees the existence of a function's zero within an interval.
📉 Graph Analysis and Function Continuity
The focus of this paragraph is on analyzing the graph of a function to determine continuity and identify points of discontinuity. The speaker explains how to use the three-part definition of continuity to determine why a function is not continuous at a specific point, using the left and right-hand limits and the function's value at that point. The paragraph also explores the conditions for a piecewise function to be continuous everywhere, solving equations to find the values of a and b that ensure continuity at the domain splits.
📌 Limits, Asymptotes, and Intermediate Value Theorem Application
The paragraph discusses finding limits of a function as x approaches specific values, identifying vertical and horizontal asymptotes, and applying the intermediate value theorem. The speaker calculates the limit of a function as x approaches π/2 and identifies a vertical asymptote at x=1 by showing the limit approaches infinity or negative infinity. Horizontal asymptotes are determined by evaluating the limit as x approaches both positive and negative infinity. The intermediate value theorem is used to guarantee the existence of a value c within a given interval where the function equals a specific y-coordinate, and the value of c is approximated using a graphing calculator.
📐 Continuity and Discontinuity in Functions
This final paragraph examines the continuity and discontinuity of functions, particularly focusing on a function with a piecewise definition. The speaker identifies a single point of discontinuity at x=1 and confirms that the function is continuous on the interval from 1.5 to 2.5. The values of the function at the endpoints of this interval are calculated, and the intermediate value theorem is used to establish the existence of a value c within the interval where the function equals a given y-coordinate. The value of c is found using a graphing calculator, and it is verified to lie within the specified interval.
Mindmap
Keywords
💡Limit
💡Indeterminate Form
💡Factoring
💡Continuity
💡Horizontal Asymptote
💡Vertical Asymptote
💡Intermediate Value Theorem
💡Piecewise Function
💡Discontinuity
💡Algebraic Manipulation
Highlights
The video begins with an explanation of how to find the limit of a function as X approaches 0, highlighting the indeterminate form 0/0.
A method for simplifying expressions involving X to the power of 6 and X cubed is demonstrated to find the limit by substitution.
The concept of factoring out X cubed to simplify the limit calculation is introduced.
The limit of a function as X approaches 2 from the left is discussed, involving the behavior of the function near a critical point.
The approach to determine if the limit exists by examining the left and right-hand limits is explained.
A piecewise function's continuity at a specific point is analyzed using the three-part definition of continuity.
The process of finding the value of K for a function to be continuous at X equals 2 is shown.
The existence of a horizontal asymptote at y equals 2 for a given function is discussed, along with its implications.
The method to determine the value of B in a function by analyzing vertical asymptotes is presented.
The use of the intermediate value theorem to evaluate the truth of certain statements about a function's behavior is explained.
A detailed analysis of the reasons why a function G might not be continuous at X equals 3 is provided.
The conditions under which the intermediate value theorem guarantees the existence of a value C within a specific interval are outlined.
The calculation of the limit of a function as X approaches a specific value using properties of limits is demonstrated.
The identification of discontinuities in a function G by examining the left and right-hand limits is shown.
The determination of values for a and b that ensure a piecewise function's continuity everywhere is discussed.
The process of finding the limit of a function H as X approaches PI/2, including simplification techniques, is explained.
The identification of vertical asymptotes in a function by setting the denominator to zero and justifying their existence is shown.
The method to determine horizontal asymptotes by evaluating the limit as X approaches infinity and negative infinity is presented.
The explanation of why the intermediate value theorem guarantees a value C such that H of C equals a specific value within an interval is given.
A practical application of the intermediate value theorem to find an approximate value of C where the function equals a given value is demonstrated.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: