Calculus AB/BC – 8.2 Connecting Position, Velocity, and Acceleration of Functions Using Integrals
TLDRIn this engaging calculus lesson, Mr. Bean guides students through the relationship between acceleration, velocity, and position using integrals. Starting with a particle's acceleration function, he demonstrates how to find the velocity and position functions by integrating. The lesson includes practical examples, such as calculating the position of a particle after a certain time, given its acceleration and initial velocity. Mr. Bean also explores the concept of displacement versus total distance traveled, emphasizing the importance of using the absolute value of velocity when calculating total distance. He concludes with a problem-solving approach to finding the total distance traveled by a particle during a specific time interval, using both graphical and algebraic methods. The lesson is a comprehensive exploration of kinematic equations, ideal for students seeking to deepen their understanding of calculus applications in physics.
Takeaways
- 📚 The relationship between position, velocity, and acceleration is fundamental in calculus, where the derivative of position gives velocity, and the second derivative gives acceleration.
- ➡️ To find the position function from acceleration, integrate the acceleration function twice, accounting for constants of integration.
- ⏱️ Given an initial velocity and position at a specific time, you can determine the position function at any time by integrating the velocity and then the acceleration.
- 🚗 An example problem involves calculating the position and distance traveled by a particle with a given acceleration and initial conditions.
- 📊 The integral of velocity over time gives the displacement, while the integral of the absolute value of velocity gives the total distance traveled.
- ⛔ The integral of the absolute value of velocity is not the same as speed; the former gives total distance, while speed is the magnitude of velocity without direction.
- 🔢 For problems involving motion, it's crucial to find when the velocity is zero to split the integral into separate parts that represent different segments of motion.
- 📐 When given a graph of velocity over time, you can visually determine the areas to integrate for calculating displacement or total distance.
- 🔄 If no graph is provided, you must solve algebraically, taking the absolute value of each integral segment to ensure you get the total distance traveled, not just displacement.
- 📝 When calculating the position of a particle after a certain time, you start with the initial position and add the integral of the velocity function over the time interval.
- 🔢 The position function can be found by integrating the velocity function and then using the initial conditions to solve for the constant of integration.
- 📉 Understanding the concept of antiderivatives is essential for calculating position from acceleration and for solving motion problems in calculus.
Q & A
What is the relationship between position, velocity, and acceleration in calculus?
-In calculus, the position function (s(t)) of an object is related to its velocity and acceleration through derivatives. The first derivative of the position function, s'(t), gives the velocity, and the second derivative, s''(t), gives the acceleration.
How does integration relate to finding velocity and position from acceleration?
-Integration is used to find velocity and position from acceleration by working backwards. The integral of acceleration gives velocity, and the integral of velocity gives position.
What is the initial step in finding the position function of a particle given its acceleration?
-The initial step is to find the velocity function by taking the integral of the acceleration function.
How can you determine the constant of integration for the velocity function?
-You can determine the constant of integration for the velocity function by using a given condition, such as the velocity at a specific time.
What is the next step after finding the velocity function in determining the position function?
-The next step is to find the position function by taking the integral of the velocity function.
How is the initial position of a particle used to determine the constant of integration for the position function?
-The initial position of the particle is used to evaluate the position function at time zero, which allows you to solve for the constant of integration.
What is the difference between displacement and total distance traveled?
-Displacement is the straight-line distance between the starting and ending points, while total distance traveled is the entire path length covered by the object, regardless of the direction.
How do you calculate the total distance traveled by a particle?
-You calculate the total distance traveled by taking the integral of the absolute value of the velocity function over the given time interval.
What is the significance of finding when the velocity is zero in calculating total distance?
-Finding when the velocity is zero helps to break the time interval into segments where the velocity is either positive or negative, which simplifies the calculation of the integral for total distance.
How does the concept of absolute value apply to the integral of velocity?
-The absolute value of the integral of velocity gives the total distance traveled, as it accounts for both positive (forward) and negative (backward) movements.
Why is it incorrect to take the absolute value of the integral of velocity to find total distance?
-Taking the absolute value of the integral of velocity would actually yield the magnitude of displacement, not the total distance, because it would convert all values to positive, disregarding the direction of travel.
What is the difference between velocity and speed?
-Velocity is a vector quantity that includes both the speed (magnitude) and direction of an object's motion, while speed is a scalar quantity that only measures the magnitude of the velocity, without direction.
Outlines
📚 Introduction to Calculus of Motion with Integrals
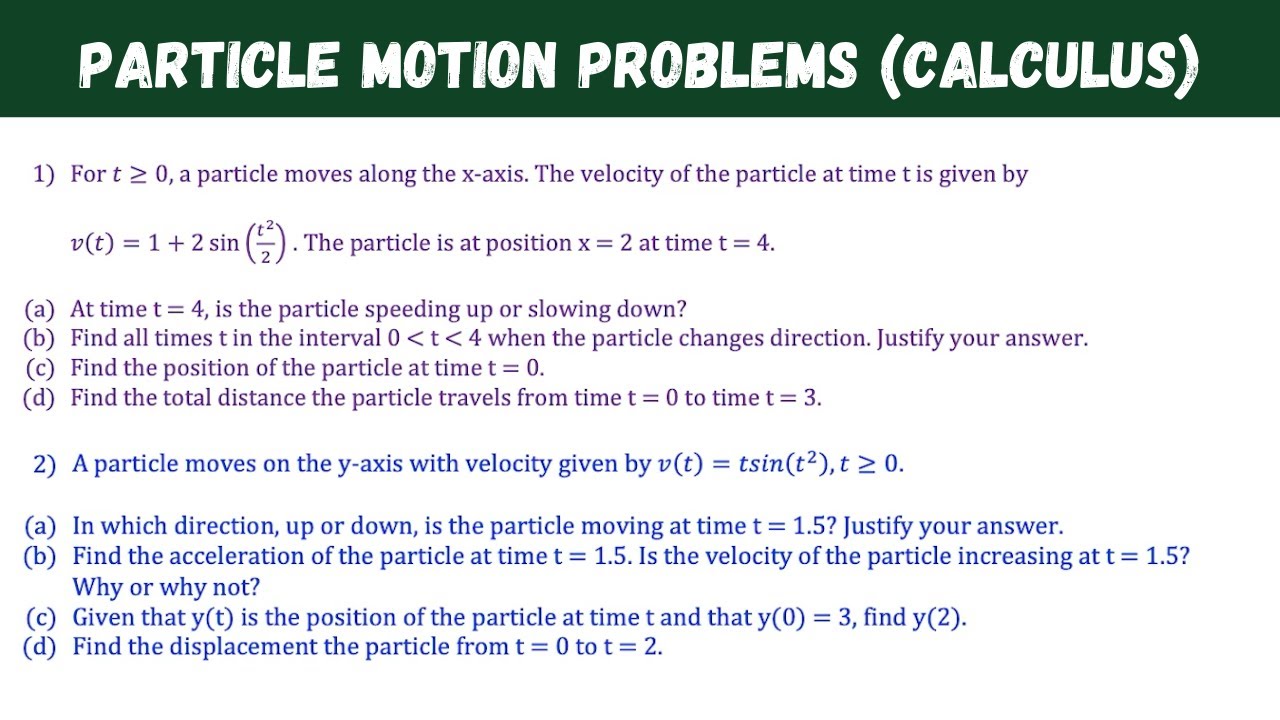
This paragraph introduces the topic of calculus, specifically focusing on the relationship between position, velocity, and acceleration using integrals. Mr. Bean starts by reminding students of the basic derivatives: the first derivative of position (s'(t)) gives velocity, and the second derivative (s''(t)) gives acceleration. The lesson then moves to integrating these quantities to find the others, starting with acceleration to find velocity, then integrating velocity to find position. An example is given involving a particle with a known acceleration function and initial velocity and position, and the process to find the position function x(t) is demonstrated.
🚗 Calculating Distance and Displacement with Velocity Functions
The second paragraph explores the concept of distance and displacement through the use of velocity functions. It presents a scenario involving Mr. Brust driving to Mr. Sullivan's house, with a graph showing his velocity over time. The calculation involves integrating the velocity function from 0 to 10 minutes to find how far Mr. Brust is from his house at the 10-minute mark. The process is then repeated for the 15-minute and 30-minute marks, and the total distance traveled by Mr. Brust is found by integrating the absolute value of the velocity function from 0 to 30 minutes. The difference between displacement, distance, and speed is clarified, emphasizing that the integral of the absolute value of velocity gives total distance, while the integral of velocity gives displacement.
🚀 Applying Calculus to Find Position and Distance Traveled
The third paragraph demonstrates how to use calculus to find the position of a particle after a certain time, given its initial position and velocity function. It first shows how to find the position after three seconds by adding the initial position to the integral of the velocity function over the time interval. The process is repeated for two seconds, starting from an initial position at t=1 second. The paragraph also explains how to calculate the total distance traveled by a particle during the first four seconds, using the concept of integrating the absolute value of the velocity function in different time intervals.
📏 Understanding the Concepts of Distance and Displacement
The final paragraph emphasizes the importance of understanding the difference between distance and displacement. It clarifies that the integral of velocity gives displacement, which is the straight-line distance between the start and end points, regardless of the path taken. In contrast, the integral of the absolute value of velocity gives the total distance traveled. The paragraph also cautions against confusing speed with velocity, as speed is the absolute value of velocity and does not consider direction. The summary concludes with an encouragement to practice these concepts to master the material.
Mindmap
Keywords
💡Position, Velocity, Acceleration
💡Integration
💡Antiderivative
💡Displacement
💡Absolute Value
💡Net Distance
💡Rate of Change
💡Graphical Representation
💡Total Distance
💡Net Change
Highlights
The lesson focuses on the relationship between position, velocity, and acceleration using integrals.
Derivatives of position functions give velocity and acceleration, while integrals allow solving for position and velocity from acceleration.
An example problem involves finding the position function x(t) given an acceleration function and initial conditions.
Integration of the acceleration function gives the velocity function, which can then be integrated again to find the position function.
A second example involves calculating the distance traveled by a person driving to a destination with changing velocity.
The integral of velocity gives displacement, while the integral of the absolute value of velocity gives total distance traveled.
The lesson emphasizes the difference between displacement, total distance, and speed, noting that speed is the absolute value of velocity.
Another example calculates the position of a particle after a certain time given its initial position and velocity function.
The integral of the velocity function over a time interval gives the displacement and final position of the particle.
The lesson provides a method for calculating total distance traveled by finding when velocity is zero and breaking the integral into separate parts.
When working with a graph, it's easy to determine the intervals for integration, but algebraic manipulation is needed without a graph.
The final example calculates the total distance traveled by a particle during a time interval with a given velocity function.
The key takeaway is to use the integral of the absolute value of velocity to find total distance, not just displacement or net change.
The lesson provides a solid foundation for solving problems involving position, velocity, and acceleration using calculus.
Practical applications of these concepts can be seen in real-world scenarios like calculating distances traveled with changing velocities.
The lesson emphasizes the importance of understanding the mathematical concepts and being able to apply them to a variety of problems.
The instructor provides helpful examples and explanations to guide students through the problem-solving process.
The lesson concludes by encouraging students to practice and master the concepts for success in related assessments.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: