AP Calculus AB 2012 Multiple Choice (calculator) - Questions 76 - 92
TLDRThe video transcript is a comprehensive walkthrough of the 2012 AP Calculus AB multiple-choice section by a presenter named Van. It covers a range of calculus topics, including the behavior of functions (increasing/decreasing, concavity), the application of the intermediate value theorem, finding slopes of graphs at certain points, calculating average velocities, analyzing derivatives to determine local maxima and points of inflection, and using integral calculus to find net changes and volumes. The presenter uses the graphing and derivative functions of a calculator to solve problems and emphasizes the importance of understanding calculus concepts and applying them correctly. The video concludes with a problem involving Cavalieri's principle to calculate the volume of a region bounded by two curves. Throughout the script, Van provides step-by-step explanations, making the complex concepts of calculus more accessible to students preparing for the AP exam.
Takeaways
- 📈 The concept of a function being increasing and concave up is explained through the example of question 76, where f'(x) being positive indicates an increasing function and f''(x) > 0 indicates a concave up shape.
- 🌀 The intermediate value theorem, discussed in question 77, guarantees that a continuous function will take on every value between its given function values, hence f(x) = 13 must have at least one solution between f(2) = 10 and f(4) = 20.
- 🔍 For question 78, finding the slope of a graph at a point where it crosses the x-axis involves finding the root of the function and then evaluating the derivative at that root.
- 🚀 The average velocity from t=0 to t=8, as in question 79, is calculated using the average value formula, which in this context is the integral of the velocity function over the time interval divided by the length of the interval.
- 📉 In question 80, understanding the relationship between the graph of the derivative and the original function's behavior is crucial, such as determining where the function has a relative minimum or is concave down.
- 💧 The concept of integrating a rate to find a net change, as in question 81, is a fundamental topic in AP calculus, where the integral of the rate of water being pumped into a tank gives the total amount of water in the tank after a certain time.
- 🔑 Question 82 focuses on identifying where a function has a local maximum by looking for where the derivative changes from positive to negative.
- 🏃 The best estimate of distance traveled, as in question 83, can be approximated by calculating the area under the velocity function using methods like the midpoint Riemann sum.
- 📌 Question 84 deals with determining intervals where a graph is concave down by finding where the second derivative of the function is less than zero.
- ✅ For question 85, identifying where a function has a local maximum from the graph of its derivative involves finding where the sign of the derivative changes from positive to negative.
- 🔍 Question 86 uses the information that the integral of a function from 4 to 7 is zero to deduce a table of values for the function that starts negative and ends positive, reflecting equal areas above and below the x-axis.
- 📏 Question 87 applies the concept of concavity to determine where a function is concave down by looking for intervals where the second derivative graph is below the x-axis.
- 🚶 The related rates problem in question 88 involves using similar triangles to find the rate at which a person's shadow lengthens as they walk away from a street light.
- 🚀 Question 89 involves finding the final velocity of an object given its acceleration function and initial velocity by using the concept that the integral of acceleration is velocity.
- 🔩 The final question demonstrates the use of Cavalieri's principle to calculate the volume of a solid formed by revolution by integrating the difference of two functions over a given interval.
Q & A
What does it mean for a function to be 'concave up'?
-A function is 'concave up' when its second derivative is positive, which graphically means the curve is 'smiling' or has an upward-facing U-shape.
What is guaranteed by the Intermediate Value Theorem for a continuous function?
-The Intermediate Value Theorem guarantees that for a continuous function, if you have two function values, the function must take on every value between those two values at least once.
How do you find the slope of a graph at a specific point where it crosses the x-axis?
-To find the slope of the graph at the point where it crosses the x-axis, you first determine the x-coordinate (c) of the crossing point where the function value is zero, and then evaluate the derivative of the function at that x-coordinate (f'(c)).
What is the formula for calculating the average velocity of a particle over an interval?
-The formula for calculating the average velocity (v_avg) over an interval from t=a to t=b is given by (1/(b-a)) * ∫[a, b] v(t) dt, where v(t) is the velocity function and the integral represents the total change in velocity over the interval.
How can you determine if a function has a relative minimum at a specific point using the graph of its derivative?
-To determine if a function has a relative minimum at a specific point, you look at the graph of its first derivative (f'). If f' changes from negative to positive at that point, it indicates a relative minimum.
What is the significance of the integral of a rate in the context of calculus?
-The integral of a rate, such as gallons per minute in a flow problem, represents the net change in the quantity over a given time interval. It is used to calculate the total amount of something accumulated over time.
How does the graph of a function's derivative (f') relate to the concavity of the original function (f)?
-The concavity of the original function (f) is related to the sign of the second derivative (f''). If f'' is positive, the function is concave up, and if f'' is negative, the function is concave down. The graph of f' shows where the slope is increasing or decreasing, which corresponds to the concavity of f.
What is the concept of Cavalieri's principle in the context of finding the volume of a solid?
-Cavalieri's principle states that if two solids have the same height and the areas of their corresponding cross-sections are equal, then the volumes of the two solids are equal. It is used to calculate the volume of a solid by integrating the product of the thickness of the cross-sections and the difference between the upper and lower bounding functions over a given interval.
How do you find the distance traveled by an object given its velocity function?
-The distance traveled can be found by calculating the definite integral of the velocity function over the time interval of interest. This integral represents the area under the curve of the velocity function, which corresponds to the total distance covered by the object.
What does it mean for a function to have a local maximum, and how can you find it?
-A local maximum is a point on the graph of a function where the function value is greater than the function values at neighboring points. To find a local maximum, you look for points where the first derivative changes from positive to negative.
How can you use the given values of a function's derivative to determine the concavity of the function?
-By analyzing the given values of the function's derivative (f'), you can determine if the derivative is increasing or decreasing. If f' is decreasing, it implies that the second derivative (f'') is negative, and thus the function is concave down. Conversely, if f' is increasing, f'' is positive, and the function is concave up.
Outlines
📈 Understanding Increasing Functions and Concavity
The video begins with an exploration of a calculus problem involving the conditions under which a function f is increasing and concave up. Van, the presenter, explains that if the derivative f' is positive, the function is increasing, and if the second derivative f'' is also positive, the function is concave up. This is visualized as the 'smiling' part of the curve. The problem is solved by identifying the correct point on the curve where these conditions are met, which corresponds to choice E.
🔍 Applying the Intermediate Value Theorem
The next topic is the application of the Intermediate Value Theorem to a continuous function on a closed interval. The theorem guarantees that for any two function values within the interval, the function will take on every value between them. By plotting the given function values and understanding the theorem, Van concludes that the function must have at least one solution at f(x) = 13, which is between the given values of 10 and 20, leading to the selection of choice A.
🧮 Calculating the Slope at a Specific Point
The video then addresses a problem involving finding the slope of a graph at the point where it crosses the x-axis. Van uses a calculator to find the root of the function where it intersects the x-axis, denoted as x=c. After finding the root, the derivative at that point is calculated, resulting in a slope of 2.961. This leads to the selection of choice D as the correct answer.
🚗 Calculating Average Velocity
The discussion moves on to calculating the average velocity of a particle over a given time interval. Van uses the average value formula to find the average value of the velocity function from t=0 to t=8. By understanding the relationship between the integral of the velocity function and the average velocity, Van matches the calculated value to choice B.
📊 Analyzing the Graph of a Derivative
The video continues with an analysis of the graph of f', the derivative of a function f. Van evaluates several statements about the graph, determining which must be true based on the behavior of f'. After careful examination, Van concludes that statements I and III are true, which corresponds to choice E.
💧 Calculating Water Volume in a Tank
The topic shifts to a problem involving the calculation of water volume in a tank being filled at a certain rate. Van uses the concept of the integral of the rate to find the net change in water volume over 20 minutes, starting with an initial 800 gallons. The integral calculation results in a total of approximately 1220 gallons, which rounds to choice D.
🔺 Finding Local Maximums and Concave Intervals
The video concludes with a series of calculus problems. Van addresses questions about finding local maxima, the intervals where a function is concave down, and estimating distances traveled by a car. Each problem is approached methodically, with the application of appropriate calculus concepts such as derivatives and integrals. The solutions are matched to the provided answer choices.
📏 Cavalieri's Principle for Volume Calculation
The final topic is the application of Cavalieri's principle to calculate the volume between two curves. Van explains how to set up the integral by considering the cross-sections perpendicular to the x-axis as squares. By integrating the difference of the two functions squared over the given interval, the volume of the solid is found to be 0.129, which corresponds to choice A.
Mindmap
Keywords
💡Concave Up
💡Intermediate Value Theorem
💡Derivative
💡Average Velocity
💡Point of Inflection
💡Cavalieri's Principle
💡Definite Integral
💡Relative Minimum
💡Continuous Function
💡Velocity Function
💡Second Derivative
Highlights
The concept of f'(x) being positive indicates that the function f(x) is increasing.
f'(x) increasing implies f''(x) > 0, which means the function f(x) is concave up.
The Intermediate Value Theorem guarantees that a continuous function will take on every value between given function values.
To find the slope of a graph at a point where it crosses the x-axis, one must calculate the derivative at that point.
The average velocity of a particle from t=0 to t=8 can be found using the average value formula.
The graph of f'(x) can be used to determine where f(x) has a relative minimum by looking for a sign change from negative to positive.
The integral of the rate of water being pumped into a tank can be used to find the total amount of water in the tank after a certain time.
Finding a local maximum of a function involves looking for where the derivative changes from positive to negative.
Estimating the distance traveled can be done by approximating the area under a velocity curve using a midpoint Riemann sum.
The concavity of a function can be determined by analyzing the sign of the second derivative, f''(x).
Cavalieri's principle can be applied to find the volume of a solid formed by revolving regions around an axis.
A function that is always increasing has an integral equal to zero over an interval if the areas above and below the x-axis are equal.
A graph of the second derivative can help identify intervals where the function is concave down by looking for negative regions.
Related rates problems, such as the rate at which a shadow lengthens, can be solved using similar triangles and derivatives.
The integral of the acceleration function from an initial time to a final time can determine the final velocity of an object.
u-Substitution, or in this case t-substitution, can be used to evaluate integrals by changing the variable to simplify the integrand.
A polynomial function's behavior, such as concavity and points of inflection, can be inferred from the monotonicity of its derivative.
Cavalieri's principle allows for the calculation of the volume of a solid by integrating the area of cross-sections perpendicular to an axis.
Transcripts
Browse More Related Video
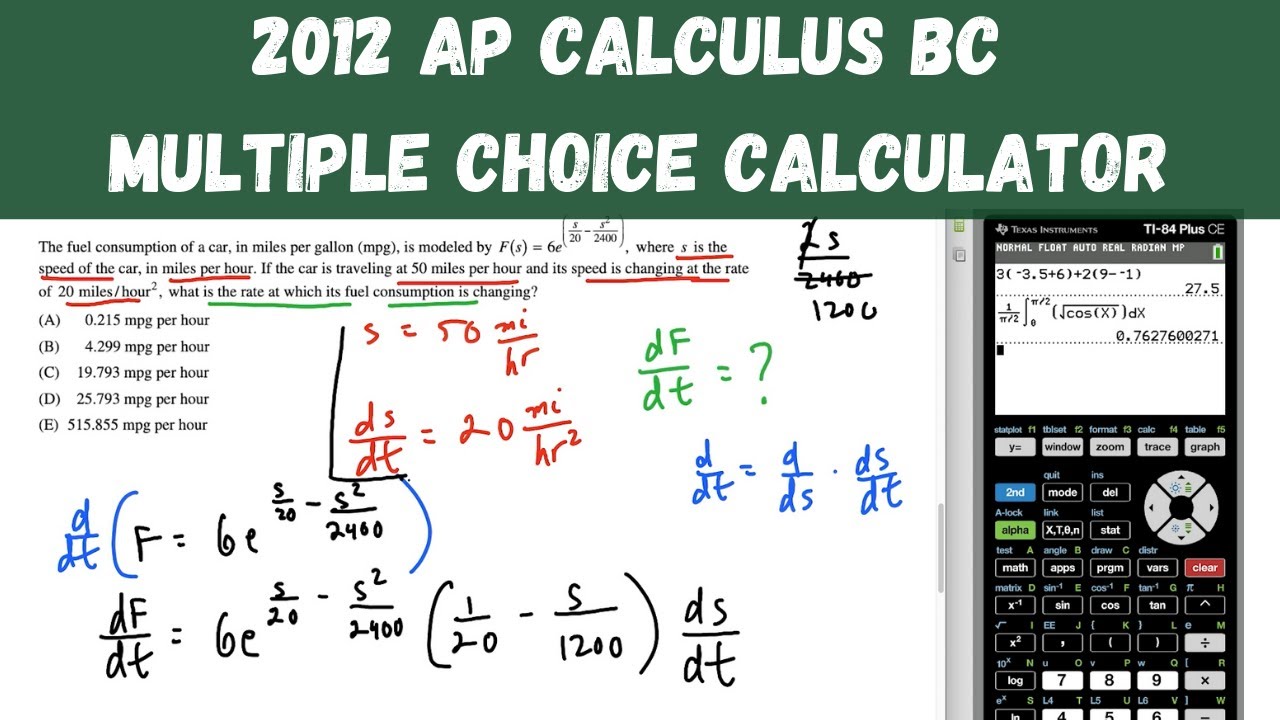
AP Calculus BC Practice Exam 2012 - Calculator Multiple Choice questions 76-92

AP Calculus BC 2003 Multiple Choice (calculator) - Questions 76 - 92

2012 AP Calculus: AB FRQ Solutions
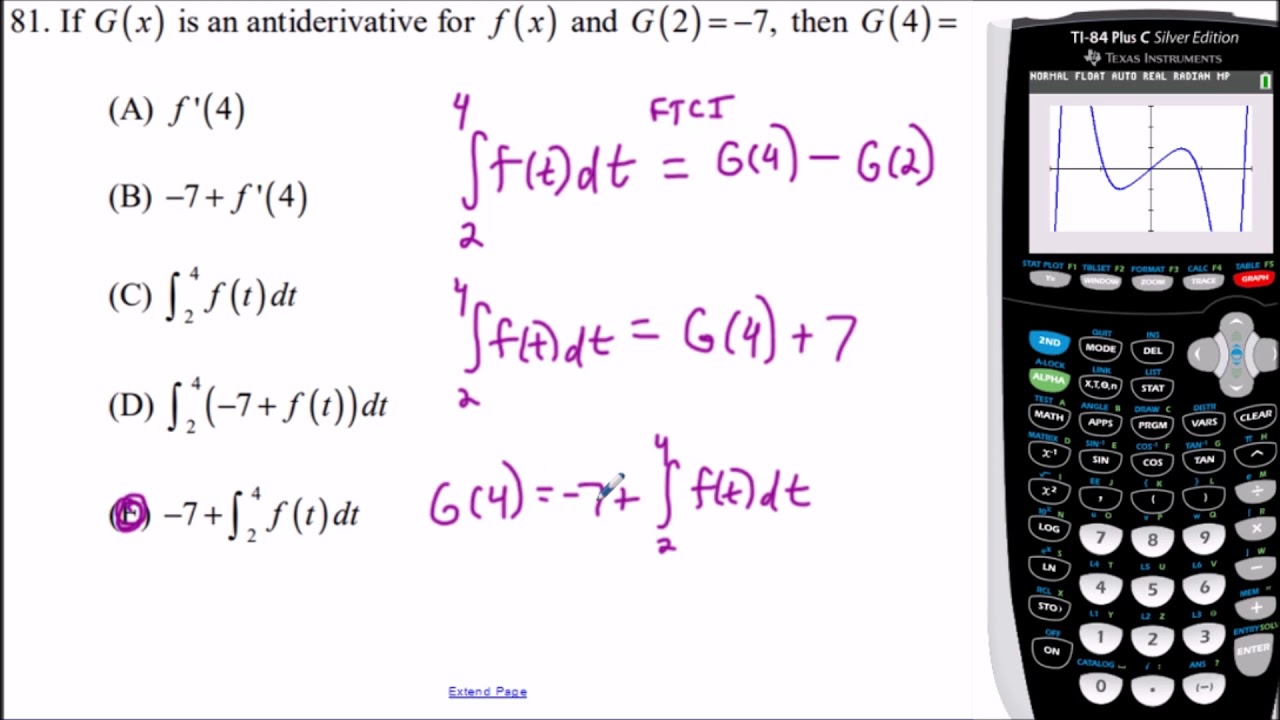
AP Calculus AB 2008 Multiple Choice (Calculator) - Questions 76-92

Math Test Practice Test [ 30 Minutes ] Math for Business and Economics
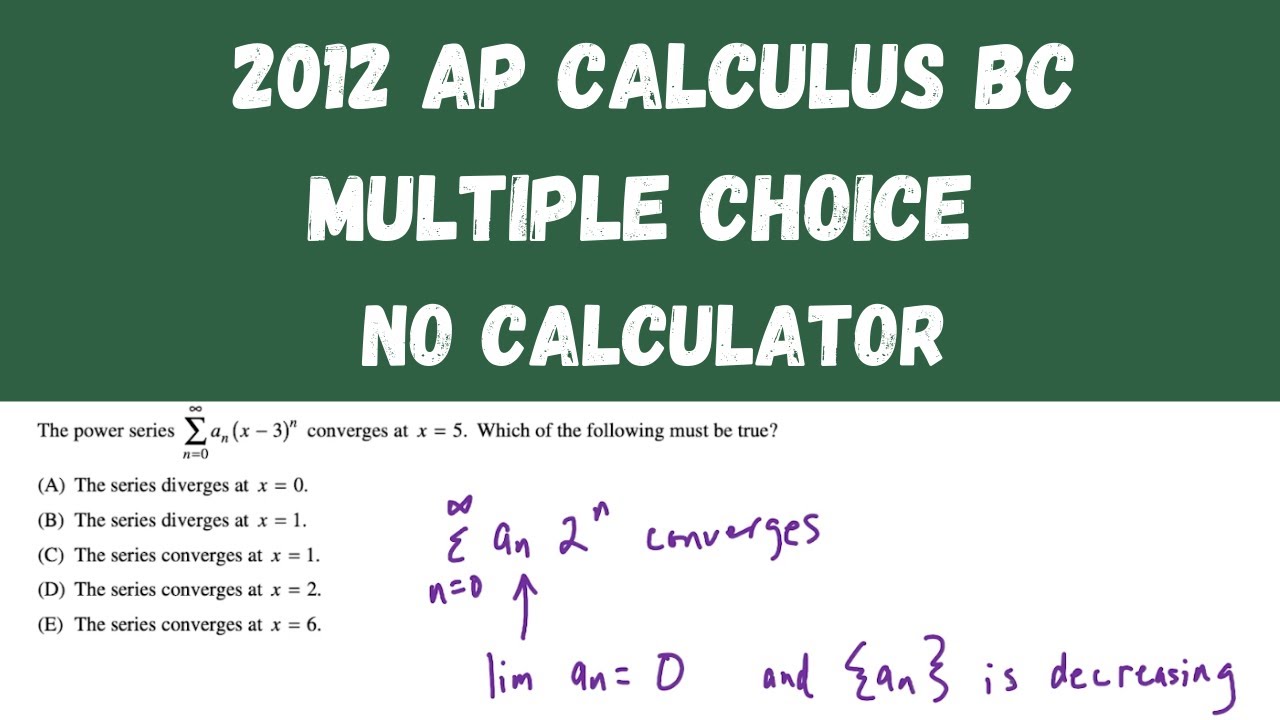
AP Calculus BC Practice Exam 2012 - Multiple Choice questions 1-28
5.0 / 5 (0 votes)
Thanks for rating: