Motion problems: when a particle is speeding up | AP Calculus AB | Khan Academy
TLDRThe video script discusses the concept of a particle's motion along a number line and how to determine when it is speeding up. It introduces the position function s(t) = t^3 - 6t^2 + 9t for positive time and explains that speeding up occurs when the particle is moving in a direction and accelerating in the same direction. The script then differentiates the position function to find the velocity function v(t) = 3t^2 - 12t + 9 and plots it to identify intervals where the velocity is positive or negative. By further differentiating to find the acceleration, the script determines the intervals where the particle is speeding up: between the first and second seconds and after the third second. The summary provides a clear understanding of the particle's motion and the conditions for speeding up, engaging viewers with the mathematical underpinnings of motion.
Takeaways
- 📐 **Position Function**: The position of the particle is described by the function \( s(t) = t^3 - 6t^2 + 9t \) for \( t \geq 0 \).
- ⏱️ **Time Domain**: The domain of time is restricted to positive values, meaning \( t \) is greater than or equal to zero.
- 🚀 **Speeding Up Definition**: A particle is considered to be speeding up if it is moving in the direction of its velocity and its acceleration is in the same direction.
- ⬅️ **Leftward Motion**: If moving leftward (negative velocity), speeding up occurs when acceleration is also negative, increasing the negative velocity.
- ➡️ **Rightward Motion**: If moving rightward (positive velocity), speeding up happens when acceleration is positive, increasing the positive velocity.
- 📉 **Slowing Down**: If the direction of velocity and acceleration are opposite, the particle is slowing down, regardless of the velocity's sign.
- 🔄 **Velocity Function**: The velocity \( v(t) \) is the first derivative of the position function with respect to time, resulting in \( v(t) = 3t^2 - 12t + 9 \).
- 📶 **Zero Velocity Points**: The velocity function intersects the t-axis at \( t = 1 \) and \( t = 3 \), where the velocity is zero.
- 🔺 **Parabola Shape**: The velocity function forms an upward-opening parabola, with the vertex indicating the maximum or minimum velocity.
- ⏳ **Acceleration Function**: The acceleration \( a(t) \) is the second derivative of the position function, indicating the rate of change of velocity.
- 📈 **Speeding Up Intervals**: The particle speeds up between \( t = 1 \) and \( t = 2 \) (leftward direction with negative acceleration) and after \( t = 3 \) (rightward direction with positive acceleration).
Q & A
What is the particle's position function with respect to time?
-The particle's position function with respect to time is given by s(t) = t^3 - 6t^2 + 9t, with the domain restricted to positive time (t ≥ 0).
What does it mean for the particle to be speeding up?
-The particle is speeding up in two scenarios: 1) It is moving in the rightward direction (velocity > 0) and its acceleration is also in the rightward direction (acceleration > 0), or 2) It is moving in the leftward direction (velocity < 0) and wants to go faster in that direction (acceleration < 0).
How is velocity related to the position function?
-Velocity is the derivative of the position function with respect to time. It represents the instantaneous rate of change of position, which can be written as v(t) = s'(t) or dv/dt.
What is the expression for the velocity function of the particle?
-The velocity function, which is the derivative of the position function, is v(t) = 3t^2 - 12t + 9.
How can you determine the points where the velocity is zero?
-By setting the velocity function equal to zero (3t^2 - 12t + 9 = 0) and solving for t, you find the points where the velocity is zero. Factoring the quadratic gives (t - 3)(t - 1) = 0, so t = 1 and t = 3.
What is the significance of the vertex of the parabola representing the velocity function?
-The vertex of the parabola represents the minimum or maximum value of the velocity function, depending on the direction of the parabola's opening. In this case, it's an upward-opening parabola, so the vertex represents the minimum velocity, which occurs at t = 2.
What is the acceleration function of the particle?
-Acceleration is the rate of change of velocity with respect to time, which is the second derivative of the position function. It can be written as a(t) = v'(t) or d^2s/dt^2.
How can you determine the intervals where the particle is speeding up?
-You determine the intervals by analyzing the velocity and acceleration functions. Speeding up occurs when the velocity and acceleration have the same sign (both positive or both negative). In this case, the particle speeds up between t = 1 and t = 2 (leftward direction with negative velocity and negative acceleration) and after t = 3 (rightward direction with positive velocity and positive acceleration).
What is the v-intercept of the velocity function?
-The v-intercept is the value of the velocity function when t = 0, which in this case is v(0) = 9, indicating the initial velocity of the particle.
What does the graph of the velocity function look like?
-The graph of the velocity function is an upward-opening parabola with a vertex at t = 2 and intercepts at t = 1 and t = 3, where the velocity is zero. The graph shows positive velocity for t > 3 and t < 1, and negative velocity between t = 1 and t = 2.
How can you tell if the particle is slowing down?
-The particle is slowing down when the velocity and acceleration have opposite signs. If the particle is moving rightward (velocity > 0) and the acceleration is negative (acceleration < 0), or if the particle is moving leftward (velocity < 0) and the acceleration is positive (acceleration > 0), it is slowing down.
What is the physical interpretation of the second derivative of the position function?
-The second derivative of the position function, which is the acceleration function, represents the rate at which the velocity is changing over time. It indicates how the speed or the direction of the particle's movement is changing at any given moment.
Outlines
📐 Understanding Particle Motion on a Number Line
The video script introduces the concept of a particle moving along a number line, starting at the origin (0). It explains the particle's position as a function of time, denoted as s(t), and provides a specific function: t^3 - 6t^2 + 9t, with the domain restricted to non-negative time values (t ≥ 0). The main question addressed is to determine when the particle is speeding up. Speeding up is defined by two scenarios: either the particle is moving rightward (velocity > 0) and accelerating in the same direction (acceleration > 0), or it is moving leftward (velocity < 0) and accelerating leftward (acceleration < 0). The script then introduces the concept of velocity as the first derivative of the position function with respect to time, symbolized as v(t) or ds/dt. The velocity function for the given particle is derived as 3t^2 - 12t + 9 and is used to discuss when the velocity is positive or negative, which is essential for determining the particle's speed and direction of motion.
📉 Analyzing Velocity and Acceleration Graphs
The script continues by graphing the velocity function to understand when the particle's velocity is positive or negative. It identifies the vertical intercept at v(0) = 9 and finds the roots of the velocity function, t = 1 and t = 3, indicating where the velocity is zero. The vertex of the parabola, which represents the maximum velocity, is calculated at t = 2, with a velocity of -3. The graph of velocity as a function of time is sketched, showing the intervals where the velocity is positive and negative. The concept of acceleration is then introduced as the rate of change of velocity, symbolized as the second derivative of the position function with respect to time, a(t) or dv/dt. The acceleration function is described as the slope of the tangent line to the velocity curve. The intervals where the particle is speeding up are identified: between the first and second seconds (when velocity and acceleration are both negative) and after the third second (when both are positive). The summary concludes with the specific time intervals when the particle is speeding up.
Mindmap
Keywords
💡Particle
💡Number Line
💡Time
💡Position Function
💡Velocity
💡Acceleration
💡Derivative
💡Graph
💡Domain Restriction
💡Speeding Up
💡Parabola
Highlights
The particle's motion along the number line is described by the function s(t) = t^3 - 6t^2 + 9t, with the domain restricted to positive time (t ≥ 0).
Speeding up is defined as either moving rightward with positive velocity and positive acceleration, or moving leftward with negative velocity and negative acceleration.
Velocity is the first derivative of the position function with respect to time, indicating the instantaneous rate of change of position.
The velocity function v(t) is derived as 3t^2 - 12t + 9, which will be graphed to understand its behavior over time.
The vertical intercept of the velocity function occurs at v(0) = 9, aiding in graphing the function.
The velocity function intersects the t-axis at t = 1 and t = 3, both resulting in a velocity of 0.
The vertex of the velocity function, indicating the maximum or minimum value, occurs at t = 2 with a velocity of -3.
The graph of velocity as a function of time is an upward-opening parabola, with the vertex between t = 1 and t = 3.
The particle is speeding up (velocity and acceleration both negative) between t = 0 and t = 1, and between t = 1 and t = 2.
Acceleration is the rate of change of velocity, which is the second derivative of the position function with respect to time.
The slope of the tangent line to the velocity function represents the acceleration, indicating whether the particle is speeding up or slowing down.
The particle speeds up in the rightward direction when the velocity is positive and the slope of the velocity function is also positive, occurring after t = 3.
The particle is speeding up between the first and second seconds and after the third second, as indicated by the positive velocity and acceleration in those intervals.
The concept of acceleration is crucial in determining the direction and rate of change in the particle's speed.
The graph of the velocity function helps visualize when the particle is speeding up, slowing down, or moving at a constant speed.
Understanding the relationship between velocity, acceleration, and the position function is key to analyzing the particle's motion over time.
The problem-solving approach involves differentiating the position function to find velocity and acceleration, and then analyzing their graphs to determine the particle's behavior.
Transcripts
Browse More Related Video
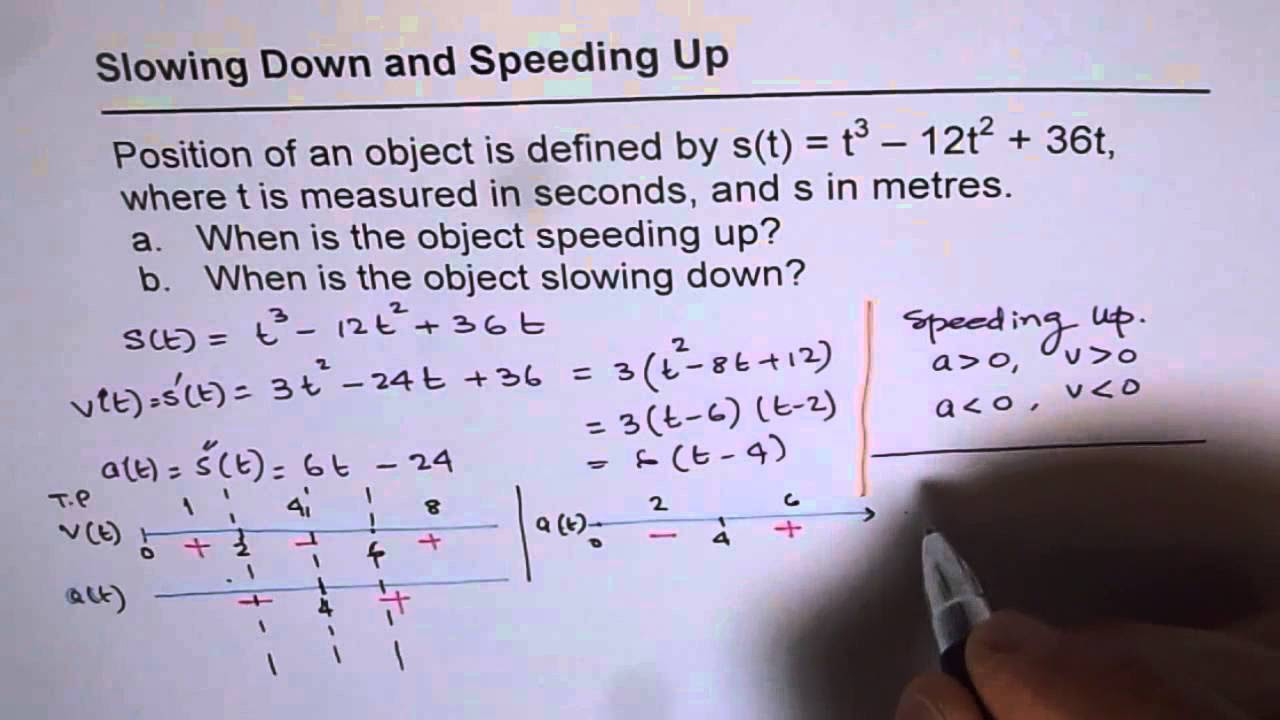
Slowing Down Speeding Up Derivatives Application Calculus MCV4U

Worked example: Motion problems with derivatives | AP Calculus AB | Khan Academy

Particle MotionDay1
2008 AP Calculus AB Free Response #4
Particle motion problems - Calculus
Particle Motion - Speeding Up and Slowing Down (Example, AP Calculus)
5.0 / 5 (0 votes)
Thanks for rating: