Double integral 1 | Double and triple integrals | Multivariable Calculus | Khan Academy
TLDRThis video script delves into the concept of using integrals to calculate the area under a curve, setting the stage for understanding how to extend this principle to find the volume under a surface in three dimensions. It explains the process of breaking down the area into infinitesimally thin rectangles and how this can be translated into calculating the volume by considering thin slices under a surface. The script provides a clear and intuitive explanation, making complex mathematical concepts accessible and engaging for the viewer.
Takeaways
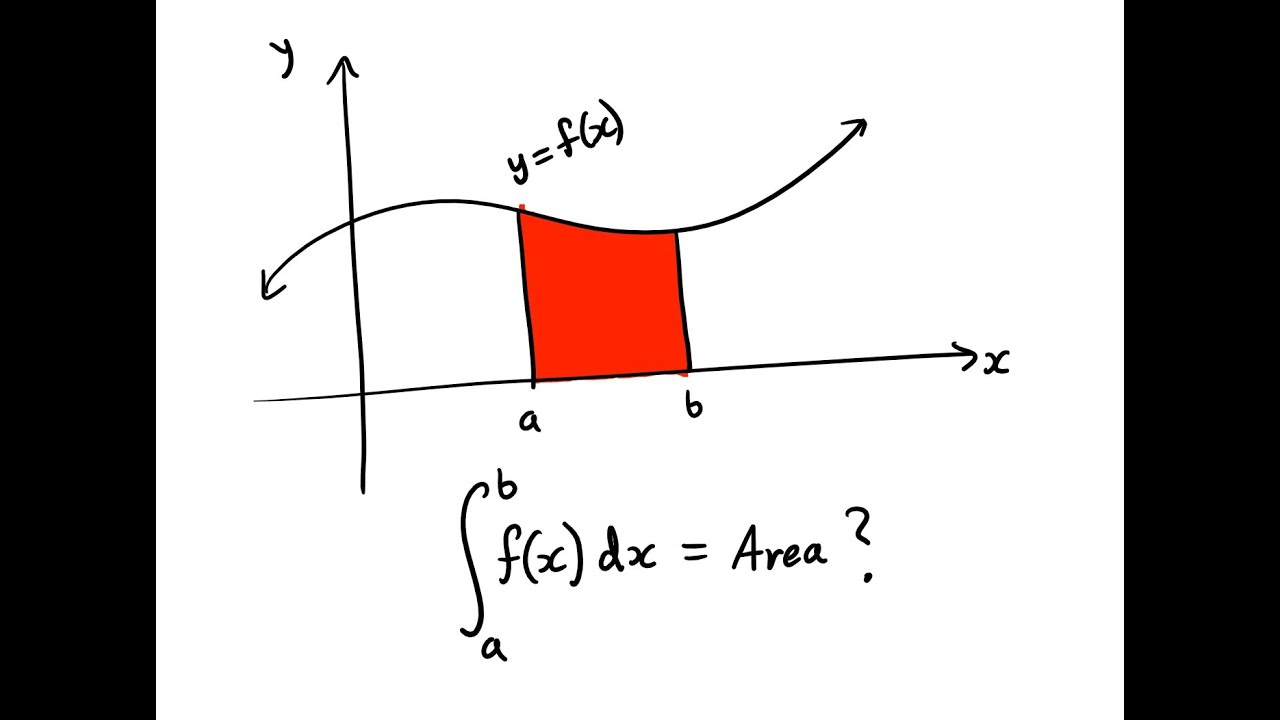
- 📈 Understanding Integrals: The video begins with a review of integrals and their use in calculating the area under a curve.
- 🔄 Intuition Behind Integrals: The process of breaking the area into infinitesimally thin rectangles (columns) and summing their areas is explained.
- 📐 Rectangles and Area: The area of each rectangle is base times height, with base being dx and height being f(x) at that point.
- 🚀 Infinite Summation: By making the rectangles infinitely small (dx) and summing an infinite number of them, we obtain the exact area under the curve.
- 📊 Transition to 3D: The concept of a surface in three dimensions is introduced as a function of x and y, z = f(x, y).
- 🌐 Domain Expansion: The domain for the function has expanded from the x-axis to the xy plane, allowing for x and y inputs.
- 🏞️ Surface Visualization: A surface is visualized as a 3D shape, like a piece of paper bent in space, with height determined by the function's output.
- 🔄 Volume Calculation: The method for calculating the volume under a surface is analogous to area calculation, but in three dimensions.
- 📚 Bounds Specification: To calculate the volume, bounds must be specified, similar to how bounds were set for the definite integral in 2D.
- 🔄 Slicing Volume: The process of 'slicing' the volume into thin layers parallel to the y-axis (dy) is described to find the volume contribution from each layer.
- 📈 Double Integral: The volume under the surface is found by integrating the area function with respect to x (from a to b) and then with respect to y (from 0 to a).
Q & A
What is the primary purpose of integrals as discussed in the script?
-The primary purpose of integrals discussed in the script is to calculate the area under a curve, specifically within the given interval from x=a to x=b.
How does the process of breaking down a curve into infinitesimally small rectangles help in understanding integrals?
-Breaking down a curve into infinitesimally small rectangles helps in understanding integrals by approximating the area under the curve through the sum of the areas of these rectangles. As the rectangles become infinitely small, the sum approaches the exact area under the curve, providing an intuitive understanding of the definite integral.
What is the significance of the 'dx' and 'f(x)' terms in the context of the integral?
-In the context of the integral, 'dx' represents an infinitesimally small change in the x variable, and 'f(x)' is the function that defines the y-coordinate at a given x-coordinate. The product of 'f(x)' and 'dx' gives the height of the rectangle, which when summed (integrated) over the interval, yields the area under the curve.
How does the concept of a surface differ from that of a curve in two-dimensional space?
-A surface in three-dimensional space is defined by a function of two variables, typically z as a function of x and y (z = f(x, y)). This is different from a curve in two-dimensional space, which is defined by a function of one variable (y as a function of x). A surface encompasses the additional dimension of depth or height, whereas a curve is confined to the plane.
What is the domain of a function in the context of a surface?
-In the context of a surface, the domain is the set of all valid combinations of x and y values that can be input into the function to produce a z value. It represents the region in the xy-plane over which the surface is defined.
How does the process of calculating the volume under a surface differ from that of calculating the area under a curve?
-Calculating the volume under a surface involves integrating the cross-sectional area of the surface along the third dimension (typically the z-axis). This is done by first finding the area under a curve (which is a cross-section of the surface at a constant y value) and then integrating this area with respect to the remaining variable (y), thus adding the depth dimension to obtain volume.
What is the significance of the 'dy' term in the volume calculation?
-The 'dy' term represents an infinitesimally small change in the y variable when calculating the volume under a surface. By multiplying the area of a cross-sectional sliver (found by integrating with respect to x) by 'dy', a small volume element is obtained. Integrating this volume element with respect to y over the desired interval yields the total volume under the surface.
How does the process of integrating the area function with respect to y (from y=0 to y=a) contribute to finding the volume?
-Integrating the area function with respect to y from y=0 to y=a sums up the infinitesimally small volume elements, each obtained by multiplying the area of a cross-sectional sliver by 'dy', over the entire range of y values. This summation, when performed correctly, results in the total volume under the surface within the specified bounds.
What is the role of the bounds in calculating the area under a curve or the volume under a surface?
-Bounds are essential in specifying the interval or region over which the calculation is performed. In the case of a curve, bounds define the interval from x=a to x=b, while for a surface, they define the region in the xy-plane within which the volume is to be calculated. Without specifying bounds, the calculations for area or volume would be undefined.
How does the concept of a shadow of the surface on the xy-plane help in visualizing the volume calculation?
-The concept of a shadow of the surface on the xy-plane helps in visualizing the volume calculation by providing a two-dimensional projection of the region under the surface. This projection, or the shadow, represents the area bounds in the xy-plane, which correspond to the volume bounds when considering the third dimension.
What is the practical application of calculating volumes under surfaces?
-Calculating volumes under surfaces has practical applications in various fields such as physics, engineering, and computer graphics. It can be used to determine the volume of materials in 3D printing, the amount of fluid contained within a shaped container, or in modeling and simulation for visual effects in the entertainment industry.
Outlines
📊 Understanding Integrals and Area Under a Curve
This paragraph introduces the concept of using integrals to calculate the area under a curve. It begins with a review of the basic intuition behind integrals, explaining how to find the area under a curve by dividing it into infinitesimally thin rectangles and summing their areas. The process involves setting up the function in the xy-plane, selecting the interval from x=a to x=b, and using the function f(x) to determine the height of each rectangle. The paragraph emphasizes the importance of understanding this foundational concept before moving on to more complex applications such as calculating volumes under surfaces.
📈 Extending the Concept to Volume Under a Surface
The second paragraph builds upon the area under a curve by extending the concept to calculate the volume under a surface in three-dimensional space. It explains that a surface is defined by a function of x and y, and the volume under the surface is found by considering the bounds of the region in the xy-plane. The paragraph discusses the process of visualizing the projection of the surface onto the xy-plane to determine the bounds and how to calculate the volume by summing up infinitesimally thin slices (slivers) of the volume, each represented by an area function of f(x, y) times a small change in y (dy). This lays the groundwork for the next video where the actual calculation of volumes will be demonstrated.
🎥 Intuition Behind Volume Calculation
In the final paragraph, the video script aims to clarify the intuition behind calculating the volume under a surface using integrals. It reiterates the process of breaking down the volume into a series of thin slices, each with a calculable area, and then multiplying by the infinitesimal change in y (dy) to obtain a volume element. The paragraph emphasizes that the process is an extension of the method used for finding the area under a curve, and it assures viewers that the actual calculations for volumes can be quite straightforward, especially when clear x and y bounds are provided. The paragraph concludes by setting the stage for the next video, where the focus will be on practical calculations of volumes under surfaces.
Mindmap
Keywords
💡integrals
💡area under a curve
💡volume under a surface
💡function of x and y
💡xy plane
💡sums
💡infinitely small
💡definite integral
💡surface
💡bounds
💡dx and dy
Highlights
Integrals are used to calculate the area under a curve.
The process involves breaking the area into infinitesimally thin columns or rectangles.
The area of each rectangle is base times height, with base as dx and height as f(x).
Definite integral sums up the areas of these rectangles from x=a to x=b.
The concept of a surface in three dimensions is introduced as a function of x and y.
The domain for a surface is the xy plane, allowing for any real number inputs for x and y.
A surface can be visualized as a height that pops out from the xy plane based on the function z = f(x, y).
Volume under a surface is calculated by specifying bounds for the x and y regions.
The process of calculating volume involves creating a 'shadow' of the surface on the xy plane.
For a constant y, the area under the curve can be viewed as a sliver of the volume.
The area of the sliver is given by the integral of f(x, y) dx from x=0 to x=b.
Multiplying the area of the sliver by dy gives a thin slice of volume.
The volume of the slice is calculated as the integral of f(x, y) dx times dy from y=0 to y=a.
Calculating volumes can be straightforward with fixed x and y bounds.
The method for calculating volume under a surface is an extension of the process used for finding area under a curve.
The video provides a clear and detailed explanation of the mathematical concepts involved in calculating volume under a surface.
The process is intuitively explained by comparing it to the area calculation under a curve, making it easier to understand.
The video concludes with a teaser for the next video, where the actual calculation of volumes will be demonstrated.
Transcripts
Browse More Related Video

Defining Double Integration with Riemann Sums | Volume under a Surface

Double integrals 4 | Double and triple integrals | Multivariable Calculus | Khan Academy
Calculus: Why does integrating a function give area under its curve?

Disc method around x-axis | Applications of definite integrals | AP Calculus AB | Khan Academy

Introduction to the surface integral | Multivariable Calculus | Khan Academy

Introduction to the line integral | Multivariable Calculus | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: