Definite Integrals (part 5)

Khan Academy
22 Oct 200709:57
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the process of calculating the area between two curves, specifically the square root of x and x squared, from 0 to 1. The presenter uses calculus and the concept of antiderivatives to find the area, which simplifies to a neat result of 1/3. An additional problem involving the area under an absolute value function is introduced, highlighting the use of symmetry and algebraic manipulation to solve complex problems.

Takeaways
  • ๐Ÿ“ˆ The problem involves calculating the area between two curves, specifically from x=0 to x=1.
  • ๐ŸŒŸ The first approach is to find the area under the square root of x and then subtract the area under x squared.
  • ๐Ÿ“š The indefinite integral is used to solve the problem, relying on the fundamental theorem of calculus.
  • ๐ŸŽจ The script uses different colors to illustrate the process, with magenta highlighting the main area of interest.
  • ๐Ÿ”„ The antiderivative of the square root of x is found by raising the power to 3/2 and dividing by the new exponent.
  • โž– The final area is determined by evaluating the antiderivative at the bounds and subtracting the results (1/3 in this case).
  • ๐Ÿ”ข The second problem involves finding the area under x to the fifth power up to the height of 32.
  • ๐Ÿ”„ A horizontal line function at y=32 is used as the upper boundary to calculate the area.
  • ๐Ÿ”ข The area is calculated by setting up an integral from -2 to 2, utilizing symmetry to simplify the process.
  • ๐Ÿค” The final calculation involves an algebraic expression that needs to be evaluated and then multiplied by 2 to account for the symmetry.
  • โณ The script ends with an unfinished calculation, leaving the audience to complete the problem on their own.
Q & A

  • What is the area being calculated in the script?

    -The area between the curves y = sqrt(x) and y = x^2 from x = 0 to x = 1.

  • How does the script suggest finding the area under the square root of x from 0 to 1?

    -By finding the indefinite integral of the function sqrt(x) from 0 to 1.

  • What is the significance of subtracting the purple area in the script's explanation?

    -Subtracting the purple area, which represents the area under x^2, helps in determining the area between the two curves.

  • What is the antiderivative of sqrt(x)?

    -The antiderivative of sqrt(x) is (2/3)x^(3/2).

  • What is the antiderivative of x^2?

    -The antiderivative of x^2 is (1/3)x^3.

  • What is the final result of the area between the square root of x and x squared from 0 to 1?

    -The final result is 1/3.

  • How does the script introduce the second problem involving x to the fifth power?

    -The script introduces the second problem by asking for the area inside the curve y = x^5 where the height is 32.

  • What horizontal function is used to find the area inside the curve y = x^5?

    -A constant function g(x) = 32 is used to find the area inside the curve.

  • What is the mistake corrected in the script when discussing the second problem?

    -The mistake corrected is the initial choice of function; instead of y = x^5, it should be y = |x^5| to have a cup-shaped graph.

  • What is the symmetry observed in the second problem?

    -The symmetry observed is that the area inside the curve y = |x^5| where the height is 32 is symmetric about the y-axis, allowing the calculation to be done for one interval and then multiplied by 2.

  • What is the final expression for the area inside the curve y = |x^5| where the height is 32?

    -The final expression is (128 - 128/6) * 2, which simplifies to (128 * 5/6).

Outlines
00:00
๐Ÿ“ˆ Calculating the Area Between Two Curves

The paragraph begins with a discussion on finding the area between two curves, specifically between the square root of x (from 0 to 1) and x squared. The speaker proposes a method to calculate this area by first finding the area under the square root of x and then subtracting the area under x squared. The process involves using the indefinite integral and the fundamental theorem of calculus. The speaker emphasizes the simplicity of the resulting area, which is 1/3, despite the complexity of the curves involved. Additionally, the speaker presents another problem involving the area between x to the fifth and a horizontal line at y equals 32, highlighting the use of symmetry to simplify the calculation.

05:05
๐Ÿ“Š Solving a Tricky Area Problem with a Fifth-Degree Function

This paragraph delves into a trick problem involving the calculation of the area between a fifth-degree function and a horizontal line. The speaker initially makes a mistake by choosing a function that does not produce the desired cup shape but corrects it by considering the absolute value of x to the fifth power. The focus is on finding the area enclosed by the curve where the height is 32 and the x-axis between -2 and 2. The speaker outlines the process of setting up the indefinite integral and leveraging symmetry to find the area. However, the speaker acknowledges the complexity introduced by the initial function choice and leaves the final calculation unfinished, promising to continue in the next session.

Mindmap
Keywords
๐Ÿ’กArea between curves
The term 'area between curves' refers to the region enclosed by two curves but not including the curves themselves. In the video, this concept is central to solving the problem, where the area between the square root of x and x squared from 0 to 1 is calculated. This is a fundamental concept in calculus, often solved using integration techniques.
๐Ÿ’กIndefinite integral
An indefinite integral represents a family of functions that differentiate to the same original function. It is used to find the antiderivative, or the function whose derivative is the original function. In the context of the video, the indefinite integral is used to find the antiderivative of the function โˆšx, which is then used to calculate the area under the curve.
๐Ÿ’กFundamental theorem of calculus
The Fundamental Theorem of Calculus is a key result that connects differentiation and integration. It states that if a function is continuous on a closed interval and has an antiderivative on that interval, then the definite integral of the function over that interval can be computed as the difference of the antiderivative's values at the endpoints of the interval. The video assumes the viewer's familiarity with this theorem and uses it to calculate the area under the curve.
๐Ÿ’กSquare root of x
The square root of x, denoted as โˆšx, is a function that represents the non-negative number which, when squared, equals x. In the video, the square root of x is one of the functions used to define the curve for which the area is being calculated. It is an important concept in the study of algebra and calculus, often used in various mathematical and real-world applications.
๐Ÿ’กx squared
The term 'x squared' refers to the mathematical operation of squaring a number x, which is่ฎก็ฎ—ไธบxไน˜ไปฅ่‡ช่บซ (x * x). In the video, x squared is another function that defines a curve, and the area under this curve is subtracted from the area under the curve of the square root of x to find the final area of interest.
๐Ÿ’กAntiderivatives
An antiderivative, also known as an indefinite integral, is a function that represents the collection of all functions that could be the result of integrating a given function. In the video, the antiderivative of the square root of x and x squared are found to evaluate the integrals and calculate the areas.
๐Ÿ’กEvaluation
In the context of the video, 'evaluation' refers to the process of calculating the value of a function or an expression at a specific point. This is a crucial step in determining the net area under a curve by evaluating the antiderivative at the bounds of the integration.
๐Ÿ’กSymmetry
In mathematics, symmetry refers to a property of an object or a function that allows it to be mapped onto itself in a certain way. In the video, the concept of symmetry is used to simplify the calculation of the area between the absolute value of x to the fifth and a horizontal line at y = 32 by recognizing that the shape is symmetric about the y-axis.
๐Ÿ’กAbsolute value
The absolute value of a number is its non-negative value, regardless of its sign. It is denoted by two vertical lines on either side of the number. In the video, the absolute value is used to correct a mistake in the choice of function, changing x to the fifth to the absolute value of x to the fifth, which results in a curve that has a 'cup' shape and is symmetric about the y-axis.
๐Ÿ’กDefinite integral
A definite integral represents the signed area under a curve between two points. It is a fundamental concept in calculus that is used to calculate the accumulated change under a curve over an interval. In the video, the definite integral is used to find the area between the functions of interest over the specified interval [0, 1] and [-2, 2].
๐Ÿ’กExponential functions
Exponential functions are mathematical functions of the form f(x) = a^x, where a is a constant and x is the independent variable. In the video, the concept of exponents is used when discussing the function x to the fifth, which is an example of an exponential function where the base is the variable x.
๐Ÿ’กAlgebraic manipulation
Algebraic manipulation refers to the process of transforming and solving mathematical expressions using the rules of algebra. In the video, algebraic manipulation is used to solve for x when the absolute value of x to the fifth equals 32, revealing that x equals plus or minus 2.
Highlights

The problem of finding the area between two curves is introduced, specifically between the square root of x and x squared from 0 to 1.

A method is proposed to solve the area problem by calculating the area under the square root of x from 0 to 1 and then subtracting the area under x squared.

The concept of indefinite integral is mentioned as a tool to solve the area problem without rewriting the fundamental theorem of calculus.

An alternative approach is suggested, which involves rewriting the problem as the integral of the difference of two functions, square root of x minus x squared.

The antiderivative of the inner expression (square root of x minus x squared) is calculated, resulting in 2/3 x to the 3/2 minus 1/3 x to the 3.

The area between the curves is found to be 1/3 by evaluating the antiderivative at the bounds 0 and 1 and subtracting.

The presenter expresses excitement about the clean, integer result of the area calculation, which contrasts with the expectation of a more complex number.

A second problem is introduced, involving the area between the function f(x) = x to the fifth and a horizontal line at y equals 32.

The function is corrected to f(x) = absolute value of x to the fifth to create a cup-shaped graph.

The area calculation involves finding the points where the absolute value of x to the fifth equals 32, which are x equals plus or minus 2.

The area is found by setting up an indefinite integral from -2 to 2 of 32 minus the absolute value of x to the fifth.

Due to symmetry, the problem is simplified by calculating the area from 0 to 2 and then multiplying by 2.

The integral is expressed as 32x minus x to the sixth over 6, evaluated from 2 to 0.

The final result involves a fraction problem, multiplying the evaluated integral by 2 to get the total area.

The presenter acknowledges the initial function choice was not ideal but continues to work through the problem.

The video ends with an unresolved fraction, leaving the audience to figure out the final numerical value.

Transcripts

Browse More Related Video

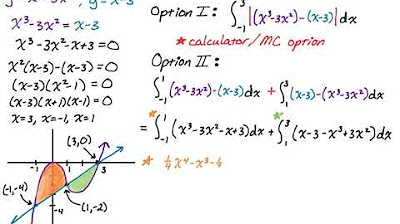
Area Between Two Curves with Multiple Regions: y = x^3-3x^2 and y = x-3

The Definite Integral Part III: Evaluating From The Definition

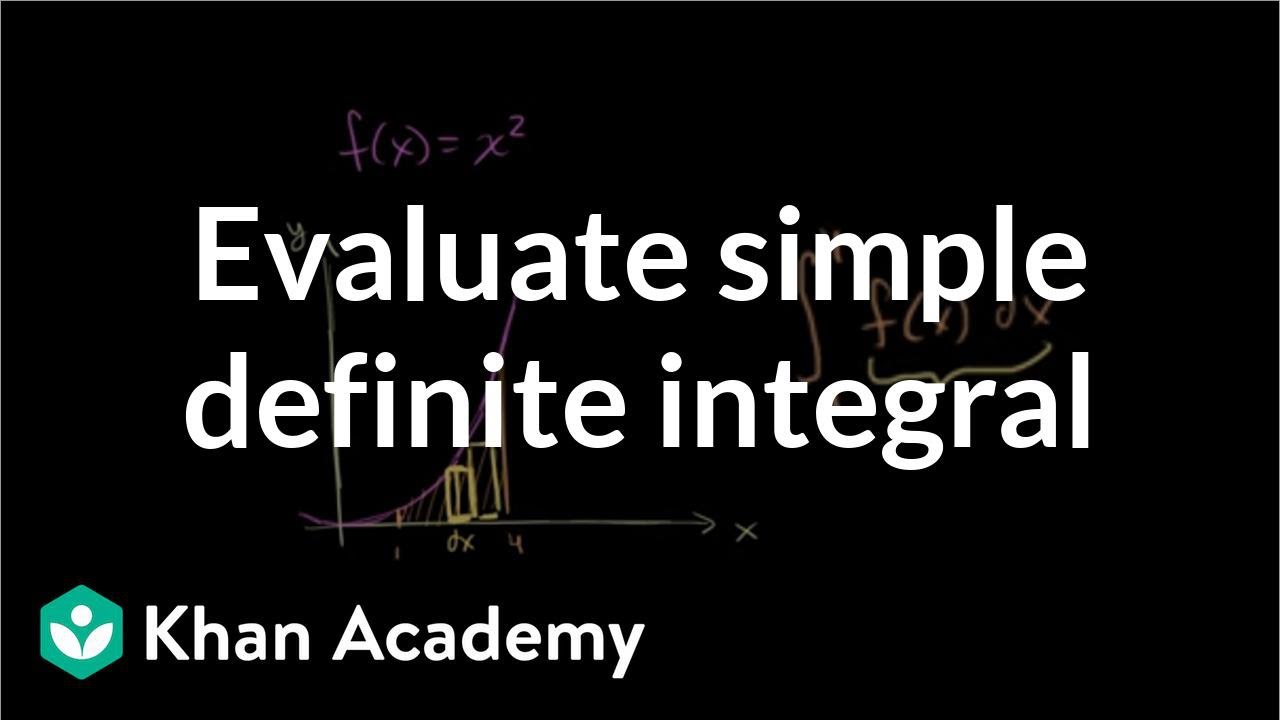
Area between a curve and the x-axis | AP Calculus AB | Khan Academy

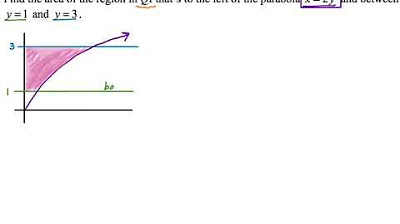
Area Between Curves: Integrating with Respect to y (Example 1)

Area between curves with multiple boundaries

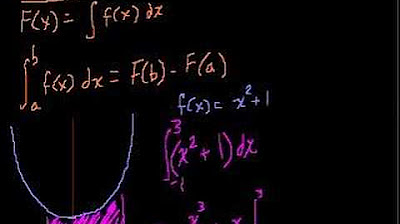
Definite Integrals (part 4)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusArea CalculationSquare RootX to the PowerIntegralsAntiderivativeMathematical Problem SolvingEducational ContentMath IntuitionTrigonometry