Definite Integrals (part 4)

Khan Academy
22 Oct 200709:52
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the application of definite integrals to calculate areas under curves and between curves. It emphasizes the importance of understanding the fundamental theorem of calculus, which connects derivatives and antiderivatives to compute definite integrals. The script provides a step-by-step demonstration of finding the area under a parabola from x=-1 to x=3 and sets up a problem for the next video involving areas between two intersecting curves.

Takeaways
  • πŸ“š The fundamental theorem of calculus is introduced as a basis for calculating definite integrals.
  • 🌟 The concept of antiderivatives is emphasized as crucial for understanding the relationship between derivatives and integrals.
  • πŸ“ˆ The process of finding the area under a curve (specifically, a parabola) using definite integrals is demonstrated.
  • πŸ€” The importance of understanding the intuition behind integrals as the sum of infinitely small rectangles is mentioned.
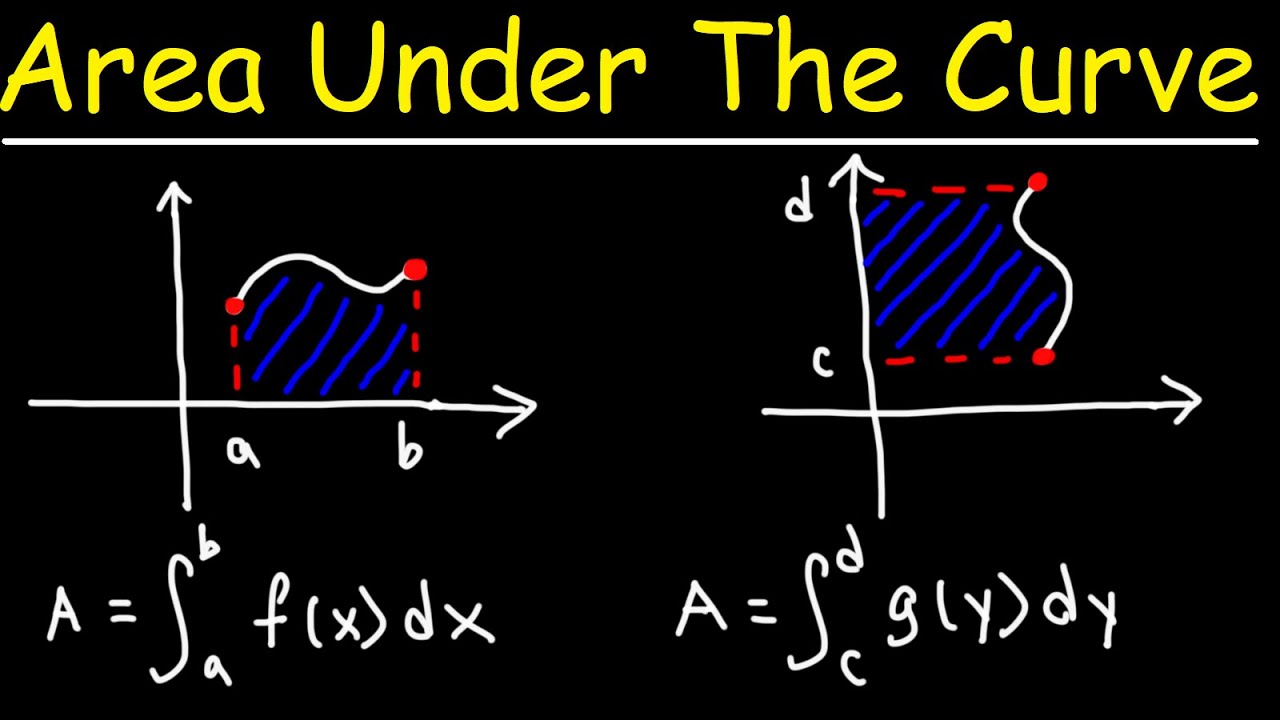
  • πŸ“Š A practical example is provided, calculating the area under the curve y = x^2 + 1 from x = -1 to x = 3.
  • πŸ› οΈ The steps for finding the antiderivative of a given function and applying the fundamental theorem to find the definite integral are outlined.
  • πŸ“Œ The calculation of the area between two curves is introduced, with the example of y = √x and y = x^2.
  • πŸ” The method for finding the intersection points of two functions, which define the boundary for calculating the area between curves, is discussed.
  • πŸ“ The approach for calculating the area between two curves by finding the difference in area under the two curves is suggested.
  • 🎯 The video script serves as an educational tool for illustrating the application of calculus in solving real-world problems.
  • πŸš€ The content is aimed at building a solid foundation in calculus, particularly in the understanding and application of definite integrals.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is the use of definite integrals to find areas under curves and between curves.

  • What is the Fundamental Theorem of Calculus?

    -The Fundamental Theorem of Calculus states that if a function f(x) has an antiderivative F(x), then the definite integral of f(x) from a to b is equal to F(b) minus F(a).

  • Why is it important to understand the Fundamental Theorem of Calculus?

    -Understanding the Fundamental Theorem of Calculus is important because it provides a powerful tool for calculating the areas under curves, which is a key concept in many areas of mathematics and its applications.

  • How does the video demonstrate the process of finding the area under a curve?

    -The video demonstrates the process by first finding the antiderivative of the function, then evaluating the antiderivative at the upper limit of integration and subtracting the value of the antiderivative evaluated at the lower limit.

  • What is the function f(x) used in the example in the video?

    -In the example, the function f(x) used is x squared plus 1.

  • What is the area under the curve of f(x) from x = -1 to x = 3?

    -The area under the curve of f(x) from x = -1 to x = 3 is 12 and 4/3 squared units.

  • How does the video approach the problem of finding the area between two curves?

    -The video suggests finding the area under the top curve and then subtracting the area under the bottom curve to find the area between the two curves.

  • What are the two functions discussed in the video for finding the area between them?

    -The two functions discussed are f(x) = the square root of x and g(x) = x squared.

  • How are the boundary points for the area between the two curves determined?

    -The boundary points are determined by finding where the two functions intersect, which can be done by setting the functions equal to each other and solving for x.

  • What is the significance of the intersection point at (0,0) for the two functions?

    -The intersection point at (0,0) is significant because it is the point where both functions have the same value, and it is one of the boundary points for finding the area between the curves.

  • What is the next step suggested by the video for finding the area between the two curves?

    -The next step suggested is to calculate the area under the top curve and the area under the bottom curve separately between the boundary points, and then subtract the area under the bottom curve from the top curve to find the area between them.

Outlines
00:00
πŸ“š Introduction to Definite Integrals and the Fundamental Theorem of Calculus

This paragraph introduces the concept of using definite integrals to calculate areas under curves, and possibly between curves if time permits. The speaker revisits the fundamental theorem of calculus, emphasizing its importance and providing a brief overview of its application. The explanation includes the relationship between a function and its antiderivative, and the impact of a constant term on the antiderivative. The speaker also clarifies that while there are multiple antiderivatives, the constant terms cancel out when calculating definite integrals, making the choice of antiderivative less critical. To illustrate the concept, the speaker plans to draw a graph and apply the fundamental theorem to a specific function, x squared plus one, to find the area under the curve from x equals negative one to x equals three.

05:01
πŸ“ˆ Calculating Areas Under Curves: A Practical Approach

In this paragraph, the speaker continues the discussion on calculating areas under curves using definite integrals. The focus is on applying the fundamental theorem of calculus to specific examples. The speaker calculates the area under the curve of the function f(x) = x squared plus one, from x equals negative one to x equals three. The process involves finding the antiderivative of the function, evaluating it at the boundary points, and subtracting the results to find the area. The speaker also discusses the concept of areas between two curves, using the functions f(x) = square root of x and g(x) = x squared as examples. The speaker identifies the intersection points of the two functions and suggests calculating the area under each curve separately and then finding the difference to determine the area between the curves. The paragraph concludes with a plan to demonstrate this in the next video due to time constraints.

Mindmap
Keywords
πŸ’‘Definite Integrals
Definite integrals are a fundamental concept in calculus used to calculate the area under a curve within a specified interval. In the video, the presenter aims to use definite integrals to find the areas under various curves, which is central to the theme of understanding the application of calculus in determining such areas. The example given involves finding the area under the curve of the function f(x) = x^2 + 1 from x = -1 to x = 3.
πŸ’‘Fundamental Theorem of Calculus
The Fundamental Theorem of Calculus is a key result that connects differentiation and integration, two main concepts in calculus. It states that if a function is continuous on the interval [a, b] and F'(x) = f(x), then the definite integral of f(x) from a to b is equal to F(b) - F(a). This theorem is crucial in the video as it provides the mathematical foundation for calculating areas under curves using definite integrals.
πŸ’‘Antiderivatives
An antiderivative, also known as an indefinite integral, is a function whose derivative is a given function. In the context of the video, the presenter is looking for antiderivatives of functions like f(x) = x^2 + 1 to apply the Fundamental Theorem of Calculus and find the definite integrals, which in turn helps in determining the areas under the curves.
πŸ’‘Graphing Functions
Graphing functions is the process of visually representing the mathematical relationship between variables on a coordinate plane. In the video, the presenter sketches graphs of functions such as f(x) = x^2 + 1 and g(x) = √x to visually demonstrate the concept of finding areas under and between curves, which is essential for understanding definite integrals and their applications.
πŸ’‘Area Under a Curve
The area under a curve refers to the region enclosed by the curve and the x-axis on a graph. In the video, the main objective is to calculate this area using definite integrals, which is a practical application of calculus in determining the extent of the curve's覆盖 on the x-y plane.
πŸ’‘Parabolas
A parabola is a U-shaped curve defined by a quadratic equation. In the video, the function f(x) = x^2 + 1 is described as a parabola, which is significant because it illustrates the shape of the curve that the presenter is analyzing for the area under it.
πŸ’‘Intersection Points
Intersection points are the values of x where two graphs meet. In the video, the presenter is interested in finding these points for the functions f(x) = √x and g(x) = x^2 to determine the boundaries for calculating the area between the curves.
πŸ’‘Calculus
Calculus is a branch of mathematics that deals with rates of change and accumulation. In the video, calculus is the subject matter, with a focus on teaching the application of definite integrals to find areas under curves, which is a fundamental skill in this mathematical field.
πŸ’‘Derivatives
Derivatives are a central concept in calculus that represent the rate of change of a function with respect to its variable. In the video, the presenter refers to derivatives when discussing antiderivatives and the Fundamental Theorem of Calculus, highlighting the connection between these two concepts.
πŸ’‘Constant Term
The constant term in a function refers to a value that does not change when the variable changes. In the context of the video, the presenter mentions that antiderivatives include a constant term, which is not specified when finding definite integrals because it cancels out when evaluating the difference between two points.
πŸ’‘Square Roots
Square roots are a mathematical operation that finds a number which, when multiplied by itself, gives the original number. In the video, the function g(x) = √x is introduced, and the presenter discusses finding the area between this function and another curve, demonstrating the application of square roots in calculus problems.
Highlights

The use of definite integrals to calculate areas under curves and between curves is discussed.

The fundamental theorem of calculus is written down for better understanding.

It is clarified that f prime of x equals f of x, which means f of x is the antiderivative of f prime of x.

The concept of antiderivatives having a constant term is mentioned, affecting the choice of antiderivative.

The fundamental theorem of calculus is applied to calculate the definite integral from a to b of f of x dx.

The importance of constants canceling out when calculating definite integrals is highlighted.

A graph is drawn to illustrate the calculation of the area under a curve (x squared plus 1) between x equals -1 and x equals 3.

The area under the curve is found to be 12 and 4/3 squared units using the fundamental theorem of calculus.

Another example is introduced with f of x as the square root of x and g of x as x squared to find the area between the two curves.

The intersection points of the two functions (0,0) and (1,1) are determined.

The method to calculate the area between two curves is suggested, which involves finding the area under one curve and subtracting the area under the other.

The process of squaring both sides to find the intersection points of the two functions is briefly mentioned.

The practical application of calculus in calculating areas under curves is emphasized, which was a challenging task before the development of calculus.

The concept of infinitesimals and the sum of small squares with infinitely small bases to approximate areas is referenced.

The mechanical computation of integrals is discussed, emphasizing the process over the deeper understanding.

Transcripts

Browse More Related Video

Finding The Area Under The Curve Using Definite Integrals - Calculus

Math 1325 Lecture 13 2

Business Calculus - Math 1329 - Section 5.3 - The Definite Integral and Fundamental Thm of Calculus

Definite Integrals!

Fundamental theorem of calculus (Part 2) | AP Calculus AB | Khan Academy

Area Between Two Curves with Multiple Regions: y = x^3-3x^2 and y = x-3

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusIntegralsAntiderivativesArea CalculationFundamental TheoremParabolasCurve IntersectionMathematical AnalysisEducational ContentMath Tutorial