INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 25 | BETA FUNCTION SOLVED PROBLEM

TIKLE'S ACADEMY OF MATHS
7 Jul 202217:57
EducationalLearning
32 Likes 10 Comments

TLDRThe video transcript appears to be a lecture on integral calculus, specifically focusing on solving a problem involving the integral of a function from -sin to sin, with a power rule application. The lecturer guides the audience through the process of proving the integral, using the properties of functions and their power rules, and emphasizes the importance of practice for better understanding. The lecture is part of a series, with the current being lecture number 25, and encourages further study and upcoming topics.

Takeaways
  • 📚 The video is a lecture on integral calculus, specifically focusing on the unit of 2013讲师.
  • 🔢 The lecture involves solving a beta function-related integral problem, demonstrating the process step by step.
  • 📈 The problem requires proving two equations, which are integral expressions involving the beta function.
  • 🧠 The讲师 uses the extended form of the beta function to solve the problem, emphasizing the importance of understanding its formula.
  • 🤝 The讲师 guides the audience through the process of substitution and simplification of the integral expressions.
  • 📋 The讲师 highlights the importance of checking the left-hand side of the equation, which contains the beta function of pi.
  • 🔄 The讲师 explains the process of replacing certain elements in the equation, such as shifting 'to' in the multiplication and division.
  • 🌟 The讲师 emphasizes the standard formula for the beta function and its application in solving the problem.
  • 📊 The solution involves simplifying the right-hand side of the equation and comparing it with the left-hand side to prove the integral.
  • 🎓 The讲师 concludes by encouraging the audience to practice and review the problem to ensure understanding of the concepts.
  • 🔍 The video serves as a comprehensive guide for learners to grasp the intricacies of solving integral calculus problems involving the beta function.
Q & A

  • What is the main topic of this lecture?

    -The main topic of this lecture is integral calculus, specifically focusing on solving a problem involving the integral from CO2 side to the power minus one.

  • What is the specific problem being solved in the lecture?

    -The specific problem involves solving the integral from 0 to 2 of the function (2sin^3 x) / (2 - cos(2x)) dx.

  • What is the importance of the Beta function in this context?

    -The Beta function is used in the problem as part of the integrand, and understanding its properties is crucial for solving the integral.

  • How does the讲师 approach the problem?

    -The讲师 approaches the problem by first identifying the integrand, discussing the properties of the Beta function, and then proceeding to solve the integral step by step.

  • What is the role of the power rule in this integral?

    -The power rule is used to simplify the integrand by reducing the power of the sine function, which is crucial for solving the integral.

  • How is the concept of proof by parts utilized in this lecture?

    -Proof by parts is utilized to break down the integral into simpler components that can be solved more easily, and then reassembling the parts to find the solution to the original integral.

  • What is the significance of the substitution method in this context?

    -The substitution method is significant as it allows the讲师 to transform the integral into a more manageable form, facilitating the solving process.

  • What is the final result of the integral as presented in the lecture?

    -The final result of the integral is not explicitly stated in the transcript, but the process leads towards finding the integral of the given function.

  • What advice does the lecturer give for further study?

    -The lecturer advises students to study the Beta function and related integral calculus concepts further, and to practice solving problems like the one discussed in the lecture.

  • How does the讲师 ensure clarity in the explanation?

    -The lecturer ensures clarity by breaking down the problem, explaining each step in detail, and using the substitution and proof by parts methods to simplify the process.

Outlines
00:00
📚 Introduction to Integral Calculus - Lecture 2013

The video begins with an introduction to Integral Calculus, specifically focusing on the 2013 lecture series. The speaker welcomes viewers to the educational channel and sets the stage for solving a problem involving the integral calculus unit. The problem involves proving an equation related to CO2 side oxygen to power and minus one half of the function of end and boy doing to give the function of and for the debit back to the function of and clubs and by two. The speaker emphasizes the importance of understanding the problem statement and prepares the audience for a detailed walkthrough of the solution.

05:02
🔢 Solving the Beta Function Problem

This section delves into solving a specific problem related to the beta function. The speaker explains the use of the beta function's extended formula and how it applies to the given problem. The process involves understanding the form of the beta function, its definition, and how to apply it to prove the equations. The speaker also discusses the importance of the left-hand side and right-hand side of the equation, and how to manipulate them to reach the solution. This part of the lecture is crucial for understanding the application of integral calculus in complex problem-solving scenarios.

10:03
📈 Proof of Definite Integrals and Function of Sin and Cos

The speaker continues with the proof of definite integrals, focusing on the function of sin and cos. The problem involves integrating from 0 to 2 of sin to the power of 2. The speaker explains the standard formula for sin of 2t and how to set it up for the proof. The process includes adjusting the formula, understanding the power of sin, and applying the integral formula correctly. The speaker also discusses the importance of the left-hand side and the right-hand side of the equation and how to balance them to reach the correct solution. This part of the lecture is essential for grasping the concept of definite integrals and their application in calculus.

15:05
🎓 Finalizing the Proof and Moving Forward

In the final part of the lecture, the speaker wraps up the proof of the equations and discusses the next steps in the learning process. The speaker emphasizes the importance of understanding each step of the proof and how it contributes to the overall solution. The speaker also encourages viewers to practice the problems and to look forward to future lectures that will cover more integral calculus topics. The lecture concludes with a reminder to viewers about the importance of consistent practice and the upcoming content in the series.

Mindmap
Keywords
💡Integral Calculus
Integral calculus is a core concept in mathematics that deals with the study of integrals, which are used to find areas under curves, volumes of solids, and other quantities that can be accumulated. In the context of the video, the speaker is discussing a specific problem in integral calculus, likely involving the computation of integrals and their applications.
💡Function
In mathematics, a function is a relation that maps each element of a set (the domain) to exactly one element of another set (the codomain). Functions are fundamental to the video's content as they are used to describe mathematical relationships and are integral to solving calculus problems. The script refers to 'beta function' and 'proof of functions', indicating the importance of functions in the problem at hand.
💡Proof
Proof in mathematics is a logical demonstration that shows a statement to be true. It is a critical part of the video's content as the speaker is working through a problem that requires proving certain mathematical statements or results. The process of proof often involves a series of logical steps and the application of mathematical theorems and axioms.
💡Power
In mathematics, power refers to an exponentiation operation, which is the process of multiplying a number by itself a certain number of times. Powers are used to express how many times a number called the base is multiplied by itself. In the context of the video, the term 'power' is used in various expressions like 'to the power minus one' and 'power to two', indicating that the problem involves exponentiation.
💡Exponent
An exponent is a number that indicates how many times a base is used as a factor in a power expression. Exponents are fundamental in understanding the video's content as they are used to describe the repeated multiplication of a base number. The script mentions 'power to the power minus one', which involves exponentiation and is a key concept in the problem being solved.
💡Beta Function
The beta function is a special function in mathematics that is closely related to the factorial function and is used in various areas of mathematics, including probability theory and combinatorics. In the video, the beta function is mentioned as part of the problem being solved, indicating that it plays a significant role in the mathematical proof or calculation.
💡Proof Method
The proof method refers to the systematic approach used to demonstrate the truth of a mathematical statement or theorem. In the context of the video, the proof method is crucial as the speaker is working through a problem that requires a logical and structured approach to arrive at the solution.
💡Exponential Function
An exponential function is a mathematical function where the base is a constant, and the exponent is the variable. These functions are important in modeling growth and decay processes and are fundamental to calculus. In the video, the term 'exponential' is likely used in reference to the form of the functions being integrated or the nature of the mathematical problem being solved.
💡Power Series
A power series is an infinite series that represents a function as the sum of a sequence of terms calculated from a power of the independent variable. Power series are used to approximate functions and are a key concept in calculus. The video script mentions 'power series' in the context of solving a problem, indicating that the speaker is likely using a power series to approximate or represent a function.
💡Variable
In mathematics, a variable is a symbol that represents a number, letter, or expression that can change. Variables are fundamental to the language of mathematics and are used in the video to denote the changing elements in the mathematical expressions and equations being discussed.
Highlights

The video is a lecture on Integral Calculus, specifically focusing on a problem involving the Beta function.

The problem requires integrating from CO2 side to the power minus one to the point, establishing a foundation for the solution process.

The use of the Beta function in the integral is emphasized, which is a key concept in the problem.

The lecturer introduces the extended formula for the Beta function, which is crucial for solving the integral.

The process of proving the left-hand side of the equation is detailed, involving the use of the Beta function's formula.

The importance of the question's left-hand side is highlighted, as it is the starting point for the proof.

The讲师 explains the need to replace certain elements in the equation to align with the form required for the proof.

The concept of 'shifting' elements from the left to the right side of the equation is discussed, which is a common technique in proofs.

The lecturer emphasizes the importance of the denominator in the function, which is a critical part of the proof.

The process of simplifying the right-hand side of the equation is outlined, which involves several steps.

The lecturer provides a clear explanation of how to handle the power of negative one in the integral, which is a challenging aspect.

The use of the Pythagorean identity in the proof is mentioned, which is a fundamental trigonometric identity.

The final equation after the proof is presented, which is the culmination of the problem-solving process.

The lecturer discusses the second question, which involves a different approach but follows a similar pattern of proof.

The concept of 'moment' is introduced in the context of the integral, adding another layer to the problem.

The lecturer concludes by summarizing the steps taken to solve the problem and encourages further practice.

Transcripts

Browse More Related Video

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 28 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 27 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

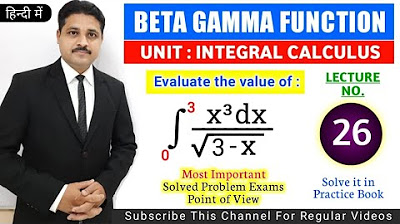
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 26 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

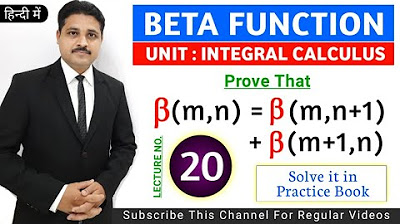
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 20 | BETA FUNCTION SOLVED PROBLEM @TIKLESACADEMY

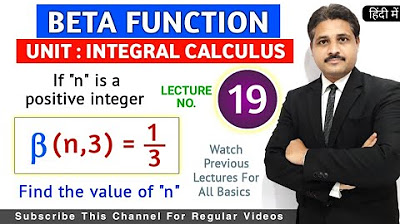
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 19 | BETA FUNCTION SOLVED PROBLEM @TIKLESACADEMY

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 12

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
IntegralCalculusMathLectureComplexProblemSolvingFunctionsExponentsLogarithmsEducationalContentMathematicsOnlineLearningAcademic