INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 27 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

TIKLE'S ACADEMY OF MATHS

4 Aug 202223:35

EducationalLearning

32 Likes 10 Comments

TLDRThe video transcript discusses the process of solving an integral calculus problem involving the Beta function. The speaker guides the audience through converting the given integral into the standard form of the Beta function and then solving it using techniques previously discussed. The problem is from a 2017 lecture on integral calculus, and the solution requires understanding basic concepts of the Beta function, including its definition and properties. The video emphasizes the importance of practice and familiarity with various forms of the Beta and Gamma functions to successfully solve such problems.

Takeaways

📚 The video is a lecture on integral calculus, specifically focusing on solving a problem involving the Beta function and Gamma function.
🔢 The problem given in the script involves integrating from zero to 2x, with a function involving the power of 153 multiplied by a bracketed expression.
📈 The lecture explains the process of converting the given integral into the standard form of the Beta function and then solving it using technical methods previously discussed.
🧮 The Beta function is defined in the script as a mathematical function that is used in the context of the integral, with its standard form and properties explained.
🌟 The process of solving the integral involves converting it into a form that can be easily manipulated using the properties of the Beta function.
🔄 The video emphasizes the importance of understanding the basic concepts of Beta and Gamma functions before attempting to solve such problems.
📊 The lecture demonstrates the step-by-step approach to solving the integral, including the use of brackets, powers, and the application of the Beta function's properties.
🎓 The solution to the problem is shown to be a combination of the Beta function in its standard form and the application of mathematical techniques such as factorization and simplification.
💡 The video highlights the use of mathematical tools and techniques to simplify complex integral problems and arrive at the solution.
📝 The importance of practice and review is stressed, encouraging students to work through similar problems to gain a better understanding of the material.
🔍 The video serves as a resource for students to learn and apply the concepts of Beta and Gamma functions in the context of integral calculus.

Q & A

What is the main topic of the video?
-The main topic of the video is solving an integral calculus problem involving beta functions.
What specific problem is being solved in the video?
-The problem involves integrating a function with a beta function from zero to 2X and then simplifying the result.
What is the definition of a beta function?
-The beta function, denoted as B(x, y), is a function defined as B(x, y) = (x^y * (1 - x)^(y - 1)) / (y! * B(y, x)), where B(y, x) is the beta function of y and x.
How does the video approach the problem-solving process?
-The video approaches the problem-solving process by first converting the given integral into the standard form of the beta function, then simplifying and solving it using technical methods previously discussed.
What is the significance of converting the integral into the standard form of the beta function?
-Converting the integral into the standard form of the beta function is significant because it simplifies the problem and makes it easier to apply the known properties and methods for solving integrals involving beta functions.
How does the video handle the simplification of the problem?
-The video handles the simplification by applying algebraic manipulations, such as factoring out common terms, reducing powers, and using properties of the beta function to simplify the expression.
What is the final solution presented in the video?
-The final solution presented in the video is an expression involving the beta function, powers, and other mathematical terms, which is the result of the integral simplification process.
What is the importance of practicing problems like the one presented in the video?
-Practicing problems like the one presented in the video is important for understanding the properties and applications of the beta function in integral calculus, as well as for developing problem-solving skills in mathematical analysis.
What advice does the video give for students struggling with similar problems?
-The video advises students to always keep their notebooks handy, to practice regularly, and to review the forms and properties of beta and gamma functions to better understand and solve such problems.
How does the video conclude?
-The video concludes by summarizing the problem-solving process, emphasizing the importance of practice, and promising to cover more values and applications of the beta and gamma functions in future videos.

Outlines

00:00

📚 Introduction to Integral Calculus and Problem Solving

The paragraph introduces the viewer to a lecture on Integral Calculus, specifically focusing on the concept of Beta Function and its application in solving a given problem. The speaker welcomes the audience to the educational YouTube channel and sets the stage for a detailed walkthrough of the problem-solving process using the integral calculus unit from the 2017 lecture series. The problem involves solving an integral from zero to 2X with a specific function, and the speaker emphasizes the importance of understanding Beta Functions and their standard form before proceeding with the solution.

05:02

🔢 Conversion of Given Integral and Application of Beta Function

In this segment, the speaker delves into the conversion of the given integral into the standard form of the Beta Function. The process involves understanding the definition of the Beta Function and converting the given integral to match this form. The speaker then proceeds to solve the integral using the techniques previously discussed, highlighting the importance of recognizing the Beta Function's role in the problem-solving process. The explanation includes the conversion of the integral, the use of the Beta Function's properties, and the simplification of the expression to arrive at the final solution.

10:04

📈 Explanation of Beta and Gamma Functions in Detail

This paragraph provides an in-depth explanation of the Beta and Gamma Functions, including their definitions and applications in the context of the problem at hand. The speaker clarifies the relationship between Beta and Gamma Functions and how they can be used to solve complex integrals. The explanation involves the use of mathematical notations and the manipulation of expressions to demonstrate the functions' properties and their role in the overall problem-solving strategy.

15:04

🔄 Transformation and Factorization Techniques in Solving Integrals

The speaker discusses various techniques for transforming and factorizing the integral expressions to simplify the problem. The paragraph covers the use of different mathematical operations such as addition, subtraction, and multiplication to manipulate the integral and reach a solvable form. The speaker also introduces the concept of limits and how they are applied in the context of the integral calculus problem, providing a step-by-step approach to arriving at the final solution.

20:06

🎓 Comprehensive Solution and Understanding of Beta and Gamma Functions

In the concluding part, the speaker provides a comprehensive solution to the problem, emphasizing the application of Beta and Gamma Functions. The speaker also discusses the importance of understanding these functions for solving similar problems in the future. The paragraph wraps up with a final solution and a brief overview of the entire process, encouraging the viewers to practice and familiarize themselves with the concepts discussed in the video.

Mindmap

Keywords

💡Integral Calculus

Integral Calculus is a branch of mathematics that deals with the study of integrals, which are used to find areas under curves, volumes of solids, and other similar quantities. In the context of the video, the speaker is discussing the process of solving an integral calculus problem, indicating that the video's theme revolves around mathematical problem-solving and the application of calculus concepts.

💡Beta Function

The Beta function is a special function in mathematics commonly used in probability theory, statistics, and combinatorics. It is defined as a function of two positive real parameters and is closely related to the Gamma function. In the video, the Beta function is central to the problem being solved, as the speaker is working on converting a given integral into the standard form of the Beta function.

💡Gamma Function

The Gamma function is a generalization of the factorial function to complex numbers and is used extensively in various branches of mathematics, including the Beta function. It is defined for all complex numbers except for the non-positive integers. In the video, the Gamma function is likely related to the definition and properties of the Beta function discussed.

💡Power Functions

Power functions are mathematical functions that have the form f(x) = x^n, where n is a real number. They represent the relationship between variables where one variable is a fixed power of the other. In the video, power functions are likely involved in the expressions and calculations related to the integral calculus problem.

💡Brackets

Brackets are symbols used in mathematics to enclose expressions or groups of elements. They are often used to indicate the order of operations or to set apart certain elements in a formula or equation. In the context of the video, brackets are likely used to structure the mathematical expressions involved in the integral calculus problem.

💡Solving Problems

Solving problems in the context of the video refers to the process of finding solutions to mathematical problems, specifically those related to integral calculus and the Beta function. The video's content is focused on walking through the steps required to solve a complex integral calculus problem, which involves understanding and applying advanced mathematical concepts.

💡Technical Usage

Technical usage refers to the application of specific terminology and methods within a particular field, such as mathematics in this case. In the video, technical usage is evident as the speaker employs mathematical jargon and procedures to solve the integral calculus problem, which requires a deep understanding of the subject matter.

💡Conversion

Conversion in the context of the video refers to the process of transforming a given mathematical expression or function into a different form that is more manageable or easier to work with. The speaker is focused on converting a given integral into the standard form of the Beta function to simplify the problem-solving process.

💡Algebraic Manipulation

Algebraic manipulation involves the use of algebraic rules and techniques to transform and simplify mathematical expressions. In the video, the speaker is likely to use algebraic manipulation to solve the integral calculus problem, which includes steps like expanding, factoring, and combining like terms.

💡Limits

In mathematics, limits are used to describe the behavior of a function or sequence as the input approaches a particular value. They are a fundamental concept in calculus and are used to define continuity, derivatives, and integrals. The video's discussion of integral calculus suggests that limits may be relevant in understanding the behavior of the functions involved.

💡Exponential Functions

Exponential functions are mathematical functions of the form f(x) = a^x, where a is a constant and x is the variable. They describe growth or decay processes and are commonly used in fields like finance, physics, and biology. In the video, exponential functions may be part of the expressions being integrated or used to model certain phenomena within the problem.

Highlights

The video discusses the integral calculus unit, focusing on solving a specific problem involving beta functions.

The problem involves integrating from zero to 2X with respect to a power function and a cube function.

The video introduces the definition of the beta function, which is crucial for solving the problem.

The process of converting the given integral into the standard form of the beta function is explained step by step.

The video demonstrates the use of technical methods previously used in the process to solve the problem.

The importance of understanding basic concepts of beta functions is emphasized for solving such problems.

The video shows how to simplify the problem using properties of beta functions and algebraic manipulations.

The solution involves the use of power rules and the cube root of numbers in the context of the integral.

The video explains how to handle the power of negative one in the context of the integral.

The process of converting the integral into a form that can be solved is detailed, including the handling of brackets and powers.

The video provides a clear example of how to apply the beta function to an integral calculus problem.

The final solution of the problem is presented, showing the application of the learned concepts and methods.

The video concludes by emphasizing the importance of practice and understanding of beta and gamma functions for solving similar problems.

The lecture number-27 is mentioned, indicating a series of lessons on integral calculus and related functions.

The video encourages students to study the upcoming problems in the series for better understanding and practice.

The practical application of theoretical concepts in solving mathematical problems is demonstrated through the video.

Transcripts

Browse More Related Video

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 28 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

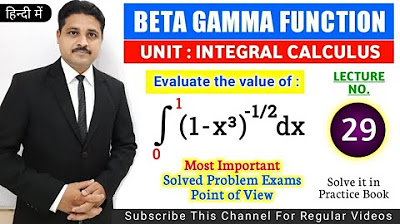
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 29 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

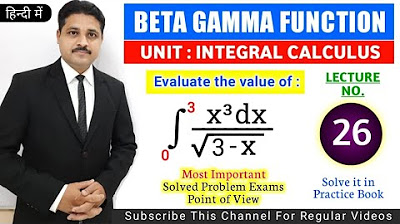
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 26 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

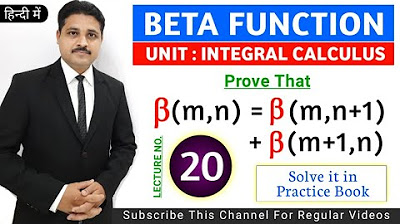
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 20 | BETA FUNCTION SOLVED PROBLEM @TIKLESACADEMY

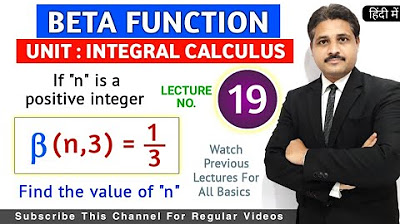
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 19 | BETA FUNCTION SOLVED PROBLEM @TIKLESACADEMY

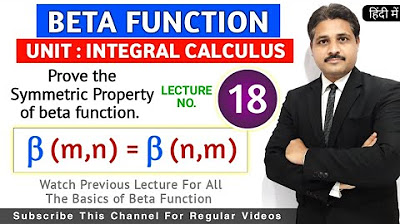
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 18 | BETA FUNCTION SOLVED PROBLEM @TIKLESACADEMY

Related Tags

Mathematics Integral Calculus Beta Functions Gamma Functions Lecture Series Educational Content Problem Solving Math Tutorials Advanced Math Academia