INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 26 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

TIKLE'S ACADEMY OF MATHS
16 Jul 202217:48
EducationalLearning
32 Likes 10 Comments

TLDRThe video lecture introduces the concept of integral calculus, focusing on solving a specific problem involving Beta functions and power functions. It emphasizes the importance of understanding the basic concepts and properties of Beta functions before attempting to solve the problem. The lecture guides the viewer through the process of converting the given problem into a standard form suitable for solving using integral calculus. It also touches on the application of limits and the manipulation of expressions under roots and powers. The video is designed to help viewers build a strong foundation in integral calculus, particularly with Beta functions, and to prepare them for solving more complex problems in future lessons.

Takeaways
  • 📚 The video is a lecture on integral calculus, specifically focusing on the unit related to the integral calculus of 2016.
  • 🔢 The problem solved in the video involves integrating a function with a specific form, which includes a cube under the root of three minus x.
  • 📝 The lecture emphasizes the importance of understanding the basic concepts of beta functions and their properties before attempting to solve the problem.
  • 🌟 The process of solving the integral involves converting the given expression into the standard form of the beta function and then applying the properties of the function.
  • 🛠️ The video provides a step-by-step guide on how to convert the given integral into a form that can be easily solved using the properties of beta functions.
  • 📊 The lecture also discusses the concept of limits in the context of integral calculus, explaining how to handle them when converting expressions and solving integrals.
  • 🔍 The problem-solving approach demonstrated in the video involves simplifying complex expressions by applying the rules of algebra and the properties of the functions involved.
  • 🎓 The importance of practice is highlighted, with the lecturer recommending that viewers review the material covered in previous lectures for a better understanding of the concepts.
  • 📈 The video concludes by solving the integral, demonstrating the application of the learned concepts and the steps involved in the problem-solving process.
  • 💡 The lecture serves as a reminder of the importance of a solid foundation in beta functions and integral calculus for solving more complex problems in the future.
  • 🚀 The video is part of a series on integral calculus, with the promise of more in-depth coverage of related topics in upcoming lessons.
Q & A

  • What is the topic of the lecture in the transcript?

    -The topic of the lecture is the integration of a specific function in calculus, focusing on the application of beta functions and their properties.

  • What type of problem is being solved in the video?

    -The problem being solved involves integrating a function that includes a term under a root and a power function, specifically ∫(23x^(2/3) * dx) from 0 to 100.

  • What is the significance of the beta function in this context?

    -The beta function is crucial in this context as it is used to evaluate the integral of the given function, which is a part of the calculus lecture series.

  • How does the讲师 approach the problem of converting the given function into a standard form?

    -The讲师 approaches the problem by first identifying the components of the function that need to be converted, such as the term under the root and the power function, and then applying the definition of the beta function to transform it into a standard integral form.

  • What is the role of the power rule in solving the integral?

    -The power rule is used to simplify the power function within the integral. It helps in reducing the complexity of the expression and making it easier to apply the beta function.

  • How does the讲师 handle the limits of integration?

    -The讲师 handles the limits of integration by first converting the given function into the form of an integral with specified limits, and then applying the properties of the beta function to evaluate the integral within those limits.

  • What is the final result of the integral as presented in the video?

    -The final result of the integral is not explicitly stated in the transcript. However, the process involves applying the beta function and its properties to simplify and evaluate the integral.

  • Why is it important to understand the properties of the beta function in this lecture?

    -Understanding the properties of the beta function is important because it allows for the simplification and evaluation of complex integrals, which is a key concept in advanced calculus.

  • What advice does the 讲师 give for students who may not have seen previous lectures on beta functions?

    -The 讲师 advises students who have not seen previous lectures on beta functions to review the material to gain a clear understanding of the basic concept, which is essential for comprehending the current lecture.

  • How does the 讲师 ensure that the audience can follow along with the problem-solving process?

    -The 讲师 ensures that the audience can follow along by breaking down the problem into manageable steps, explaining each part of the process, and applying the relevant mathematical properties and formulas.

Outlines
00:00
📚 Introduction to Integral Calculus - Lecture 26

The paragraph introduces the video lecture on Integral Calculus, specifically focusing on Lecture 26. It mentions that the video will cover solving a problem involving the Beta function and its properties. The speaker welcomes the audience to the YouTube channel and sets the stage for the mathematical discussion ahead, emphasizing the importance of understanding basic concepts of Beta functions and their applications in solving integral calculus problems.

05:00
🔢 Beta Function and Limits - Transforming the Given Expression

This paragraph delves into the specifics of the Beta function, its definition, and the process of transforming the given mathematical expression to solve the problem at hand. The speaker explains the need to convert the expression into the standard form of the Beta function and discusses the importance of understanding the lower and upper limits of integration. The paragraph also touches on the concept of factorization and how it aids in simplifying complex expressions within the context of integral calculus.

10:02
📈 Solving the Integral - Applying Function Forms and Properties

The speaker continues with the problem-solving process, applying the properties of the Beta function and its standard form to find the solution. The paragraph outlines the steps taken to simplify the integral, including factorization, application of function forms, and the use of limits. The speaker also emphasizes the importance of recognizing and applying the correct mathematical techniques to arrive at the final answer for the integral.

15:02
🎓 Conclusion - Understanding Beta Functions for Exam Success

Concluding the lecture, the speaker summarizes the importance of comprehending Beta functions and their integral calculus applications, especially for exam success. The paragraph highlights the practical problem solved in the video and encourages viewers to practice such problems regularly. It also mentions that future videos will cover more advanced topics, ensuring a thorough understanding and preparation for the audience.

Mindmap
Keywords
💡Integral Calculus
Integral Calculus is a branch of mathematics that deals with the study of integrals, which are used to find areas under curves, volumes of solid figures, and other similar quantities. In the video, the讲师 (lecturer) progresses through the unit on Integral Calculus, solving a specific problem related to the subject, demonstrating the application of integral concepts and methods.
💡Beta Function
The Beta Function is a special function in mathematics that is used in various areas of analysis and has applications in probability theory, statistics, and combinatorics. In the context of the video, the讲师 discusses the Beta Function in relation to solving an integral calculus problem, emphasizing its importance in the given mathematical context.
💡Lecture
A lecture is an educational presentation where a讲师 (lecturer) imparts knowledge or information on a particular topic to an audience, typically in an academic or educational setting. In the video, the讲师 delivers a lecture on Integral Calculus, teaching viewers about the concepts and techniques involved in this area of mathematics.
💡Problem Solving
Problem solving refers to the process of finding solutions to given problems, often involving critical thinking and the application of relevant knowledge and skills. In the video, the讲师 demonstrates problem-solving skills by working through a mathematical problem related to Integral Calculus, step by step.
💡Mathematical Concepts
Mathematical concepts are the fundamental ideas and principles that form the basis of mathematics. They include definitions, theorems, formulas, and notations that are used to describe mathematical relationships and solve problems. In the video, the讲师 introduces and explains various mathematical concepts necessary for understanding and solving the integral calculus problem presented.
💡YouTube Channel
A YouTube Channel is a user-created service on the YouTube platform where individuals or organizations can upload and share videos on various topics. In the context of the video, the讲师 welcomes viewers to their YouTube Channel, where educational content, specifically on mathematics, is provided.
💡Educational Content
Educational content refers to material that is designed to teach or instruct individuals on various subjects, often with the intent of improving their knowledge or skills. In the video, the讲师 provides educational content in the form of a lecture on Integral Calculus, aiming to enhance the viewers' understanding of mathematical concepts and problem-solving techniques.
💡Functions
In mathematics, a function is a relation that pairs each element from a set (called the domain) with exactly one element from another set (called the codomain). Functions are fundamental to many areas of mathematics and are used to describe relationships between different quantities. In the video, the讲师 discusses functions, particularly the Beta Function, as part of the integral calculus unit.
💡Series
A series in mathematics is the sum of the terms of a sequence, often approaching a limit as the number of terms increases. Series are used to represent infinite processes and are fundamental in calculus and analysis. In the video, the讲师 may refer to series when discussing the summation of terms in the context of integral calculus.
💡Limits
In calculus, limits are used to describe the behavior of a function as the input (or argument) approaches a particular value. Limits are fundamental to understanding continuity, derivatives, and integrals. The讲师 might discuss limits when explaining how to evaluate certain integrals or when determining the behavior of functions within the context of the problem.
Highlights

Introduction to the Integral Calculus unit, focusing on solving a problem related to the Beta Gamma function.

Explanation of the 2016 lecture series on Integral Calculus.

The problem statement involves evaluating an integral with a specific function.

Importance of understanding the Beta function and its properties for solving the problem.

Discussion on the transformation of the given problem into the standard form of the Beta function.

Detailed explanation of the Beta function's definition and its application in problem-solving.

Techniques for converting the given integral into a form that can be addressed using the Beta function.

Methodology for simplifying the integral's limits and the integrand to match the Beta function's form.

Strategies for dealing with the denominator in the integral to facilitate the application of the Beta function.

Use of variable substitution to align the given problem with the standard Beta function form.

Calculation steps to solve the integral using the Beta function's properties.

Final solution of the integral problem by applying the Beta function formula.

Recommendation to view previous videos on the Beta function for a clearer understanding of the concepts.

Importance of practice and understanding the fundamental concepts of the Beta function for solving complex problems.

Closure of the lecture with an emphasis on the importance of the Beta Gamma function in Integral Calculus.

Transcripts

Browse More Related Video

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 27 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

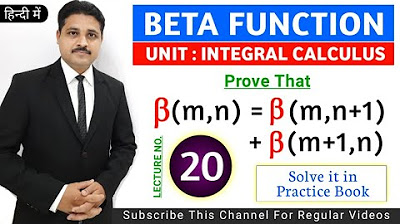
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 20 | BETA FUNCTION SOLVED PROBLEM @TIKLESACADEMY

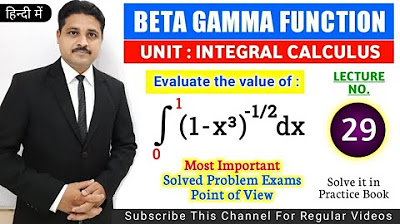
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 29 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

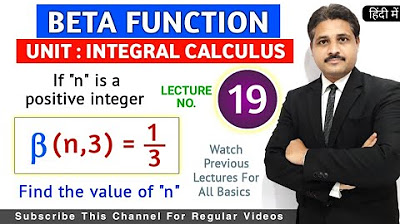
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 19 | BETA FUNCTION SOLVED PROBLEM @TIKLESACADEMY

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 28 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

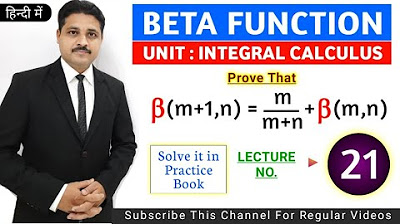
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 21 | BETA FUNCTION SOLVED PROBLEM

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
IntegralCalculusBetaFunctionsMathLectureComplexProblemsLimitsSolvingFactorialsEducationalContentAdvancedMathematicsOnlineLearningMathEnthusiasts