INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 19 | BETA FUNCTION SOLVED PROBLEM @TIKLESACADEMY

TIKLE'S ACADEMY OF MATHS
11 May 202109:22
EducationalLearning
32 Likes 10 Comments

TLDRIn this engaging lecture on integral calculus, the focus is on solving a specific problem involving the beta function. The video welcomes viewers to the 19th lecture of the series and emphasizes the importance of understanding the properties of the beta function, particularly its relationship with the game function. The problem-solving process is detailed, highlighting the use of various functions and their properties to arrive at the solution. The lecture is designed to clear the basic concept of beta functions and is complemented by a previous video on the family of functions. The final answer is derived through a step-by-step calculation, demonstrating the application of knowledge functions and domain expertise in integral calculus.

Takeaways
  • ๐Ÿ“š The video is a lecture on integral calculus, specifically focusing on the Beta function and its properties.
  • ๐Ÿ”ข The problem solved in the video involves finding the value of an integral involving the Beta function of two variables.
  • ๐Ÿ“ˆ The Beta function is related to the Game of Functions, which is a concept used to understand the behavior of functions in integral calculus.
  • ๐ŸŒŸ The 19th lecture of the integral calculus series covers the Beta function and its application to solving the given problem.
  • ๐Ÿ“ The script mentions the importance of understanding the properties of the Beta function, including its relation to the Game of Functions.
  • ๐Ÿค” The problem-solving process requires knowledge of the Beta function's properties and the use of certain mathematical techniques such as factorization.
  • ๐Ÿง  The video emphasizes the importance of practice and reviewing previous lessons to grasp the concepts of Beta functions and their families.
  • ๐ŸŽฅ The video is part of a YouTube channel dedicated to teaching integral calculus and related mathematical concepts.
  • ๐Ÿ‘จโ€๐Ÿซ The่ฎฒๅธˆ uses a clear and step-by-step approach to solve the problem, making it easier for viewers to follow along.
  • ๐Ÿ“Š The video includes a detailed explanation of the formulae and methods used to solve the integral, ensuring a comprehensive understanding of the subject.
  • ๐Ÿ”œ The่ฎฒๅธˆ promises to cover more numbers and royals in upcoming videos, indicating a continuation of the series and a deeper dive into the topic.
Q & A

  • What is the topic of the video lecture?

    -The topic of the video lecture is solving a problem involving Beta functions and Gamma functions in Integral Calculus.

  • Which specific property of the Beta function is important for solving the problem?

    -The important property of the Beta function for solving the problem is its relation to the Gamma function, which is essential in finding the solution.

  • What is the significance of the Gamma function in this context?

    -The Gamma function is significant because it is related to the Beta function, and understanding this relationship is crucial for solving the integral calculus problem presented in the video.

  • How does the speaker introduce the problem?

    -The speaker introduces the problem by presenting an equation involving the Beta function of a negative integer to positive integer by two three, and then proceeds to solve it step by step.

  • What is the role of the edit property in the Beta function?

    -The edit property in the Beta function is used to adjust the argument of the function to fit into a form that can be integrated easily, which is crucial for solving the problem.

  • What is the formula for the Beta function of a and plus one?

    -The formula for the Beta function of a and plus one is not explicitly stated in the script, but it is implied that it involves a relationship with the Gamma function and is used to simplify the integral.

  • How does the speaker ensure the concept of Beta functions is clear?

    -The speaker ensures the concept of Beta functions is clear by referring to a previous special video made for understanding the basics of Beta functions and their family of functions.

  • What is the final result of the problem?

    -The final result of the problem is a value for 'a' that satisfies the condition of being a positive integer, as required by the problem's question.

  • What is the next step suggested by the speaker for further learning?

    -The speaker suggests watching the next videos in the series to cover more numbers of Royals and to have a good practice of the integral calculus involving Beta functions.

  • How does the speaker address the importance of practice in learning Integral Calculus?

    -The speaker emphasizes the importance of practice by encouraging the audience to solve the problem and to look forward to the next videos for more practice with different Royals.

Outlines
00:00
๐Ÿ“˜ Introduction to Integral Calculus and Problem Solving

The paragraph introduces the viewer to a new video on Integral Calculus, specifically focusing on the 19th lecture of the series. The speaker welcomes the audience and sets the stage for solving a problem involving the Beta function. The key points include understanding the properties of the Beta function, its relation to the Game function, and the importance of recognizing the family of functions. The speaker also emphasizes the need to watch a previous video for a clear understanding of the basic concept of the Beta function before proceeding with the problem-solving.

05:02
๐Ÿ”ข Advanced Problem Solving with Beta Function

This paragraph delves into the advanced problem-solving techniques using the Beta function within the realm of Integral Calculus. The speaker guides the viewer through the process of solving a complex equation involving the Beta function, emphasizing the use of various mathematical properties and formulas. The explanation includes the application of factorial functions, the use of domain and range in function definitions, and the manipulation of equations to simplify the problem. The speaker also touches on the importance of understanding the relationship between different functions and how they can be combined or transformed to find the solution. The paragraph concludes with the speaker providing the final answer to the problem and encouraging the viewer to practice these concepts for better understanding in future videos.

Mindmap
Keywords
๐Ÿ’กIntegral Calculus
Integral Calculus is a branch of mathematics that deals with the study of integrals, which are used to find areas under curves, volumes of solid shapes, and other similar quantities. In the video, the speaker is discussing a problem related to integral calculus, indicating that the main theme revolves around solving mathematical problems using this calculus method.
๐Ÿ’กBeta Function
The Beta Function is a special function in mathematics that is used in various areas of analysis and has applications in probability theory, statistics, and combinatorics. In the context of the video, the speaker is likely to be solving a problem that involves the Beta Function, which is a key concept in the topic of integral calculus being discussed.
๐Ÿ’กGame of Functions
The term 'Game of Functions' seems to be a metaphorical or playful way to refer to the process of working with mathematical functions, which are expressions that relate one quantity to another. In the context of the video, it might suggest an engaging and interactive approach to learning about functions, particularly in the realm of integral calculus.
๐Ÿ’กProperties
In mathematics, properties are the characteristics or behaviors that are typical of a given mathematical object or the rules that govern the behavior of an object. In the context of the video, the speaker is emphasizing the importance of understanding the properties of the beta function to solve the integral calculus problem presented.
๐Ÿ’กFactorial
The factorial of a non-negative integer n, denoted by n!, is the product of all positive integers less than or equal to n. Factorials are used in various areas of mathematics, including combinatorics and probability. In the video, the speaker might be using factorials as part of the calculations or the problem-solving process in integral calculus.
๐Ÿ’กDomain
In mathematics, the domain of a function is the set of all possible input values (typically denoted as 'x' values), for which the function is defined. Understanding the domain is crucial for correctly applying and interpreting functions. In the video, the domain is likely discussed in relation to the beta function and how it affects the integral calculus problem.
๐Ÿ’กCoefficients
Coefficients are the numerical factors in front of variables in a mathematical expression or equation. They play a critical role in determining the equation's slope, direction, and other characteristics. In the context of the video, coefficients might be used in the context of polynomials or other algebraic expressions related to the integral calculus problem.
๐Ÿ’กEditing
In the context of the video, 'editing' seems to refer to the process of modifying or adjusting mathematical expressions or equations as part of the problem-solving process. This could involve simplifying expressions, combining like terms, or other algebraic manipulations.
๐Ÿ’กMultiply
Multiplication is one of the four basic arithmetic operations, where two numbers are combined to find their product. In mathematics and especially in the context of the video, multiplying different terms or expressions is a common operation used to solve problems, whether it's to find the area under a curve or to simplify complex expressions.
๐Ÿ’กExponents
Exponents are a way of expressing repeated multiplication. In the context of the video, understanding exponents and their properties is crucial for working with functions and equations, especially when dealing with expressions that involve powers or factors raised to certain powers.
๐Ÿ’กSolve
To solve a mathematical problem means to find the solution or answer to that problem. In the context of the video, the speaker is guiding the viewer through the process of solving a specific problem in integral calculus, which involves understanding and applying various mathematical concepts and techniques.
Highlights

The video is a lecture on integral calculus, specifically focusing on the Beta function and its properties.

The problem to be solved involves the Beta function with parameters equal to positive integers.

Understanding the properties of the Beta function is crucial for solving the problem, including its relation to the Game function.

The lecture is the 19th in a series on integral calculus, indicating a progressive approach to the topic.

The Beta function is introduced with its basic concept, preparing the audience for the more complex problem-solving ahead.

The problem statement is presented, involving the Beta function of entry and the relation to positive integers.

The solution process requires knowledge of the properties of the Beta function and its relation to the Game function.

The use of the definition of the Beta function and the domain of the function is emphasized for problem-solving.

The video encourages viewers to watch previous special videos on the Beta function for a clearer understanding of its concept.

The problem-solving process involves the use of the formula for the Beta function and its application to the given parameters.

The importance of the knowledge of the domain and the function of the Beta function in solving the problem is highlighted.

The video demonstrates the step-by-step process of solving the problem, including the use of the formula and the properties of the Beta function.

The solution involves the use of the product and domain knowledge of the Beta function to arrive at the final answer.

The final answer to the problem is presented, showcasing the application of integral calculus and the Beta function.

The video concludes with an encouragement to practice the problem and to look forward to upcoming videos on related topics.

The lecture series is designed to provide a comprehensive understanding of integral calculus, with a focus on practical problem-solving skills.

The video serves as a resource for those looking to deepen their knowledge of integral calculus and the Beta function.

Transcripts

Browse More Related Video

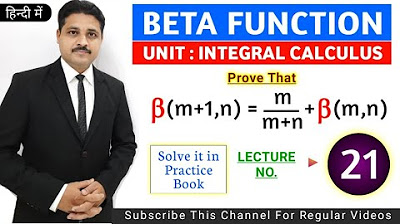
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 21 | BETA FUNCTION SOLVED PROBLEM

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 28 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

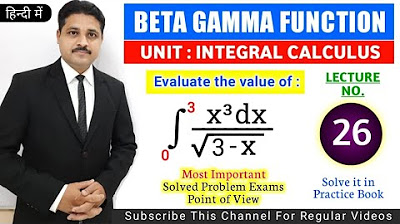
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 26 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 27 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

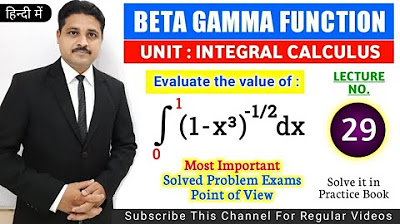
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 29 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

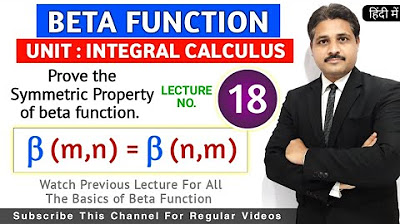
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 18 | BETA FUNCTION SOLVED PROBLEM @TIKLESACADEMY

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Integral CalculusBeta FunctionsMathematics EducationYouTube LecturesProblem SolvingFunction PropertiesInteractive LearningSeries RecapMathematical ConceptsEducational Content