INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 17 | BETA FUNCTION FORMULAS @TIKLESACADEMY

TIKLE'S ACADEMY OF MATHS
15 Apr 202105:36
EducationalLearning
32 Likes 10 Comments

TLDRThe video script introduces the concept of the Beta function, its definition, and basic study. It discusses the properties and important relationships of the Beta function, including its symmetric property and relation to other functions. The script also touches on the integral calculus unit and how the Beta function is applied within it, emphasizing the need to understand its properties and relationships for a clear grasp of the concept. The video aims to deepen the audience's knowledge of the Beta function and its significance in mathematical analysis.

Takeaways
  • πŸ“š The script introduces the concept of the Beta function, emphasizing its importance in mathematics.
  • πŸ”’ The Beta function is defined as a symmetric function with respect to its parameters, often denoted by B(x, y).
  • πŸ“ˆ The script discusses the integral definition of the Beta function, highlighting its calculation from 0 to 1.
  • πŸ“Š The Beta function has certain properties and important relationships that are crucial for understanding its applications.
  • πŸ” The first property mentioned is the symmetry property of the Beta function, where the roles of x and y can be interchanged without affecting the result.
  • πŸ“Œ The second property involves the relationship between the Beta function and other functions, such as the Gamma function and the multiplication and division of parameters.
  • πŸ“ˆ The script also covers important relationships, such as the total differential relation and the relationship between the Beta function and the power function.
  • πŸ“š The concept of limits is discussed in relation to the Beta function, particularly the limit as x approaches 0 and infinity.
  • πŸ“ The script emphasizes the importance of checking the condition for the applicability of the Beta function formula before using it.
  • πŸŽ“ The video aims to clarify the concept of the Beta function, its definition, properties, and important relationships through further examples and explanations.
  • πŸ”œ Upcoming videos will delve deeper into the properties and relationships of the Beta function, providing a comprehensive understanding of its mathematical applications.
Q & A

  • What is the definition of the Beta function?

    -The Beta function, denoted by B(x, y), is a mathematical function defined as the integral of a product of two gamma functions along a line segment in the complex plane. It is related to the gamma function and is often used in probability theory and statistics.

  • How is the Beta function related to the Gamma function?

    -The Beta function is closely related to the Gamma function. Specifically, the Beta function B(x, y) can be expressed in terms of the Gamma function as B(x, y) = Ξ“(x)Ξ“(y) / Ξ“(x+y), where Ξ“ is the Gamma function.

  • What are the properties of the Beta function that were discussed in the script?

    -The script discussed two main properties of the Beta function: the symmetric property and the relation between the Beta function and other functions. The symmetric property states that B(x, y) = B(y, x), while the relation to other functions involves the integral and limit of the Beta function with respect to real numbers.

  • What is the condition for the applicability of the Beta function formula?

    -The condition for the applicability of the Beta function formula is that a certain parameter, denoted as 'a' in the script, must be greater than 0. This condition ensures that the integral converges and the formula can be used.

  • How does the Beta function relate to integration and limits?

    -The Beta function is defined in terms of an integral from 0 to 1 of a product of two functions, which involves limits as one of the variables approaches 0 and the other approaches 1. The limits are crucial in determining the value of the Beta function for given parameters.

  • What is the significance of the Beta function in probability and statistics?

    -The Beta function is significant in probability and statistics because it is used in the definition of the beta distribution, which is a continuous probability distribution often used in Bayesian statistics and finance for modeling random variables that are bounded between 0 and 1.

  • What are the two important relations mentioned in the script regarding the Beta function?

    -The two important relations mentioned are the total difference relation and the relation between the Beta function and the product of two functions. The total difference relation involves the difference of two Beta functions, while the second relation connects the Beta function with the product of two functions involving limits.

  • How does the script suggest improving the understanding of the Beta function?

    -The script suggests creating special videos to further prove the properties and relations of the Beta function, which would help clarify the concepts and deepen the understanding of the function's applications and behavior.

  • What is the role of the script in the educational content about the Beta function?

    -The script serves as a foundational guide for understanding the Beta function, its definition, properties, and important relations. It sets the stage for more detailed exploration in future educational content, including special videos and further mathematical proofs.

  • What is the Beta function's relation number three as mentioned in the script?

    -The Beta function's relation number three, as mentioned in the script, involves the Beta function of a product and a quotient of two functions. It discusses the integration from 0 to 1 and the limits of the function as the quotient approaches specific values.

Outlines
00:00
πŸ“š Introduction to Beta Functions and Properties

This paragraph introduces the topic of Beta functions, emphasizing their importance in mathematics. It begins with a definition of the Beta function, explaining it as a special function denoted by 'B'. The script then delves into the basic study of Beta functions, discussing their definition, properties, and important relationships. It sets the stage for a deeper exploration of Beta functions, hinting at upcoming topics such as integral calculus units and the addition of new topics to the existing knowledge base.

05:01
πŸ“ˆ Beta Function's Properties and Important Relations

The second paragraph focuses on the properties and important relations of Beta functions. It discusses the symmetric property of 'B' functions and how the argument can change interest rates. The script also covers the second property, detailing the relationship between Beta functions and other functions. It mentions the integration from 0 to infinity and its limit, as well as the difference in integration from 0 to infinity and from 2 to infinity. The paragraph concludes by highlighting the importance of understanding these properties and relations for future videos, where the concepts will be further explored and proven.

Mindmap
Keywords
πŸ’‘Beta Function
The Beta Function, often denoted by B(x, y), is a special function in mathematics that plays a crucial role in various areas such as probability theory, statistics, and combinatorics. In the video, the definition and properties of the Beta Function are explored, including its integral representation and its relation to other mathematical functions.
πŸ’‘Integration
Integration is a fundamental concept in calculus that involves finding the area under a curve or the accumulated quantity associated with a function. In the context of the video, integration is used to define the Beta Function and to establish its properties and limits.
πŸ’‘Properties
Properties of a mathematical function describe its behavior under certain conditions or transformations. In the video, properties of the Beta Function are highlighted, such as its symmetry and the relationships it holds with other functions.
πŸ’‘Relations
In mathematics, relations refer to the connections or correspondences between different mathematical objects or functions. The video delves into important relations involving the Beta Function, such as its relation to the incomplete Beta function and its role in integral calculus.
πŸ’‘Limits
Limits are a way to describe the behavior of a function as its input approaches a certain value. In the video, the concept of limits is used to study the behavior of the Beta Function at specific points, such as when x approaches 0 or 1.
πŸ’‘Incomplete Beta Function
The incomplete Beta function is a generalization of the Beta function that involves an integral over a finite interval. It is used in various applications, including statistical analysis and the evaluation of certain types of integrals. The video explores the connection between the incomplete Beta function and the Beta Function.
πŸ’‘Symmetry
Symmetry in mathematics often refers to the balanced arrangement of elements or the property of a function that allows it to look the same after certain transformations. In the video, the symmetry property of the Beta Function is discussed, highlighting how it remains invariant under certain changes in its input.
πŸ’‘Domains and Ranges
Domains and ranges are fundamental concepts in the study of functions. The domain of a function is the set of all possible input values, while the range is the set of all possible output values. In the video, the domains and ranges of the Beta Function and related functions are examined to understand their applicability and behavior.
πŸ’‘Applications
Applications refer to the practical use of a concept or function in solving real-world problems or in theoretical studies. The video touches upon the applications of the Beta Function in various mathematical and statistical contexts, emphasizing its importance and utility.
πŸ’‘Calculus
Calculus is a branch of mathematics that deals with rates of change and accumulation. It is foundational to the study of functions and their properties. In the video, calculus is used to explore the Beta Function's integral representations and its limits.
Highlights

Welcome to the YouTube channel 'Hello Hello Everyone Tips Ecademy of Mathematics' for today's video on Integral Calculus Unit.

Today's topic is the Beta function, and we will explore its basic studies.

The definition of the Beta function is introduced, symbolized as B(x, y) and explained in simple terms.

The formula for the Beta function is discussed, including its domain and range.

The condition for the applicability of the Beta function formula is explained, emphasizing the need for the condition to be met.

Properties of the Beta function are selected and presented, highlighting two main properties.

The first property, symmetric property of B, is explained, allowing for the interchange of variables without affecting the result.

The second property discusses the relationship between the Beta function and other functions, including the function of the minimum and maximum.

Important relations involving the Beta function are outlined, starting with the total differential relation.

The first relation compares the Beta function with a constant, showing the maximum similarity in terms of differentiation.

The second relation involves the integration from 0 to infinity, with specific limits and coefficients.

Differences in the integration limits are noted, highlighting the impact on the function's value.

The Beta proof of a vast integral from 0 to 2 is mentioned, with a tick and scanning center involved.

The power and minimum of the Beta function are examined, with limits added to the discussion.

The relationship number two is explained, adding another layer to the understanding of the Beta function's properties.

The domain of the relation number two is discussed, noting the similarities and differences in the integration from 0 to 2.

The last Beta function relation number three is introduced, involving the integration for 0 to 5 and the subtraction of one data point.

The integration with respect to one is explained, providing clarity on the relationship between the Beta function and integration.

The video concludes with a summary of the basic knowledge of the Beta function, its definition, properties, and important relations, ensuring clarity for the viewers.

Transcripts

Browse More Related Video

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 27 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

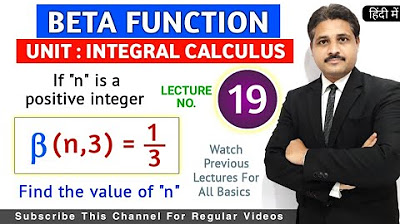
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 19 | BETA FUNCTION SOLVED PROBLEM @TIKLESACADEMY

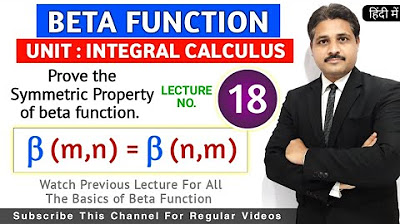
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 18 | BETA FUNCTION SOLVED PROBLEM @TIKLESACADEMY

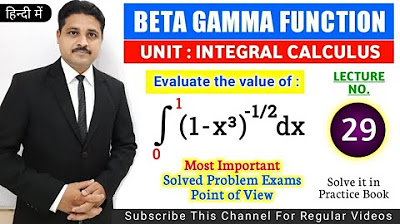
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 29 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

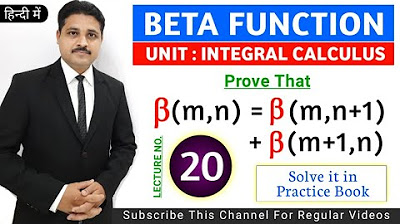
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 20 | BETA FUNCTION SOLVED PROBLEM @TIKLESACADEMY

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 21 | BETA FUNCTION SOLVED PROBLEM

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsCalculusBeta FunctionsIntegral CalculusFunction PropertiesMath TutorialEducational ContentAdvanced MathYouTube ChannelMathematical Concepts