INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 18 | BETA FUNCTION SOLVED PROBLEM @TIKLESACADEMY

TIKLE'S ACADEMY OF MATHS
19 Apr 202109:25
EducationalLearning
32 Likes 10 Comments

TLDRIn this video from the Tips Academy of Maths on YouTube, viewers are welcomed into a comprehensive session on Integral Calculus, focusing on solving a problem related to the Beta function. The instructor methodically demonstrates the process of proving the symmetric property of the Beta function, utilizing definitions and properties previously discussed in the course. Through a step-by-step approach, the video showcases the application of integral calculus techniques to establish the relationship between the Beta functions of different arguments, illustrating the concept with clarity and precision. This educational content is designed to enhance the viewers' understanding of integral calculus, specifically the Beta function, making complex concepts accessible and engaging.

Takeaways
  • 📚 The video is a tutorial on integral calculus, focusing on a specific unit and solving a beta function problem.
  • 🔢 The problem involves finding the integral of the beta function of a given expression, which includes CO2 and other constants.
  • 🎓 The video assumes prior knowledge of beta functions and their properties, suggesting viewers study these concepts if not familiar.
  • 🧠 The concept of 'Proof by Picture' is introduced, using the definition of the beta function to solve the problem.
  • 📈 The video demonstrates the use of integration by substitution and the properties of the beta function to find the solution.
  • 🔄 The process includes identifying and applying the limits of integration, both lower and upper, to the problem at hand.
  • 🔧 The video shows how to set up the problem, including the use of integration techniques and the handling of constants.
  • 📊 The solution involves understanding the symmetric properties of the beta function and how they relate to the problem's limits.
  • 🛠️ The video emphasizes the importance of correctly applying the limits of integration and how they affect the final result.
  • 📝 The final solution is presented, showing the integral of the beta function in its simplified form.
  • 📚 The video concludes by encouraging further study and practice of integral calculus, with more problems to be covered in future videos.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is solving a problem related to the integral calculus unit in mathematics, specifically focusing on the Beta function and its properties.

  • What is the Beta function in mathematics?

    -The Beta function is a generalization of the factorial function and is used in various areas of mathematics including probability theory, statistics, and combinatorics. It is defined as an integral of a certain function and has properties that are useful in mathematical analysis.

  • How does the video approach the problem-solving process?

    -The video approaches the problem-solving process by first presenting the problem, then using the definition of the Beta function and its properties to work towards a solution. It involves understanding the limits of integration and applying mathematical techniques to find the value of the given function.

  • What is the significance of the lower and upper limits in the integral of the Beta function?

    -The lower and upper limits in the integral of the Beta function are crucial as they define the range over which the integration is performed. These limits can affect the value of the integral and thus the result of the function.

  • How does the video explain the concept of limits in integral calculus?

    -The video explains the concept of limits in integral calculus by discussing how they are used to evaluate the integral. It shows how to calculate the lower and upper limits and how these limits can change depending on the function being integrated.

  • What is the role of the substitution method in solving the problem presented in the video?

    -The substitution method is used to simplify the integral by replacing the variables in the function with new variables that make the integration easier. This method is particularly useful when dealing with complex functions, as it can simplify the process of finding the integral.

  • How does the video demonstrate the use of the properties of the Beta function in solving the problem?

    -The video demonstrates the use of the properties of the Beta function by applying them to the given problem. It shows how understanding these properties can help in simplifying the integral and finding the solution more efficiently.

  • What is the importance of understanding the properties of the Beta function in mathematics?

    -Understanding the properties of the Beta function is important as it allows mathematicians and students to solve a wide range of mathematical problems. These properties can simplify complex integrals and provide insights into the behavior of the function under different conditions.

  • How does the video ensure that the audience understands the problem and its solution?

    -The video ensures that the audience understands the problem and its solution by breaking down the process step by step. It starts by presenting the problem, then explains the relevant concepts, and finally applies these concepts to find the solution. The video also provides a clear and concise explanation of each step, making it easier for the audience to follow along.

  • What is the role of practice in learning integral calculus as suggested by the video?

    -The video suggests that practice plays a crucial role in learning integral calculus. By working through a series of problems, students can gain a deeper understanding of the concepts and improve their problem-solving skills. The video also implies that covering more problems in upcoming videos will provide good practice for the audience.

  • What is the final message or advice given by the video to the viewers?

    -The final message given by the video is to ensure that viewers practice the problems covered in the video and look forward to future videos that will cover more problems. This implies that consistent practice and engagement with the material are key to mastering integral calculus.

Outlines
00:00
📚 Introduction to Integral Calculus - Beta Function

This paragraph introduces the topic of Integral Calculus, specifically focusing on the Beta function. It mentions a problem to be solved involving the Beta function and its properties. The speaker welcomes the audience to their YouTube channel and sets the stage for exploring the Beta function's role in Integral Calculus, including its definition and its application to a particular problem. The paragraph emphasizes the importance of understanding the basic concept of the Beta function and its various properties and relations.

05:03
🔢 Solving the Beta Function Integral Problem

The second paragraph delves into the process of solving a specific problem related to the Beta function integral. It discusses the steps to solve the problem, including the use of limits and the properties of the Beta function. The speaker explains the need to adjust the lower and upper limits for the integration and how to handle the resulting expressions. The paragraph also touches on the concept of limits and how they are applied in the context of the Beta function, ultimately aiming to provide a clear solution to the presented problem.

Mindmap
Keywords
💡Integral Calculus
Integral Calculus is a branch of mathematics that deals with the study of integrals, which are used to find the area under a curve or the accumulation of a quantity. In the video, the focus is on solving a problem related to integral calculus, specifically using the properties of beta functions and their integral representations.
💡Beta Function
The Beta function is a special function in mathematics that is often used in probability theory and statistics. It is defined for positive arguments and is related to the Gamma function. In the context of the video, the Beta function is central to the problem being solved, with its definition and properties being key to the solution process.
💡Proof
In mathematics, a proof is a logical demonstration that shows a statement to be true. Proofs are essential in establishing the validity of mathematical theorems and results. The video seems to be focused on providing a proof for a particular property of the Beta function using integral calculus.
💡Limits
Limits are a fundamental concept in calculus that describe the behavior of a function as its input approaches a particular value. In the context of the video, limits are crucial for understanding how the Beta function behaves as its arguments approach certain values, which is essential for the integral calculus problem.
💡Differentiation
Differentiation is the process of finding the derivative of a function, which represents the rate of change of the function with respect to its input. In the video, differentiation might be used to find the derivative of the Beta function or to analyze its properties in relation to the integral calculus problem.
💡Integration
Integration is the reverse process of differentiation and is used to find the original function given its derivative or to calculate the area under a curve. In the video, integration is central to the problem, as it involves finding the integral of the Beta function.
💡Symmetry
Symmetry in mathematics refers to a balanced and harmonious proportion and arrangement of parts. In the context of the video, symmetry might be a property of the Beta function or the integral that is being analyzed, which could simplify the problem-solving process.
💡Lower Limit
The lower limit in the context of integration refers to the starting point of the interval over which the integration is performed. In the video, the lower limit is a specific value that defines the range for the integral of the Beta function.
💡Upper Limit
The upper limit, similar to the lower limit, is the end point of the interval over which an integration is performed. It is crucial in determining the total accumulated quantity represented by the integral.
💡Replacement
In mathematics, replacement refers to the process of substituting one value or expression for another within a given equation or formula. In the video, replacement is likely used to simplify the integral expression or to transform the problem into a more manageable form.
💡Power
In mathematics, power refers to an exponentiation operation, where a number is raised to a certain power. Powers are used to describe multiplication of the same number by itself a specified number of times. In the context of the video, powers might be involved in the expressions that are being integrated or differentiated.
Highlights

Welcome to the Integral Calculus unit on the Hello Hello Everyone Tips Academy YouTube channel.

Today's video will focus on solving a problem involving the Beta function in Integral Calculus.

The problem involves the proof date of the Beta function of Lemn equals to the integral from CO2 extra power minus one to one minus X to the power minus one.

The Beta function's properties and important relations will be used to solve the problem.

The definition of the Beta function is crucial for solving the problem and is provided in the video.

The solution process involves the use of limits and the properties of the Beta function with respect to limits.

The lower and upper limits of the integral are manipulated to find the value of the Beta function.

The concept of limits and their manipulation is key to solving the integral calculus problem.

The video provides a step-by-step approach to solving the integral calculus problem, making it easier for viewers to understand.

The final solution involves the use of the properties of the Beta function and the limits of the integral to prove the equation.

The video emphasizes the importance of understanding the basic concepts of the Beta function for solving such problems.

The problem-solving approach demonstrates the practical application of the Beta function in Integral Calculus.

The video concludes with a summary of the solution and the importance of practicing such problems for better understanding.

The video encourages viewers to study the previous problems created for better practice and understanding of Integral Calculus.

The next video promises to cover more problems of Integral Calculus, providing a comprehensive learning experience.

Transcripts

Browse More Related Video

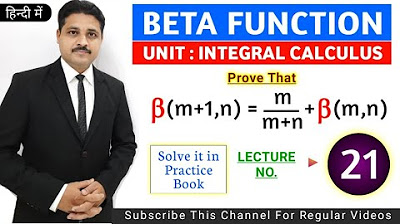
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 21 | BETA FUNCTION SOLVED PROBLEM

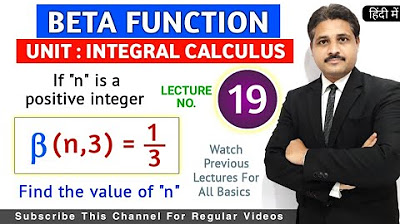
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 19 | BETA FUNCTION SOLVED PROBLEM @TIKLESACADEMY

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 27 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

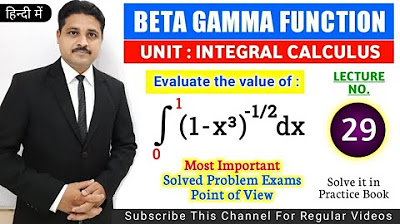
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 29 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

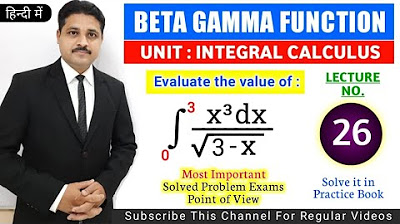
INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 26 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 28 | BETA GAMMA FUNCTION SOLVED PROBLEM IN HINDI

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Integral CalculusBeta FunctionMathematics EducationYouTube TutorialProblem SolvingMathematical AnalysisEducational ContentAdvanced MathOnline LearningAcademic Channel